Write an equation for
Two-thirds a number x plus 8 is 11
Answers
Answer:
2/3x+8 = 11
Step-by-step explanation:
Two-thirds a number x
2/3x
plus 8
2/3x+8
is means equals
2/3x+8 = 11
Related Questions
What is the square root of 30 to two decimal places
Answers
Answer:
30.0
Step-by-step explanation:
Sole this addtion question by substituting numbers for the given letters, to find the value of START Each letter represents a unique digit.
JOAN
+ CAN
______
Answers
HELP I WILL GIVE BRAINLIEST!

Answers
Answer:
I think it's 41°
Step-by-step explanation:
Because the angle represents Tan, by using the inverse of tan and the formula of Opp/Adj, the answer will be 41.08 Which is 41°
I hope This helps! and make me brainliest as you said, PLEASE..
Yo what is 2+2? ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀ ⠀⠀
Answers
Answer:
4
Step-by-step explanation:
Answer:2+2 = 4.............
find the missing variables using substitution

Answers
Answer: x: 1... y: 2... z: -5
Step-by-step explanation:
You need to get z by itself. Divide -4 on both sides and z=-5
Then, get y by itself. Move 6x... and -3y=-6x (the zero doesn't matter now)... then divide by 3 and... y=2x
Now, plug y and z into the first equation...
-2x + 3(2x)+5(-5)=-21
Now solve!
-2x + 6x -25 = -21
4x - 25 = -21
4x = 4
x = 1
Now, you can plug x into the third equation to find y...
6(1) - 3y = 0
Solve!
6 - 3y = 0
-3y = -6
y = 2
Now we have all of them!
Find the relative maximum and minimum values. f(x,y)=x^2+y^2−16x+8y−6 Select the correct choice below and, if necessary, fill in the answer boxes to complete your choice. A. The function has a relative maximum value of f(x,y)= ___at (x,y)=___ (Simplify your answers. Type exact answers. Type an ordered pair in the second answer box.) B. The function has no relative maximum value. Select the correct choice below and, if necessary, fill in the answer boxes to complete your choice. A. The function has a relative minimum value of f(x,y)=___ at (x,y)=___ (Simplify your answers. Type exact answers. Type an ordered pair in the second answer box.) B. The function has no relative minimum value.
Answers
A. The function has a relative maximum value of f(x,y) = 82 at (x,y) = (8, -4). B. The function has no relative minimum value.
To find the relative extrema of the function, we need to find the critical points where the partial derivatives of the function are equal to zero or do not exist. Taking the partial derivatives with respect to x and y, we have:
∂f/∂x = 2x - 16
∂f/∂y = 2y + 8
Setting these partial derivatives equal to zero, we can solve for x and y:
2x - 16 = 0 => x = 8
2y + 8 = 0 => y = -4
So, the critical point is (8, -4). To determine whether it is a relative maximum or minimum, we can use the second derivative test. Calculating the second partial derivatives:
∂²f/∂x² = 2
∂²f/∂y² = 2
Since both second partial derivatives are positive, the critical point (8, -4) corresponds to a relative minimum. However, the problem statement does not provide any information about the range of the variables x and y, so there could potentially be other points in the domain that yield lower function values.
Therefore, we conclude that the function does not have a relative minimum value.
Learn more about derivatives here: https://brainly.com/question/25324584
#SPJ11
Suppose that an airline uses a seat width of 16. 6in assume men have hip breadths that are normally distributed with a mean of 14. 7in and a standard deviation of 1. 1in Find the probability that if an individual man is randomly selected, his hip breadth will be greater than 16. 6in
Answers
The probability that a aimlessly named man's hipsterism breadth will be lesser than16.6 elevation is roughly0.042, or 4.2 %.
To break this problem, we need to find the probability that a aimlessly named man's hipsterism breadth is lesser than16.6 elevation, given that men's hipsterism breadths are typically distributed with a mean of14.7 elevation and a standard divagation of1.1 elevation.
First, we need to regularize the value of16.6 elevation using the formula z = ( x- μ)/ σ
where x is the observed value,
μ is the mean, and
σ is the standard divagation.
In this case, we have z = (16.6-14.7)/1.1 = 1.727
Next, we need to find the area under the standard normal distribution wind to the right of z = 1.727. We can use a standard normal distribution table or a calculator to find this area. Using a calculator, we have P( Z>1.727) = 0.042
Learn more about Z table deviation at
https://brainly.com/question/15059923
#SPJ4
4. Convert 23 miles per hour to kilometers per minute. (1 mile = 1.609 kilometers)
Answers
Answer:
Ewan ko lng po when you find someone ma mas better less sa kanya
Select the correct answer.
What is the end behavior of this radical function?
f(x) = 3√x-4
As x approaches positive infinity, f(x) approaches positive infinity.
As x approaches negative infinity, f(x) approaches positive infinity.
As x approaches positive infinity, f(x) approaches negative infinity.
As x approaches negative infinity, f(x) approaches 0.

Answers
Answer:
The correct answers are:
As x approaches positive infinity, f(x) approaches positive infinity.
As x approaches negative infinity, f(x) approaches 0.
Step-by-step explanation:
Given the discrete uniform population: 1 fix} = E El. elseweltere .x=2.4ifi. Find the probability that a random sample of size 511, selected with replacement, will yield a sample mean greater than 4.1 but less than 4.11. Assume the means are measured to the any level of accuracy. {3 Points}.
Answers
The probability of obtaining a sample mean between 4.1 and 4.11 in a random sample of size 511 is 0.
To calculate the probability that a random sample of size 511, selected with replacement, will yield a sample mean between 4.1 and 4.11 in a discrete uniform population with x = 2.4, we can use the properties of the sample mean and the given population.
In a discrete uniform population, all values are equally likely. Since the mean of the population is x = 2.4, it implies that each value in the population is 2.4.
The sample mean is calculated by summing all selected values and dividing by the sample size. In this case, the sample size is 511.
To find the probability, we need to calculate the cumulative distribution function (CDF) for the sample mean falling between 4.1 and 4.11.
Let's denote X as the value of each individual in the population. Since X is uniformly distributed, P(X = 2.4) = 1.
The sample mean, denoted as M, is given by M = (X1 + X2 + ... + X511) / 511.
To find the probability P(4.1 < M < 4.11), we need to calculate P(M < 4.11) - P(M < 4.1).
P(M < 4.11) = P((X1 + X2 + ... + X511) / 511 < 4.11)
= P(X1 + X2 + ... + X511 < 4.11 * 511)
Similarly,
P(M < 4.1) = P(X1 + X2 + ... + X511 < 4.1 * 511)
Since each value of X is 2.4, we can rewrite the probabilities as:
P(M < 4.11) = P((2.4 + 2.4 + ... + 2.4) < 4.11 * 511)
= P(2.4 * 511 < 4.11 * 511)
Similarly,
P(M < 4.1) = P(2.4 * 511 < 4.1 * 511)
Now, we can calculate the probabilities:
P(M < 4.11) = P(1224.4 < 2099.71) = 1 (since 1224.4 < 2099.71)
P(M < 4.1) = P(1224.4 < 2104.1) = 1 (since 1224.4 < 2104.1)
Finally, we can calculate the probability of the sample mean falling between 4.1 and 4.11:
P(4.1 < M < 4.11) = P(M < 4.11) - P(M < 4.1)
= 1 - 1
= 0
Therefore, the probability that a random sample of size 511, selected with replacement, will yield a sample mean between 4.1 and 4.11 in the given discrete uniform population is 0.
Learn more about Probability click here :brainly.com/question/30034780
#SPJ11
Solve the system of equations using substitution or elimination:
x = 3y + 6
2x - 4y = 8
Answers
Answer:
x=0, y=-2
Step-by-step explanation:
Let x=3y+6 be equation 1
and 2x-4y=8 be equation 2
sub equation 1 into equation2,
2(3y+6)-4y=8
6y+12-4y=8
2y=-4
y=-2
sub y=-2 into equation 1,
x=3(-2)+6
x=0
sub is an abbreviation for substitute
Please help with the first one im confused

Answers
The measure of internal angle m∠V = 103°
What is the sum of the interior angles of a polygon?The sum of the interior angles of a polygon is given by the formula
Sum of Interior angles of a polygon with n sides is
nθ = 180 ( n - 2 )
where n is the number of sides
θ = angle in degrees
Given data ,
Let the sum of interior angles of the polygon be S
Now , the number of sides of the polygon n = 6 sides
So , Sum of Interior angles of a polygon with n sides is
nθ = 180° ( n - 2 )
Substituting the values in the equation , we get
nθ = 180° ( 6 - 2 )
nθ = 180° x 4
nθ = 720°
Therefore , the value of S = 720°
Now , the sum of measures of internal angles inside the polygon is
m∠S = 90°
S = 90° + ( 9x - 19 )° + 111° + ( 5x + 8 )° + 128° + ( 7x + 3 )°
On simplifying the equation , we get
( 9x - 19 )° + ( 5x + 8 )° + ( 7x + 3 )° + 329° = 720°
Subtracting 329° on both sides of the equation , we get
( 9x - 19 )° + ( 5x + 8 )° + ( 7x + 3 )° = 391°
And , on further simplification , we get
21x - 8 = 391
Adding 8 on both sides , we get
21x = 399
Divide by 21 on both sides , we get
x = 19
Now , the measure of m∠V = ( 5x + 8 )°
Substitute the value of x in the equation , we get
The measure of m∠V = ( 5 ( 19 ) + 8 )°
The measure of m∠V = 103°
Hence , the measure of angle m∠V = 103°
To learn more about internal angles of polygon click :
https://brainly.com/question/14611583
#SPJ1
These tables represent a quadratic function with a vertex at (0, -1). What is
the average rate of change for the interval from x = 9 to x = 10?
A. -82
B. -2
C. -101
D. -19
X
0
1
2345
6
y
-1
-2
-5
-10
-17
-26
-37
Interval
0
to 1
1 to 2
2 to 3
3 to 4
4 to 5
5 to 6
Average rate
of change
-1
-3
-5
-7
-9
-11
1-2
J-2
J-2
3-2
1-2

Answers
The average rate of change for the interval from x = 9 to x = 10 is -19
How to determine the average rate of change for the intervalFrom the question, we have the following parameters that can be used in our computation:
The table of values
From the table of values, we have
Rate from 5 to 6 = -11
Also, we have
Common difference = -2
This means that
Rate from 8 to 9 = -11 - 2 * 2 * 2
Evaluate
Rate from 8 to 9 = -19
Hence, the rate is -19
Read more about rate of change at
https://brainly.com/question/31965106
#SPJ1
A) 8= d/p
B) p= 8d
C) d=8p
D)=8/p

Answers
Answer:
B) p= 8d
Step-by-step explanation:
Each day she reads that many pages.
When u multiply 8 x the number of days u get that many pages.
Please give me Brainliest
OMG!! U LIKE BTS!!! AND U LIKE KPOP!!!!
Using the figure below, Maggie used the definition of vertical angles to help her solve for x. Since vertical angles are congruent, she set the two given expressions equal to each other. After solving for x, her teacher said she got the wrong value for x. Explain where Maggie made her mistake when solving for x.

Answers
Maggie's mistake on the solution of the expression for x was when she subtracted 7x to both sides, the result should have been -3x and not 3x.
What is the solution for the equation?The equation is given as follows, as the angles are congruent, that is, they have the same measure:
4x + 2 = 7x - 25.
First, we isolate x on the left side of the equality, subtracting 7x from both sides, hence:
4x + 2 - 7x = 7x - 25 - 7x
Then the correct expression is:
-3x + 2 = -25
Which is where Maggie's mistake happened, on the subtraction of 4x and 7x.
Then, isolating x, we subtract 2 from both sides of the equality, to remove the +2 term on the left side of the equality.
-3x = -27
3x = 27
x = 27/3
x = 9.
The correct solution is x = 9.
More can be learned about solution of equations at https://brainly.com/question/22688504
#SPJ1
Write an equation of the line that passes through the given point and is
perpendicular to the given line.
y = 2/3 x + 1 (3,-2)
Answers
Answer:
since the equation of the line passing through the given point is perpendicular to the given line(m2= -1/m1)
using the formula y=mx+c
y=2/3x +1
m1=2/3
m2= -1/2/3
m2= -3/2
the slope of the second line is -3/2 and it passes through the point(3,-2)
using the formula y-y1 = m(x-x1)
y-(-2)= -3/2(x-3)
y+2= -3/2(x-3)
multiply both sides by 2
2(y+2) = -3(x-3)
2y+4 = -3x+9
2y = -3x+5
y= -3/2x + 5/2
Name: Date: 5. If you paid $300 every month, how many months would it take you to pay off your credit card? (Assuming you don't add any more debt to your credit card.)
Answers
Workers are loading boxes onto a truck. So far, they have loaded 48 boxes onto the truck. According to their inventory, they still need to load 96% of the boxes on the truck. What is the total number of boxes the workers are loading on the truck?
Answers
The total number of boxes of inventory which is loading on the truck by the workers are 1,200 boxes.
What is percentage of a number?
Percentage of a number is the part of the number expressed in the in every hundred. Percentage of a number is expressed with the symbol '%'.
Workers are loading boxes onto a truck . So far, they have loaded 48 boxes onto the truck.
According to their inventory, they still need to load 96% of boxes on the truck. There must be 100% box at the start of the loading. Thus, the percentage of boxes they have load,
p=100-96 = 4%
Thus the percentage of boxes they have load in the truck is 4%. This is equal to the 48 boxes they have loaded.
Let suppose there is x number of total boxes. Thus the 4 percent of x will be equal to the 48.
(4/100)x=48
x = 4800/4 = 1200
Thus, the total number of boxes of inventory which is loading on the truck by the workers are 1,200 boxes.
To learn more about the percentage here;
brainly.com/question/24304697
#SPJ1
at the newest animated movie, for every 9999 children, there are 4444 adults. there are a total of 39393939 children and adults at the movie.
Answers
In this movie, the ratio of children to adults suggests that there are 27 children in attendance.
We have to give that,
There are 9 children for every 4 adults.
This means that if there were 13 people, 9 of them would be children and 4 would be adults.
Children would therefore be:
= 9 / 13
If there were 39 people, the number of children would be:
= 9/13 x 39
= 27 children
In conclusion, there are 27 children.
To learn more about the function visit:
https://brainly.com/question/11624077
#SPJ4
The complete question is,
In the newest animated movie, for every 9 children, there are 4 adults. There are a total of 39 children and adults in the movie.
How many children are in the movie?
A model rocket is launched from the roof of a building. It’s height can be found by using h(t)= -5t^2 + 30t + 9 where h is its height in meters and t is the time after the launch in seconds, as shown in the graph. Find the maximum height of the rocket. Show work

Answers
Answer:
bsidhdurn4yfwrgvbgsudu 7ctwruskdbygdst7fvryrd3qroznrftdyejsnahdurvdbdurh
In a class of students, the following data table summarizes how many students play an instrument or a sport. What is the probability that a student plays a sport given that they play an instrument?
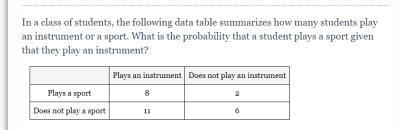
Answers
8 students out of 27
the total of students is 27 out of the 27 students 19 dont play and instrument and a sport
(5.) Verify the first four Euclidean postulates in single elliptic geometry. Hint: Imitate the corresponding proofs of these results in hyperbolic geometry. (See Chapter 7.)
Answers
In elliptic geometry, which is a non-Euclidean geometry, the first four Euclidean postulates are not valid.
However, we can still examine how they are violated and discuss the corresponding proofs in hyperbolic geometry.
1. First Postulate (Postulate of Line Existence):
Euclidean Postulate:
Given any two distinct points, there exists a unique line that passes through them.
Violation in Elliptic Geometry:
In elliptic geometry, any two distinct points do not have a unique line passing through them.
Instead, there are multiple lines that pass through any two points.
Proof in Hyperbolic Geometry:
In hyperbolic geometry, we can prove that given any two distinct points, there are infinitely many lines passing through them.
This can be demonstrated using the Poincaré disk model or the hyperboloid model.
2. Second Postulate (Postulate of Line Extension):
Euclidean Postulate:
Any line segment can be extended indefinitely to form a line.
Violation in Elliptic Geometry:
In elliptic geometry, a line segment cannot be extended indefinitely since the lines in this geometry are closed curves.
Proof in Hyperbolic Geometry:
In hyperbolic geometry, we can show that a line segment can be extended indefinitely by demonstrating the existence of parallel lines that do not intersect.
3. Third Postulate (Postulate of Angle Measure):
Euclidean Postulate:
Given a line and a point not on the line, there exists a unique line parallel to the given line.
Violation in Elliptic Geometry:
In elliptic geometry, there are no parallel lines.
Any two lines will eventually intersect.
Proof in Hyperbolic Geometry:
In hyperbolic geometry, we can prove the existence of multiple parallel lines through a given point not on a line.
This can be achieved by showing that the sum of angles in a triangle is always less than 180 degrees.
4. Fourth Postulate (Postulate of Congruent Triangles):
Euclidean Postulate:
If two triangles have three congruent sides, they are congruent.
Violation in Elliptic Geometry:
In elliptic geometry, two triangles with three congruent sides may not be congruent.
Additional conditions, such as congruent angles, are necessary to determine triangle congruence.
Proof in Hyperbolic Geometry:
In hyperbolic geometry, we can prove that two triangles with three congruent sides are congruent.
This can be demonstrated using the hyperbolic version of the SAS (Side-Angle-Side) congruence criterion.
In summary, in elliptic geometry, the first four Euclidean postulates are not valid, and their corresponding proofs in hyperbolic geometry show how these postulates are violated or modified to fit the geometrical properties of the respective geometries.
Lean more about Euclidean postulates from this link:
https://brainly.com/question/28165056
#SPJ11
a surveyor has been asked to determine the perimeter of a triangular plot of land. The diagram shows the plot with the angle and length measurements that she is able to make. What is the perimeter of the plot of land to the nearest meter
A: 1920m
B: 1825m
C: 1881m
D: 2032m

Answers
Perimeter of the plot of land = 1,920 m.
Perimeter = sum of all the sides of the triangular plot of land.
We only know two of the sides, therefore, we would find the third side length by applying the Law of Cosines, which is:
\(c^2 = a^2 + b^2 - 2ab \times Cos $ C\)
Where,
\(c = $ third $ side $ = ?\\a = 345\\b = 780\\C = 79.8\)
Substitute the values into the formula:
\(c^2 = 345^2 + 780^2 - 2(345)(780) \times Cos $ 79.8\\c^2 = 727,425- 538,200 \times 0.1771\\c^2 = 632,109.78\\c = \sqrt{632,109.78} \\\c = 795.1\)
The third side is 795.1 m long.
Therefore:
Perimeter = \(345 + 780 + 795 = 1,920$ m\)
The perimeter of the plot of land is 1,920 m.
Learn more here:
https://brainly.com/question/17204889
Select the equivalent expression. 2^-4 = ?
Answers
Answer:
1/16
Step-by-step explanation:
This means 1/2x2x2x2 = 1/16
Can one of y’all help me with this pls

Answers
Answer:
D
Step-by-step explanation:
D says four-tenths and 2 times 2 is 4 and 5 times 2 is 10 so 2/5 is equal to 4/10.
- If x = 12, then 2x - 5 = 19.
Converse:
Is the converse true?
Biconditional:
Answers
Answer:
yes
Step-by-step explanation:
the converse is Right
y
The diagram shows part of
regular nonagon. Work
out the size of the angle
marked y.

Answers
Answer: 40 degrees
Step-by-step explanation:
There are 9 sides in a nonagon and 360 degrees make the shape up.
360 degrees divided by 9 EQUAL SIDES of a nonagon = 40 degrees
A cone has volume 3π.
If the cone’s radius is 1, what is its height?
If the cone’s radius is 2, what is its height?
If the cone’s radius is 5, what is its height?
If the cone’s radius is 12, what is its height?
If the cone's radius in r, then what is the height?
Im so confused on how to start
Answers
Answer:
r=1, h=9
r=2, h=2.25
r=5, h=0.36
r=12, h=0.0625
r=r, h=9/r²
Step-by-step explanation:
Volume of a cone = πr²h/3
3π=πr²h/3
r²h=9
when r=1?
r²h=9
1²h=9
h=9
when r=2?
r²h=9
2²h=9
4h=9
h=2.25
when r=5?
r²h=9
5²h=9
25h=9
h=0.36
when r=12?
r²h=9
12²h=9
144h=9
h=0.0625
when r=r?
r²h=9
h=9/r²
The height of a cone is dependent on its radius. The height for a cone radius of 1 is 9.
VolumeVolume is the amount of space that is occupied by a three dimensional object.
The volume of a cone (V) is given by:
V = (1/3)πr²h
Where r is the radius and h is the height.
For a radius of 1:
3π = (1/3)π*(1)² * h
h = 9
For a radius of 2:
3π = (1/3)π*(2)² * h
h = 2.25
For a radius of 5:
3π = (1/3)π*(5)² * h
h = 0.36
For a radius of 12:
3π = (1/3)π*(12)² * h
h = 0.02
The height of a cone is dependent on its radius. The height for a cone radius of 1 is 9.
Find out more on Volume at: https://brainly.com/question/1972490
isaak is writing an explicit formula to represent the sequence. 64, 112, 196, 343, ... what value should he use as the common ratio in the formula? write the answer as a decimal rounded to the nearest hundredth. a) 0.75. b) 1.75. c) 58. d) 279.
Answers
To determine the common ratio for the sequence 64, 112, 196, 343, ... we need to find the ratio between any two consecutive terms.
The ratio between the second and first terms is:
112/64 = 1.75
The ratio between the third and second terms is:
196/112 = 1.75
The ratio between the fourth and third terms is:
343/196 = 1.75
Since the ratios between all consecutive terms are the same, we can conclude that the common ratio for this sequence is 1.75.
Therefore, the answer is (b) 1.75.
To learn more about explicit formula refer below:
https://brainly.com/question/13877261
#SPJ11
In this class, we've been thinking of real-valued functions as vectors. Likewise, we've talked about derivatives aslinear operators ortransformations of these vectors.
Answers
Real-valued functions as vectors and derivatives as linear operators or transformations of these vectors are related. Here, we will discuss this relationship. The derivative of a real-valued function is a vector space. That is, the derivative has the following properties: It is linear; It has a zero vector; It has a negative of a vector.
For example, consider a real-valued function\(, f(x) = 2x + 1\). The derivative of this function is 2. Here, 2 is a vector in the vector space of derivatives. Similarly, consider a real-valued function, \(f(x) = x² + 2x + 1.\)The derivative of this function is 2x + 2.
The vector space of derivatives is closed under addition, which is also a vector in the vector space of derivatives. Furthermore, the vector space of derivatives is closed under scalar multiplication. For example, the product of 2 and\(2x + 2 is 4x + 4,\)which is also a vector in the vector space of derivatives.
To know more about derivatives visit:
https://brainly.com/question/25324584
#SPJ11