Write an equivalent expression for 7 + 5 k minus 2 minus 3 k + n. Which statements are true about the steps for writing the equivalent expression? Check all that apply.
Combine the constant terms by adding 7 and –2.
The equivalent expression is 9 + 3 k.
n, 5 k, and negative 3 k are like variable terms.
Combine the like variable terms by adding the coefficients.
5 k and negative 3 k are like variable terms.
The constants 7 and –2 are like terms.
The equivalent expression is 5 + 2 k + n.
Combine the constant terms by adding 7 and 2.
PLEASE ANSWER ASAP
Answers
Answer: The last one
Step-by-step explanation:
If you combine the like terms, you'll have your simplified expression, which is 2k+5+n
Related Questions
81 + 7x² +8yt-5x
if x = 2 what is the answer
Answers
Heres your answer 109 +8yt -5x
if a password is alphabetic only (all letters) and not case-sensitive, how many possible combinations are there if it has seven characters?
Answers
if the password is alphabetic only, not case-sensitive, and has seven characters, there are a total of \(26^7\) possible combinations.
Since the password is alphabetic only and not case-sensitive, it means that there are 26 possible choices for each character of the password, corresponding to the 26 letters of the alphabet. The fact that the password is not case-sensitive means that uppercase and lowercase letters are considered the same.
For each character of the password, there are 26 possible choices. Since the password has seven characters, the total number of possible combinations is obtained by multiplying the number of choices for each character together: 26 × 26 × 26 × 26 × 26 × 26 × 26.
Simplifying the expression, we have 26^7, which represents the total number of possible combinations for the password.
Therefore, if the password is alphabetic only, not case-sensitive, and has seven characters, there are a total of \(26^7\) possible combinations.
Learn more about combinations here:
https://brainly.com/question/13095958
#SPJ11
In the PE closet, there are 4
soccer balls for every 3 volleyballs. The PE
closet has 24 soccer balls. How many
volleyballs are in the PE closet?
Answers
Answer:
18
Step-by-step explanation:
4/3 is the ratio of soccer balls to volley balls
4/3=24/x
4x=24*3
4x=72
x=18
Answer:
18
Step-by-step explanation:
4/3 is the ratio of soccer balls to volley balls
4/3=24/x
4x=24*3
4x=72
x=18
4x + y = -7 solve for y
Answers
Answer: -7
Step-by-step explanation:
First, simplify the equation and make y isolated.
4x + y = -7
y = -7 - 4x
Plug in 0 for x.
y = -7 - 4(0)
y = -7
Y=-4x-7.
Next plug in 0
Y=-4(0)-7
Now we have
Y=-7
Hope this helps:)
“Write a proof to show that the triangles are congruent.”

Answers
The given triangles ABD and CBD are congruent because their corresponding sides and angles are equal.
What are congruent triangles?Congruent triangles are two triangles that have exactly the same size and shape. In other words, all corresponding sides and angles of the two triangles are equal.
There are several ways to show that two triangles are congruent, including:
Side-Side-Side (SSS) Congruence: If the three sides of one triangle are equal to the three sides of another triangle, then the two triangles are congruent.
Side-Angle-Side (SAS) Congruence: If two sides and the included angle of one triangle are equal to two sides and the included angle of another triangle, then the two triangles are congruent.
Angle-Side-Angle (ASA) Congruence: If two angles and the included side of one triangle are equal to two angles and the included side of another triangle, then the two triangles are congruent.
In the given triangles ABD and CBD, each of their angles is 60⁰, since the triangles are equilateral, so the triangles are congruent.
Learn more about congruent triangles here: https://brainly.com/question/2938476
#SPJ1
Find the y-intercept of the function y = -3(x+2)(x-1)(x+3)
Answers
The y-intercept of a function happens when the function "cuts" the y-axis.
This can be calculated as the value of the function f(x) when x=0.
Then, if we replace x by 0, we get the y-intercept:
\(\begin{gathered} f(x)=-3\mleft(x+2\mright)\mleft(x-1\mright)\mleft(x+3\mright) \\ f(0)=-3(0+2)(0-1)(0+3) \\ f(0)=-3\cdot2\cdot(-1)\cdot3 \\ f(0)=18 \end{gathered}\)The y-intercept for this function is f(0)=18.
What is the quotient when the sum of — 1/2 and -- 3/4 is divided by the product of
5 and -- 1/8?
PLS HELP ITS DUE TOMORROW!!
Answers
When conducting a survey about choosing vacation destinations, Megan should __________ in order to get reluctant respondents to provide honest information.
Answers
When conducting a survey about choosing vacation destinations, Megan should consider using anonymity, confidentiality, or assurance of privacy in order to get reluctant respondents to provide honest information. This can include ensuring that respondents are not required to provide their names or contact information, or guaranteeing that their responses will not be shared with anyone else without their permission.
Additionally, Megan could assure respondents that their responses will be kept confidential, and that their participation in the survey will not have any negative consequences for them. By taking these steps, Megan can encourage reluctant respondents to feel more comfortable sharing their honest opinions and preferences.
Megan should establish rapport and ensure anonymity in order to get reluctant respondents to provide honest information. By creating a comfortable environment and ensuring the respondents that their information will be kept confidential, Megan can increase their willingness to participate and share honest opinions.
Learn more about survey here : brainly.com/question/17373064
#SPJ11
every time these two wheels are spun, two numbers are selected by the pointers. what is the probability that the sum of the two selected numbers is even?
Answers
The probability that the sum of the two selected numbers is even is 1/2.
The parity of the two chosen numbers must match for the sum to be even.
Given this information, we can divide the larger problem into smaller ones. First, we may determine the likelihood that the first spinner will land on an even number.
Exists a \($\frac{1}{2}$\) chance that this happens, and a \($\frac{1}{3}\) there is a chance that the second spinner will also land on an even number, hence
\($\frac{1}{2} \cdot \frac{1}{3} = \frac{1}{6}$\)
There is a win if both the first and second spinners land on an odd number.
\($\frac{1}{2} \cdot \frac{2}{3} = \frac{2}{6}$\)
chance of this happening. Adding these two probabilities together, we get
\(\frac{1}{6} +\frac{2}{6} =\frac{3}{6} =\frac{1}{2}\)
Therefore, The probability that the sum of the two selected numbers is even is 1/2.
Learn more about Probability:
https://brainly.com/question/664351
#SPJ4
A board of directors consists of eight men and four women. A four-member search committee is randomly chosen to recommend a new company president. What is the probability that all four members of the search committee will be women?
Answers
Answer:
Total probability = 0.002 or 1 / 495
Step-by-step explanation:
Given:
Number of men = 8
Number of women = 4
Total number of person = 12
Find:
All four members will be women
Computation:
1st women probability = (4/12)
2nd women probability = (3/11)
3rd women probability = (2/10)
4th women probability = (1/9)
Total probability = (4/12)×(3/11)×(2/10)×(1/9)
Total probability = 24 / 11,880
Total probability = 0.002 or 1 / 495
The probability that all four members of the search committee will be women is 1.42%.
Since a board of directors consists of eight men and four women, and a four-member search committee is randomly chosen to recommend a new company president, to determine what is the probability that all four members of the search committee will be women the following calculation should be performed:
4/8 x 3/7 x 2/6 x 1/5 = X 0.5 x 0.42857 x 0.333 x 0.20 = X 0.01428 = X 100X = 1.42
Therefore, the probability that all four members of the search committee will be women is 1.42%.
Learn more in https://brainly.com/question/15943145
a sign in the elevator of a college library indicates a limit of 16 persons. in addition, there is a weight limit of 2,500 pounds. assume that the average weight of students, faculty, and staff at this college is 155 pounds, that the standard deviation is 29 pounds, and that the distribution of weights of individuals on campus is approximately normal. a random sample of 16 persons from the campus will be selected.
Answers
The probability that a randomly selected group of 16 individuals from the campus will be selected is 0.8023 or 80.23%
Based on the sign in the elevator of the college library, the limit of 16 persons and weight limit of 2,500 pounds need to be adhered to. To ensure compliance with both limits, we need to consider both the number of people and their weight.
Assuming that the distribution of weights of individuals on campus is approximately normal with an average weight of 155 pounds and a standard deviation of 29 pounds, we can use this information to estimate the total weight of a group of 16 randomly selected individuals.
The total weight of a group of 16 individuals can be estimated as follows:
Total weight = 16 x average weight = 16 x 155 = 2480 pounds
To determine if this total weight is within the weight limit of 2,500 pounds, we need to consider the variability in the weights of the individuals. We can do this by calculating the standard deviation of the total weight using the following formula:
Standard deviation of total weight = square root of (n x variance)
where n is the sample size (16) and variance is the square of the standard deviation (29 squared).
Standard deviation of total weight = square root of (16 x 29^2) = 232.74
Using this standard deviation, we can calculate the probability that the total weight of the group of 16 individuals is less than or equal to the weight limit of 2,500 pounds:
Z-score = (2,500 - 2,480) / 232.74 = 0.86
Using a standard normal distribution table or calculator, we can find that the probability of a Z-score less than or equal to 0.86 is approximately 0.8023.
Therefore, the probability that a randomly selected group of 16 individuals from the campus will comply with both the number and weight limits in the elevator of the college library is approximately 0.8023 or 80.23%.
To know more about probability click on below link :
https://brainly.com/question/14210034#
#SPJ11
After Eating at the restaurant, Tom, Jessica, and Melanie decided to divide the bill evenly. If each person paid 22 dollars, what was the total amount of the bill?
Answers
Answer:
$66.00
Step-by-step explanation:
22+22+22=66
Answer:
66
Step-by-step explanation:
because 22+22 is 44 so 44+22 is 66
On average, mary has noticed that 18 trains pass by her house daily (24 hours) on the nearby train tracks. what is the probability that exactly 1 train will pass her house in a 3 hour time period?
Answers
The probability that exactly 1 train will pass Mary's house is 0.00889.
According to the given question.
On average, 18 trains pass by Mary's house daily i.e. in 24 hours.
As, we know that "Probability can be defined as the ratio of the number of favorable outcomes to the total number of outcomes of an event".
Therefore,
The probability that exactly one train will pass Mary's house in a 3 hour period
= \(\frac{^{18C_{1} } }{^{24} C_{3} }\)
\(= \frac{\frac{18!}{1!\times17!} }{\frac{24!}{3!\times 21!} }\)
\(= \frac{18}{\frac{24\times23\times22}{3\times2\times1} }\)
\(=\frac{18}{8\times23\times\ 11}\)
= 18/2024
= 0.00889
Hence, the probability that exactly 1 train will pass Mary's house is 0.00889.
Find out more information about probability here:
https://brainly.com/question/13098829
#SPJ4
Algebra and Geometry Question: Seven of the angles of a decagon have measures whose sum is complementary and exactly two are supplementary. Find the measures of these three angles.

Answers
From the present question, let's say that the missing angles are a, b, and c. We know that the sum of the other seven is equal to 1220°. By definition, the sum of the inner angles of any polygon is given by:
\(S=(n-2)\times180\degree\)In the present case, n = 10. Which means:
\(\begin{gathered} S=(10-2)\times180\degree \\ S=8\times180\degree=1440\degree \\ S=1440\degree \end{gathered}\)From the information given, we are able to say that:
\(\begin{gathered} 1440=1220+a+b+c \\ a+b+c=1440-1220=220 \\ a+b+c=220 \end{gathered}\)Here we found the first equation. The information about supplementary and complementary angles can be written as:
\(\begin{gathered} a+b=90\degree \\ b+c=180\degree \end{gathered}\)Now we have the following system of equations:
\(\begin{gathered} a+b+c=220\degree \\ a+b=90\degree \\ b+c=180\degree \end{gathered}\)If we substitute the second equation in the first one, we are able to find the value for c. Performing this calculation, we find the following:
\(\begin{gathered} (a+b)+c=220\to90+c=220 \\ c=220-90=130\degree \\ c=130\degree \end{gathered}\)With this value, we are able to substitute the value of c in the third equation, and find the value of b, as follows:
\(\begin{gathered} b+c=180\degree\to b+130\degree=180\degree \\ b=180\degree-130\degree=50\degree \\ b=50\degree \end{gathered}\)Now, we can substitute the value of b in the second equation of the system, to find the value of a, as follows:
\(\begin{gathered} a+b=90\degree\to a+50\degree=90\degree \\ a=90\degree-50\degree=40\degree \\ a=40\degree \end{gathered}\)Now, from all the given information, we were able to find that, the three missing angles are:
\(\begin{gathered} a=40\degree \\ b=50\degree \\ c=130\degree \end{gathered}\)Because the question did not name the angles, their name and order are not important, just the values.
let c the curve be parametrized by ()=⟨2−1,−22,4−6⟩.by r(t)=⟨t2−1,t−2t2,4−6t⟩. evaluate ()r(t) at =0,t=0, =1,t=1, and =4.
Answers
Therefore, () r(t) = 4 - 8t evaluated at \(t=0\) is 4, at \(t=1\) is -4, and at \(t=4\)is -28.
To evaluate the dot product ()r(t), we first need to find the coordinates of the vector :
() = ⟨2, -2, 4⟩
Then we can substitute the coordinates of r(t) into the dot product formula:
\(()r(t) = (2t^2 - 2 - 2t^2, -2t^2 - 2t^3, 4 - 6t) ⋅ ⟨2, -2, 4⟩\)
Simplifying this expression yields:
\(()r(t) = 4 - 8t\)
To evaluate () r(t) at different values of t, we substitute those values into the expression we just derived:
\(() r(0) = 4 - 8(0) = 4\)
\(() r(1) = 4 - 8(1) = -4\)
\(() r(4) = 4 - 8(4) = -28\)
To know more about coordinates visit:
https://brainly.com/question/16634867
#SPJ1
What’s number 2 ???
Please help
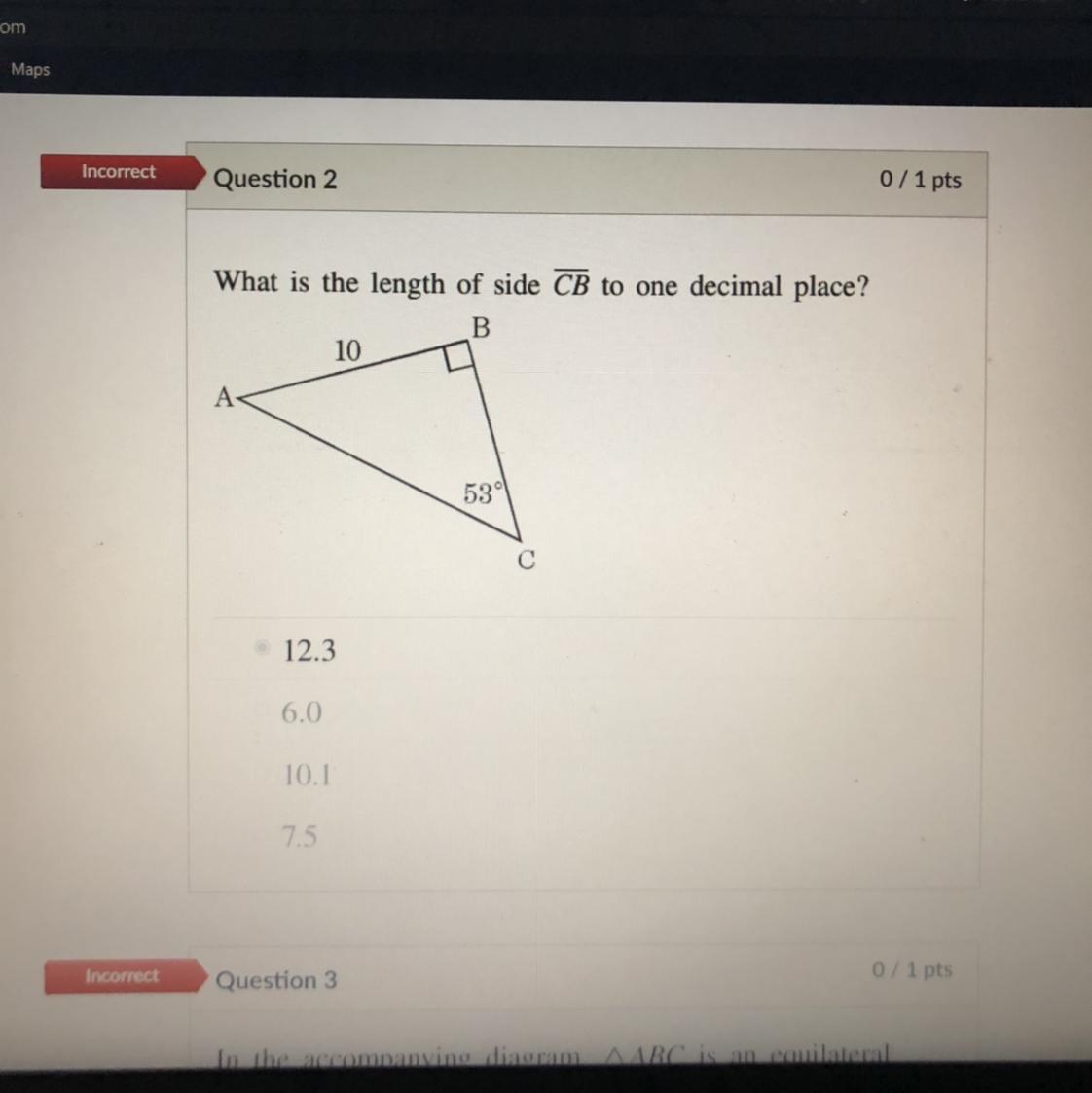
Answers
Answer:
c
Step-by-step explanation:
i think
Answer:
\(CB = 7.5\)
Step-by-step explanation:
Well, if the question gave you a right triangle, an angle, and the side length of the side opposite to that angle, this question should scream (TRIGONOMETRY). So tap into your brain and think about how you can use sin, cosine, and/or tangent to solve the problem.
So notice that the question is asking for the side length of CB, the side adjacent to the angle C (53 degrees). Since we already have the length of the side opposite to C (10), we can use tangent, because we know the value of tan58 if we put it in a calculator, and we know the length opposite to C.
\(tan(53) = 10/CB\)
\(CB * tan(53) = 10\)
\(CB = \frac{10}{tan(53)}\)
\(CB = \frac{10}{1.327} = 7.5359...\)
The base of this prism is [blank] triangle.
an isosceles
a scalene
an equilateral
PLEASE HELP 50 POINTS PLEASE ANSWER I DON'T HAVE MUCH TIME
![The base of this prism is [blank] triangle.an isosceles a scalenean equilateralPLEASE HELP 50 POINTS](https://i5t5.c14.e2-1.dev/h-images-qa/contents/attachments/0LlqyFO80CTzDzugUhv93IJvx3yOozOU.gif)
Answers
Answer:
Scalene triangle
Step-by-step explanation:
A scalene triangle means all three sides have different lengths and all three angles have different measures. (2, 2.5, and 1.5 are all different)
An isosceles has two equal sides and one different side.
An equilateral triangle has three equal sides.
Determine if the following statements are true or false, and explain your reasoning for statements you identify as false.
(a) When comparing means of two samples where n1 = 20 and n2 = 40, we can use the normal model for the difference in means since n2 ? 30.
(b) As the degrees of freedom increases, the T distribution approaches normality.
(c) We use a pooled standard error for calculating the standard error of the difference between means when sample sizes of groups are equal to each other.
Answers
The following statements are true or false: a. False b. True c. True.
What is T-distribution?T-distribution, also known as Student's t-distribution, is a probability distribution that is used in statistical inference for calculating confidence intervals and performing hypothesis tests on small sample sizes. It is similar to the standard normal distribution, but it has heavier tails, which means that it has more probability in the tails and less in the center compared to the normal distribution.
Here,
(a) False. When comparing means of two samples, we need to consider the distribution of the difference in means, which follows a t-distribution rather than a normal distribution. The normal model can be used if the sample sizes are large enough (n1 and n2 both greater than or equal to 30), but this is not the case when n2 is only 40.
(b) True. As the degrees of freedom increase, the T distribution approaches normality. The T distribution becomes closer and closer to the normal distribution as the sample size increases.
(c) True. When the sample sizes of the two groups being compared are equal, we can use a pooled standard error to estimate the standard error of the difference between means. This involves combining the variance estimates from each group into a single estimate of the variance of the population, which allows for a more precise estimate of the standard error of the difference between means.
To know more about T-distribution,
https://brainly.com/question/13574945
#SPJ1
A boat radioed a distress call to a Coast Guard station. At the time of the call, a vector A from the station to the boat had a mignitude of 45.0 km and was directed 15.0∘ east of north. A vector from the station to the point where the boat was later found is A=30.0 km. 15.0∗ north of east. What are the components of the vector from the point where the distress call was made to the point where the boat was found? in other words, what are the components of vector C−B:−A ? x component y component 17.3 km east 35.7 km, south 40.6 km east 51.2 km south 17.3 km, west 51.2 km, south 35.7 km, west 17.4 km, north 40.6 km east 35.7 km north
Answers
The components of the vector from the point where the distress call was made to the point where the boat was found are 17.3 km east and 35.7 km south.
To determine the components of the vector from the point where the distress call was made to the point where the boat was found, we need to subtract the components of vector A from the components of vector B. Vector A is given as 45.0 km at 15.0° east of north, and vector B is given as 30.0 km at 15.0° north of east.
To find the x-component, we need to subtract the east component of vector A (45.0 km * cos(15.0°)) from the east component of vector B (30.0 km * sin(15.0°)). This gives us 17.3 km east.
To find the y-component, we need to subtract the north component of vector A (45.0 km * sin(15.0°)) from the south component of vector B (30.0 km * cos(15.0°)). This gives us 35.7 km south.
Therefore, the components of the vector from the point where the distress call was made to the point where the boat was found are 17.3 km east and 35.7 km south.
Learn more about vector
brainly.com/question/30958460
#SPJ11
The area of a rectangular garden is 38 square meters. The width of
the garden is 4 meters. Find the length of the garden. (Lesson 4.4)
Answers
Answer:
Length = 9.5 mStep-by-step explanation:
area of garden = 38 m²
width = 4 m
find Length
area = L x W
38 = L (4)
L = 38 / 4
L = 9.5 m
9.5x4=9.5
9.5
What is the equation of a line that contains (2,1) and is perpendicular to the line: y=3x-4?
Answers
The equation of a line that contains (2,1) and is perpendicular to the line: y=3x-4 can be calculated as follows:Given the line y=3x-4
Then slope of the line will be; y = mx + b (slope-intercept form)
where m = slope of the lineSince the given line is y=3x-4then, slope of the line will be m=3
Then, slope of the line perpendicular to it will be;Perpendicular slopes are negative reciprocal of each other. Therefore, slope of the line perpendicular to the given line will be:m1 = -1/m= -1/3
The given line passes through (2, 1) which means x=2 and y=1
Substituting these values in point-slope form of equation;y - y1 = m(x - x1)y - 1 = (-1/3)(x - 2)
Multiplying by 3 on both sides to get rid of the denominator;3(y - 1) = -1(x - 2)3y - 3 = -x + 2
Rearranging;3y = -x + 5y = (-1/3)x + 5/3
Therefore, the equation of a line that contains (2,1) and is perpendicular to the line: y=3x-4 is y = (-1/3)x + 5/3.
To know more about perpendicular , visit
https://brainly.com/question/12746252
#SPJ11
The wind speeds throughout the month of April were normally distributed with a mean of 17 mph. What percent of the days had wind speeds between 5
Answers
Answer:38
Step-by-step explanation:
trapezoid ABCD is shown on the graph if the trapezoid is rotated 90 degrees counterclockwise about the origin and the resulting image is translated 6 unit left, what are the coordinates of C

Answers
Answer:
3
Step-by-step explanation:
The coordinates of C'' is \(C''(x,y) = (-7,4)\).
In this question we must rotate every point of the trapezoid ABCD around the origin, which is defined by the following vectorial formula:
\(P'(x,y) = O(x,y) + (r_{OP, x}\cdot \cos \theta - r_{OP,y}\cdot \sin \theta, r_{OP,x}\cdot \sin \theta +r_{OP,y}\cdot \cos \theta)\) (1)
Where:
\(O(x,y)\) - Center of rotation.\(r_{OP}\) - Norm of the vector OP.\(\theta\) - Angle of rotation, in sexagesimal degrees.Later, we apply the following translation:
\(P''(x,y) = P'(x,y) + (-6,0)\) (2)
If we know that \(O(x,y) = (0,0)\), \(r_{OC,x} = 4\), \(r_{OC,y} = 1\), \(\theta = 90^{\circ}\) and \(C(x,y) = (4,1)\), the coordinates of C'' are:
\(C'(x,y) = (0,0) + (4\cdot \cos 90^{\circ}-1\cdot \sin 90^{\circ}, 4\cdot \sin 90^{\circ}+1\cdot \cos 90^{\circ})\)
\(C'(x,y) = (-1, 4)\)
\(C''(x,y) = (-1,4) +(-6,0)\)
\(C''(x,y) = (-7,4)\)
The coordinates of C'' is \(C''(x,y) = (-7,4)\).
We kindly invite to see this question on rigid transformations: https://brainly.com/question/18613109
What is the area of a triangle whose vertices are D(1, 1), E(3, -1), and
F(4, 4)?
Answers
The area of the triangle from the vertices is 3 square units
How to determine the area of the triangle?From the question, we have the following parameters that can be used in our computation:
D(1, 1), E(3, -1), and F(4, 4)
Represent the vertices properly
So, we have
D = (1, 1)
E = (3, -1)
F = (4, 4)
The area of the triangle is calculated using
Area = 0.5 * |Dx(Ey - Fy) + Ex(Dy - Fy) + Fx(Dy - Ey)|
Substitute the known values in the above equation, so, we have the following representation
Area = 0.5 * |1 * (-1 - 4) + 3 * (1 - 4) + 4 * (1 + 1)|
Evaluate the sum of products
Area = 0.5 * |-6|
Remove the absolute bracket
Area = 0.5 * 6
Evaluate
Area = 3
Hence, the area of DEF with vertices is 3 square units
Read more about areas at:
brainly.com/question/22972014
#SPJ1
Question content area top
Part 1
Write an equivalent expression without parentheses. Then simplify the result.
m−(8−3m)
Answers
using exponents, On simplifying the equation, we get =4m+8.
The PEMDAS order of operations must be followed when you want to simplify a mathematical equation without using parenthesis (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction). There are no parenthesis in the expression, so you may start looking for exponents. If it does, first make that simpler.
What is the main objective of simplification?Work simplification is to develop better work processes that boost output while cutting waste and costs.
What does simplifying mean in algebra?Simplifying an expression is the same as solving a mathematical issue. When you simplify an equation, you essentially try to write it as simply as you can. There shouldn't be any more multiplication, dividing, adding, or deleting to be done when the process is finished.
Given equation,
m-(8-3m)
=m-8+3m
=4m+8
To know more about exponents visit:-
brainly.com/question/11709654
#SPJ1
FREE ANSWER!!!!!!!!
Explain the differences between an isometric transformation and a dilation.
An isometric transformation is a transformation in which the image is congruent to the pre-image. Both corresponding sides and corresponding angles are equal. A dilation is a transformation in which the corresponding angles of the image are congruent to the pre-image, but the corresponding sides are proportional. This ratio is determined by a scale factor. Dilations create similar images.
Answers
An isometric transformation is a transformation in which the image is congruent to the pre-image. Both corresponding sides and corresponding angles are equal. A dilation is a transformation in which the corresponding angles of the image are congruent to the pre-image, but the corresponding sides are proportional. This ratio is determined by a scale factor. Dilations create similar images.
Answer:
An isometric transformation is a transformation in which the image is congruent to the pre-image. Both corresponding sides and corresponding angles are equal. A dilation is a transformation in which the corresponding angles of the image are congruent to the pre-image, but the corresponding sides are proportional. This ratio is determined by a scale factor. Dilations create similar images.
Step-by-step explanation:
This is the sample response. Have a nice day <333
Find the 8th term of the geometric sequence 10, -20, 40, ...
Answers
Answer:
-1280
Step-by-step explanation:
10×(-2)= -20
-20×(-2)= 40
4th term: 40×(-2)= -80
5th term: -80×(-2)= 160
6th term: 160×(-2)= -320
7th term: -320×(-2)= 640
8th term: 640×(-2)= -1280
Which of the following is an incorrect description for the number -11/3 A. Negative number B. Rational number C. Repeating decimal D. Terminating decimal
Answers
Answer:
D
Step-by-step explanation:
because -11/3 is a repeating decimal. Terminating decimals are decimals that come to an end.
Finding the surface area of the paraboloid z=1−x2−y2
that lies above the plane z=−4
Answers
The surface area of the paraboloid z = 1 - x^2 - y^2 that lies above the plane z = -4 is 3π√5 square units.
The paraboloid z = 1 - x^2 - y^2 lies above the plane z = -4. To find the surface area of this paraboloid, we need to calculate the area of its intersection with the plane z = -4 and subtract it from the total surface area of the paraboloid.
To find the intersection, we set z = -4 and solve for x and y:
-4 = 1 - x^2 - y^2
x^2 + y^2 = 5
This is the equation of a circle with radius √5 centered at the origin. The area of this circle is π(√5)^2 = 5π.
To find the total surface area of the paraboloid, we use the formula for the surface area of a surface of revolution:
S = 2π∫a^b f(x)√(1 + (f'(x))^2) dx
In this case, we want to revolve the curve z = 1 - x^2 - y^2 around the z-axis. Solving for y in terms of x and z, we get:
y = ±\(\sqrt(1 - x^2 - z)\)
We can now use this formula to calculate f(x):
f(x) = 2sqrt(1 - x^2 + 4)
f'(x) = (-2x) / sqrt(1 - x^2 + 4)
Substituting these into the formula for surface area, we get:
S = 2π∫-√5^√5 2*\(\sqrt(1 - x^2 + 4) / \sqrt(1 - x^2 + 4) dx\)
Simplifying, we get:
S = 4π∫-√5^√5 dx
S = 8π√5
Subtracting the area of the intersection with the plane, we get:
S = 8π√5 - 5π
S = 3π√5
For more such questions on paraboloid
https://brainly.com/question/31477010
#SPJ8
Five years ago, someone used her $40,000 saving to make a down payment for a townhouse in RTP. The house is a three-bedroom townhouse and sold for $200,000 when she bought it. After paying down payment, she financed the house by borrowing a 30-year mortgage. Mortgage interest rate is 4.25%. Right after closing, she rent out the house for $1,800 per month. In addition to mortgage payment and rent revenue, she listed the following information so as to figure out investment return: 1. HOA fee is $75 per month and due at end of each year 2. Property tax and insurance together are 3% of house value 3. She has to pay 10% of rent revenue for an agent who manages her renting regularly 4. Her personal income tax rate is 20%. While rent revenue is taxable, the mortgage interest is tax deductible. She has to make the mortgage amortization table to figure out how much interest she paid each year 5. In last five years, the market value of the house has increased by 4.8% per year 6. If she wants to sell the house today, the total transaction cost will be 5% of selling price Given the above information, please calculate the internal rate of return (IRR) of this investment in house
Can you show the math as far as formulas go?
Answers
Given the following information: Five years ago, someone used her $40,000 saving to make a down payment for a townhouse in RTP. The house is a three-bedroom townhouse and sold for $200,000 when she bought it. After paying down payment, she financed the house by borrowing a 30-year mortgage.
Mortgage interest rate is 4.25%. Right after closing, she rent out the house for $1,800 per month. In addition to mortgage payment and rent revenue, she listed the following information so as to figure out investment return: 1. HOA fee is $75 per month and due at end of each year 2. Property tax and insurance together are 3% of house value 3. She has to pay 10% of rent revenue for an agent who manages her renting regularly 4. Her personal income tax rate is 20%. While rent revenue is taxable, the mortgage interest is tax deductible. She has to make the mortgage amortization table to figure out how much interest she paid each year 5. In the last five years, the market value of the house has increased by 4.8% per year 6.
To know more about interest visit:
https://brainly.com/question/30393144
#SPJ11