Answers
Answer:
{-2, 1}
Step-by-step explanation:
\(\frac{y+4}{2y} +\frac{y-2}{3} =\frac{3y^2+10}{6y}\)
Make the fractions have a common denominator.
\(\frac{3}{3} (\frac{y+4}{2y}) +\frac{x}{y} (\frac{y-2}{3}) =\frac{3y^2+10}{6y}\\\frac{3y+12}{6y} +\frac{2y^2-4y}{6y} =\frac{3y^2+10}{6y}\\\frac{2y^2-y+12}{6y} =\frac{3y^2+10}{6y}\)
Now that both sides of the equation have a common denominator, you can cancel out the denominators.
\(2y^2-y+12=3y^2+10\)
Now, set the equation equal to zero and factor.
\(2y^2-y+12=3y^2+10\\-y^2-y+2=0\\(-y-2)(y-1)=0\\\\-y-2=0\\y=2\\\\y-1=0\\y=-1\)
The y-values are {-2, 1}
Related Questions
Plz plz hurry plz hurry plz hurry
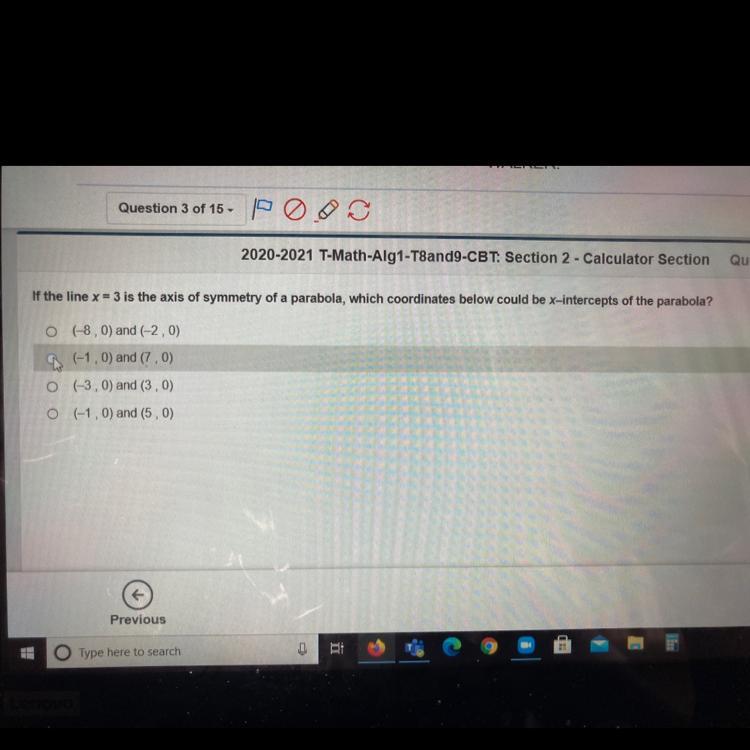
Answers
Answer:
C
I took the same assignment, have a nice day.
A right rectangular box has a volume of 1144 ft.³. The box is 8 feet long and 13 feet high. How wide is the box?
Answers
To solve for the volume of a rectangular prism, we could use the following formula:
\(V=l*w*h\)
V = volumel = lengthw = widthh = heightAlthough we typically use this formula to solve for the volume, we could also use it to determine any of the variables above. All we have to do is rearrange the equation to isolate what we need to find.
Solving the QuestionWe're given:
V = 1144 ft³l = 8 fth = 13We must solve for the width of the boxFirst, rearrange the volume formula to isolate width (w):
\(V=l*w*h\)
⇒ Divide both sides by \(l*h\):
\(\dfrac{V}{l*h}=\dfrac{l*w*h}{l*h}\\\\\dfrac{V}{l*h}=w\\\\w=\dfrac{V}{l*h}\)
⇒ Plug in the given values for V, l and h:
\(w=\dfrac{1144}{8*13}\\\\w=11\)
Therefore, the box is 11 ft wide.
AnswerThe box is 11 ft wide.
Answer:
(A) 11 ft.
Step-by-step explanation:
got it right
What scale factor is used to create a figure with an area of 25 square units from a figure with an area of 16 square units?
Answers
The scale factor of the dilation is k = 5/4
Given data ,
A figure with an area of 25 square units is dilated from a figure with an area of 16 square units
Let the dilation scale factor be k
Now , Given that the original area (A) is 16 square units and the target area (B) is 25 square units, we can write the equation as:
B = k² A
k² = 25/16
Taking square roots on both sides , we get
k = 5/4
Hence , the dilation factor is 5/4
To learn more about dilation click :
https://brainly.com/question/13176891
#SPJ1
According to the eScience Lab Manual for Lab 1, Experiment 3, you need to prepare 65 ml of 60% soda/syrup prescription. To do this you can use the 80% soda solution and the syrup solution (0% strength) that you have in inventory. How many mls of soda solution do you need to create this final solution?
Answers
Answer:
16.25 mL
Step-by-step explanation:
Since we are mixing from the syrup in the inventory, we can say that the syrup in the inventory must be equal to that in the final solution. Thus, we will use the dilution equation to solve for the Initial volume;
Dilution equation is;
V₁C₁ = V₂C₂
Where;
V₁ is initial volume
C₁ is initial concentration or initial molarity
V₂ is final volume
C₂ is final concentration of final molarity
We are given;
C₁ = 80% = 0.8
C₂ = 60% = 0.6
V₂ = 65 mL
Making V₁ the subject in the dilution equation, we have;
V₁ = (V₂C₂)/C₁
V₁ = (65 × 0.6)/0.8
V₁ = 48.75 mL of inventory syrup
Since we have 48.75 mL of inventory syrup and the dilute solution is 65 ml, then the volume of soda solution required = 65mL - 48.75 mL = 16.25 mL
It took Todd 11 hours to travel over pack ice from one town in the Arctic to another town 330 miles away. During the return journey, it took him 15 hours.
Assume the pack ice was drifting at a constant rate, and that Todd’s snowmobile was traveling at a constant speed relative to the pack ice.
What was the speed of Todd's snowmobile?
Answers
Answer:
The speed of Todd's snowmobile was 22 miles an hour
Step-by-step explanation:
:))
The speed of Todd's automobile is 31 miles per hour.
What is speed?Speed is defined as the ratio of the time distance travelled by the body to the time taken by the body to cover the distance. Speed is the ratio of the distance travelled by time. The unit of speed in miles per hour.
Given that It took Todd 11 hours to travel over pack ice from one town in the Arctic to another town 330 miles away. During the return journey, it took him 15 hours.
For the first journey,
v₁ + v₂ = 330 / 11 ......................( 1 )
For the return journey,
v₂ - v₁ = 330 / 15 .........................( 2 )
From equation ( 1 ) and equation ( 2 ),
2v₂ = ( 330 / 11 ) + ( 330 / 15 )
2v₂ = ( 330 ) ( 31 / 165 )
v₂ = 165 ( 31 / 165 )
v₂ = 31 miles per hour
To know more about speed follow
https://brainly.com/question/27384390
#SPJ2
Which of the following is the correct factorization of the polynomial below?
2x^2 - 12x + 18
A. 2(x - 3)^2
B. 2(x + 6)(x + 3)
C. (2x + 6)(x + 3)
D. The polynomial is irreducible.

Answers
A is the answer

Helppppp!! Pleaseeeee

Answers
A bag contains 6 batteries, all of which are the same size and are equally likely to be selected. Each battery is a different brand. If you select 3 batteries at random, use the counting principle to determine how many points will be in the sample space if the batteries are selected
Answers
Answer:
there are 120 possible points in the sample space if we select 3 batteries at random from a bag containing 6 batteries of different brands.
Step-by-step explanation:
The counting principle states that if there are n ways to do one thing and m ways to do another, then there are n x m ways to do both.
In this case, we want to determine how many ways we can select 3 batteries out of 6. Using the counting principle, we can break down the selection process into three steps:
Select the first battery. There are 6 choices for the first battery.
Select the second battery. There are 5 choices left for the second battery, since we've already selected one.
Select the third battery. There are 4 choices left for the third battery.
Using the counting principle, we can multiply the number of choices for each step to get the total number of ways to select 3 batteries:
6 x 5 x 4 = 120
Therefore, there are 120 possible points in the sample space if we select 3 batteries at random from a bag containing 6 batteries of different brands.
Let (-3,-2) be a point on the terminal side of theta. Find the csc(theta)
Answers
The csc(theta) = 1/sin(theta) = 1/(-2/√13) = -√13/2. So, the cosecant of theta is -√13/2.
To find the cosecant (csc) of theta when a point (-3, -2) lies on its terminal side, we need to determine the hypotenuse (r) of the right triangle formed by this point.
Using the Pythagorean theorem, r^2 = (-3)^2 + (-2)^2. So, r^2 = 9 + 4 = 13, and r = √13.
The csc(theta) is the reciprocal of sin(theta). Sin(theta) is calculated as the ratio of the opposite side (y-coordinate) to the hypotenuse, or sin(theta) = -2/√13.
To learn more about : cosecant
https://brainly.com/question/31708994
#SPJ8
What is the value of f(−6) when f(x)=x2+8x+1
Answers
Answer:
-11
Step-by-step explanation:
Notice how
\(f(x)=x^{2} +8x+1\)
So when we have
\(f(-6)=(-6)^{2} +8(-6)+1= 36-48+1=-11\)
The answer is:
f(-6) = -11
Step-by-step explanation:
Plug in -6 for x:
\(\sf{f(x)=x^2+8x+1}\)
\(\sf{f(-6)=(-6)^2+8(-6)+1}\)
Simplify this:
\(\sf{f(-6)=36-48+1}\)
\(\sf{f(-6)=-12+1}\)
\(\sf{f(-6)=-11}\)
∴ f(-6) = -11alex, bella, cara share £90; work how much each of them have if:
alex has £x
bella has 3 times as much as alex
cara has £10 less than alex
Answers
Step-by-step explanation:
given,
alex = x
bella = 3x
cara = x - 10
and also given,
\(x + 3x + (x - 10) = 90 \\ x + 3x + x - 10 = 90 \\ 5x - 10 = 90 \\ 5x = 90 + 10 \\ 5x = 100 \\ x = 100 \div 5 \\ = 20\)
Given x = £20,
Alex = £x = £20
Bella = £3x = £3x20 = £60
Cara = £(x-10) =£(20-10) =£10
A contractor needs to buy nails to build a house. The nails come in small boxes and large boxes. Each small box has 100 nails and each large box has 350 nails. The contractor bought a total of 9 boxes that have 2400 nails altogether. Write a system of equations that could be used to determine the number of small boxes purchased and the number of large boxes purchased. Define the variables that you use to write the system.
Answers
Answer:
3 small boxes and 6 large ones
Step-by-step explanation:
3 times 100 equals 300 and 6 times 350 is 2100 and 2100 plus 300 is 2400.
Find the missing number to make the average correct. The average of 11, 17, 18, and ? is 17.
Answers
Answer:
The missing number is:
22
Step-by-step explanation:
There are 3 numbers and one more
4 numbers and your average is 17
then:
17*4 = 68
then:
11 + 17 + 18 + a = 68
46 + a = 68
a = 68 - 46
a = 22
Check:
(22 + 11 + 17 + 18)/4 = 17
68/4 = 17
Using a standard deck of cards, a gamer drew one card and recorded its value. They continued this for a total of 100 draws. The table shows the frequency of each card drawn.
Card A 2 3 4 5 6 7 8 9 10 JQK
Frequency 4 7 5 6 7 6 8 10 7 10 8 12 10
Based on the table, what is the experimental probability that the card selected was a K or 6?
Answers
The experimental probability that the card selected was a K or 6 is 17/100 or 0.17.
What is probability?
Probability is a measure of the likelihood or chance of an event occurring. It is expressed as a number between 0 and 1, where 0 indicates that an event is impossible and 1 indicates that it is certain. The probability of an event can be calculated by dividing the number of favorable outcomes by the total number of possible outcomes. In other words, it is the ratio of the number of desired outcomes to the total number of outcomes.
The frequency of card 6 is 7 and the frequency of card K is 12. However, the card K is also counted in the total count for JQK, so we need to subtract 2 from the frequency of K to get the actual count of K.
Actual count of K = 12 - 2 = 10
Total count of 6 and K = 7 + 10 = 17
The experimental probability of drawing a K or 6 is the frequency of drawing K or 6 divided by the total number of draws:
Experimental probability = (frequency of K or 6) / (total number of draws)
Experimental probability = 17 / 100
Therefore, the experimental probability that the card selected was a K or 6 is 17/100 or 0.17.
To know more about probability and given link below -
https://brainly.com/question/11234923
#SPJ1
Any answer? Stuck on this one

Answers
quizizz thx 10 pts each brainiest guaranteed with pic

Answers
Answer:
option B
polynomial cannot have negative power
hope it helps
Represent using an integer.
a loss of 4 points
Answers
Answer:
-4
Step-by-step explanation: An integer is a number that can be negative and positive, is a whole number not a fraction so a loss of 4 with an integer would be -4 going back 4 on the number line.
The scale on a drawing reads 1/16^ prime prime =1^ prime .A2 inch long line represents
A. 8 feet
B. 16 feet
C. 32 feet
D. 64 feet
Answers
So
1 in line represents 16ft i e 2^4 ftHence
2in line represents
2(2^4)2(16)32ftTo pay for a home improvement project that totals $20,000, a homeowner is choosing between two different credit card loans with an interest rate of 9%. The first credit card compounds interest quarterly, while the second credit card compounds monthly. The homeowner plans to pay off the loan in 10 years.
Part A: Determine the total value of the loan with the quarterly compounded interest. Show all work and round your answer to the nearest hundredth. (4 points)
Part B: Determine the total value of the loan with the monthly compounded interest. Show all work and round your answer to the nearest hundredth. (4 points)
Part C: What is the difference between the total interest accrued on each loan? Explain your answer in complete sentences. (2 points)
Please only responded if you know how to do it, will give the brainiest to however answers it correctly
Answers
The total value of the loan with quarterly compounded interest is approximately $45,288.38, while the total value of the loan with monthly compounded interest is approximately $45,634.84. The difference in total interest accrued is approximately $346.46.
Part A: To determine the total value of the loan with quarterly compounded interest, we can use the formula for compound interest:
A = P(1 + r/n)^(nt),
where:
A is the total value of the loan,
P is the principal amount (initial loan amount),
r is the interest rate (in decimal form),
n is the number of times interest is compounded per year,
and t is the number of years.
Given:
P = $20,000,
r = 9% or 0.09,
n = 4 (quarterly compounding),
t = 10 years.
Substituting the values into the formula, we have:
A = 20000(1 + 0.09/4)^(4*10).
Calculating this value, we find:
A ≈ $45,288.38.
Therefore, the total value of the loan with quarterly compounded interest is approximately $45,288.38.
Part B: To determine the total value of the loan with monthly compounded interest, we follow the same formula but with a different value for n:
n = 12 (monthly compounding).
Substituting the values into the formula, we have:
A = 20000(1 + 0.09/12)^(12*10).
Calculating this value, we find:
A ≈ $45,634.84.
Therefore, the total value of the loan with monthly compounded interest is approximately $45,634.84.
Part C: The difference between the total interest accrued on each loan can be calculated by subtracting the principal amount from the total value of each loan.
For the loan with quarterly compounding:
Total interest = Total value - Principal
Total interest = $45,288.38 - $20,000
Total interest ≈ $25,288.38.
For the loan with monthly compounding:
Total interest = Total value - Principal
Total interest = $45,634.84 - $20,000
Total interest ≈ $25,634.84.
The difference between the total interest accrued on each loan is approximately $346.46.
for such more question on compounded interest
https://brainly.com/question/20406888
#SPJ8
how many itegers from 15 to 85, inclusive are multibles of 8
Answers
Answer:
9 multiples
Step-by-step explanation:
16,24,32,40,48,56,64,72,80
9
Which regular polygon has a minimum rotation of 36゚ about its center that carries the polygon onto itself
Answers
Answer:
decagon (10-sided polygon)
Step-by-step explanation:
A regular polygon has a minimum rotation of 360/n degrees about its center, where n is the number of sides in the polygon.
Therefore, the regular polygon with a minimum rotation of 36 degrees about its center is a polygon with 360/36 = 10 sides, or a decagon.
if this helps you, it would help me a lot if you could mark this as brainliest :)
4x – 22 = -14 pls help again
Answers
Answer:
x = 2
Step-by-step explanation:
Given equation,
→ 4x - 22 = -14
Now the value of x will be,
→ 4x - 22 = -14
→ 4x = -14 + 22
→ 4x = 8
→ x = 8/4
→ [ x = 2 ]
Hence, the value of x is 2.
A geometric sequence begins with 5 -15 45 -135 405 which option below represents the formula for the sequence
Answers
The formula for the geometric sequence above is Tₙ= 5. (-3⁽ⁿ⁻¹⁾)
How to find formula (Tₙ)In geometric sequence, the general formula of geometric sequence is:
Tₙ= ar⁽ⁿ⁻¹⁾
Information:
Tₙ = the n-th term
a = first term
r = ratio
n = the number of terms
In the question above,
a= 5
r= T2/T1=-15/5=-3 (This is the ratio)
Substitute the value of a and r to the formula of Tₙ:
Tₙ= ar⁽ⁿ⁻¹⁾
Tₙ= 5. (-3⁽ⁿ⁻¹⁾)
So the formula of Tₙ for the sequence above is Tₙ= 5. (-3⁽ⁿ⁻¹⁾)
In the above formula there is a ratio of geometric sequences. In a geometric sequence, two successive terms have the same ratio. Comparisons to geometric sequences are referred to as ratios (r). This is where the quotient of adjacent terms will be obtained.
Learn more about geometric sequences brainly.com/question/585785.
#SP1
PLEASE HELP ASAP!! (Please do this if ur good at Scientific Notation) please tell me which 4 should I put in the box! (Click picture) ‼️‼️

Answers
Answer:
1st one is 6.9 X 10^6
2nd one is 2 x 10^5
Step-by-step explanation:
how can you use pythagora's theorem to solve problems involving right-angled triangles

Answers
Using Pythagorean theorem, the length of the ladder is 10ft
What is Pythagorean Theorem?In mathematical terms, if y and z are the lengths of the two shorter sides (also known as the legs) of a right triangle, and x is the length of the hypotenuse, the Pythagorean theorem can be expressed as:
x² = y² + z²
In the questions given, the only one we can use Pythagorean theorem to solve is the one with ladder since it's forms a right-angle triangle.
To calculate the length of the ladder, we can write the formula as;
x² = 8² + 6²
x² = 64 + 36
x² = 100
x = √100
x = 10
The length of the ladder is 10 feet
Learn more on Pythagorean theorem here;
https://brainly.com/question/343682
#SPJ1
Consider random samples of size 1200 from a population with proportion 0.65 . Find the standard error of the distribution of sample proportions. Round your answer for the standard error to three decimal places.
Answers
Answer:
The standard error of the distribution of sample proportions is of 0.014.
Step-by-step explanation:
Central Limit Theorem
The Central Limit Theorem establishes that, for a normally distributed random variable X, with mean \(\mu\) and standard deviation \(\sigma\), the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean \(\mu\) and standard deviation \(s = \frac{\sigma}{\sqrt{n}}\).
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean \(\mu = p\) and standard deviation \(s = \sqrt{\frac{p(1-p)}{n}}\)
Consider random samples of size 1200 from a population with proportion 0.65 .
This means that \(n = 1200, p = 0.65\)
Find the standard error of the distribution of sample proportions.
This is s. So
\(s = \sqrt{\frac{0.65*0.35}{1200}} = 0.014\)
The standard error of the distribution of sample proportions is of 0.014.
Help me and explain

Answers
Answer:
2.5
Step-by-step explanation:
you cut the diameter in half and it's 5 so do the math
Find the zeros of the function: p(x)=(x−1)(x^2−2x−2).
Answers
Answer:
x = 6, x = -4
Step-by-step explanation:
a player scored 89 points in a single professional basketball game. he made a total of 55 baskets, consisting of field goals (worth two points) and fouls shots (worth one point). find the number of field goals and the number of fouls shots that the player made
Answers
The player made 38 foul shots.
Let's indicate the player's total number of field goals made by F and foul shots attempted by S. Based on the data in the problem, we may construct an equation system:
F + S = 55 (the player made a total of 55 baskets) (the player made a total of 55 baskets)
2F + S = 89 (the player scored a total of 89 points) (the player scored a total of 89 points)
The substitution approach can be used to find the values of F and S. We start by resolving the initial S equation:
S = 55 - F
Next, we change S in the second equation to the following expression:
2F + (55 - F) = 89
When we simplify and find F, we obtain:
F = 17
Hence, the player connected on 17 field goals. We may change this value of F into the first equation and then solve for S to determine the total number of foul shots:
17 + S = 55
S = 38
The player so scored 38 fouls.
To know more similar question visit:
https://brainly.com/question/11338475
#SPJ1
look at this diagram. if BD and EG are parallel lines and m

Answers
Given:
The line BD and EG are parallel lines.
\(\angle\text{EFH}=67^{\circ}\)To find: The measure of an angle,
\(\angle\text{GFC}\)Explanation:
We know that,
Vertical angles are angles opposite each other where two lines cross.
The vertically opposite angles are always equal in measure.
Hence, the angle of GFC must be equal to the angle of EFH.
Therefore,
\(\begin{gathered} \angle GFC=\angle EFH \\ \angle GFC=67^{\circ} \end{gathered}\)Final answer: The measure of angle,
\(\angle GFC=67^{\circ}\)