y cant people just put the answer y do they have to put all the things like just put a,b,c,d or ect...
Answers
what do you mean bruv?
N O R M A L people put the answer up top
Related Questions
In an ideal, unlimited environment, a population's growth follows a(n) __________ model exponential logistic hypergeometric geometric
Answers
In an ideal and unlimited environment, a population's growth follows an exponential model.
Exponential growth is when a population's growth rate keeps increasing over time because the population has access to an unlimited supply of resources, and its rate of reproduction is not limited by a lack of food, water, or space. In a population, exponential growth would result in an increase in the number of individuals in the population over time. Thus, in an ideal, unlimited environment, a population's growth follows an exponential model.Exponential growth can be mathematically represented by the following formula:Nt = Noertwhere:Nt = the population size at time tNo = the initial population sizee = Euler's numberr = the per capita growth rate of the populationt = the amount of time that has elapsed.
Learn more about exponential model here:
https://brainly.com/question/28596571
#SPJ11
The graph of a nonlinear function is shown on the coordinate plane. In the graph, y is a function of x. When the input of the function is -3, what is the output of the function?
Answers
The output of the function is -4, when the input of the function is -3
What are non-linear functions?Non-linear functions are functions that do not have a constant rate or slope
From the graph of the function, we have the following highlight:
At x = -3, the value of the function is -4
Hence, the output of the function is -4, when the input of the function is -3
Read more about non-linear functions at:
https://brainly.com/question/12676647

For the function f(x, y) = x² - 4x²y - xy + 2y¹, find the following: (5/5/3/3 pts) a) S b) fy A(1-1) d) ƒ,(1,-1) c)
Answers
For the function f(x, y) = x² - 4x²y - xy + 2y¹: (a) \(f(1, -1) = 8\), (b) \(f_y(1, -1) = -9\), (c) \(\nabla f(1, -1) = (11, -9)\), (d) \(f(1, -1) = 8\)
To find the requested values for the function \(f(x, y) = x^2 - 4x^2y - xy + 2y^2\), we evaluate the function at the given points and calculate the partial derivatives.
(a) The value of \(f(x, y)\) at the point (1, -1) can be found by substituting \(x = 1\) and \(y = -1\) into the function:
\[f(1, -1) = (1)^2 - 4(1)^2(-1) - (1)(-1) + 2(-1)^2\]
\[f(1, -1) = 1 - 4(1)(-1) + 1 + 2(1)\]
\[f(1, -1) = 1 + 4 + 1 + 2 = 8\]
Therefore, \(f(1, -1) = 8\).
(b) The partial derivative \(f_y\) represents the derivative of the function \(f(x, y)\) with respect to \(y\). We can calculate it by differentiating the function with respect to \(y\):
\[f_y(x, y) = -4x^2 - x + 4y\]
To find \(f_y\) at the point (1, -1), we substitute \(x = 1\) and \(y = -1\) into \(f_y(x, y)\):
\[f_y(1, -1) = -4(1)^2 - (1) + 4(-1)\]
\[f_y(1, -1) = -4 - 1 - 4 = -9\]
Therefore, \(f_y(1, -1) = -9\).
(c) The gradient of \(f(x, y)\), denoted as \(\nabla f\), represents the vector of partial derivatives of \(f\) with respect to each variable. In this case, \(\nabla f\) is given by:
\[\nabla f = \left(\frac{{\partial f}}{{\partial x}}, \frac{{\partial f}}{{\partial y}}\right) = \left(2x - 8xy - y, -4x^2 - x + 4y\right)\]
To find \(\nabla f\) at the point (1, -1), we substitute \(x = 1\) and \(y = -1\) into \(\nabla f\):
\[\nabla f(1, -1) = \left(2(1) - 8(1)(-1) - (-1), -4(1)^2 - (1) + 4(-1)\right)\]
\[\nabla f(1, -1) = \left(2 + 8 + 1, -4 - 1 - 4\right) = \left(11, -9\right)\]
Therefore, \(\nabla f(1, -1) = (11, -9)\).
(d) The value of \(f\) at the point (1, -1), denoted as \(f(1, -1)\), was already calculated in part (a) and found to be \(8\).
To know more about function refer here:
https://brainly.com/question/28750217#
#SPJ11
given list: ( 6, 13, 14, 30, 38, 50, 60, 72, 76, 87, 90, 92 ) ex: 42, 32, 12 which list elements will be compared to key 60 using binary search? enter elements in the order checked.
Answers
Using binary search to find the key 60 in the given list, the elements compared in order are: 38, 76, 60.
1. We start by comparing the key (60) to the middle element of the list, which is 38. Since 60 is greater than 38, we know that the key must be in the second half of the list.
2. Next, we compare the key to the middle element of the second half of the list, which is 76. Since 60 is less than 76, we know that the key must be in the first half of the second half of the list.
3. We then compare the key to the middle element of the first half of the second half of the list, which is 50. Since 60 is greater than 50, we know that the key must be in the second half of the first half of the second half of the list.
4. Next, we compare the key to the middle element of the second half of the first half of the second half of the list, which is 72. Since 60 is less than 72, we know that the key must be in the first half of the second half of the first half of the second half of the list.
5. Finally, we compare the key to the middle element of the first half of the second half of the first half of the second half of the list, which is 60. Since 60 is equal to 60, we have found the position of the key in the list.
To know more about binary search visit :-
https://brainly.com/question/15178888
#SPJ11
the coeffiecient for 6m + 7 is
m
Answers
Answer:
The coefficient of 6m is 6
Step-by-step explanation:
PLS HELP ME ON THIS QUESTION I WILL MARK YOU AS BRAINLIEST IF YOU KNOW RHE ANSWER!!
Answers
DOES ANYONE KNOW THE ANSWER

Answers
Answer:
D. none
Step-by-step explanation:
we only know about the right angle at the bottom right.
that is not enough information.
we always need 3 pieces of confirmed information of each triangle to be able to say that the 2 triangles are indeed of the same shape and size.
like the other answer options would indicate :
SAS : side - angle - side.
2 sides and the enclosed angle. if we know they are equal, then the rest of the triangles follow automatically.
SSS : all 3 sides. with 3 defined sides there is only one possible shake and size for that triangle.
HL : Hypotenuse and a leg of a right-angled triangle (also 3 pieces of information - 2 sides and the right angle).
since we have only the right angle and nothing else, we cannot prove that the triangles are congruent.
What is this expression in simplified form?
5√63-6√28
O A. 3√7
OB. 21√7
O C. 27√7
O D. 11√7
Answers
Answer:
23467788
Step-by-step explanation:
666789909 +75445899553235653889
I'm sure the
Here are two closed containers and four balls just fit in each container. Curved surface area of a cylinder is 2rrh Container A Each ball has a diameter of 60 mm. Which container has the smaller surface area? You must show your working (using mm). Show your working Container B A or B?
Answers
Two closed container with ball having the diameter of 60 mm, the container having the smaller surface area is Container B.
The area is the area occupied by a two-dimensional flat surface. It has a square unit of measurement. The surface area of a three-dimensional object is the space taken up by its outer surface.
We have the diameter of the ball as 60 mm
In container A we can see it is a cylinder:
Therefore, the four balls will give us the height of cylinder as
(4x60 = 240mm)
Area of cylinder is given as = 2πrh
A = 2 π (30) (240)
A = 45,239 mm²
In container B we can see it is a cube:
Length = 120 mm
Area of cube = 6a²
A = 6(120)²
A = 86,400 mm²
After comparing both the container square we can say that the Container B has more surface area than the container A.
86,400>45,239.
Learn more Surface area:
https://brainly.com/question/16519513
#SPJ4

What is the answer to #14 the picture is provided. MAKE SURE the radius of your clock is 2 inches.

Answers
Answer:
see attached
Step-by-step explanation:
You want a clock face with an angle of 240° between the hour and minute hands.
240°There are 22 possible locations for the minute hand such that the long arc between the hour and minute hands is 240°. The two of these that do not involve fractions of a second are 4:00 and 8:00.
The next occurrence will be 21 minutes 49 1/11 seconds later. And the one after that will be 43 minutes 38 2/11 seconds later, or 1 hour 5 minutes and 27 3/11 seconds after the first occurrence of the pair.
ImageA clock face illustrating 8:00 is attached. You may need to scale it to your desired radius.
You can set your compass to 2" and draw the clock face circle. The points for 12 and 8 can be located by using the 10:00 position as the center of arcs with the same radius. Where those arcs intersect the original circle are the locations of 12 and 8.

Simplify 2 root 1/2 - 6 root 1/8 - 10 root 4/5
Answers
Answer:
0.70710678 is 2 root 1/2 simplified
Step-by-step explanation:
which equation represents a parabola opening to the right with a vertex at the origin and a focus at (9,0)?
Answers
Answer:
y² = 36x
Step-by-step explanation:
Parabola opens right.
Thus equation is from; y² = 4ax
And focus is at (a, 0)
Since we are given focus at (9, 0),it implies that a = 9
Thus, equation of parabola is;
y² = 4 × 9 × x
y² = 36x
Find the value of X. Round the length to the nearest tenth.
A. 9.0 yd
B. 31.2 yd
C. 10.4 yd
D. 15.6 yd

Answers
Answer:
C. 10.4 yd
Cause why not?
(5y - 23)
(2x + 13)
(3x)
47°
Answers
ANSWER = 8460x (5y-23)(2x+13)
60
Let R be a relation defined on ℤ as follows: For all m, n ε ℤ, m R n iff 4 | (m2 – n2). a) Prove that R is an equivalence relation. b) Describe the distinct equivalence classes of the relation R. c) Do the distinct equivalence classes form a partition of ℤ? Explain.
Answers
the distinct equivalence classes [0], [1], [2], and [3] form a partition of ℤ.
What is Equivalence relation?
An equivalence relation is a relation between elements of a set that satisfies three properties: reflexivity, symmetry, and transitivity.
a) To prove that R is an equivalence relation, we need to show that it satisfies three properties: reflexivity, symmetry, and transitivity.
Reflexivity: For any integer m, we need to show that m R m, i.e., 4 |\((m^2 - m^2).\) Since the difference of squares is 0, which is divisible by 4, the relation is reflexive.
Symmetry: For any integers m and n, if m R n, then we need to show that n R m. If 4 | \((m^2 - n^2)\), then \((m^2 - n^2)\) is divisible by 4. Taking the negative of both sides, we have\((-n^2 + m^2) = (m^2 - n^2)\), which is also divisible by 4. Therefore, n R m, and the relation is symmetric.
Transitivity: For any integers m, n, and p, if m R n and n R p, then we need to show that m R p. Suppose 4 |\((m^2 - n^2)\)and 4 \(| (n^2 - p^2)\). This means \((m^2 - n^2) and (n^2 - p^2)\)are both divisible by 4. Adding these two divisibility statements, we get (m^2 - p^2) is also divisible by 4, which implies m R p. Hence, the relation is transitive.
Since the relation R satisfies reflexivity, symmetry, and transitivity, it is an equivalence relation.
b) The distinct equivalence classes of the relation R can be described by the set of all integers that are congruent modulo 4. In other words, each equivalence class contains integers that have the same remainder when divided by 4.
The distinct equivalence classes can be denoted as follows:
[0] = {..., -8, -4, 0, 4, 8, ...}
[1] = {..., -7, -3, 1, 5, 9, ...}
[2] = {..., -6, -2, 2, 6, 10, ...}
[3] = {..., -5, -1, 3, 7, 11, ...}
Each equivalence class consists of integers that satisfy the condition 4 | (m^2 - n^2), and within each class, any two integers have their squares yielding the same remainder when divided by 4.
c) The distinct equivalence classes [0], [1], [2], and [3] form a partition of ℤ. A partition of a set is a collection of non-empty, pairwise disjoint subsets whose union is the entire set.
In this case, the equivalence classes [0], [1], [2], and [3] are non-empty and pairwise disjoint because no integer can simultaneously belong to two different equivalence classes. Also, the union of all the equivalence classes covers the entire set of integers, as each integer belongs to exactly one of the equivalence classes.
Therefore, the distinct equivalence classes [0], [1], [2], and [3] form a partition of ℤ.
To know more about Equivalence relation visit:
https://brainly.com/question/15828363
#SPJ4
Help please i need this last question

Answers
Answer:
(D) or 703.4^2 is the answer here.
Step-by-step explanation:
Well I would imagine this.
Imagine a cylinder right and its liek a soda can. If you cut the middle straight down (which is the height) and you pull out the whole sheet and flatten it, you get a rectangle. You have the height but no width. The width can be found be finding the Circumfrnce of the circle or the perimeter of the circle on top.
Circumfrence formula is C=dπ or in this case the radius is 7, double the radius which would be 14 and the diameter, multiply that by π, you’d Get 43.98229 smth.
Now you have the surface for that rectangle or the surface recall for the whole cylinder except for the top and bottom, you just gotta find the top and double it!.
The area of a circle is a=πr^2
Raidus in. This case is 7, 7^2=49. 49π double it (top and bottom) is 307 smth Yu add those two up and you’d get 703.4 smth so the answer is (D).
what information is depicted in the p vs. 1/v graph that is not readily apparent from the p vs. v plot?
Answers
The information depicted in the p vs. 1/v graph can be used to determine the pressure of a gas at different volumes by measuring the slope of the line and the pressure at zero volume.
The p vs. 1/v graph provides the following additional information that is not readily apparent from the p vs. v.
plot: A linear relationship between pressure and the inverse of volume:
The p vs. 1/v graph shows a linear relationship between pressure and the inverse of volume.
This is due to the fact that as the volume decreases, the pressure increases proportionally, and as the volume increases, the pressure decreases proportionally.
The slope of the line represents the constant k in Boyle's law, which is a measure of the proportionality between pressure and the inverse of volume.
The pressure at zero volume:
The p vs. 1/v graph intersects the y-axis (1/v axis) at a value that represents the pressure at zero volume.
This value is also known as the maximum pressure, and it can be used to calculate the pressure that would be required to compress a gas to zero volume (which is impossible in practice).
For similar question on graph.
https://brainly.com/question/26865
#SPJ11
Let X and Y denote the tarsus lengths of male and female grackles, respectively. Assume that X is N(,) and Yis N(4,²). Given that the sample number of X and Y are n=m=25, and X = 33.8, S=3.9,Y=32.5, S=5.1. Use these observations to give a level a=0.05 test for H₁:μx = μy VS Hoxy. Give the p-value of this test. (10 pts)
Answers
To test the hypothesis H₁: μx = μy versus Hoxy, where μx and μy represent the means of X and Y respectively, we can perform a two-sample t-test. The test compares the means of two independent samples to determine if they are significantly different from each other.
The given information provides the sample means (X = 33.8, Y = 32.5) and the sample standard deviations (Sx = 3.9, Sy = 5.1). The sample sizes for both X and Y are n = m = 25.
Using this information, we can calculate the test statistic, which is given by:
t = (X - Y) / sqrt((Sx^2 / n) + (Sy^2 / m))
Plugging in the values, we get:
t = (33.8 - 32.5) / sqrt((3.9^2 / 25) + (5.1^2 / 25))
Next, we need to determine the degrees of freedom for the t-distribution. Since the sample sizes are equal (n = m = 25), the degrees of freedom for the test is given by (n + m - 2).
Using the t-distribution table or software, we can find the critical value corresponding to a significance level of α = 0.05 and the degrees of freedom.
Finally, we compare the calculated test statistic with the critical value. If the test statistic falls within the rejection region (i.e., the absolute value of the test statistic is greater than the critical value), we reject the null hypothesis. The p-value can also be calculated, which represents the probability of observing a test statistic as extreme or more extreme than the calculated value, assuming the null hypothesis is true.
Learn more about probability here: brainly.com/question/13604758
#SPJ11
The Birdwatching Club has 8 members, 2 girls and 6 boys. What is the ratio of
girls to boys in the Birdwatching Club?
O A. 2:1
O B. 3:1
"O c. 1:3
Answers
Answer:
c 1:3
Step-by-step explanation:
2/6 divide it by 1/3 and u get 1:3
which expression is equivalent to (x1/4y^16)^1/2
Answers
Answer:
y8x122
Step-by-step explanation:
Simplify this expression.
The coin box of a vending machine contains $4.55 in dimes and quarters. If
there are 23 coins altogether how many of each kind are there?
Answers
the answer is 9 because I know it's 9
TRUE/FALSE When inserting a value into a partially-filled array, in descending order, the insertion position is the index of the first value smaller than the value.
Answers
The given statement When inserting a value into a partially-filled array in descending order, the insertion position is indeed the index of the first value smaller than the value being inserted is true.
What is partially filled array?
A partially filled array, also known as a sparse array, is an array data structure where not all elements are populated with values. In other words, it is an array that contains empty or uninitialized elements.
When inserting a new value into this sorted array, we start from the beginning and compare the value with each existing element until we find the first element that is smaller. The insertion position for the new value is the index of this first smaller element.
For example, if we have a partially-filled array [10, 8, 5, 3] and we want to insert the value 6 into the array in descending order, we compare 6 with each element from left to right. The first element smaller than 6 is 5, and its index is 2. Therefore, the insertion position for the value 6 would be index 2, resulting in the updated array [10, 8, 6, 5, 3].
Learn more about partially filled array here:
https://brainly.com/question/6952886
#SPJ4
determine algebraically whether the given function is even, odd, or neither.
Answers
Answer:
To determine whether a function is even, odd, or neither, check if the function satisfies the properties of even or odd functions algebraically. If f(x) = f(-x), the function is even. If f(x) = -f(-x), the function is odd. Otherwise, it is neither.
Step-by-step explanation:
To determine whether a given function is even, odd, or neither, we need to analyze the function's algebraic properties based on how it behaves with respect to symmetry and the sign of its variables.
Even Function: An even function is symmetric with respect to the y-axis, meaning that if you reflect the graph of the function across the y-axis, it remains unchanged. Algebraically, an even function satisfies the property: f(x) = f(-x) for all x in the function's domain.
Odd Function: An odd function is symmetric with respect to the origin (0,0), meaning that if you rotate the graph of the function by 180 degrees around the origin, it remains unchanged. Algebraically, an odd function satisfies the property: f(x) = -f(-x) for all x in the function's domain.
Neither Function: If a function does not satisfy the properties of even or odd functions, it is considered neither.To determine the nature of a specific function, you would need to provide the function itself. Please provide the function, and I will be able to help you determine whether it is even, odd, or neither.
Learn more about even function here, https://brainly.com/question/2284364
#SPJ11
Which is the better buy?
Frozen Peas
Cost (dollars)
Weight (ounces)
O Brand A
A B
2
16
3
28
O Brand B
O The unit cost is the same.
Answers
The better buy is given by the following brand:
Brand A.
How to obtain the better buy?The better buy is obtained applying the proportions in the context of the problem.
A proportion is applied as the cost per ounce is given dividing the total cost by the number of ounces.
Then the better buy is given by the option with the lowest cost per ounce.
The cost per ounce for each brand is given as follows:
Brand A: 16/2 = $8 per ounce.Brand B: 28/3 = $9.3 per ounce.$8 per ounce is a lesser cost than $9.3 per ounce, hence the better buy is given by Brand A.
More can be learned about proportions at https://brainly.com/question/24372153
#SPJ1
will mark branily PPPPPPPLLLLLLLLLLLLLZzzzzzzzzzzz
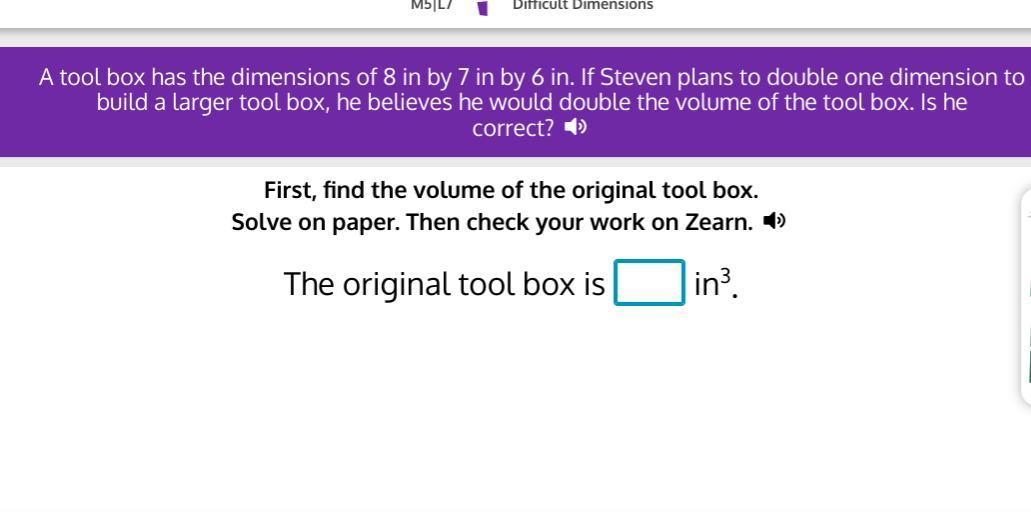
Answers
Answer:
336
Step-by-step explanation:
trus
Answer:
336 in³
Step-by-step explanation:
8 x 7 x 6 = 336 in³
Also the volume will double
A roller skating rink charges a skate rental fee and an hourly rate to skate. The total cost to skate for 2 hours is $9.50 and for 5 hours is $18.50. Assume the relationship is linear, where x represents the number of hours and y represents the total cost. Find and interpret the rate of change and initial value
Answers
To find the rate of change, we can use the slope formula, which is:
rate of change = (y2 - y1) / (x2 - x1)
where x1, y1 represent the first point (2 hours and $9.50), and x2, y2 represent the second point (5 hours and $18.50).
rate of change = ($18.50 - $9.50) / (5 - 2) = $9 / 3 = $3 per hour
To find the initial value, we can use the point-slope form of a linear equation, which is:
y - y1 = m(x - x1)
where m is the rate of change (slope) and (x1, y1) is a point on the line.
y = $3x + b
We can use the first point (2 hours and $9.50) to find the value of b:
$9.50 = $3(2) + b
b = $9.50 - $6 = $3.50
So the equation of the line is:
y = $3x + $3.50
The rate of change means that for every additional hour of skating, the cost increases by $3. The initial value of $3.50 means that the cost of the skate rental fee is $3.50.
Amber and Kieran bought some lengths of fabrics. Amber bought h meters of fabric and Kieran bought 7 times as much fabric as Amber. Kieran then decided to return 48 meters. He still had more fabric than Amber.
Write this as an inequality and solve to work out the values that h could take.
Answers
Step-by-step explanation:
Let's solve your inequality step-by-step.
7ℎ−48>ℎ
Step 1: Subtract h from both sides.
7ℎ−48−ℎ>ℎ−h
6ℎ−48>0
Step 2: Add 48 to both sides.
6ℎ−48+48>0+48
6ℎ>48
Step 3: Divide both sides by 6.
\( \frac{6ℎ}{6} > \frac{48}{6} \\ \\
\)
= ℎ>8
Which is it? hypothesis or theory? drag the statements under the correct heading.
Answers
Hypothesis is a proposed explanation for a phenomenon that can be tested through further investigation and theory is well-established explanation.
what is hypothesis?A proposed explanation for a phenomenon that can be tested through further investigation.
An educated guess based on available evidence.
What is theory?A well-established and widely accepted explanation for a phenomenon that has been extensively tested and supported by a substantial amount of evidence.
A scientific explanation that has been thoroughly tested and is widely accepted by experts in the field.
To learn more about hypothesis visit:
https://brainly.com/question/29519577
#SPJ4
a certain population of bacteria doubles every 10 minutes. if the number of bacteria in the population initially was 104, what was the number in the population 1 hour late
Answers
An hour later, the number of bacteria in the population was 6656.
To solve the how many bacteria are there in the population, use the exponential growth function.
Exponential growth and decay functions are written in standard form as
A = A₀•b^kt
where A = final amount
A₀ = initial amount = 104
b = growth factor = 2
k = growth rate = 1/10 minutes
t = time = 1 hour = 60 minutes
Plug in the values to the equation and solve for the final amount, A.
A = A₀•b^kt
A = 104•(2)^(1/10 minutes)(60 minutes)
A = 104•(2)^(6)
A = 104•64
A = 6656
Learn more about exponential growth here: https://brainly.com/question/13970958
#SPJ4
What is the probability that the sample proportion is between 0.2 and 0.42?
Answers
The probability that the sample proportion is between 0.2 and 0.42 can be calculated using the standard normal distribution.
To calculate the probability, we need to assume that the sample proportion follows a normal distribution. This assumption holds true when the sample size is sufficiently large and the conditions for the central limit theorem are met.
First, we need to calculate the standard error of the sample proportion. The standard error is the standard deviation of the sampling distribution of the sample proportion and is given by the formula sqrt(p(1-p)/n), where p is the estimated proportion and n is the sample size.
Next, we convert the sample proportion range into z-scores using the formula z = (x - p) / SE, where x is the given proportion and SE is the standard error. In this case, we use z-scores of 0.2 and 0.42.
Once we have the z-scores, we can use a standard normal distribution table or a statistical software to find the corresponding probabilities. The probability of the sample proportion falling between 0.2 and 0.42 is equal to the difference between the two calculated probabilities.
Alternatively, we can use the z-table to find the individual probabilities and subtract them. The z-table provides the cumulative probabilities up to a certain z-score. By subtracting the lower probability from the higher probability, we can find the desired probability.
In conclusion, the probability that the sample proportion is between 0.2 and 0.42 can be calculated using the standard normal distribution and z-scores. This probability represents the likelihood of observing a sample proportion within the specified range.
Learn more about probability here:
https://brainly.com/question/31828911
#SPJ11