y=7x
2x+y=63
Solve this equation
Answers
the answer is
2x + 7x = 63
9x = 63
x = 7
Answer:
Y=7X...........1
2X+Y=63......2 PUT THE Y =7X IN EQUATION 2
2X+7X=63
9X=63
X=63/9
X=7
VALUE X=7 IS PUT IN EQUATION 1
Y=7X
Y=7×7
Y= 49
ANS X=7
Y=49
Step-by-step explanation:
Related Questions
let x represent the amount of compensation that a doctor requests from a health insurer for heart surgery. let y represent the amount of compensation that the doctor actually receives from the health insurer. an actuary determines that x and y have the joint probability density function f(x,y)
Answers
The value of Var(Y) is 1/18 for x and y have the joint probability density function f(x,y).
In probability proposition, a probability viscosity function, or viscosity of a nonstop arbitrary variable, is a function whose value at any given sample in the sample space can be interpreted as furnishing a relative liability that the value of the arbitrary variable would be equal to that sample.
We have the equation as,
f(x, y) = [2y/x²]
\(Since f(y) =\) \(\int\limits^1_y {f(x, y)} \, dx\)
\(\int\limits^1_y {2y/x^2} \, dx = 2y\int\limits^1_y {x^-1} \, dx\\\\2y (1/y - 1)\)
= 2 - 2y
\(E(Y) =y\int\limits^1_0 {(2-2y)} \, dy = \frac{1}{3}\)
\(E(Y^2) =y^2\int\limits^1_0 {(2-2y)} \, dy = \frac{1}{6}\)
V(Y) = E(Y²) - E(Y)² = 1/6 - 1/9
V(Y) = 1/18
Therefore, Var(y) = 1/18.
Learn more about Probability density function:
https://brainly.com/question/28705601
#SPJ4
Complete question:
let x represent the amount of compensation that a doctor requests from a health insurer for heart surgery. let y represent the amount of compensation that the doctor actually receives from the health insurer. an actuary determines that x and y have the joint probability density function f(x,y)
f(x, y) = {2y/x²}
Calculate Var(Y)

Help!!!! I don't get this!!!!!!

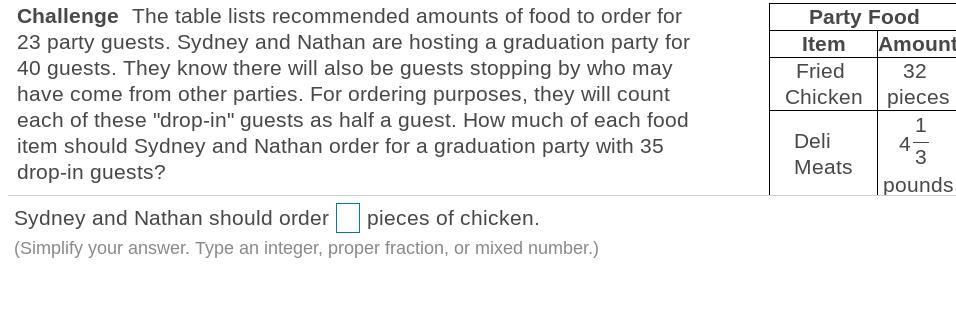

Answers
Answer:
C
Step-by-step explanation:
One pound (lb) is equal to 16 (oz). So, if you are trying to find how many ounces are in one pound, you have to divide the number of pounds by the 16, the amount of ounces in one pound.
Because if you see you I searched it up and it said that the answer was C simple
Hello,
Do these look correct?
3. Given: f(x) = [(3)^-x ] – 2. Find f(-2).
[3^-(-2)] - 2 = 9 - 2 = 7
f (-2) = 7
4. Given h(x) = 4 * 5^(x - 2) – 3. Find h(4).
4 *5^(4-2) - 3 = 4*5^2 - 3 = 4*25 -3 = 97
h(4) = 97
Thank you so much
Answers
Answer:
Yes they do :)
(o´▽`o)ノ x
The function y = f(x) is graphed below. What is the average rate of change of the
function f(x) on the interval -8 ≤ x ≤-7?

Answers
Answer:
2
Step-by-step explanation:
You want to know the average rate of change on the interval -8 ≤ x ≤ -7 of the cubic function shown in the graph.
Average rate of changeThe average rate of change of a function f(x) on interval [a, b] is given by ...
rate of change = (f(b) -f(a))/(b -a)
Here, we have ...
a = -8b = -7f(-8) = 0f(-7) = 2Using these values in the formula for rate of change, we get ...
rate of change = (2 -0)/(-7 -(-8)) = 2/1 = 2
The average rate of change of f(x) on [-8, -7] is 2.
Assume that the function f is a one
-to-one function.
(a) If f (7)
= 5, find f-1 (5)
Your answer is
(b) 18 f-1 (-3) = -2, find f(-2).I
Your answer is
Answers
For the given inverse functions we have:
a) f⁻¹(5) = 7
b) f(-2) = -3
How to work with inverse functions?
Remember that if f(x) and g(x) are inverse functions, then:
\(f(x) = y\\then\\g(y) = x\)
a) Now we know that:
f(7) = 5
Then if f⁻¹(x) is the inverse, we have:
f⁻¹(5) = 7
b) Now we know that:
f⁻¹(-3) = -2
Again, using the rule for inverse functions, we will have:
f(-2) = -3
If you want to learn more about inverse functions:
https://brainly.com/question/14391067
#SPJ1
Use the translation rule (x, y) (x - 2y 7) to find the image of F(-2, 6)
Answers
Answer:
Use photomath and look at each step! Sorry I would help but I’m bad at math.
Step-by-step explanation:
Select the correct answer.
Evaluate the following expression when x = -4 and y = 4.
x
6
−
x
4
y
A.
1
,
025
4
B.
1
,
023
4
C.
16
,
385
4
D.
−
1
,
023
4

Answers
Answer:
1023/4
Step-by-step explanation:
shown in the picture

There are 26 boys and 20 girls in a class.
The boys and the girls have some counters.
The mean number of counters that the boys have is 28.
The mean number of counters that the girls have is 19.
Work out the mean number of counters the 46 children have.
Answers
Computing the total number of counters in the class as 1,108, the mean number of counters that the 46 children have is 24.
What is the mean?The mean refers to the average value.
The average is the quotient of the total value divided by the number of items in the data set.
The number of boys in the class = 26
The number of girls in the class = 20
The total number of boys and girls in the class = 46
The mean number of counters that the boys have = 28
The total number of counters that the boys have = 728 (28 x 26)
The mean number of counters that the girls have =19
The total number of counters that the girls have = 380 (19 x 20)
The total number of counters that the class has = 1,108 (728 + 380)
The average or mean number of counters in the class = 24 (1,108 ÷ 46)
Learn more about the average at https://brainly.com/question/130657.
#SPJ1
Points A and B are on opposite sides of a lake. Another point, C. is 94.4 meters from Angle A. The measure of Angle A is 72° and the measure of Angle C is 30°. Find the distance between A and B.
Answers
To find the distance between points A and B, we can use trigonometry and the given information.
Let's label the distance between A and B as "d". We know that point C is 94.4 meters away from point A. From angle A, we have the measure of 72°, and from angle C, we have the measure of 30°.
Using trigonometry, we can use the tangent function to find the value of "d".
tan(72°) = d / 94.4
To solve for "d", we can rearrange the equation:
d = tan(72°) * 94.4
Using a calculator, we can evaluate the expression:
d ≈ 4.345 * 94.4
d ≈ 408.932
Therefore, the distance between points A and B is approximately 408.932 meters.
Question 6 of 15, Step 1 of 1
Correct
Jessie recently drove to visit her parents who live 385 miles away. On her way there her average speed was 24 miles per hour faster than on her way home (she ran into
some bad weather). If Jessie spent a total of 11 hours driving, find the two rates (in mph). Round your answer to two decimal places, if needed.
Answers
Answer: 84 mph 60 mph
Step-by-step explanation:
Let her average speed to home was x mph
Hence, the average speed to her parents was (x+24) mph
Thus:
Jessie spent for the trip to her parents' house: 385/x hours
Jessie spent for the trip to home: 385/(x+24) hours
\(\displaystyle\\\frac{385}{x} +\frac{385}{x+24} =11\\\\385(x+24)+385(x)=11(x)(x+24)\\\\385x+9240+385x=11x^2+264x\\\\770x+9240=11x^2+264x\\\\770x+9240-770x=11x^2+264x-770x\\\\9240=11x^2-506x\\\\9240-9240=11x^2-506x-9240\\\\0=11x^2-506x-9240\\\\Divide\ both\ parts\ of\ the \ equation\ by\ 11:\\\\0=x^2-46x-840\)
\(\displaystyle.\\Thus,\\\\x^2-46x-840=0\\\\D=(-46)^2-4*1*(-840)\\\\D=2116+3360\\\\D=5476\\\\\sqrt{D} =\sqrt{5476} \\\\\sqrt{D}=74 \\\\x=\frac{466б74}{2*1} \\\\x=\frac{46-74}{2} \\\\=\frac{-28}{2} \\\\x=-14\notin(x > 0)\\\\x=\frac{46+74}{2}\\\\x=\frac{120}{2} \\\\x=60\ miles\ per\ hour\)
\(60+24=84\ miles\ her\ hour\)
what’s the correct radical form of b^1/5
Answers
The correct radical form of b^1/5 is 5^√b.
What is the radical form?Square root and nth roots are represented by the symbol "radical," which. a square root is a component of a radical expression, which is an expression.
A number's or an algebraic expression's simplest radical form is referred to as this. When a number or algebraic expression contains no elements that are perfect nth powers under the radical, it is said to have an nth root and is said to be in its simplest radical form.
When a number or algebraic expression contains no elements that are perfect nth powers under the radical, it is said to have an nth root and is said to be in its simplest radical form.
Explanation:
Convert to radical form using the formula
a^x/n=n^√a^x
5^√b.
To learn more about radical form refer to:
https://brainly.com/question/27887772
#SPJ1
Please help me with all 3, I will mark brainliest as long as u tell me how u got those answers

Answers
1- (2,7) x values are not repeated
2-(3,4) bc the x none of the x values are the same, so it makes a function
3-(7,1) the same ^^
For any positive integer n, the value of n! is the product of the first n positive integers. For example, 4! = 4 * 3 * 2 * 1 =24. What is the greatest common divisor of 5! and 7! ?
Answers
The GCD of 5! and 7! is 2^3 * 3^1 * 5^1 = 120.
the greatest common divisor of 5! and 7! is 120.
To find the greatest common divisor (GCD) of 5! and 7!, we need to factorize both numbers and identify the common factors.
First, let's calculate the values of 5! and 7!:
5! = 5 * 4 * 3 * 2 * 1 = 120
7! = 7 * 6 * 5 * 4 * 3 * 2 * 1 = 5,040
Now, let's factorize both numbers:
Factorizing 120:
120 = 2^3 * 3 * 5
Factorizing 5,040:
5,040 = 2^4 * 3^2 * 5 * 7
To find the GCD, we need to consider the common factors raised to the lowest power. In this case, the common factors are 2, 3, and 5. The lowest power for 2 is 3 (from 120), the lowest power for 3 is 1 (from 120), and the lowest power for 5 is 1 (from both numbers).
For more such question on greatest common divisor
https://brainly.com/question/219464
#SPJ8
you need the previous answer to tell how to do the answer. "1232". Now that that's over with, onto the question.
The baker’s recipe for a loaf of bread calls for 12 ounces of flour. If he uses all of his flour to make loaves of bread, how many full loaves can he bake in two weeks?
Answers
Answer:
Step-by-step explanation:
1232 oz flour × (1 loaf)/(12 oz flour) = 102 loaves remainder 8 oz flour
Please read the question

Answers
For Questions 10-13:
10. An equation that relates the time required to the number of people is T = k/P.11. The table is attached.12. It takes 5 hours for 10 people to hand out these fliers13. YesHow to determine equations?10. The equation that relates the time required to the number of people can be written as:
T = k/P
where
T = time required,
P = number of people, and
k = constant of variation.
Using the given information that it takes 25 hours for 2 people to hand out fliers, use this to find the value of k.
Plugging these values into the equation:
25 = k/2
To solve for k, multiply both sides of the equation by 2:
50 = k
11. Now complete the table:
People Time
2 25
4 12.5
6 8.3
8 6.3
10 5
(rounding to the nearest tenth of an hour)
12. To find out how long it takes for 10 people to hand out the fliers, we can substitute P=10 into the equation:
T = 50/10
T = 5 hours
13. Yes, the result makes sense with what we know about inverse variation. In inverse variation, as the number of people increases, the time required decreases. This is evident in the table, where as the number of people increases from 2 to 10, the time required decreases from 25 hours to 5 hours. This inverse relationship is consistent with the concept of inverse variation.
Find out more on equation relations here: https://brainly.com/question/26126627
#SPJ1

What is the starting term of 64?
Answers
Answer:
1
Step-by-step explanation:
One is the starting term
1, 2, 4, 8, 16, 32, 64, 128, 256,
Solve:
(-4)^7*(-8^)^3+12/2=?
Show your work
Answers
Answer:
−16890
Step-by-step explanation:
Simplify the expression.
BONJOUR AIDEZ MOI SIL VOUS PLAIT

Answers
The distance from the center of the Earth to the point where the net gravitational force is zero is one-ninth the distance from the Earth to the Moon.
Let's assume that the distance from the center of the Earth to this point is denoted as x.
Given:
Mass of the Moon (M\(_{moon}\)) = 1/81 × M\(_{earth}\)
Distance from Earth to Moon (d\(_{moon}\)) = distance on center
According to the principle of gravitational equilibrium, the gravitational force from the Earth and the gravitational force from the Moon acting on an object at that point must balance out. Mathematically, we can express this as:
F\(_{earth}\) = F\(_{moon}\)
The gravitational force between two objects can be calculated using Newton's law of universal gravitation:
F \(_{gravity}\)= G × (m₁ × m₂) / r²
Where:
G is the gravitational constant (approximately 6.67430 x 10⁻¹¹m²/kg/s²)
m₁ and m₂ are the masses of the two objects
r is the distance between the centers of the two objects
Considering the gravitational forces involved:
F\(_{gravity}\)\(_{earth}\) = G ₓ (M\(_{EARTH}\) ₓ m\(_{OBJECT}\)) / (d\(_{earth}\))²
F\(_{gravity}\) \(_{moon}\) = G ₓ (M \(_{moon}\) ₓ m\(_{object}\)) / (d \(_{moon}\))²
Since we are looking for the point where the net gravitational force is zero, we set these two forces equal to each other:
G × (M\(_{earth}\) × m\(_{object}\)) / (d\(_{earth}\))² = G × (M \(_{moon}\) × m\(_{object}\)) / (d \(_{moon}\))²
Canceling out the common factors of G and m\(_object}\), and substituting the given values:
(M\(_{earth}\) × 1) / (d\(_{earth}\))² = (M \(_{moon}\) × 1) / (d \(_{moon}\))²
Rearranging the equation:
(d\(_{earth}\))²/ (M\(_{earth}\)) = (d \(_{moon}\))² / (M \(_{moon}\))
Taking the square root of both sides:
d\(_{earth}\) / √(M \(_{moon}\))) = d_moon / √(M \(_{moon}\))
Substituting the given values:
d\(_{earth}\) /√(M\(_{earth}\)) = d\(_{moon}\) / √(1/81 × M\(_{earth}\))
Simplifying further:
d\(_{earth}\) / √(M\(_{earth}\)) =d\(_{moon}\) / (1/9 × √(M\(_{earth}\)))
Multiplying both sides by √(M\(_{earth}\)):
d\(_{earth}\) = (1/9) × d\(_{moon}\)
To know more about gravitational force
https://brainly.com/question/27943482
#SPJ4
for each parallel lines. you are given the measure of one angle
Answers
Answer:
The question is not complete
HELPP I WILL GIVE BRAINLIST!!!
Which set of fraction bars shows two equivalent fractions?

Answers
Answer:
The middle! Please vote Brainliest!
Step-by-step explanation:
Answer:
The middle choice
Step-by-step explanation:
The bars go the same amount foward
Caden deposited $4,251 into a savings account 15 years ago. The account has an interest rate of 3.4% and the balance is currently $7,063.91. How often does the interest compound? a daily b monthly c quarterly d annually
Answers
Answer:
quarterly
Step-by-step explanation:
i just took the unit exam i got it right, its quarterly.
find the linear factorization of the function f(x)=6x^4-11x^3-19x^2+164x-770
Answers
Using the Factor Theorem, it is found that the linear factorization of the function is:
\(f(x) = \left(x + \frac{11}{3}\right)\left(x - \frac{7}{2}\right)(x - 1 - 3i)(x - 1 + 3i)\)
Factor Theorem:The Factor Theorem states that a polynomial function with roots has linear factorization given by:
\(f(x) = (x - x_1)(x - x_2) \cdots (x - x_n)\)
Using a calculator, the roots of the function \(f(x) = 6x^4 - 11x^3 - 19x^2 + 164x - 770) are given by: \(x_1 = -\frac{11}{3}, x_2 = \frac{7}{2}, x_3 = 1 + 3i, x_4 = 1 - 3i\)
Hence, applying the Factor Theorem, considering the given roots, the linear factorization given by:
\(f(x) = a(x - x_1)(x - x_2)(x - x_3)(x - x_4)\)
\(f(x) = \left(x + \frac{11}{3}\right)\left(x - \frac{7}{2}\right)(x - 1 - 3i)(x - 1 + 3i)\)
You can learn more about the Factor Theorem at https://brainly.com/question/24380382
Simplify the expression:
7(1 + 7x) =
Answers
Answer:
7 x 8x
Step-by-step explanation:
Let A be an n x n real matrix with the property that A^T = A. Show that if Ax = λx for some nonzero vector in C^n. then in fact, λ is real and the real part of x is an eigenvector of A if it is nonzero.
Answers
"Let x be any vector in \(C^n\), let q = \(x^T\)Ax, and let A be an n-by-n real matrix with the characteristic that \(A^T\) = A. By confirming that q = q, the equalities below demonstrate that q is a real number.
Let A be n x n real matrix with the property that. \(A^T = A\).
if Ax = λx.
(Ax) = λx
\(xA^T=\) λx
xA = λx( and therefore dλ are real, A =\(A^{T}\) = and dλ = λ).
x(A-λL) = 0
Therefore, A-λL is a real matrix.
Assume that A is a n by n matrix with a characteristic polynomial defined by det(λI−A). The number of times an eigenvalue of A appears as a root of that distinctive polynomial is known as its multiplicity.
and x (λI-A) = 0
Therefore, x(λL-A) is also real.
X1 is real.
Therefore, since is one of A's eigenvalues, there exists a nonzero vector X\(C^n\) such that AX=X
Learn more about real matrix here:
https://brainly.com/question/29583738
#SPJ4
A school is arranging a field trip to the zoo. The school spends 634.24 dollars on passes for 30 students and 2 teachers. The school also spends 256.20 dollars on lunch for just the students. How much money was spent on a pass and lunch for each student?
Answers
Therefore, the school spent $28.36 on a pass and lunch for each student.
What is cost?The term "cost" typically refers to the amount of resources, usually money, time, or effort, that is required to produce or obtain something. It is the expense incurred in order to achieve a particular goal or outcome.
cost can be further classified into different types, such as fixed costs (expenses that do not change regardless of the level of production), variable costs (expenses that change depending on the level of production), and opportunity costs (the cost of the next best alternative that is forgone when choosing a particular course of action).
First, we need to find the total number of people who need passes for the zoo.
30 students + 2 teachers = 32 people in total
The cost of the passes for 32 people is $634.24, so the cost per person is:
$634.24 ÷ 32 = $19.82 per person
Next, we need to find the cost of lunch per student. We know that the school spent $256.20 on lunch for just the students, so:
$256.20 ÷ 30 students = $8.54 per student
Finally, we can add the cost of the pass and the cost of lunch together to find the total cost per student:
$19.82 (pass) + $8.54 (lunch) = $28.36
To know more about cost visit:
https://brainly.com/question/30045916
#SPJ1
What is 6721 x 381 divided by 14 + 84
Answers
Let's break down the expression step by step:
1. Multiply: 6721 x 381 = 2,561,901.
2. Divide: 2,561,901 divided by 14 = 182,993.64 (rounded to two decimal places).
3. Add: 182,993.64 + 84 = 183,077.64 (rounded to two decimal places).
Therefore, the result of the expression 6721 x 381 divided by 14 + 84 is approximately 183,077.64.
Answer: 26129.6
Step-by-step explanation:
6721 x 381 = 2560701
14 + 84 = 98
2560701 / 98 = 26129.6
How do I find the possible degree(s) of a function from the graph alone?
Answers
Answer:
To determine the possible degree(s) of a function from the graph alone, you need to examine the behavior of the graph at the extremes (far left and far right) and consider the number of turning points or changes in direction. Here's a step-by-step approach:
Look at the far left side of the graph: Determine the behavior of the graph as it approaches negative infinity. Does the graph approach a specific value, such as a horizontal line (asymptote) or the x-axis? If the graph approaches a horizontal line, it suggests a polynomial function of even degree. If the graph approaches the x-axis, it indicates a polynomial function of odd degree or possibly a function with a root of multiplicity greater than one.
Look at the far right side of the graph: Determine the behavior of the graph as it approaches positive infinity. Similar to step 1, observe if the graph approaches a specific value or a horizontal line. The behavior at the far right side should be consistent with the behavior at the far left side. This can help you identify if the function is even or odd degree.
Examine the number of turning points or changes in direction: Count the number of times the graph changes direction. These points are where the slope of the graph changes from positive to negative or vice versa. The number of turning points can provide an indication of the degree of the polynomial. For example, if there are two turning points, it suggests a polynomial function of degree 3.
Remember that this method provides potential degrees, but it may not definitively determine the exact degree of the function. Additional information or analysis might be required for a more accurate determination.
The value of investments in the stock market change daily. Suppose you buy a stock for $4,811. It increases in value by 7% and then decreases 8% and then increases again by 9%. What is the new value?
Answers
Un tercio es menor que cuatro centésimos.?
Answers
Answer:
no por que 1/3 es menor que 4
Step-by-step explanation:
Whenever Deven and Laura owe each other money, they "pay" each other using stickers. They've agreed that a Harry Potter sticker is worth 49 dollars and a Twilight sticker is worth 35 dollars. They can even use stickers as "change" if one person overpays the other. For example, if Deven owes Laura 189 dollars, he can give her 6 Harry Potter stickers ($6 \cdot 49 = 294$ dollars), and she can return 3 Twilight stickers ($3 \cdot 35 = 105$ dollars). This trade is like a transfer of $294-105=189$ dollars. What is the smallest positive debt, in dollars, that can be paid off using sticker trading?
Answers
The smallest positive debt that can be paid off using sticker trading is $7$ dollars.
To find the smallest positive debt that can be paid off using sticker trading, we need to consider the values of the stickers (in dollars) and find the smallest positive amount that can be reached through a combination of these values.
Given that a Harry Potter sticker is worth $49 and a Twilight sticker is worth $35, we can approach this problem using the concept of the greatest common divisor (GCD) of these two values.
The GCD of $49$ and $35$ is $7$. This means that any multiple of the GCD can be represented using these sticker values.
In other words, any positive multiple of $7$ dollars can be paid off using sticker trading.
Therefore, the smallest positive debt that can be paid off using sticker trading is $7$ dollars.
For such more questions on debt
https://brainly.com/question/28929846
#SPJ8