....................
Answers
The solution is exactly the same we did with the number of defects twenty minutes ago.
In this case, this is the calculation:
1 * 0.413
2 * 0.287
3 * 0.218
and so on....you add them up and that is the mean.
Related Questions
what is $20.00 take away $4.60
Answers
Ms. Random’s math students collect data to determine the correlation between the number of hours of study outside of the classroom and the change in a student’s ACT scores. The students ask their classmates who took the ACT as juniors and as seniors to anonymously submit their ACT scores and the hours per week they studied outside of the classroom. The number of hours spent studying is then related to the change in ACT scores using this equation, where x is the number of hours studied per week and y is the change in ACT score.
y = 1 + 2x
Interpret the y-intercept of this equation in its context.
Answers
Y-intercept: (0,1)
If f(x)=6x+1 and g(x)=2x^2-5x, what is f+g
Answers
Answer:
2x²+x+1
Step-by-step explanation:
you are adding the functions together so it is collecting like terms
6x+1
+ 2x²-5x
2x²+x+1
One root of f(x) = x + 10x2 – 25x – 250 is x = -10. What are all the roots of the function? Use the Remainder Theorem.
x= -25 or x = 10
x = -25, x = 1, or x = 10
x = -10 or x = 5
x = -10, x=-5, or x = 5
Answers
Answer:
-10
-5
5
Step-by-step explanation:
From the answers given, you probably mean f(x) = x^3 + 10x2 – 25x – 250
The Remainder Theorem is going to take a bit to solve.
You have to try the factors of 250. One way to make your life a lot easier is to graph the equation. That will give you the roots.
The graph appears below. Since the y intercept is -250 the graph goes down quite a bit and if you show the y intercept then it will not be easy to see the roots.
However just to get the roots, the graph shows that
x = -10
x = - 5
x = 5
The last answer is the right one. To use the remainder theorem, you could show none of the answers will give 0s except the last one. For example, the second one will give
f((10) = 10^3 + 10*10^2 - 25*10 - 250
f(10) = 1000 + 1000 - 250 - 250
f(10) = 2000 - 500
f(10) = 1500 which is not 0.
==================
f(1) = (1)^3 + 10*(1)^2 - 25(1) - 250
f(1) = 1 + 10 - 25 - 250
f(1) = -264 which again is not zero

Answer:
yes its true
Step-by-step explanation:
just took the test
factor this expression 64x^3+125
Answers
Answer:
I think it's a but I'm not sure
Answer:
(4x +5)(16\(x\)2-20\(x\)+25)
Step-by-step explanation:
How do you check the factorization of a polynomial?
Answers
Completely factorize the polynomial using any methods that are appropriate, then look for shared factors and the polynomial's roots. Next, double-check your work to confirm accuracy.
Follow these steps to determine a polynomial's factorization:
Completely factor the polynomial using any relevant techniques, including grouping, utilizing the difference of squares or cubes, or using the quadratic formula.
By multiplying them all together, you can verify that each factor is accurate. The final figure must match the initial polynomial.
Look for shared factors among the polynomial factors. If the polynomial has common components, remove them from the equation and rewrite it as the product of the common factors and the other factors.
The polynomial's roots can be verified by setting each factor to zero and calculating the variable. The roots ought to correspond to the original polynomial.
Verify your work a second time to make sure all relevant elements have been identified and taken into consideration.
To learn more about polynomials, visit the link below:
https://brainly.com/question/11536910
#SPJ4
The points A, B, and C lie on a line so that AB = 12 cm and BC = 13.5 cm. Find the length of segment AC. Give all possible answers.
Give two possible answers. Not just one please.
Answers
Answer:
25.5 and 1.5
Step-by-step explanation:
AC = 12 cm
BC = 13.5 cm
12 + 13.5 = 25.5 cm
13.5 - 12 = 1.5 cm
The possible length of segment AC is 25.5 cm and 1.5 cm.
What is a line segment?A line segment is a part of line that is bounded by two distinct end-points. A line segment has a fixed length. Figures such as a triangle, polygon, hexagon, square are made of different numbers of line segments.
For the given situation,
The points A, B, and C lie on a line.
Length AB = 12 cm
BC = 13.5 cm
The possible length of segment AC can be found by adding and subtracting the lengths of AB and BC.
a) Length of AC = \(13.5+12\)
⇒ \(25.5\) cm
b) Length of AC = \(13.5-12\)
⇒ \(1.5\) cm
Hence we can conclude that the possible length of segment AC is 25.5 cm and 1.5 cm.
Learn more about line segment here
https://brainly.com/question/25727583
#SPJ2
in an article in the journal of management, joseph martocchio studied and estimated the costs of employee absences. based on a sample of 176 blue-collar workers, martocchio estimated that the mean amount of paid time lost during a three-month period was 1.3 days per employee with a standard deviation of 1.4 days. martocchio also estimated that the mean amount of unpaid time lost during a three-month period was 1.1 day per employee with a standard deviation of 1.6 days.
suppose we randomly select a sample of 100 blue-collar workers. based on martocchio
Answers
The probability shows that if the mean μ=1, then only 0.0027 is at least as small as the sample mean x=1.5. This also leads to the conclusion that if we believe that μ = 1, we must also believe that there is a 27 in 10,000 chance that our observed sample mean can be described as such.
To achieve the goal of this task, use the mean μ x and the standard deviation σ x.
The task has two conditions, the first is that the standard deviation σ is 1.3 days and the second is that the standard deviation σ is 1.8 days.
If the standard deviation σ is 1.3, then the mean μ is 1.4. And if the standard deviation is σ 1.8, then the mean is μ₁. In this case, the given standard deviation is μ 1.8, so use the second condition to achieve the task goal.
we know that:
μₓ = μ and σₓ = σ/√n
Given that:
μ = 1, σ = 1.8 and n = 100
So, by the formula of standard deviation σₓ,
σₓ = σ/√n = 1.8/√100
= 0.18
Calculate the probability with the mean μ=1, and standard deviation
σₓ =0.18.
P(x > 1.5) = P(z > 0.5/0.18)
= P( z > 2.78)
Looking at the z table, first, find the units and decimals in the first column of the z table. In this case, search for 2.7 first. Then look in which column the 100th value is placed. In this case, 0.08 and follow the number of lines placed 2.7.
Therefore, the value of 2.78 in table z is 0.9973 because z=0.9973, and the right tail area is 0.0027 or (1−0.9973).
Learn more about Probability:
https://brainly.com/question/30034780
#SPJ4
I NEED MEGA HELP WITH THIS QUESTION

Answers
Answer:
C. \(y = - \frac{1}{4} x - 2\)
Step-by-step explanation:
To find slope using coordinates, use the formula above.
\((4, - 3) \: (0, - 2)→ \frac{ - 2 - ( - 3)}{0 - 4}→ \frac{ - 2 + 3}{ - 4} → - \frac{1}{4} \)
Now, using the \(y = mx + b\) formula, plug in \( - \frac{1}{4} \) as \(m\) (slope) and either coordinate as the \(x\) and \(y\) values. It looks like this:
\( - 3 = - \frac{1}{4}(4) + b\)
To solve for \(b\), continue to use PEMDAS.
\( - 3 = - \frac{1}{4}(4) + b→ - 3 = - 1 + b→ - 2 = b\)
Now use \(m\) and \(b\) to solve for your equation:
\(y = - \frac{1}{4} x - 2\)
The answer is C.

7. (a) [2 marks] Determine the number of non-negative integers x where x≤999,999 and the sum of the digits in x equals 31 . (b) [3 marks] Determine the number of non-negative integers x where x≤98,394 and the sum of the digits in x equals 20 . (Hint: First try to figure out how many numbers x where 98,395≤x≤99,999 have digits that add up to 20 , then figure out how many numbers x where x≤99,999 have digits that add up to 20
Answers
(a) There are 100 numbers between 0 and 999,999 inclusive whose digits add up to 31. (b) There are 4,951 numbers between 0 and 99,999 inclusive whose digits add up to 20.
(a) We can solve this problem by using casework. The different cases are: The number is a multiple of 100. In this case, the sum of the digits is 31, so there is only 1 number in this case.
The number is between 100 and 999, inclusive. In this case, the sum of the digits can be 31 only if the number is 31 less than a multiple of 100. There are 31 numbers between 1 and 31 inclusive, so there are 31 numbers in this case.
The number is between 1,000 and 9,999, inclusive. In this case, the sum of the digits can be 31 only if the number is 31 less than a multiple of 100, or 31 more than a multiple of 100. There are 31 numbers between 31 and 61 inclusive, and 31 numbers between 61 and 91 inclusive, so there are 62 numbers in this case.
The number is between 10,000 and 99,999, inclusive. In this case, the sum of the digits can be 31 only if the number is 31 less than a multiple of 100, or 31 more than a multiple of 100, or 310 more than a multiple of 100. There are 31 numbers between 91 and 121 inclusive, 31 numbers between 121 and 151 inclusive, and so on, up to 31 numbers between 961 and 991 inclusive. There are a total of 31 * 7 = 217 numbers in this case.
Adding up the numbers in each case, we get a total of 1 + 31 + 62 + 217 = 311 numbers.
(b) We can solve this problem by using a similar casework approach as in part (a). The different cases are: The number is a multiple of 10. In this case, the sum of the digits is 20, so there are only 2 numbers in this case.
The number is between 10 and 99, inclusive. In this case, the sum of the digits can be 20 only if the number is 20 less than a multiple of 10. There are 20 numbers between 1 and 20 inclusive, so there are 20 numbers in this case.
The number is between 100 and 999, inclusive. In this case, the sum of the digits can be 20 only if the number is 20 less than a multiple of 10, or 20 more than a multiple of 10. There are 20 numbers between 20 and 40 inclusive, and 20 numbers between 40 and 60 inclusive, so there are 40 numbers in this case.
The number is between 1,000 and 9,999, inclusive. In this case, the sum of the digits can be 20 only if the number is 20 less than a multiple of 10, or 20 more than a multiple of 10, or 200 more than a multiple of 10. There are 20 numbers between 40 and 60 inclusive, and so on, up to 20 numbers between 980 and 1,000 inclusive. There are a total of 20 * 9 = 180 numbers in this case.
Adding up the numbers in each case, we get a total of 2 + 20 + 40 + 180 = 242 numbers.
Learn more about numbers here:
brainly.com/question/24908711
#SPJ11
Evaluate ( r - s) 3 + r 2 if r=8 s=5
Answers
determine if a line can have a constant rate and not to be proportional. write an agreement to defend your response.
Answers
The graph of a proportional relationship is a line through the origin. Relationships can have a constant rate of change but not be proportional.
It is required to write an agreement to defend your response.
What is line?A line is a straight one-dimensional figure having no thickness and extending infinitely in both directions. A line is made of a set of points which is extended in opposite directions infinitely. It is determined by two points in a two-dimensional plane. The two points which lie on the same line are said to be collinear points.
Given:
Any linear equation in the form y = mx+b has a constant rate of change, and that rate of change is the slope m. If b = 0, then it turns into y = mx. Here m is the constant of proportionality. Direct proportion equations always go through the origin. If b is nonzero, then y = mx+b will not be proportional.
So for example, y = 2x is proportional while y = 2x+1 is not proportional.
Therefore, the graph of a proportional relationship is a line through the origin. Relationships can have a constant rate of change but not be proportional.
Learn more about line here:
https://brainly.com/question/2696693
#SPJ2
draw a contour map of the function showing several level curves. f(x, y) = y/(x2 + y2) − 4
Answers
The contour map of the given function illustrates the function varies across the xy-plane, with regions of higher and lower values of f(x, y) indicated by the spacing and arrangement of the level curves.
To draw a contour map of the function f(x, y) = y/(x^2 + y^2) - 4, we need to plot several level curves.
Level curves are curves in the xy-plane that represent points where the function f(x, y) takes a constant value.
To find the level curves, we set f(x, y) equal to different constant values and solve for y in terms of x.
Let's choose some constant values for f(x, y) and find the corresponding level curves:
When f(x, y) = 0:
Setting y/(\(x^2 + y^2\)) - 4 = 0, we have y = 4(\(x^2 + y^2\)).
This equation represents a circle centered at the origin with radius 4.
When f(x, y) = -2:
Setting y/(\(x^2 + y^2\)) - 4 = -2, we have y = 2(\(x^2 + y^2\)). This equation represents a circle centered at the origin with radius 2.
When f(x, y) = 2:
Setting y/(\(x^2 + y^2\)) - 4 = 2, we have y = 6(\(x^2 + y^2\)). This equation represents a circle centered at the origin with radius 6.
By plotting these level curves on the xy-plane, we can create a contour map that shows the behavior of the function f(x, y).
The circles centered at the origin with radii 2, 4, and 6 represent the level curves corresponding to f(x, y) = -2, 0, and 2, respectively.
The contour map will illustrate how the function varies across the xy-plane, with regions of higher and lower values of f(x, y) indicated by the spacing and arrangement of the level curves.
Learn more about contour map here:
https://brainly.com/question/29887377
#SPJ11
The following list gives the number of pets for each of 10 students.
4.0.2.4.4.0.0.4.0.1
Find the modes of this data set.
If there is more than one mode, write them separated by commas.
If there is no mode, click on "No mode."
Answers
Answer:
4 and 2 are the modes I hope this helps u :))
Step-by-step explanation:
Question Help
The football team has a total of 25 jerseys. There are 10 medium-sized jerseys. What percent of the
jerseys are medium-sized jerseys?
Answers
Then you move the decimal over to the left by two places, so it would now be 40.0, so 40% of the jerseys are medium
On the average, Mr. Z drinks and drives once in 4 years. He knows that
Every time when he drinks and drives, he is caught by police.
According to the laws of his state, the third time when he is caught drinking and driving results in the loss of his driver's license.
Poisson process is the correct model for such "rare events" as drinking and driving.
What is the probability that Mr. Z will keep his driver's license for at least 10 years?
Answers
The probability that Mr. Z will keep his driver's license for at least 10 years is approximately 0.2212 or 22.12%.
To find the probability that Mr. Z will keep his driver's license for at least 10 years, we can use the Poisson distribution to model the occurrence of the rare event of him being caught drinking and driving.
The average frequency of Mr. Z drinking and driving is once in 4 years. Since the Poisson distribution assumes a constant average rate, we can use this information to calculate the average rate parameter (λ) for the Poisson distribution.
λ = Average frequency = 1 event in 4 years
To find the probability of Mr. Z not being caught drinking and driving for at least 10 years, we need to calculate the cumulative probability of zero events occurring in a 10-year period.
\(P(X > = 0) = 1 - P(X < 0)\)
Using the Poisson distribution formula, we can calculate the probability of zero events:
\(P(X = 0) = (e^(-λ) * λ^0) / 0!\)
Substituting the value of λ into the formula, we get:
\(P(X = 0) = (e^-(1/4) * (1/4)^0) / 0!\)
\(P(X = 0) = e^-(1/4)\)
To find the probability of Mr. Z not being caught drinking and driving for at least 10 years, we take the cumulative probability:
\(P(X > = 0) = 1 - e^-(1/4)\)
Calculating this value, we find:
\(P(X > = 0) = 0.2212\)
Therefore, the probability that Mr. Z will keep his driver's license for at least 10 years is approximately 0.2212 or 22.12%.
For more questions on probability, click on:
https://brainly.com/question/24756209
#SPJ8
Check out this rectangular prism 7 2 3
Answers
Answer:
Is there a picture/ screenshot we can look at to figure out the answer to your question here?
Step-by-step explanation:
Find the distance between the points.

Answers
Answer:
8
Step-by-step explanation:
Answer:
it will be 8
Step-by-step explanation:
(Its a tree) Look at the image of the organism below. What kind of organism is this?
(A,Prey) (B Consume) (C Producer) (D Predator)
Answers
Answer:
C. producer
Step-by-step explanation:
producers are plants and they provide food and shelter for small animals and stuff.
5(6+9) what is the sum of 30 and another whole number
Answers
Answer:
75
Step-by-step explanation:
5(6+9)
Times the five by the numbers in the bracket
30 + 45
= 75
Answer:
75
Step-by-step explanation:
5 x 6 = 30
5 x 9 = 45
30 + 45 = 75
Breast-feeding mothers secrete calcium into their milk. Some of the calcium may come from their bones, so mothers may lose bone mineral. Researchers measured the percent change in mineral content of the spines of 47 mothers during three months of breast-feeding.6Here are the data:
Blend Images/Superstock
(a)
The researchers are willing to consider these 47 women as an SRS from the population of all nursing mothers. Suppose that the percent change in this population has standard deviation ? = 2.5%. Make a stemplot of the data to verify that the data follow a Normal distribution quite closely. (Don
Answers
To create a stemplot, we first need to separate the data into "stems" and "leaves." Each stem represents the first digit(s) of each data point, while each leaf represents the last digit(s). For example, if the data point is 12.3, the stem would be 12 and the leaf would be 3.
To make a stemplot, the data should be arranged in increasing order, and then separated into stems and leaves.
STEMPLOT OF THE DATAHowever, you can use the data given and arrange it in increasing order and separate it into stems and leaves to make a stemplot. A stemplot is a good way to check if the data follow a normal distribution quite closely, the more the data is spread out and the more symmetric it is, the more likely it is to follow a normal distribution.
Also, it is important to mention that from the given data, it is not possible to conclude if the percent change in this population has standard deviation of 2.5%. This parameter can only be estimated from a sample if it follows a normal distribution which is not clear from the given data.
Learn more about Stemplot here:
https://brainly.com/question/29174445
#SPJ4
True or false: The average length of time between successive
events of a given size (or larger) is reffered to as the recurrence
interval (RI).
True
False
Answers
True. The average length of time between successive events of a given size or larger is indeed referred to as the recurrence interval (RI).
The concept of recurrence interval is commonly used in various fields, such as hydrology, seismology, and climatology, to analyze the frequency and magnitude of events.
In hydrology, for example, recurrence intervals are used to estimate the likelihood of extreme events such as floods. By analyzing historical data and calculating the time between floods of a certain magnitude, hydrologists can determine the average recurrence interval for floods of that magnitude. This information is valuable for assessing flood risks and designing infrastructure to withstand such events.
Similarly, in seismology, recurrence intervals are used to understand the frequency of earthquakes. By studying the past occurrence of earthquakes and measuring the time between events of a specific magnitude, seismologists can estimate the average recurrence interval for earthquakes of that magnitude. This helps in assessing the seismic hazard of a region and implementing appropriate mitigation strategies.
In climatology, recurrence intervals are employed to analyze extreme weather events such as hurricanes or heatwaves. By examining historical data and calculating the time between events with specific characteristics, climatologists can determine the recurrence interval for such events. This aids in understanding climate patterns, assessing future risks, and developing climate change adaptation strategies.
The statement is true. The average length of time between successive events of a given size or larger is known as the recurrence interval and is widely used in various disciplines to analyze and predict the occurrence of events.
Learn more about calculating here:
https://brainly.com/question/30151794
#SPJ11
3.5 decimals to mixed numbers
Answers
It’s one and two thirds written out
Can somebody please help me with this question??:,,)

Answers
Answer:
30 and 60
Step-by-step explanation:
1. "The measure of angle is twice the measure of its complement" means that one angle is twice the amount of another angle, and when both of them are added together, they equal 90°.
2. 60 is twice the amount of 30, and when both of them are combined, they equal 90°.
Therefore, the answer is 30 and 60.
Are the triangles congruent ? Please answer correctly !!!!!!!!!!!!!! Will mark Brianliest !!!!!!!!!!!!!!!!!!!

Answers
Answer:
no they are the illuminati
Step-by-step explanation:
x + y = 8
how do i solve for x
Answers
Answer:
x = 8 - y
Step by step explaination:
==>x + y = 8
==> x = 8 - y
==> substract the value
==> Done
What is the equation of the line that passes through the point ( − 4 , − 3 ) and has a slope of − 43?
Answers
Answer:
y = -43x - 175
Step-by-step explanation:
Slope-intercept form is y = mx + b
m = -43
x = -4
y = -3
-3 = -43(-4) + b
b + 172 = -3
b = -3 - 172 = -175
=> y = -43x - 175
Find the product.
(-2x 2)3 ·3x
Answers
Answer:
-15x
hope it's helpful ❤❤❤❤❤❤
THANK YOU.

Which of the following is an irrational number? How do you know? Use key words and definitions to explain your answer choice.
1632
0.25
0.0300300030000…
0
Answers
Answer:
0.0300300030000...
Step-by-step explanation:
An irrational number has endless non-repeating digits to the right of the decimal point. They can be written as decimals, but not as fractions.
1632 can be written as 3264/2
0.25 can be written as 1/4
0 could be written as 0/0
but 0.0300300030000 cannot be written as a fraction, and it satisfies the condition of an irrational number having endless non-repeating digits to the right of the decimal point.
Evaluate 6x^2+18x-15 at x=3. A-75
B-93
C-133
D-363
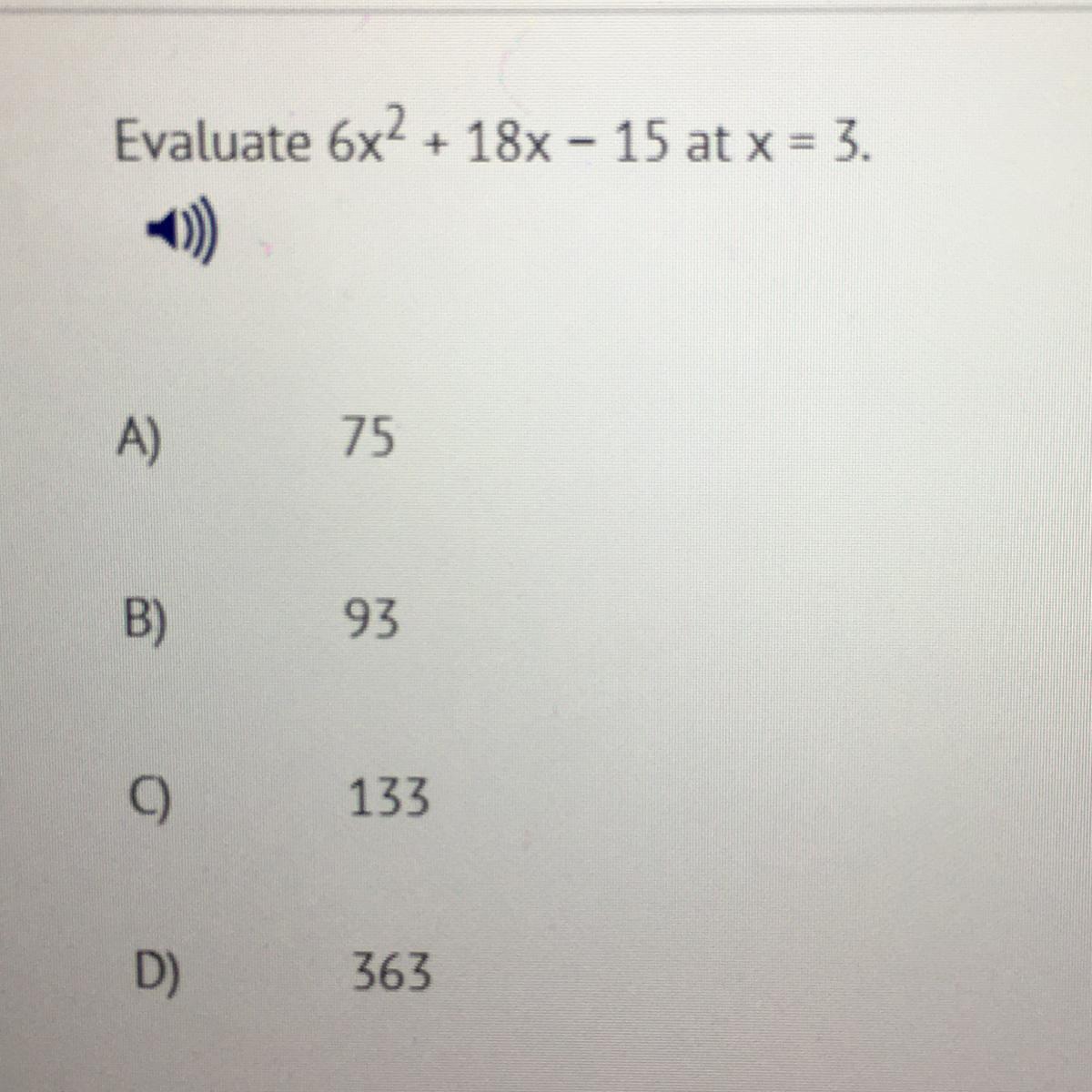
Answers
Step-by-step explanation:
When x = 3,
6x² + 18x - 15
= 6(3²) + 18(3) - 15 = 54 + 54 - 15 = 93. (B)