a rectangle has a length that is 5 inches greater than its width, and its area is 104 square inches. The equation(x+5) x=104 represents the situation, where x represents the width of the rectangle. (x+5) x=104 x2+5x-104=0. Determine the solutions of the equation. What solution makes sense for the situation?
Answers
Answer:
x=8
Step-by-step explanation:
Area of a rectangle=length×width
Area=104
Width=x
Length=5+x
104=x*(5+x)
104=5x+x^2
104-5x-x^2=0
x^2+5x-104=0
Can also be written as
-x^2-5x+104=0
Solve the quadratic equation using formula
−x2−5x+104=0
using the Quadratic Formula where
a = -1, b = -5, and c = 104
x=−b±√b2−4ac/2a
x=−(−5)±√(−5)2−4(−1)(104)/2(−1)
x=5±√25−(−416)/−2
x=5±√441/−2
The discriminant b^2−4ac>0
so, there are two real roots.
Simplify the Radical:
x=5±21/−2
x=-26/2 or 16/2
x=-13 or 8
The value of x can't be negative
So, x=8 is the answer
Related Questions
When data is positively skewed the mean will be?
Answers
Write an equation in point-slope form for the line that passes through the point with the given slope.

Answers
Answer:
Slope: 3
Equation: y + 2 = 3(x + 1)
Step-by-step explanation:
Find the slope using (-1, -2) and (0, 1):
\( slope (m) = \frac{y_2 - y_1}{x_2 - x_1} = \frac{1 - (-2)}{0 - (-1)} = \frac{3}{1} = 3 \)
Slope (m) = 3
The equation can be represented in point-slope form, y - b = m(x - a)
Where,
(-1, -2) = (a, b)
m = 3
Plug in the values into y - b = m(x - a)
y - (-2) = 3(x - (-1))
y + 2 = 3(x + 1)
Consider this expression. 7m^2 (2m -1 )(m 9) what expression is equivalent to the given expression?
Answers
The expression 126 \(m^4\) - 63\(m^3\) is equivalent to the given expression 7m² * (2m -1 )*(m9).
Properties of MultiplicationThe properties of multiplication are:
Distributive: a(b±c)= ab±ac Comutative: a . b = b. a Associative: a(b+c)= c(a+b) Identity: b.1=bPower RulesThere are different power rules, see some them:
1. Multiplication with the same base: you should repeat the base and add the exponents.
2. Division with the same base: you should repeat the base and subctract the exponents.
3.Power. For this rule, you should repeat the base and multiply the exponents.
4. Zero Exponent. When you have an exponent equals to zero, the result must be 1.
Here, you should apply the distributive property and power rules for solving this expression and finding an equivalent expression.
First, you should multiply the factors (2m -1)*(9m). As result, you find 18m²-9m.
After that, you should do the product between the previous result (18m²-9m) and the factor 7m². Thus,
(18m²-9m) * 7m²= 126 \(m^4\) - 63\(m^3\).
Read more about the distributive property here:
brainly.com/question/4077386
#SPJ1
how to find the third side of an isosceles triangle with only 2 sides known
Answers
Answer:
To obtain the third side of an isosceles triangle with two sides known, use the Pythagorean theorem if it is a right triangle or provide additional information if it is no
Step-by-step explanation:
You can follow these steps:
Identify the two sides that are known. In an isosceles triangle, these will be the two equal sides, often referred to as the legs of the triangle.
Determine the length of the base. The base is the third side of the triangle, and it is the side that is not equal to the other two sides.
If the isosceles triangle is also a right triangle, you can use the Pythagorean theorem to find the length of the base. The Pythagorean theorem states that in a right triangle, the square of the hypotenuse (the longest side) is equal to the sum of the squares of the other two sides. So, you can use the formula:
base^2 = (leg1)^2 + (leg2)^2
Take the square root of both sides to solve for the base:
base = √((leg1)^2 + (leg2)^2)
If the isosceles triangle is not a right triangle, you need additional information to determine the length of the base. This could be the measure of an angle or another side length.
Remember that the lengths of the two equal sides (legs) in an isosceles triangle are always equal, while the length of the base is different.
Learn more about isosceles triangle here, https://brainly.com/question/1475130
#SPJ11
What is the volume of a hemisphere with a radius of 4.3 cm, rounded to the nearest tenth of a cubic centimeter?
Answers
Step-by-step explanation:
\( \frac{4}{3} \pi {(4.3)}^{3} \\ \frac{4}{3} \pi(79.507) \\ \frac{318.028}{3} \pi \\ 106.0093\pi \\ \frac{1}{2} 333.038 \\ 166.5 {cm}^{3} \)
Jonah has two small bags of assorted doughnuts. Each bag contains exactly 8 powdered doughnuts.
Bag 1 contains 20 total doughnuts.
Bag 2 contains 24 total doughnuts.
What is the probability of Jonah grabbing
a powdered doughnut from
Bag 1?
Answers
Answer:
There is a 40% probability of Jonah grabbing a powdered doughnut from Bag 1.
Step-by-step explanation:
Total number of doughnuts in the bag 1 =20
Total powdered doughnuts in each bag = 8
Probability of selecting powdered doughnut from Bag 1 by Jonah =
\(\frac{8}{20} * 100\\40\)%
TEXT ANSWER
The speed of light is 299,792 kilometers per second. Zipporah tried converting the speed light to miles per hour using the following equivalencies:
1 km = 0.62 mi
1 min = 60 s
1 hr = 60 min
How fast is the speed of light in miles per hour?
Answers
The speed of the light in miles per hour is
66913574.4 miles per hour
What is unit conversion?It is the conversion of one unit to another unit with its standard conversion.
Example:
1 minute = 60 seconds
1 km = 1000 m
1 hour = 60 minutes
We have,
1 km = 0.62 mi
1 min = 60 s
1 hr = 60 min
Speed of light = 299,792 km per second
This can be written as,
1 km = 0.62 miles
299792 km = 299792 x 0.62 miles
299792 km = 185871.04 miles
1 hour = 60 minute
1 hour = 360 seconds
1/360 hour = 1 second
1 second = 1/360 hour
Now,
= 299792 km / 1 second
= 185871.04 miles / 1/360 hour
= 185871..04 x 360 miles per hour
= 66913574.4 miles per hour
Thus,
The speed of the light is 66913574.4 miles per hour.
Learn more about unit conversion here:
https://brainly.com/question/13899873
#SPJ1
Which two ratios represent quantities that are proportional?
A. 20/25 and 16/20
B. 15/21 and 20/24
C. 15/10 and 10/15
D. 5/6 and 17/12
Answers
Answer:A
Step-by-step explanation:
20/25 = 4/5
16/20 = 8/10 = 4/5
what is the term for the value that occurs most often in a series of numbers?
Answers
The term for the value that occurs most often in a series of numbers is called the mode.
The mode is one of the three main measures of central tendency, along with the mean and the median. It is a useful descriptive statistic that can provide insights into the characteristics of a dataset.
To find the mode of a set of data, you first need to arrange the data in order, either in increasing or decreasing order. Then, you simply identify the most frequent data point, which is the mode. In some cases, there may be more than one mode if multiple data points occur with the same maximum frequency.
The mode is particularly useful when dealing with categorical or nominal data, where there are distinct categories or values that cannot be ordered in a meaningful way. For example, the mode can help identify the most popular color among a group of people or the most common type of car on a given street. It can also be used for continuous data, although it may be less useful in this case than the mean or median.
For such more questions on value
https://brainly.com/question/843074
#SPJ8
Can an isoceles triangle be an obtuse triangle? Explain.
Answers
Answer:
it can and cant at the same time.
A typical person begins to lose consciousness if subjected to accelerations greater than about 5 g(49.0 m/s^2) for more than a few seconds. Suppose a 3.00×10^4−kg manned spaceship's engine has an exhaust speed of 2.50×10^3 m/s. What maximum burn rate ∣ΔM/Δt∣ could the engine reach before the ship's acceleration exceeded 5 g and its human occupants began to lose consciousness?
Answers
The maximum burn rate ∣ΔM/Δt∣ that the engine could reach before the ship's acceleration exceeded 5 g and its human occupants began to lose consciousness is approximately 51.0 kg/s.
Acceleration is directly proportional to the force acting on an object. In simple terms, if the force on an object is greater, then it will undergo more acceleration. However, there are limitations to the acceleration that can be tolerated by the human body. At about 5 g (49.0 m/s2) for more than a few seconds, an average person starts to lose consciousness. Let's use this information to answer the given question.
Let the maximum burn rate |ΔM/Δt| that the engine could reach before the ship's acceleration exceeded 5 g be x.
Let the mass of the spaceship be m and the exhaust speed of the engine be v.
Using the formula for the thrust of a rocket,
T = (mv)e
After substituting the given values into the formula for thrust, we get:
T = (3.00 × 104)(2.50 × 103) = 7.50 × 107 N
Therefore, the acceleration produced by the engine, a is given by the formula below:
F = ma
Therefore,
a = F/m= 7.50 × 107/3.00 × 104= 2.50 × 103 m/s²
The maximum burn rate that the engine could reach before the ship's acceleration exceeded 5 g is equal to the acceleration that would be produced by a maximum burn rate. Therefore,
x = a/5g= 2.50 × 103/(5 × 9.8)≈ 51.0 kg/s
Therefore, the maximum burn rate ∣ΔM/Δt∣ that the engine could reach before the ship's acceleration exceeded 5 g and its human occupants began to lose consciousness is approximately 51.0 kg/s.
Learn more about maximum burn rate
https://brainly.com/question/29328145
#SPJ11
What is one way to simplify variables with many, many levels (or decimal places) when creating a frequency distribution?A. Ignore outliers B. Organize data into class intervals C. Graph each level of the variable individually D. Compute a mean, median, and mode
Answers
One way to simplify variables with many levels (or decimal places) when creating a frequency distribution is to organize the data into class intervals (option B).
By grouping the data into intervals, the frequency distribution becomes more manageable and easier to interpret. This process involves dividing the range of values into distinct intervals or categories and then counting the number of observations falling within each interval. Class intervals provide a summary of the data by grouping similar values together, reducing the complexity of individual levels or decimal places.
This simplification technique is particularly useful when dealing with large datasets or continuous variables that have numerous levels or decimal values, allowing for a clearer representation and analysis of the data.
Option B holds true.
Learn more about frequency distribution: https://brainly.com/question/27820465
#SPJ11
Find the point, M, that is midway between P=(5,−8,−6) and Q=(-7,-2,-8).
Answers
The point, M, that is midway between P=(5,−8,−6) and Q=(-7,-2,-8) is
M = (-1, -5, -7)How to find the midwayIn geometry, the midpoint formula is an equation that determines the separation between two known coordinate locations at their halfway point.
The midway between the points P and Q is calculated using the method
Mid point = ((x₁ + x₂)/2, (y₁ + y₂)/2, (z₁ + z₂)/2)
Applying the method to the given points gives
P = (5, −8, −6) and Q = (-7, -2, -8)
M = ((5 + -7)/2, (-8 + -2)/2, (-6 + -8)/2)
M = (-1, -5, -7)
The coordinates of point M (-1, -5, -7) is the mid way between point P=(5,−8,−6) and Q=(-7,-2,-8).
Learn more about mid point here:
https://brainly.com/question/18315903
#SPJ1
which of the following equation has a graph that has a y-intercept of 4 and is parallel to 3y-9x=24?
A.-12x+4y=16
B.9x-3y=-15
C.2y=4x+8
D.7y=14x+7
E.3x-9y=14
Answers
Answer:
a
Step-by-step explanation:
in y intercept x = 0 so the choice a become the answer
Explain please thank you

Answers
8 • 9 = 7776
Therefore not equal
Find the 8th term of the geometric sequence 10, -20, 40, ...
Answers
Answer:
-1280
Step-by-step explanation:
10×(-2)= -20
-20×(-2)= 40
4th term: 40×(-2)= -80
5th term: -80×(-2)= 160
6th term: 160×(-2)= -320
7th term: -320×(-2)= 640
8th term: 640×(-2)= -1280
Find the least-squares solution x* of the system [1 0]
[0 1]
[0 0]
x = [-6]
[1]
[-3]
Answers
To find the least-squares solution x* of the system, we need to solve the equation Ax = b, where A is the coefficient matrix, x is the vector of unknowns, and b is the vector of constants. x₁ = -6 and x₂ = 1.
The given system can be written as:
[1 0] [x₁] [-6]
[0 1] * [x₂] = [ 1]
[0 0] [x₃] [-3]
Since the third row of the coefficient matrix is all zeros, it does not contribute to the equation. Therefore, we can ignore it for the purpose of finding the least-squares solution.
The reduced system becomes:
[1 0] [x₁] [-6]
[0 1] * [x₂] = [ 1]
This simplified system is already in the form of Ax = b, where A is the 2x2 identity matrix and b is the given vector. So the least-squares solution x* is simply equal to the vector b.
Therefore, the least-squares solution x* is:
x* = [-6]
[ 1]
Learn more about coefficient matrix here:
https://brainly.com/question/3086766
#SPJ11
If h = 7, what is ✓-27 + 4h?
Answers
✓-27 + 4h
✓-27 + 4x7
✓-27 + 28
5.19 + 28
33.19
Jason ran 4 miles in 2 minutes. Find his unit rate
4 minutes per mile
4 miles per minute
1 miles per 4
minutes
2 miles per minute
Answers
2 miles per minute. Dang, that's one fast guy.
Hitung nilai x bagi persamaan
Calculate the value of x for the equation
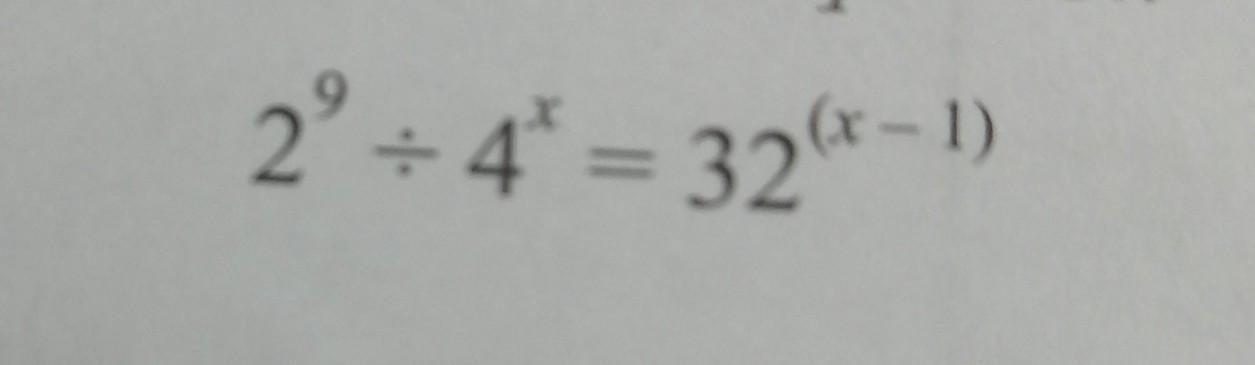
Answers
Answer:
2
Step-by-step explanation:
2⁹ ÷ (4^x) = 32^x × 32^(-1)
2⁹ ÷ 32^(-1) = 32^x × 4^x
16384 = 128^x
Kalau kamu dah belajar logaritma,
\(x = log_{128}(16384) \\ x = 2\)
Jika belum,
2¹⁴ = 2^(7x)
Bandingkan kuasa persamaan tersebut,
7x = 14
x = 2
can someone pls help me

Answers
The picture was blurry so I hope I read it right.
I hope this is correct and that it helps✨❤️
Answer:
\( {3}^{2h - 1} = {3}^{6} \times {3}^{3} \\ {3}^{2h - 1} = {3}^{9} \\ so...... \\ 2h - 1 = 9 \\ 2h = 10 \\ h = \frac{10}{2} = 5\)
plzzzzz mar it as a brilliant answrite an equation of the line in slope intercept form

Answers
The equation of the line in slope intercept form is y = (-4/3)x .
In the question ,
a line graph is given ,
we need to find the equation of that line .
the two points that are given on the line graph are (-3 , 4) and (0 , 0) ,
the equation of the line passing from the points (x₁ , y₁) with slope m in the slope intercept form is given by
(y - y₁) = m(x - x₁) .
we first need to find the slope .
So , m = (0 - 4)/(0 + 3)
m = -4/3
The equation of the line passing from the points (-3 , 4) with slope -4/3 , in the slope intercept form is
(y - 4) = (-4/3)(x -(-3))
y - 4 = (-4/3)(x + 3)
y - 4 = (-4/3)x - 4
y = (-4/3)x - 4 + 4
y = (-4/3)x
Therefore , The equation of the line in slope intercept form is y = (-4/3)x .
Learn more about Equation Of Line here
https://brainly.com/question/21298390
#SPJ1
Is 16b+ 18a equivalent to 3/4a(16b+24)?
HELP MEEEEE ASAPPPP
Answers
Answer:
Nope
Step-by-step explanation:
If you distribute the 3/4a they are no where near equal
Suppose that you wanted to estimate p, the true proportion of students at your school who have a tattoo with 98% confidence and a margin of error of no more than 0.10. How many students should you survey?
Answers
The number of students should we survey is 136.
What is Statistics?Statistics is the discipline that concerns the collection, organization, analysis, interpretation, and presentation of data.
To estimate the required sample size for estimating the proportion of students with tattoos with 98% confidence and a margin of error of no more than 0.10, we can use the formula:
n = (z² × p × (1-p)) / (E²)
where:
n = sample size
z = z-score associated with the desired confidence level (98% = 2.33)
p = estimated proportion of students with tattoos (unknown)
E = margin of error (0.10)
We don't know the value of p, but since we want the maximum possible sample size
Let us assume that p = 0.5, which gives us the largest possible value of n.
So, substituting the values into the formula, we get:
n = (2.33² × 0.5×(1-0.5)) / (0.10²) = 135.7
Hence, the number of students should we survey is 136.
To learn more on Statistics click:
https://brainly.com/question/29093686
#SPJ1
Use the imaginary number I to rewrite the expression below as a complex number simplify all radicals

Answers
Here, we want to use the imaginary number to rewrite the given expression as a complex number
Mathematically, the complex number can be written as;
\(i\text{ = }\sqrt[]{-1}\)Thus, we have;
\(\begin{gathered} -\sqrt[]{-4\text{ }}\text{ = -1 }\times\text{ }\sqrt[]{-4} \\ \\ =\text{ -1 }\times\text{ }\sqrt[]{-1\text{ }}\text{ }\times\text{ }\sqrt[]{4} \\ \\ =\text{ -1 }\times\text{ i }\times\text{ }\sqrt[]{4} \\ =\text{ -1 }\times\text{ i }\times\text{ }\pm2 \\ \\ =\text{ -2i or 2i} \end{gathered}\)Express 600g as a ratio of 1kg
please show working.
Answers
3:5
Step-by-step explanation:
change the kilogram into gram and then divide it
Answer:
3 : 5
Step-by-step explanation:
The quantities must have the same units
1 Kg = 1000 g , then
600 : 1000 ( divide both parts by 200 )
= 3 : 5 ← in simplest form
What is (f−g)(x)? f(x)=x4−4x2+4 g(x)=x3−2x2+4x−8 Enter your answer in standard form.
Answers
Answer:
x4-x3-2x2-4x+12
Step-by-step explanation:
(f-g)(x)=f(x)-g(x)
=> x4-4x2+4-(x3-2x2+4x-8)
=> x4-4x2+4-x3+2x2-4x+8
=> x4-x3-2x2-4x+12
The value of the function (f-g)(x) is \(x^4-x^3-2x^2-4x+12\).
What is the function?Functions are the fundamental part of the calculus in mathematics. The functions are the special types of relations. A function in math is visualized as a rule, which gives a unique output for every input x.
The given functions are \(f(x)=x^4-4x^2+4\) and g(x)=x³-2x²+4x-8.
Now, (f-g)(x)=f(x)-g(x)
= (\(x^4-4x^2+4\))-(x³-2x²+4x-8)
= \(x^4-4x^2+4\)-x³+2x²-4x+8
= \(x^4-x^3-2x^2-4x+12\)
Therefore, the value of the function (f-g)(x) is \(x^4-x^3-2x^2-4x+12\).
To learn more about the function visit:
https://brainly.com/question/28303908.
#SPJ2
Law of sines: startfraction sine (uppercase a) over a endfraction = startfraction sine (uppercase b) over b endfraction = startfraction sine (uppercase c) over c endfraction 2.2 units 2.4 units 3.0 units 3.3 units
Answers
The possible approximate lengths of b are: 2.3 units and 7.8 units
We know that the law of sines for triangle is:
The ratios of the length of all sides of a triangle to the sine of the respective opposite angles are in proportion.
This means, for triangle ABC,
\(\frac{sin~ A}{a} =\frac{sin~B}{b} =\frac{sin~ C}{c}\)
where a is the length of side BC,
b is the length of side AC,
c is the length of side AB.
For triangle ABC consider an equation from sine law,
\(\frac{sin~ A}{a} =\frac{sin~ C}{c}\)
here, c = 5.4, a = 3.3, and m∠A = 20°
\(\frac{sin~ 20}{3.3} =\frac{sin~ C}{5.4}\\\\\frac{0.3420}{3.3} =\frac{sin~ C}{5.4}\)
0.3420 × 5.4 = 3.3 × sin(C)
sin(C) = 0.5596
∠C = arcsin(0.5596)
∠C = 34.03° OR 145.9°
∠C ≈ 34° OR 146°
We know that the sum of all angles of triangle is 180 degrees.
so, ∠A + ∠B + ∠C = 180°
when m∠C = 34°,
20° + ∠B + 34.03° = 180°
∠B = 125.97°
m∠B = 126°
when m∠C = 146°,
20° + ∠B + 146° = 180°
m∠B = 14°
Now consider equation,
\(\frac{sin~ A}{a} =\frac{sin~ B}{b}\\\\\frac{sin~20^{\circ}}{3.3} =\frac{sin~ 126^{\circ}}{b}\)
b × 0.3420 = 0.8090 × 3.3
b = 7.8 units
when m∠B = 14°,
\(\frac{sin~20^{\circ}}{3.3} =\frac{sin~ 14^{\circ}}{b}\)
b × 0.3420 = 0.2419 × 3.3
b = 2.3 units
Learn more about the law of sines here:
https://brainly.com/question/17289163
#SPJ4
The complete question is:
Law of sines: StartFraction sine (uppercase A) Over a EndFraction = StartFraction sine (uppercase B) Over b EndFraction = StartFraction sine (uppercase C) Over c EndFraction
In ΔABC, c = 5.4, a = 3.3, and measure of angle A = 20 degrees. What are the possible approximate lengths of b? Use the law of sines to find the answer.
2.0 units and 4.6 units
2.1 units and 8.7 units
2.3 units and 7.8 units
2.6 units and 6.6 units
A club is choosing 2 members to serve on a committee. The club has nominated 3 women and 1 men. Based on chance alone, what is the probability that one woman and one man will be chosen to be on the committee? Your answer should be rounded to 4 decimal places (where applicable).
Answers
The solution of the given question is as follows :A club is choosing 2 members to serve on a committee.
Based on chance alone, the probability that one woman and one man will be chosen to be on the committee are as follows :Probability of selecting 1 woman out of 3 = 3C1 = 3Probability of selecting 1 man out of 1 = 1C1 = 1Probability of selecting 2 members from 4 = 4C2 = 6Therefore, the total number of ways that 2 members can be selected from 4 are: 3 × 1 × 6 = 18There are 18 different possible ways that a committee can be formed consisting of one woman and one man out of 3 women and 1 man.
Thus, the probability that one woman and one man will be chosen to be on the committee is: 3 × 1/6 = 1/2.00 = 0.5000 the probability that one woman and one man will be chosen to be on the committee is 0.5000 or 50% or 1/2 (in fraction) or 0.5 (in decimal).Hence, the solution of the given question is as follows: Probability that one woman and one man will be chosen to be on the committee is 0.5000.
To know more about probability visit:
https://brainly.com/question/31828911
#SPJ11
write an explicit formula for a subscript n, the nth term of the sequence 8, 11, 14,...
please please help
Answers
The required explicit formula for the given sequence to determine the nth term is given as nth term = 8 + (n - 1)3.
What is arithmetic progression?Arithmetic progression is the series of numbers that have common differences between adjacent values.
here,
The Sequences 8, 11, 14,...
first term a = 8
common difference = 11 - 8 = 3
Now,
The explicit formula is given as,
nth terms = a + (n - 1)d
nth term = 8 + (n - 1)3
Thus, the required explicit formula for the given sequence to determine the nth term is given as nth term = 8 + (n - 1)3.
Learn more about arithmetic progression here: https://brainly.com/question/20334860
#SPJ1