A snack food company advertises that they have increased the size of their standard
tortilla chips bag to 16.8 ounces, which is 20% more than the original size.
What is the original size of the bag?
Answers
Answer:
14 ounces
Step-by-step explanation:
100% + 20% = 120%
120%/20 = 6
16.8/6 = 2.8
16.8-2.8 = 14
Based on the current size being 20% more than the original size, the original size must be 14 ounces.
The current size of the bag is 20% more than the original size. Assuming that original size is x, that means that the current size is:
= x × (1 + 20%)
Solving for x would give you:
16.8 = x × (1 + 20%)
16.8 = x × 1.20
1.20x = 16.8
x = 16.8 / 1.20
x = 14 ounces
The original size was 14 ounces
Find out more at https://brainly.com/question/24980602.
Related Questions
you try feeding your dog the same amount of food both with and without added water while using the same bowls at the same time of day. what is the independent variable
Answers
If we feed the dog with same amount of food and with , without water , then the independent variable is " presence or absence of added water" .
The Independent Variable is the factor that is manipulated or varied in an experiment in order to observe the effect on the dependent variable.
In this case, the dependent variable would be the amount of food consumed by the dog.
By adding or not adding water to the food and keeping all other conditions constant ; such as: using the same bowls, feeding the dog at the same time of day ,
the experiment aims to determine the effect of the independent variable (added water) on the dependent variable (amount of food consumed).
Therefore , the independent variable in this situation is " presence or absence of water" .
The given question is incomplete , the complete question is
You try feeding your dog the same amount of food both with and without added water while using the same bowls at the same time of day.
What is the independent variable ?
Learn more about Independent Variable here
https://brainly.com/question/28382027
#SPJ4
the top box has a length of 6 yards

Answers
Answer:
42 yd²
Step-by-step explanation:
Area of a rectangle = width x length
lateral surface area is the area of the sides only
So the difference between the total surface area and the lateral surface area would be the surface area of the ceiling and floor (top and bottom).
area of floor and ceiling = 2(3.5 x 6) = 42 yd²
Or, we can calculate the total surface area and lateral surface area, then find the difference:
total surface area = 2(3.5 x 4.2) + 2(4.2 x 6) + 2(3.5 x 6) = 121.8 yd²
lateral surface area = 2(3.5 x 4.2) + 2(4.2 x 6) = 79.8 yd²
difference = 121.8 - 79.8 = 42 yd²
i really need help with 4 if anyone knows it please help im begging :(

Answers
Answer:
I think 3
Step-by-step explanation:
I'm not totally for sure about it though. sorry :(
Answer:
Triangle sum theorem
Step-by-step explanation:
The amount of money a store earns during each hour one day can be modeled by the function y = -8x^2 + 64x – 60, where y is in dollars and x is the time in hours from when the store opens. During which hour will the store earn $68?
Answers
Answer:
go to photo math thank me later
Step-by-step explanation:
Answer: After “4 hours” of work will the store earn $68.
Step-by-step explanation: To find the answer you plug in 4 into all values of x in the equation it looks like this y = -8(4)^2 + 64(4) - 60. Once you multiply, the numbers it turns into is y = -128 + 256 - 60. Then. Once you do all the addition and subtraction you get y = $68.
Have a nice day!
(!!) DO NOT REPOST OTHER UNRELATED QUESTIONS' ANSWERS PLEASE
Create an ER diagram using Chens notation with these facts:
- Each sport has different events, each event is only for one
sport.
- Events c
Answers
The ER diagram in Chen's notation for the given facts would include two entities: "Sport" and "Event." The relationship between the entities would be represented as a one-to-many relationship, where each sport can have multiple events, but each event is associated with only one sport.
In Chen's notation, entities are represented as rectangles, and relationships are represented as diamonds connected to the entities with lines. Based on the given facts, we would have two entities: "Sport" and "Event."
The "Sport" entity would have an attribute representing the name of the sport. The "Event" entity would have attributes such as the name of the event, date, location, and any other relevant information.
To represent the relationship between the entities, we would draw a line connecting the "Sport" entity to the "Event" entity with a diamond at the "Event" end. This indicates a one-to-many relationship, where each sport can have multiple events. The relationship line would have a crow's foot notation on the "Event" end, indicating that each event is associated with only one sport.
Overall, the ER diagram in Chen's notation would visually depict the relationship between sports and events, illustrating that each sport can have multiple events, but each event is specific to only one sport.
Learn more about one-to-many relationship here:
brainly.com/question/13266921
#SPJ11
2. The picture shows a feeding trough that is shaped like a right prism.
(a) Surface A and B are identical trapezoids. What are the lengths of the bases of the trapezoids? What is the height?
(b) Surfaces A and B will be painted blue. What is the total area that will be painted blue?
(c) The edges of the trapezoids need to be sanded. A piece of sandpaper advertises that it can sand up to 80 cm. Will one piece of sandpaper be enough to sand the edges of one trapezoid? Explain.

Answers
Answer:
B
Step-by-step explanation:
What is the volume of this rectangular prism? Use unit cubes to solve.

Answers
Answer:
Step-by-step explanation:
l*w*h 5 times 4 times 3 =60m
Σ10 4(1.5)n-1 = [?].
Answers
Answer: =156n−1
Step-by-step explanation:
The value of 8-[12-(-4)]
Answers
Answer:
-8
Step-by-step explanation:
Following Order of Operations rules, we evaluate anything within parentheses first:
8-[12-(-4)] = 8 - 16 = -8
One hundred people in your neighborhood always drive to work between 7:30 and 8:00 A.M. and arrive 30 minutes later. Why must two people always arrive at work at the same time, within a minute
Answers
Answer: Pigeonhole Principle
Step-by-step explanation:
The Pigeonhole Principle espouses that if there are more objects than the places the objects are supposed to fit into, some of those places will have more than one object. For instance, if there are 20 pigeons and only 19 pigeonholes, one pigeonhole will have more than one pigeon.
If one hundred people in your neighbourhood leave between 7:30 and 8:00 A.M. and arrive 30 minutes later that means they will arrive between 8:00 and 8:30 A.M. This means that 100 people will have to arrive in 30 minutes.
We would have to fit 100 people in 30 minutes. The minutes are the pigeonholes and the people are the pigeons. There will therefore be a situation where at least 2 people will arrive at the same minute because there are many more people than minutes for them to arrive in.
Convert 3% to an
equivalent decimal.
Answers
Answer:
.03
Step-by-step explanation:
I hope that this helps
Answer:
0.03
Step-by-step explanation:
3% would equal 0.03.
0.03 x 100 = 3 and that would mean 3%
Hope this helps :)
The calculation of SNN distance does not take into account the position of shared neighbors in the two nearest neighbor lists. In other words, it might be desirable to give higher similarity to two points that share the same nearest neighbors ranked higher in the nearest neighbor lists. Describe how you might modify the definition of SNN similarity to achieve that. Justify your modification.
Answers
By incorporating this information into the similarity measure, we can produce more accurate and informative results in clustering and classification tasks.
To modify the definition of clustering similarity to take into account the position of shared neighbors in the two nearest neighbor lists, we can introduce a weighting factor that considers the rank of the shared neighbor in each list. This can be achieved by multiplying the regular SNN similarity value by a weight factor that is calculated as the reciprocal of the sum of the ranks of the shared neighbors in the two lists. For example, if two points have a shared neighbor that is ranked 2nd in one list and 3rd in the other list, the weight factor would be 1/(2+3) = 0.2. This weight factor would then be multiplied by the regular SNN similarity value to produce a modified SNN similarity score that gives higher similarity to points that share the same nearest neighbors ranked higher in the nearest neighbor lists. This modification is justified because it takes into account the fact that having shared neighbors that are ranked higher in the nearest neighbor lists is a stronger indicator of similarity than having shared neighbors that are ranked lower.
Learn more about clustering here
https://brainly.com/question/29888905
#SPJ11
a fruit company delivers its fruit in two types of boxes large and small A delivery of two large boxes in 3 small boxes has a total weight of 78 kg A delivery of 6 large boxes and five small boxes has a total waste of 180 kg how much does each type of box weigh
Answers
Let the weight (in kg) of a large box be 'x' and the weight of a small box be 'y'.
Given that the weight of 2 large boxes and 3 small boxes is 78 kg,
\(2x+3y=78\)Also given that the weight of 6 large boxes and 5 small boxes is 180 kg,
\(6x+5y=180\)Here we will apply the Elimination Method to solve both the equations.
Consider the three times the first equation being subtracted from the second equation,
\((6x+5y)-3(2x+3y)=180-3(78)\Rightarrow6x+5y-6x-9y=180-234\)Simplify the terms,
\(-4y=-54\Rightarrow y=\frac{54}{4}=13.5\)Substitute this value in the first equation,
\(2x+3(13.5)=78\Rightarrow2x=78-40.5\Rightarrow2x=37.5\Rightarrow x=18.75\)Thus, the larger box weighs 18.75 kg while the small box weighs 13.5 kg
12 is what % of 50?
Please help
Answers
Answer:24%
Step-by-step explanation:
When you multiply a fraction by 100 you will get the percent
12/50 x 100=24
If Maliyah won 25 games and llost 10 what would be the ratio for the games lost to total
Answers
Answer:
10:35
Step-by-step explanation:
A prism with a heart-shaped base is sliced parallel to the base. What shape is formed?
Answers
The shape formed, when a prism with a heart-shaped base is sliced parallel to the base, will be congruent to its base. Hence, it will also be heart-shaped.
A prism is a polyhedron made up of n parallelogram faces that connect the n-sided polygon base, the second base, which is a translated duplicate of the first base, and the n faces. The bases are translated into all cross-sections that are parallel to them.
In the question, we are informed that a prism with a heart-shaped base is sliced parallel to the base.
We are asked what shape is formed.
From the above discussion, we know that the bases are translated into all cross-sections that are parallel to them, that is, all cross-sections parallel to the base, will be congruent to the base.
Thus, the shape formed, when a prism with a heart-shaped base is sliced parallel to the base, will be congruent to its base. Hence, it will also be heart-shaped.
Learn more about prisms at
https://brainly.com/question/3594469
#SPJ1
Answer:
Step-by-step explanation:
Heart Shaped
Can someone please help me with these? I tried doing them myself, but I got confused enough that I haven’t ended up with an answer yet


Answers
Solution:
Given:
\(1-3x>14-(4-6x)\)Solving the given inequality with the steps involved,
\(\begin{gathered} 1-3x>14-(4-6x) \\ E\text{ xpanding the bracket on the r}ight\text{ hand side of the inequality;} \\ 1-3x>14-4+6x \\ 1-3x>10+6x \\ \text{Collecting the like terms;} \\ 1-10>6x+3x \\ -9>9x \\ \text{Dividing both sides by 9,} \\ -\frac{9}{9}>\frac{9x}{9} \\ -1>x \\ \text{Flipping the sides, and changing the inequality using the principle if a>b, then bx, } \\ \text{then} \\ x<-1 \end{gathered}\)Therefore, the solution as inequality is;
\(x<-1\)The solution in interval notation therefore is;
\((-\infty,-1)\)Graphing the solution on a number line;

based on the question i just asked how many 2 litre bottles of juice should be purchased if 1000mi is eaqul to 1 litre
Answers
The number of 2-liter bottles of juice that should be purchased depends on the total volume of juice required.
As per the previous question, the area of the rectangular field including the path was zero, which means that there was no field present. Therefore, the question of purchasing 2-liter bottles of juice based on that field is not valid.
However, if we assume that a field of a certain area is present and we need to find the number of 2-liter bottles of juice that should be purchased if 1000ml is equal to 1 liter, further information is needed.
We would need to know how much juice is needed and what is the concentration of the juice. Once we have this information, we can determine the total volume of juice required and divide it by the volume of each 2-liter bottle of juice to get the number of bottles needed.
For example, if we need 10 liters of juice, we will require 10,000 ml of juice. Since 1000ml is equal to 1 liter, we will need 10,000/1000 = 10 2-liter bottles of juice.
For such more questions on volume
https://brainly.com/question/463363
#SPJ8
Zen Inc. manufactures two types of products, the G1 and the T1 model airplane. The manufacturing process consists of two principal departments: production and assembly. The production department has 58 skilled workers, each of whom works 7 hours per day. The assembly department has 25 workers, who also work 7-hour shifts. On an average, to produce a G1 model, Zen Inc. requires 3.5 labor hours for production and 2 labor hours for assembly. The T1 model requires 4 labor hours for production and 1.5 labor hours in assembly. The company anticipates selling at least 1.5 times as many T1 models as G1 models (this is the product mix). The company operates five days per week and makes a net profit of $130 on the G1 model, and $150 on the T1 model. Zen Inc. wants to determine how many of each model should be produced on a weekly basis to maximize net profit. If the numbers of G1 and T1 products produced each week are denoted as G and T respectively, the function that describes Zen, Inc.’s sales product mix for a week is?
Answers
Each model should be produced on a weekly basis to maximize net profit is $75,900.
What is Net profit?
In both business and accounting, net income or profit is an entity's revenue less cost of goods sold, costs, depreciation and amortisation, interest, and taxes for a certain accounting period.
As given,
Number of products sold:
T ≥ 1.5G
Maximize Profit Z = 150T + 130G
Labor Constraint:
Labor hours Available:
Production Department:
Number of labor hours available per day = 58 × 7 = 406
Number of days in a week = 5
Number of lobor ours available per week = 406 × 5 = 2030
Assembly Department:
Number of labor hours available per day = 25 × 7 = 175
Number of days in a week = 5
Number of labor hours available per week = 175 × 5 = 875.
Labor hours Required:
Labor hours required for G1 model:
Labor hours required at Production department = 3.5G
Labor hours required at assembly department = 2G
Labor hours required for T1 model:
Labor hours required at Production department = 4T
Labor hours required at Assembly department = 1.5T
Total labor hours required at Production department = 3.5G + 4T
Total labor hours required at Assembly department = 2G + 1.5T.
Constraint:
Labor hours required ≤ Labor hours Available.
Production department Labor constraint
3.5G + 4T ≤ 2030
Assembly department Labor constraint:
2G + 1.5T ≤ 875
The function:
Maximum Profit Z = 150T +130G
Constraint:
3.5G +4T ≤ 2030
2G + 1.5T ≤ 875
T ≥ 1.5G
Suppose that for example:
10.5G +12T = 6090
16G + 12T = 7000
Solve both equations simultaneously,
5.5G = 7000 - 6090
5.5G = 910
G = 910/5.5
G = 363
Since, T > G
Hence,
T = 363,
G = 165
Calculate Profit:
150T + 130G = 150 × 363 + 130 × 165
= 54450 + 21450
= 75900
Hence, each model should be produced on a weekly basis to maximize net profit is $75,900.
To learn more about Net profit from the given link.
https://brainly.com/question/28390284
#SPJ4
Having trouble question is in attachment

Answers
Answer:
\(x=\frac{35}{9} .\)
Step-by-step explanation:
\((3\sqrt{3})^{-x+1} =\frac{1}{3}*27^{x-5}\\( \sqrt{3^2*3}) ^{1-x}=\frac{1}{3} *((3)^3)^{x-5}\\(\sqrt{27} )^{1-x}=\frac{1}{3}*3^{3*(x-5)}\\ (\sqrt{3^3})^{1-x} =\frac{1}{3}*3^{3x-15}\\ 3^{\frac{3 }{2}*(1-x)}=\frac{1}{3}*3^{3x-15}\ |*3\\ 3*3^{\frac{3}{2}-\frac{3}{2}x}=3^{3x-15}\\ 3^{1+1,5-1,5}=3^{3x-15}\\ 3^{2,5-1,5x}=3^{3x-15}\ \ \ \ \Rightarrow\\2,5-1,5x=3x-15\\4,5x =17,5\ |*2\\9x=35\ |:9\\x=\frac{35}{9}.\)
O is the center of the regular octagon below. Find its area. Round to the nearest tenth if necessary.
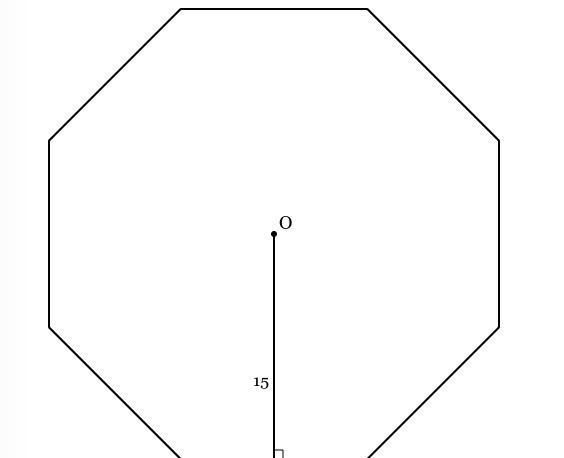
Answers
\(\underset{ \textit{angle in degrees} }{\textit{area of a regular polygon}}\\\\ A=na^2\cdot \tan\left( \frac{180}{n} \right) ~~ \begin{cases} n=sides\\ a=apothem\\[-0.5em] \hrulefill\\ n=8\\ a=15 \end{cases}\implies A=(8)(15)^2\tan\left( \frac{180}{8} \right) \\\\\\ A=1800\tan(22.5^o)\implies A\approx 745.6\)
Make sure your calculator is in Degree mode.
One day the level of the klater in the river klas 35cm above a mark. A week later it was 12cm below the mark. How far did the water level drop during the week?
Answers
If the level of the klater in the river klas 35cm above a mark. A week later it was 12cm below the mark. The water level drop during the week is 23 cm.
What is an arithmetic operation?It is defined as the operation in which we do the addition of numbers, subtraction, multiplication, and division. It has basic four operators that are +, -, ×, and ÷.
It is given that one day the latter's water level was 35 cm above the mark. It was 12 cm below the mark one week later.
We have to apply the subtraction operation for the given operation,
In mathematics, subtraction is defined as the difference between two quantities. The application of subtraction can be used broadly in different applications to find or solve the problems such as finding differences between two quantities and many more.
=35 - 12
=23 cm
Thus, if the level of the klater in the river klas 35cm above a mark. A week later it was 12cm below the mark. The water level drop during the week is 23 cm.
Learn more about the arithmetic operation here:
brainly.com/question/20595275
#SPJ1
Write the word sentence as an inequality. Then solve the inequality.
A number x divided by 7 is less than −3.
An inequality is
.
The solution of this inequality is
.
Answers
Answer:
x/7<-3
x<-21
Step-by-step explanation:
x/7<-3
multiply the seven to get rid of x denominator
x<-21
Answer:
x/7<-3x<-21
Step-by-step explanation:
HOPE IT HELPS :)
2. *** (204)+ 44) I V9+1 4. 3. Evaluate a3 – 5g + V34 - 30* if a = 2 and g = 3 ** Evaluate 9(2x + 7) + 8 + 777 + -3 2
Answers
For the part 1: the answer is 117, because:
\(((9+4)+(\frac{20}{2})^2)+2^2\)we first need to solve what is in the parenthesis:
\((13+10^2)+2^2\)know we simplify the exponents:
\((13+100)+4\)then we make the sum in the parenthesis:
\(113+4=117\)For the part 2: the answer is 33, because:
\((\frac{20}{4})^2+\sqrt[]{((14+8)+42)}\)we solve first what is in the parenthesis that have no more parenthesis inside them:
\(5^2+\sqrt[]{(22+42)}\)then we simplify the exponent and make the addition inside the square root:
\(25+\sqrt[]{64}=25+8=33\)For the part 3: the answer will be -5, because:
\(a^3-5g+\sqrt[]{34-30}\times(\frac{\sqrt[]{g+1}}{2})\)if a=2 ang g=3, we have that
\(=2^3-5(3)+\sqrt[]{34-30}\times(\frac{\sqrt[]{4}}{2})\)we will first solve the exponents and the square root:
\(=8-5(3)+\sqrt[]{4}\times(\frac{\sqrt[]{4}}{2})=8-5(3)+2\)we now solve the multiplication:
\(=8-15+2\)now, we make thee addition and the substraction:
\(=10-15=-5\)For the part 4: the answer will be 26, because:
\(9(2x+7)+8+\sqrt[]{77+4}\)when x=-3, the expression will be:
\(9(2(-3)+7)+8+\sqrt[]{77+4}\)we will first solve what is in the square root:
\(9(2(-3)+7)+8+\sqrt[]{81}=9(2(-3)+7)+8+9\)then we will solve the multiplication:
\(=9(-6+7)+8+9\)now we solve what's in the parenthesis:
\(=9(1)+8+9=9+8+9=26\)
Amaya is standing 30 ft from a volleyball net. The net is 8 ft high. Amaya serves the ball. The path of the ball is modeled by the equation y=-0.02(x-18)^2+12, where x is the ball's horizontal distance in feet from Amaya's position and y is the distance in feet from the ground to the ball. a. How far away is the ball from Amaya when it is at its maximum height? Explain. b. Describe how you would find the ball's height when it crosses the net at x=30. a. How far away is the ball from Amaya when it is at its maximum height? Explain. The ball is nothing ft away from Amaya when it is at its maximum height. Use the ▼ y-coordinate x-coordinate of the vertex.
Answers
Answer:
a) 30feet
b) 9.12feet
Step-by-step explanation:
a) Given the path of the ball is modeled by the equation y=-0.02(x-18)²+12, where;
x is the ball's horizontal distance in feet from Amaya's position
y is the distance in feet from the ground to the ball.
The ball will be at the maximum height when dy/dx = 0
Differentiate the function:
dy/dx = 2(-0.02)(x-18)^(2-1)+0
dy/dx = -0.04(x-18)
Equate the resulting expression to zero and find x as shown;
-0.04(x-18) = 0
Open the parentheses
-0.04x+0.04(18) = 0
-0.04x+0.72 =0
-0.04x = -0.72
x = -0.72/-0.04
x = 18ft
Hence the ball is 18ft away from Amaya when it is at its maximum height
b) To get the balls height y when x = 30, we will substitute x = 30 into the equation given and calculate the value of y as shown:
y=-0.02(x-18)²+12
y = -0.02(30-18)²+12
y= -0.02(12)²+12
y = -0.02(144)+12
y = -2.88+12
y = 9.12 feet
Hence the Ball's height is 9.12feet when it crosses the net at x = 30.
According to the graph, what is the value of the constant in the equation
below?
A. 0.25
B. 0.5
C. 1
D. 2
Answers
Answer:A
Step-by-step explanation:
Answer:
It is A
Step-by-step explanation:
i took the test and it showed it was corrected when i got done checking my test score please choose A.
question 3.. will give brainliest

Answers
Answer:
-0.5
Step-by-step explanation:

Answer:
-0.50
Step-by-step explanation:
4.75 + (-5.25)
Using a number line you can show the sum of points 4.75 and -5.25 is -0.50
1) Starting at 4.75 draw an arrow 5.25 units in the negative direction, arriving at -0.5.
((8y-3x)/(4))/(4)-(-7+5x)/(3)
Answers
Let's simplify step-by-step
8y-3x/4/4 -(-7+5x/3)
Distribute:
=-3/16 x +1/2 y+-5/3 x+7/3
Combine like terms:
=-3/16x+1/2 y +-5/3x+7/3
=(-3/16x+-5/3x)+(1/2y)+(7/3)
=-89/48x +1/2y+7/3
Answer: -89/48x+1/2y+7/3
Answer this for 20 POINTS

Answers
Answer: C
Step-by-step explanation: im sorry if its wrong creati deleted all my points and im at -92 points
Will give brainliest to right answer!!!

Answers
Answer:
f = 2
Step-by-step explanation:
Hope this helps you! :3
Answer:
f = 2
Step-by-step explanation:
2+1.25f = 10 - 2.75f
Add 2.75f to both sides
2 + 4f = 10
Subtract 2 on both sides
4f = 8
Divide by 4 on both sides to isolate the variable f
f = 8/4
f = 2