Alone, Brenda can dig a ditch in 5 hours. If Jack helps her, the two of them can dig
the ditch in 3 hours. How long would it take Jack to dig the ditch himself?
Brainliest if answered first
Answers
Answer:
7.5 hours
Step-by-step explanation:
1/5 in 1 hour and 1/3 in one hour
1/3-1/5 is 2/15
backwards is 15/2
7.5 hours
Hope this helps plz hit the crown ;D
Related Questions
Which of the following is not an exterior angle of triangle BHE

Answers
Answer:
I dont know
Step-by-step explanation:
I need help I am confused

Answers
1/3 = 0.3333… (the decimal 3 repeats forever)
.3 = 0.30
3% = 0.03
Therefore, in order of greatest to least, the numbers go from 3, 1/3, .3, 3%. The correct answer must be C.
Hope this was helpful and accurate! If you have any additional questions, feel free to ask.
Answer:
C. 3, 1/3, .3, 3%
Step-by-step explanation:
3 is a whole number that makes it the greatest
1/3 in a decimal form is 0.33 repeating
.3 is .30 which is smaller than previous.
3% converted in decimal form is 0.03 which is the smallest
can someone help me answer this

Answers
The number is 18 . A ) The equation is 12 + 10 x = 192 B ) x = 18 .
Linear equations are equations of the first order. The linear equations are defined for lines in the coordinate system. When the equation has a homogeneous variable of degree 1 (i.e. only one variable), then it is known as a linear equation in one variable. A linear equation can have more than one variable.
B ) Solving above equation 12 + 10 x = 192
10 x = 192 -12
10 x = 180
x = 18
To learn more on equations follow link :
https://brainly.com/question/2030026
#SPJ9
Two times the sum of a number and 8 is negative 48 what is the number?
Answers
Answer:
-32
Step-by-step explanation:
Let the number be x.
\(2(x+8)=-48 \\ \\ x+8=-24 \\ \\ x=-32\)
Look at the problem below.
p + q = 17
If p = 8, what is q?
B. II
A. 25
C. 9
D. 8
Answers
Answer:
C
Step-by-step explanation:
8+q=17
17-8=9
The answer is 9
You would replace p in the equation with 8, then subtract 8 from 17 leaving you with 9
Use Guauss-jordan elimination to solve the following system of equations.
3x + 5y = 7
6x - y = -8
Answers
Answer:
(-1,2)
Step-by-step explanation:
Write out the system in a matrix
3 5 7
2(6 -1 -8 ) Multiply row two by five and add to row one.
1 0 -1 Multiply row 1 by -6 and add it to row two.
6 -1 -8
1 0 -1
0 -1 -2 Multiply bottom row by -1
1 0 -1
0 1 2
x=-1, y=2
Duck #1 lays eggs whose weights are normally distributed with a mean of 70gramsand a standard deviation of 6 grams.Duck #2 lays eggs whose weights are also normally distributed with a mean of 65 grams and a standard deviation of 5 grams. If an egg is randomly chosen from each duck, what is the probability that Duck #2’s egg weighs more than Duck #1’s egg?
Answers
Answer:
26.11% probability that Duck #2’s egg weighs more than Duck #1’s egg
Step-by-step explanation:
When the distribution is normal, we use the z-score formula.
In a set with mean \(\mu\) and standard deviation \(\sigma\), the zscore of a measure X is given by:
\(Z = \frac{X - \mu}{\sigma}\)
The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
If an egg is randomly chosen from each duck, what is the probability that Duck #2’s egg weighs more than Duck #1’s egg?
Duck #2 egg will weigh more if the subtraction of duck's 2 egg by duck's 1 egg is larger than 0.
When we subtract normal distributions, the mean is the subtraction of the means. So
\(\mu = 65 - 70 = -5\)
The standard deviation is the square root of the sum of the variances. So
\(\sigma = \sqrt{6^2+5^2} = \sqrt{61} = 7.81\)
Now, we have to find 1 subtracted by the pvalue of Z when X = 0. So
\(Z = \frac{X - \mu}{\sigma}\)
\(Z = \frac{0 - (-5)}{7.81}\)
\(Z = 0.64\)
\(Z = 0.64\) has a pvalue of 0.7389
1 - 0.7389 = 0.2611
26.11% probability that Duck #2’s egg weighs more than Duck #1’s egg
Help me out please, been struggling and I would appreciate some help.

Answers
By using the given diagram, we can see that the ordered pair that represents point B is (-4, 0)
Which ordered pair represents B?Any point on a coordinate plane has a ordered pair associated to it, that tells us the location of the point (x, y).
For example, the intersection between the two axes is the point (0, 0).
The first value, x, represents the horizontal displacement, the second variable, y, represents the vertical displacement.
Here we can see that B is 4 units to the left of the center, then the ordered pair that represents point B is (-4, 0)
Learn more about ordered pairs:
https://brainly.com/question/1528681
#SPJ1
know how I can find the measurements in Bloxburg?
Answers
Answer:
I'm sorry, but there is no feature for this in this game. But, there is a grid and a sizing tool. You'll just have to make an educated guess based on the size of your character and your character's stage of life (baby, toddler, kid, teen, adult). Hope I helped! I once had the same question, but just did this. I think each big grid square maybe is 3-5 ft?
Every 2 centimeters on a floor plan represents
meters of the house. The dining room is 8 cm by
10 cm on the floor plan, and the bedroom is 6cm by10cm on the floor plan. If installing tile costs $34
per square meter and installing carpet costs $21 per
square meter, how much would it cost to install tile
in the dining room and install carpet in the bedroom?
Show your work.
Answers
Given statement solution is :- It would cost $680 to install tile in the dining room and $315 to install carpet in the bedroom.
To find the cost of installing tile in the dining room and carpet in the bedroom, we need to calculate the areas of both rooms first.
Given:
Every 2 centimeters on the floor plan represents 1 meter of the house.
Dining Room:
On the floor plan, the dining room is 8 cm by 10 cm.
Converting this to meters, the dimensions of the dining room are 8 cm / 2 = 4 meters by 10 cm / 2 = 5 meters.
The area of the dining room is 4 meters * 5 meters = 20 square meters.
Bedroom:
On the floor plan, the bedroom is 6 cm by 10 cm.
Converting this to meters, the dimensions of the bedroom are 6 cm / 2 = 3 meters by 10 cm / 2 = 5 meters.
The area of the bedroom is 3 meters * 5 meters = 15 square meters.
Now, let's calculate the costs.
Cost of Tile:
The cost of installing tile is $34 per square meter.
The area of the dining room is 20 square meters.
Therefore, the cost of installing tile in the dining room is 20 square meters * $34/square meter = $680.
Cost of Carpet:
The cost of installing carpet is $21 per square meter.
The area of the bedroom is 15 square meters.
Therefore, the cost of installing carpet in the bedroom is 15 square meters * $21/square meter = $315.
Therefore, it would cost $680 to install tile in the dining room and $315 to install carpet in the bedroom.
For such more questions on Cost of tile and carpet.
https://brainly.com/question/29574063
#SPJ8
A rectangular painting measures 13 inches by16 inches and contains a frame of uniformwidth around the four edges. The perimeter ofthe rectangle formed by the painting and itsframe is 82 inches. Determine the width of theframeWhat is the width of the frame?inch(es)

Answers
Since the perimeter of the painting and the frame is 82 inches we can write an expression to calculate it, considering that there is a part x over and below the painting.
Therefore the perimeter can be written:
(13+2x)+(13+2x)+(16+2x)+(16+2x)=82
26+4x+32+4x=82
58+8x=82
8x=82-58
8x=24
x=3
Given the fact that x is 3
Then the width of the frame is 16+ 2(3) = 22 inches
help me find the slope please
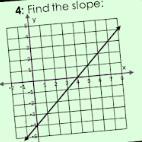
Answers
Answer:
3/4
Step-by-step explanation:
Ok, so I see that the x-intercept is (4,0), while the y-intercept is(-3,0)
Slope is rise/run
Between these two points, the line rose 3 and horizontally went 4 units to the right. Therefore, the slope is 3/4.
Feel free to tell me if I did anything wrong! :)
Also, if you're still confused, I could explain it another way.
A factory manufactures chairs and tables, each requiring the use of three operations: cutting, assembly, and finishing. The first operation can use at most 40 hours; the second at most 42 hours; and the third at most 25 hours. A chair requires 1 hour of cutting, 2 hours of assembly, and 1 hour of finishing; a table needs 2 hours of cutting, 1 hour of assembly, and 1 hour of finishing. If the profit is $20 per unit for a chair and $30 for a table, what is the maximum revenue? Round your answer to the nearest whole number. Do not include a dollar sign or comma in your answer.
Answers
Answer:
z(max) = 650 $
x₁ = 10 units
x₂ = 15 units
Step-by-step explanation:
That is a linear programming problem, we will use a simplex method to solve it
Formulation:
Let´s call x₁ number of chairs and x₂ number of tables then :
Item (in hours) cutting assembly finishing Profit ($)
Chairs (x₁) 1 2 1 20
Tables (x₂) 2 1 1 30
Availability 40 42 25
Objective Function
z = 20*x₁ + 30x₂ ( to maximize) subject to:
x₁ + 2x₂ ≤ 40
2x₁ + x₂ ≤ 42
x₁ + x₂ ≤ 25
x₁ , x₂ >= 0
Using excel or any other software we find:
z(max) = 650
x₁ = 10
x₂ = 15
The chairs and tables manufactured by the factory is an illustration of linear programming, where the maximum revenue is 674
Let x represent chairs, and y represent tables
So, the given parameters are:
Cutting:
Chairs: 1 hourTable: 2 hoursHour available: 40So, the constraint is:
\(\mathbf{x + 2y \le 40}\)
Assembly:
Chairs: 2 hoursTable: 1 hourHour available: 42So, the constraint is:
\(\mathbf{2x + y \le 42}\)
Finishing:
Chairs: 1 hourTable: 1 hourHour available: 25So, the constraint is:
\(\mathbf{x + y \le 25}\)
The unit profit on the items are:
Chairs: $20Table: $30So, the objective function to maximize is:
\(\mathbf{Max\ z = 20x + 30y}\)
And the constraints are:
\(\mathbf{x + 2y \le 40}\)
\(\mathbf{2x + y \le 42}\)
\(\mathbf{x + y \le 25}\)
\(\mathbf{x,y \ge 0}\)
Using graphical method (see attachment for graph), we have the following feasible points:
\(\mathbf{(x,y) = \{(10,15),\ (17,8),\ (14.67, 12.67)\}}\)
Calculate the objective function using the feasible points.
\(\mathbf{z = 20 \times 10 + 30 \times 15}\)
\(\mathbf{z = 650}\)
\(\mathbf{z = 20 \times 17 + 30 \times 8}\)
\(\mathbf{z = 580}\)
\(\mathbf{z = 20 \times 14.67+ 30 \times 12.67}\)
\(\mathbf{z = 673.5}\)
Approximate
\(\mathbf{z = 674}\)
Hence, the maximum revenue is 674
Read more about linear programming at:
https://brainly.com/question/14225202

given the equation
12x-4y=-20
a) solve for y if x=-2.
b) solve for y in general.
Answers
B) y=5
Because if you plug in -2 for x you get -24 then you move that over to the other side to get 4. Then divide- 4 by 4 to get -1.
For b just plug in zero for x and do the same process
Answer:
a) if x = 2, y = -1
b) y = 5
Step-by-step explanation:
12x - 4y = -20 Let's substitute x = 2
12(-2) - 4y = -20 Multiply
-24 - 4y = -20 Add 24 to each side
-4y = 4 Multiply each side by -1
4y = -4 Divide by 4
y = -1
now to solve for y in general:
12x - 4y = -20 Subtract 12x
-4y = -20 - 12x Divide by -4
y = 5 + 3x Sub 0
y = 5 + 3(0)
y = 5
what is the factored form of x^-9
Answers
Answer:
1/x^9
Step-by-step explanation:
I don't actually understand this question, but if you are asking (x^(-9)), then its the answer above
of its employees based on the number of sales per hour. One employee had the following sales for the last 20 hours 3 4 5 5 6 7 What is the median for the distribution of number of sales per hour? ____________ Express as a number
Answers
Explanation:
So they have given you the numbers 3, 4, 5, 5, 6, and 7. To find the median you have to put the numbers in numerical (which you already have) and then you cross each number out starting from either end, and then the number left in the middle , 5 is the median.
I hope this helps
Which derivation rule justifies the following argument? If n is a multiple of 8, then n is even. However, n is not even. Therefore, n is not a multiple of 8. O double negation O simplification De Morgan's Laws O modus ponens O modus tollens
Answers
Commutativity justifies the statement, of If n is a multiple of 8, then n is even. However, n is not even. Therefore, n is not a multiple of 8.
Commutativity is used in Maths equations and describes sums that can be moved around and will still give the same answer.
'Commutative' comes from the word 'commute' which means to move and travel around, so equations that are commutative have numbers that can be moved within the equation.
commutative law, in mathematics, is either of two laws relating to number operations of addition and multiplication that are stated symbolically as a + b = b + a and ab = ba.
To know more about Commutativity:
https://brainly.com/question/778086
#SPJ4
simplify 13¹/3+2¹/3-10/2
Answers
The simplified form of 13¹/3 + 2¹/3 - 10/2 is a fraction 64/6 that can be simplified by dividing both the numerator and denominator by their greatest common divisor, which is 2 the final answer is 32/3.
Given
13¹/3+2¹/3-10/2
Required simplified it =?
First, we have simplified both constants separately
13¹/3 = (3 * 13 + 1) / 3 = 40/3
2¹/3 = (3 * 2 + 1) / 3 = 7/3
now the expression become = 40/3 + 7/3 - 10/2
now taking LCM of 3 and 2 which is 6.
= 80/6 + 14/6 - 30/6
now we have to simplify this fraction by dividing by 2 = 64/6
Therefore, the simplified form of 13¹/3 + 2¹/3 - 10/2 is 32/3.
Learn more about Simplification here:
https://brainly.com/question/23509407
#SPJ1
what is 38.08÷23.80 i need help
Answers
Answer:
1.6
Step-by-step explanation:
i used calculator
Answer: 1.6
Step-by-step explanation:
3 1/2 inches so what is the area of this circle?

Answers
Answer:
19.6 in²
Step-by-step explanation:
area of circle = π r²
= π (3 1/2)²
= π (5/2)²
= π 25/4
= 22/7 x 25/4
= 275/14
=19.6 in²
Which sentence could be used to describe the equation 105 = 78 + y
Answers
Answer:
y = 22
Step-by-step explanation:
One hundred five is equal to seventy-eight plus y.
Answer:
I think answer A
Step-by-step explanation:
Im very sorry if thats wrong
A cylinder has a volume of 187 cubic inches. If the radius is 3 inches, what is the height of the cylinder in inches?
Someone please help
Answers
Answer:
Step-by-step explanation:
V = pi r^2 h
V = 187
pi = 3.14
r = 3
h = ?
187 = 3.14 * 3^2 * h
187 = 3.14 * 9 * h
187 = 28.26 * h
187 / 28.26 = h
h = 6.62
Answer:
The height of cylinder is 6.61 inches.
Step-by-step explanation:
Given that :- Volume of cylinder = 187 inches ³.Radius of cylinder, r = 3 inchesWe need to Find :-Height of cylinder , h Formula Used :-Volume of cylinder = π × r ² × h
Where,
r is the radius of cylinder. h is the height of cylinder.Solution :-Using Formula
Volume of cylinder = π × r ² × h
Substitute the values
187 inches ³ = π × ( 3 inches ) ² × h
Using π = 3.14
187 inches ³ = 3.14 × 9 inches ² × h
multiply
187 inches ³ = 28.26 inches ² × h
Divide , we get
187 inches ³ / 28.26 = h
6.61 inches = h
Therefore, The height of cylinder is 6.61 inches.
The sides of a triangle are 21 ,29 , and 11 . Use the Pythagorean Theorem to determine if the triangle is right, acute, or obtuse. The triangle is because the square of the largest side the sum of the squares of the other two sides.
Answers
The triangle is obtuse because 11^2 + 21^2 does not equal 29^2.
The Pythagorean Theorem states that the sum of the squares of the two shorter sides of a triangle must equal the square of the longest side in order for the triangle to be a right triangle.
We can use this theorem to determine the type of triangle by calculating the square of the longest side (29^2), and then comparing it to the sum of the squares of the other two sides (11^2 + 21^2). Since 11^2 + 21^2 does not equal 29^2, the triangle is obtuse.
The Pythagorean Theorem states that the sum of the squares of the two shorter sides of a triangle must equal the square of the longest side in order for the triangle to be a right triangle.
Learn more about triangle here
https://brainly.com/question/2773823
#SPJ4
A rectangular portrait is 1 yard wide and 2 yards high. It costs $30.00 per yard to put a gold frame around the portrait ¿how much will the frame cost?
Answers
The frame for the rectangular portrait will cost $180.00.
The rectangular portrait has a width of 1 yard and a height of 2 yards. To calculate the perimeter of the portrait, which represents the length of frame required, we can use the formula:
Perimeter = 2 * (Width + Height)
Substituting the values, we have:
Perimeter = 2 * (1 yard + 2 yards) = 2 * 3 yards = 6 yards
The cost of the frame is given as $30.00 per yard. To calculate the total cost of the frame, we multiply the length of the frame (in yards) by the cost per yard:
Frame Cost = Perimeter * Cost per yard = 6 yards * $30.00/yard = $180.00
Therefore, the frame for the rectangular portrait will cost $180.00.
For more such questions on cost , Visit:
https://brainly.com/question/2292799
#SPJ11
The scale on a map is 1:320000
What is the actual distance represented by 1cm?
Give your answer in kilometres.
Answers
By answering the presented question, we may conclude that Therefore, 1 expressions cm on the map corresponds to a real distance of 3.2 km.
what is expression ?In mathematics, an expression is a collection of integers, variables, and complex mathematical (such as arithmetic, subtraction, multiplication, division, multiplications, and so on) that describes a quantity or value. Phrases can be simple, such as "3 + 4," or complicated, such as They may also contain functions like "sin(x)" or "log(y)". Expressions can be evaluated by swapping the variables with their values and performing the arithmetic operations in the order specified. If x = 2, for example, the formula "3x + 5" equals 3(2) + 5 = 11. Expressions are commonly used in mathematics to describe real-world situations, construct equations, and simplify complicated mathematical topics.
Scale 1:
320000 means that 1 unit on the map represents his 320000 units in the real world.
To find the actual distance represented by 1 cm on the map, you need to convert the units to the same scale.
1 kilometer = 100000 cm
So,
1 unit on the map = 320000 units in the real world
1 cm on the map = (1/100000) km in the real world
Multiplying both sides by 1 cm gives:
1 cm on the map = (1/100000) km * 320000
A simplification of this expression:
1 cm on the map = 3.2 km
Therefore, 1 cm on the map corresponds to a real distance of 3.2 km.
To know more about expressions visit :-
https://brainly.com/question/14083225
#SPJ1
What is 8 times as many as 3 is
Answers
Answer:
24?
Step-by-step explanation:
Let f(x) =
= 6x2 + 5 and g(x) = 3x - 5. Find f(g(7)).
Answers
Answer:
1541
Step-by-step explanation:
1. Find g(7).
Plug in 7 for x in the function g(x).
3(7)-5
= 21 -5 = 16
2. Plug 16 into x for f(x).
6(16)^2 +5
= (256 x 6)+5 = 1541
62%
a) The nth term of a sequence is 2n - 3
Work out the first three terms of the sequence.
[2]
b) Write down an expression for the nth term of the following sequence.
9, 14, 19,
[2]
24,
BH
Answers
Answer:
(a) \(Sequence: -1, 1, 3\)
(b) nth term: \(T_n = 4+ 5n\)
Step-by-step explanation:
Solving (a)
Given
\(T_n = 2n - 3\)
The first 3 terms.
When n = 1
\(T_1 = 2*1 - 3\)
\(T_1 = 2 - 3\)
\(T_1 = -1\)
When n = 2
\(T_2 = 2*2 - 3\)
\(T_2 = 4 - 3\)
\(T_2 = 1\)
When n = 3
\(T_3 = 3*2 - 3\)
\(T_3 = 6 - 3\)
\(T_3= 3\)
So, the first three terms are:
\(Sequence: -1, 1, 3\)
Solving (b)
Given
\(Sequence: 9,14,19,24\)
Required
The nth term
The sequence is arithmetic.
So, first calculate the common difference (d)
\(d = 14 - 9\)
\(d = 5\)
The nth term is then calculated as:
\(T_n = a + (n - 1) * d\)
Where
\(a =First\ term\)
So:
\(a = 9\) and \(d = 5\)
\(T_n = 9 + (n - 1) * 5\)
\(T_n = 9 + 5n - 5\)
Collect like terms
\(T_n = 9 - 5+ 5n\)
\(T_n = 4+ 5n\)
King Soopers has two different sizes of sport drinks. Sigi wanted to make sure that he got the size with the better price. There was one that was 35 Fl Oz. for $1.29 and the other was 24 Fl Oz. for $1.20. Which size is the better buy?
I will give you brainliest
Answers
$1.20/(24 oz) = $0.05/oz
The 35-oz drink is a better buy.
Translate the given phrase into an algebraic expression and simplify if possible: the sum of -8 and -9, increased by 23.
Note: Enter only the simplified result.
Answers
Step-by-step explanation:
(-8-9)+23
-17+23
6.........
The value of algebraic expression [(-8) + ( -9)] + 23 is 6.
Here,
We have to simplify; the sum of -8 and -9, increased by 23.
And, find an algebraic expression.
What is Algebraic expression?
An algebraic expression in mathematics is an expression which is made up of variables and constants, along with algebraic operations.
Now,
The written expression is, the sum of -8 and -9, increased by 23.
It can be written as,
⇒ [(-8) + ( -9)] + 23
⇒ - 8 - 9 + 23
⇒ - 17 + 23
⇒ 6
Hence, The value of algebraic expression [(-8) + ( -9)] + 23 is 6.
Learn more about algebraic expression visit:
https://brainly.in/question/6578178
#SPJ2