Answers
Answer:
The answer to your query would be the Third Answer Choice.
Step-by-step explanation:
> First we need to find where the point (3,0) lies on the graph. We can do this by looking at the x coordinate, being 3, and the y, being 0. Keep in mind that the solution is where the two lines meet, not where the point on the graph is.
> This gets us to 3 on the x line, and no further. We then need to find which has an intersection of both lines on that point. If it is not (0,3), it is not the correct answer, which we are looking for.
> The first graph has a solution of (0, -1.5), as this is where the lines intersect. This is not (3, 0), so this is not our answer.
> The second graph’s lines intersect at (0,1), and since that is the solution of the graph, it is not the correct answer.
> The third graph’s lines intersect at (3, 0), and since this is it’s solution, it is the correct answer. Since we cannot be 100% sure, we still need to continue with checking the last graph.
> The fourth and last graph’s lines meet at (0,3), which is not what we are looking for, and is henceforth wrong.
> Once again, the correct answer is the Third Answer Choice. All the other graph’s have solutions on points other than (3,0), so they are not our answers.
> I hope this answered your query and any other questions you may have had on the subject. #LearningWithBrainly
Related Questions
Talwar wants to invest R5800 at simple interest rate of 12,2% per annum. How many years will it take for the money to grow to R26100
Answers
It will take approximately 28.67 years for Talwar's investment of R5,800 to grow to R26,100 at a simple interest rate of 12.2% per annum.
To calculate the number of years it will take for Talwar's investment to grow to R26,100 at a simple interest rate of 12.2% per annum, we can use the formula for simple interest:
Simple Interest = Principal × Rate × Time
Given that the principal (P) is R5,800, the rate (R) is 12.2% (or 0.122 as a decimal), and the desired amount (A) is R26,100, we need to find the time (T) it will take. Rearranging the formula, we get:
Time = (Amount - Principal) / (Principal × Rate)
Plugging in the values, we have:
Time = (R26,100 - R5,800) / (R5,800 × 0.122)
= R20,300 / R708.6
≈ 28.67 years
For more such questions on investment
https://brainly.com/question/29227456
#SPJ8
Eight more than four times a number is 28.
Choose the correct answer below.
A. 4x - 8 = 28
B. 4x + 8 = 28
C. 8x + 4 = 28
D. 8x - 4 = 28
E. 4(x + 8) = 28
Answers
A private jet flies the same distance in 14 hours that a commercial jet flies in 7 hours. If the speed of the commercial jet was 181mph less than 3 times the speed of the private jet, find the speed of each jet.
Answers
Answer:
Private Jet = 181 mph Commercial Jet = 362 mph
Step-by-step explanation:
private jet travels distance D in 14 hours
commercial jet travels distance D in 7 hours
so commercial jet speed is twice the private jet
y = 2x
commercial jet speed = 3 · (private jet speed) - 181 mph
y = 3x - 181
2x = 3x - 181
2x - 3x = -181
-x = -181
x = 181
y = 2x
y = 362
362 = 3(181) - 181
A can of tuna has a diameter of 4 Inches and a helght of 3 inches. What is the volume of the can of tuna? (Use 3.14 for
TT.)
37.68 in 3
150.72 in. 3
56.52 in 3
113.04 in. 3
Answers
Answer:
37.68 Inches cubed (A)
Step-by-step explanation:
The formula for a cylinder is:
πr^2(h)
First, let's find the radius, which is:
4/2 = 2
Now, we can plug in the values:
3.14(2)^2(3)
Do exponents first:
3.14(4)(3)
Multiply left to right:
12.56(3)
Again:
37.68 Inches
So, the answer is:
37.68 Inches cubed (Or A)
Hope this helps!
What is the midline equation of y = -4sin (2x - 7) + 3?
Answers
Step-by-step explanation:
Thank you this is your answer
Write and solve a proposition that the teacher can use to estimate how many students in the whole school would choose the aquarium.

Answers
Answer:
Let's assume that the total number of students in the school is "x". We can create a proportion to estimate how many students would choose the aquarium based on the given information:
Number of students who chose aquarium / Total number of students in the school = Percentage of students who chose aquarium / 100
We can plug in the values we know:
80 / x = p / 100
where "p" is the percentage of students who would choose the aquarium if the entire school were surveyed.
We can solve for "x" by cross-multiplying and simplifying:
8000 = px
x = 8000 / p
Now, we need to estimate the value of "p". We can do this by finding the average percentage of students who chose the aquarium, science center, planetarium, and farm:
(80 + 60 + 30 + 40) / x = (210 / x) = Average percentage of students who chose an attraction
This tells us that, on average, 210 out of every "x" students would choose one of the attractions. We can estimate that a similar percentage of the entire school would choose the aquarium:
p / 100 = 80 / 210
p = 38.1
So, we can estimate that approximately 38.1% of the students in the whole school would choose the aquarium. To find the estimated number of students who would choose the aquarium, we can plug in this value for "p" in our original proportion:
80 / x = 38.1 / 100
Cross-multiplying and solving for "x", we get:
x = 209.71
Rounding to the nearest whole number, we can estimate that approximately 210 students in the whole school would choose the aquarium.
Step-by-step explanation:
Answer:
The teacher surveyed a total of:
80 + 60 + 30 + 40 = 210 students.
If we assume that the sample of 210 students surveyed is representative of the entire population of 1000 students at Lake Middle School, we can use the relative frequency of students choosing the aquarium in the sample to estimate the number of students in the whole school who would choose the aquarium.
The relative frequency of students choosing the aquarium in the sample is:
80/210 = 0.38
This means that approximately 38% of the students in the sample chose the aquarium.
To estimate the number of students in the whole school who would choose the aquarium, we can multiply the relative frequency by the total number of students at the school:
0.38 x 1000 = 380
Therefore, the teacher can estimate that approximately 380 students out of 1000 at Lake Middle School would choose the aquarium for a field trip.
In ΔTUV, u = 3.4 inches, m∠U=144° and m∠V=35°. Find the length of v, to the nearest 10th of an inch.
Answers
The length of v to the nearest 10th of an inch is 2.0 inches.
What is a triangle?A triangle is a 2-D figure with three sides and three angles.
The sum of the angles is 180 degrees.
We can have an obtuse triangle, an acute triangle, or a right triangle.
We have,
We can use the Law of Sines,
Which states that for any triangle ABC:
a/sin(A) = b/sin(B) = c/sin(C)
where a, b, and c are the lengths of the sides of the triangle opposite the angles A, B, and C.
Now,
a = UV = u = 3.4 inches
A = ∠TUV
= 180° - m∠U - m∠V
= 180° - 144° - 35°
= 1°
B = ∠UTV
= m∠U
= 144°
C = ∠TVU
= m∠V
= 35°
Using the Law of Sines,
v/sin(C) = u/sin(B)
Substituting in the values.
v/sin(35°) = 3.4/sin(144°)
Solving for v.
v = (3.4 × sin(35°)) / sin(144°)
v = 1.97 inches
Therefore,
The length of v to the nearest 10th of an inch is 2.0 inches.
Learn more about triangles here:
https://brainly.com/question/25950519
#SPJ9
Answer Quick Please I need this asap

Answers
A half of a line = 180 degrees
So 100+x=180
180-100=80
80=x
Graph makes no sense to me

Answers
Answer:
(a) 0
(b) 0
(c) 1
The function is not continous at x = 9
Step-by-step explanation:
To the left and right of the point x = 9, the graph would seem to continue at f(x) = 0, however on the exact point x = 9 there is a hole in the graph allowing it to be equal to 1. Due to the hole in the graph, it is not continuous
Apologies if this is wrong its been a bit since I did calculus
∆ABC goes through a sequence of transformations to form ∆A′B′C′. The sequence of transformations involved is a
Answers
The sequence of transformations involved is B) translation by 2 units to the right and followed by a B) reflection across the y-axis.
How to carry out transformations?
The sequence of transformations involved:
From all the information given, it is discovered that the sequence of transformations involved in transforming ABC to A'B'C' is translation and reflection.
ABC is translated by 2 units to the right, it is shown in the graphThereafter the next transformation that followed is a reflection across the y-axisThere is no rotation.Thus, from the graph it is clear that the sequence of transformations involved is B) translation by 2 units to the right and followed by a B) reflection across the y-axis.
Complete question is;
ABC goes through a sequence of transformations to form A’B’C’. The sequence of transformations involved is a:
A) rotation 180 degrees counterclockwise about the origin
B) translation 2 units to the right
C) translation 4 units to the left
D) reflection across the line y=x
followed by a:
A) reflection across the x-axis
B) reflection across the y-axis
C) rotation 90 degrees clockwise about the origin
D) translation 4 units to the right
Read more about Transformations at; https://brainly.com/question/4289712
#SPJ1

2) | 10+ 9 | - 6-23
Absolute value
Answers

If there are 80 calories in 2/3 of an apple, How many calories are in 6 apples?
Answers
Answer:
Around 570 to 560.
A: 49
B: 33
C: 57
D: 16

Answers
Answer:
d
Step-by-step explanation:
i believe its 49 - 33= answer bc 49=49
Answer:
16°
Step-by-step explanation:
The two angles are vertical, meaning that they are the same
33 + 16 = 49
why does gradient normal x gradient tangent = -1 ??
Answers
Answer:
Gradient normal x gradient tangent = -1 is a mathematical expression that relates the normal and tangent vectors of a curve in three-dimensional space. The gradient of a scalar function is a vector that points in the direction of the greatest rate of increase of the function. The normal vector is perpendicular to the surface defined by the function, and the tangent vector is a vector that lies on the surface and points in the direction of the curve.
In mathematics, the cross product of two vectors is a vector that is orthogonal to both vectors, and the magnitude of the cross product is equal to the product of the magnitudes of the two vectors multiplied by the sine of the angle between them. In this case, the normal and tangent vectors of a curve form an orthogonal basis, meaning that they are perpendicular to each other. The expression gradient normal x gradient tangent = -1 says that the magnitude of the cross product of the normal and tangent vectors is equal to -1. This means that the normal and tangent vectors form a right-handed coordinate system, where the cross product is negative because the normal vector points in the opposite direction to the positive z-axis.
The ratio of cats to dogs at the veterinarian is 21:39. What percent of the total animals are cats?
Answers
Answer:
35%
Step-by-step explanation:
Assuming that there are no other animals in the vet other than dogs and cats, that means the total amount of animals is 21 + 39, which is 60 animals. To find the percent of cats, simply place the number of cats based on the ratio (21) over the total number of animals (60) and multiply by a 100:
21/60 = 0.35
0.35 x 100 = 35%
Hope this helps!!
Answer:
35%.
Step-by-step explanation:
Fraction that are cats = 21 / (21+39)
= 21/60
= 100 * 21/60
= 35%.
Emergency please it’s due in 20 mins




Answers
Answer:
12.11
Step-by-step explanation:
all you have to do is add them together (this is for the first page)
11.34 + 0.77 = 12.11
.........
Wich if the following is the slope of a line that passes through the points below ? Show all work

Answers
Answer: m = -1/2
Use (y2-y1)/(x2-x1) to find the slope.
Suppose a certain capsule is manufactured so that the dosage of the active ingredient follows the distribution Y ~ N(μ = 10 mg, σ = 1 mg). A dosage of 13mg is considered dangerous. Compute the probability that Y falls into the dangerous region.
a. 0.0013.
b. 0.013.
c. 0.9987.
d. 0.
Again suppose a certain capsule is manufactured so that the dosage of the active ingredient follows the distribution Y ∼ N(μ=10, σ=1), where units are in mg. A dosage of 13mg is considered dangerous. If we sample 49 capsules at random, compute the probability that the mean Y-bar falls into the dangerous region and pick the closest answer below.a. 0.0013.
b. 0.013.
c. 0.9987.
d. 0.
Answers
Answer:
(a) Probability that Y falls into the dangerous region is 0.0013.
(b) Probability that the mean Y-bar falls into the dangerous region is 0.00001.
Step-by-step explanation:
We are given that a certain capsule is manufactured so that the dosage of the active ingredient follows the distribution Y ~ N(μ = 10 mg, σ = 1 mg).
A dosage of 13 mg is considered dangerous.
Let Y = dosage of the active ingredient
The z-score probability distribution for normal distribution is given by;
Z = \(\frac{ Y-\mu}{\sigma} } }\) ~ N(0,1)
where, \(\mu\) = population mean = 10 mg
\(\sigma\) = standard deviation = 1 mg
(a) Probability that Y falls into the dangerous region is given by = P(Y \(\geq\) 13 mg)
P(Y \(\geq\) 13 mg) = P( \(\frac{ Y-\mu}{\sigma} } }\) \(\geq\) \(\frac{ 13-10}{1} } }\) ) = P(Z \(\geq\) 3) = 1 - P(Z < 3)
= 1 - 0.9987 = 0.0013
The above probability is calculated by looking at the value of x = 3 in the z table which has an area of 0.9987.
(b) We are given that a dosage of 13 mg is considered dangerous. And we sample 49 capsules at random.
Let \(\bar Y\) = sample mean dosage
The z-score probability distribution for sample mean is given by;
Z = \(\frac{\bar Y-\mu}{\frac{\sigma}{\sqrt{n} } } } }\) ~ N(0,1)
where, \(\mu\) = population mean = 10 mg
\(\sigma\) = standard deviation = 1 mg
n = sample of capsules = 49
So, Probability that the mean Y-bar falls into the dangerous region is given by = P(\(\bar Y\) \(\geq\) 13 mg)
P(Y \(\geq\) 13 mg) = P( \(\frac{\bar Y-\mu}{\frac{\sigma}{\sqrt{n} } } } }\) \(\geq\) \(\frac{13-10}{\frac{1}{\sqrt{49} } } } }\) ) = P(Z \(\geq\) 21) = 1 - P(Z < 21)
= 0.00001
What is the angle relationship?

Answers
Simplify by dividing negative five over eight ÷ negative three over four.. (5 points)
a
twenty over twenty-four
b
negative twenty over twenty-four
c
negative fifteen over thirty-two
d
fifteen over thirty-two
Answers
Answer:
b
Step-by-step explanation:
correct trust me
Answer:
The answer is A
Step-by-step explanation:
We all know that when divideing two negative numbers we always get a positive answer so with that in mind we can continue...first by using a method of "keep,change, and flip" this means you have to keep -5/8 the same then change the divideing sign to a multiplication sign and finally you have to flip -3/4 to -4/3 like this:
-5/8 x -4/3 =
Lets take away the negative signs since we know our answer would be positive anyway...
5/8 x 4/3 =
Then we proceed as we normally would with this equation ( 5 x 4 = 20 and
8 x 3 = 24)
5/8 x 4/3 = 20/24
So, our answer is twenty over twenty-four which is A.
A triangle has two side lengths of 6 cm and 4 cm. Select all the lengths that could be the third side.
Answers
The third side of the triangle can have any one of the following lengths:
2 cm < third side < 10 cm.
The triangle inequality theorem, which asserts that the sum of the lengths of any two sides of a triangle must be greater than the length of the third side, must be applied in order to find the potential lengths of the third side of the triangle.
We have sides of 6 cm and 4 cm in this instance. Hence, the third side's length must be smaller than the sum of the first two sides and bigger than the difference between them.
Contrast: 6 - 4 = 2 cm
Sum: 6 + 4 = 10 cm
As a result, the following are potential lengths for the triangle's third side:
Third side: 2 cm; length: 10 cm
Hence, the third side could be any length that falls within the range of 2 cm and 10 cm (exclusive).
To know more about Length visit:
https://brainly.com/question/30100801
#SPJ9
sat question I can’t seem to understand fully.

Answers
The value of m + n is given as follows:
A. 18.
How to obtain the value of m + n?The exponential expression for this problem is defined as follows:
\((x^5y^6)^{\frac{1}{5}}(x^3y^4}^{\frac{1}{4}}\)
Applying the power of power rule, we have that the exponents are given as follows:
x: 5/5 + 3/4 = 1 + 3/4 = 7/4.y: 6/5 + 4/4 = 6/5 + 1 = 11/5.Then the values of m and n are given as follows:
m = 7, n = 11.
Thus the sum is given as follows:
m + n = 7 + 11 = 18.
More can be learned about exponent rules at https://brainly.com/question/11975096
#SPJ1
What is the value of 7 in number 3,407,215
Answers
Answer:
7=7000
Step-by-step explanation:
To determine what the digit 7 means in 3,407,215, if we put 3,407,215 into the place value chart, we can see that the 7 appears in the units column of the thousands period.
So the digit 7 in this number means 7 thousands.
Image below.
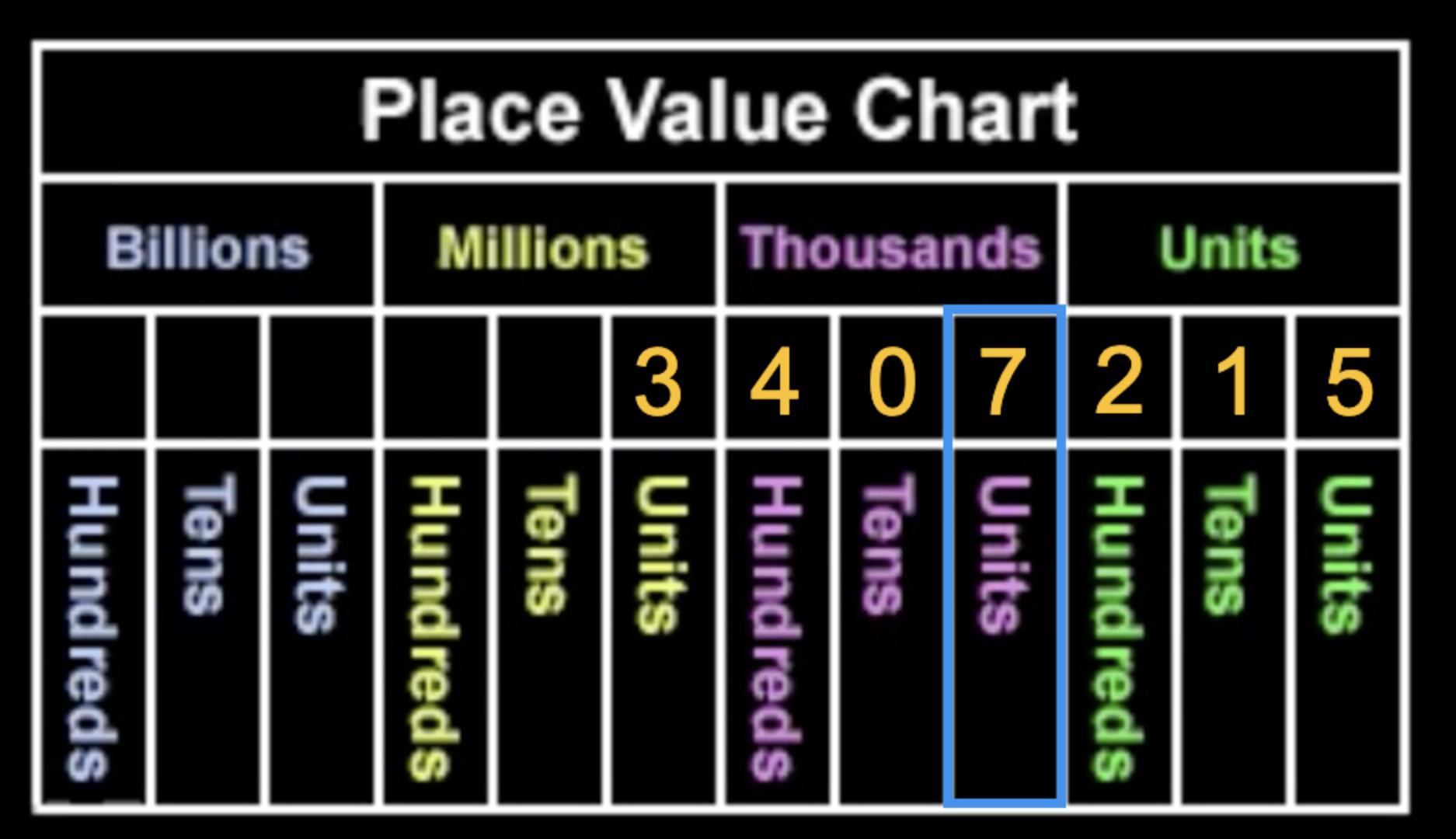
ASAP
Divide The quantity 3.185 times ten raised to the seventeenth power end quantity divided by the quantity 9.1 times ten raised to the eighth power end quantity.. Write the final answer in scientific notation.
3.5 x 108
0.35 x 109
3.5 x 109
0.35 x 1025
Answers
The division of the numbers in indices will be B.
0.35 × 10^9
How to compute the value?It should be noted that the information is about division of indices.
This will be:
(3.185 × 10^17) / (9.1 × 10^8)
= 0.35 × 10^9
In conclusion, the correct option is B.
Learn more about division on:
brainly.com/question/25289437
#SPJ1
Which ratio is greater than 5/8 ? A. 12/24 B. 3/4 C. 15/24 D. 4/12
Answers
B is the 3/4 = .75 and 5/8 = .625
Please help!!! Suppose we have two functions...

Answers
Answer:
4
Step-by-step explanation:
To find f(-1)g(-1) which is f(-1)*g(-1), first, we must find what are values of f(-1) and g(-1) first which can be found by substituting x = -1 in both functions.
\(\displaystyle{f(-1)=(-1)^2+1}\\\\\displaystyle{f(-1)=1+1 = 2}\)
Now we know that f(-1) is 2, next, we find g(-1).
\(\displaystyle{g(-1) = 3(-1)+5}\\\\\displaystyle{g(-1)=-3+5 = 2}\)
Now we know that g(-1) is 2 too. Since the question wants to find f(-1)g(-1), therefore 2*2 = 4.
Hence, the answer is 4.
Answer:
D) 4
Step-by-step explanation:
Given functions:
f(x) = x² + 1
g(x) = 3x + 5
Here, we're asked to find the value of both functions when the value of x is -1. Then, we multiply the functions in order to find the value of f(-1)•g(-1).
Note: f(-1) • g(-1) is equivalent to (f • g)(-1).
Method 1: f(-1) • g(-1)
Find the value of each function when x = -1.
f(-1) = (-1)² + 1 ⇒ f(-1) = 1 + 1 ⇒ f(-1) = 2
g(-1) = 3(-1) + 5 ⇒ g(-1) = -3 + 5 ⇒ g(-1) = 2
Multiply the functions together.
f(-1) • g(-1) ⇒ 2 • 2 ⇒ 4
Method 2: (f • g)(-1)
First, multiply the functions.
⇒ (x² + 1) • (3x + 5) [ Simplify using the Distributive Property: (a + b) • (c + d) = ac + ad + bc + bd. ]
⇒ x²(3x) + x²(5) + 1(3x) + 1(5)
⇒ 3x³ + 5x² + 3x + 5
Now, substitute -1 as the value of x.
⇒ 3(-1)³ + 5(-1)² + 3(-1) + 5 [ Exponents first. }
⇒ 3(-1) + 5(1) -3 + 5 [ Multiply the numbers. ]
⇒ -3 + 5 - 3 + 5 [ Simplify. ]
⇒ 4
Therefore, the product of both functions when x = -1 is 4.
Learn more about multiplying functions here:
brainly.com/question/12122417
brainly.com/question/16287318
\(x^{2}\) = 6
Answers
Answer:
Take the root of both sides and solve.
\(x = \sqrt{6} , - \sqrt{6} \)
Answer:
Take the root of both sides and solve:
\(x = \sqrt{6}, - \sqrt{6} \)
A grass seed company conducts a study to determine the relationship between the density of seeds planted (in pounds per 500 sq ft) and the quality of the resulting lawn. Eight similar plots of land are selected and each is planted with a particular density of seed. One month later the quality of each lawn is rated on a scale of 0 to 100. The sample data are given below where x denotes seed density, and y denotes lawn quality.x 1 1 2 3 3 3 4 5y 30 40 40 40 50 65 50 50The sample linear correlation coefficient is r=0.600. At the 1% significance level, do the data provide sufficient evidence to conclude that seed density and lawn quality are positively linearly correlated?
Answers
Answer:
I think it -1.50 to 10.58
Step-by-step explanation:
the temperature is -56F. How many degreees below zero is the temperature?
Answers
The number of degrees below zero is given by A = 56° F
What is Modulus Function?Regardless of the sign, a modulus function returns the magnitude of a number. The absolute value function is another name for it.
It always gives a non-negative value of any number or variable. Modulus function is denoted as y = |x| or f(x) = |x|, where f: R → (0,∞) and x ∈ R.
The value of the modulus function is always non-negative. If f(x) is a modulus function , then we have:
If x is positive, then f(x) = x
If x = 0, then f(x) = 0
If x < 0, then f(x) = -x
Given data ,
Let the initial temperature be represented as T
Let the number of degrees below zero be A
Now , the value of T is
T = -56° F
From the modulus function , we get
The value of the modulus function is always non-negative.
So , the measure of A = | T |
A = | -56 |
A = 56° F
Hence , the number of degrees below zero is 56° F
To learn more about modulus function click :
https://brainly.com/question/13682596
#SPJ9
Does anyone know what the answer is?

Answers
Answer:
0.974.
Step-by-step explanation:
You have to use a correlation calculator.

