Find the area of the surface, using polar coordinates, of the portion of the sphere x² + y² + z² = a², a > 0, between the places z = 2 and z = -2. Sketch clearly, the portion of the sphere and the region in the xy - plane.
Answers
The surface area of the portion of the sphere between z = 2 and z = -2 is 4πa²/3
How to determined area of the sphere?To find the area of the portion of the sphere between z = 2 and z = -2, we can use polar coordinates.
First, let's sketch the sphere and the region in the xy-plane.
The sphere x² + y² + z² = a² is a three-dimensional object, but we can draw its projection onto the xy-plane, which is a circle of radius a.
The region between z = 2 and z = -2 is a band that extends above and below the circle.
To find the limits of integration in polar coordinates,
we can use the equation of the sphere in terms of polar coordinates:
x = r cos θ sin φ
y = r sin θ sin φ
z = r cos φ
where r is the distance from the origin, θ is the angle in the xy-plane measured counterclockwise from the positive x-axis, and φ is the angle between the positive z-axis,
The vector from the origin to the point (x, y, z).
We want to integrate over the portion of the sphere
between z = 2 and z = -2.
This means that -2 ≤ r cos φ ≤ 2, or -2/r ≤ cos φ ≤ 2/r.
Since a is the radius of the sphere, we have r ≤ a.
The area element in polar coordinates is dA = r dr dθ.
The surface area of the portion of the sphere between z = 2 and z = -2 is then given by:
A = ∫∫dA = ∫[0,2π]∫[-2/a,2/a] r dr dθ
= 2π ∫[-2/a,2/a] r dr
= π(2/a)² - π(-2/a)²
= 4πa²/3
Therefore, the surface area of the portion of the sphere between z = 2 and z = -2 is 4πa²/3.
Learn more about area of the sphere
brainly.com/question/13568747
#SPJ11
Related Questions
I NEED HELP FAST PLEASE ASAP!!!

Answers
Answer:
Lines A and B
Lines D and E
Step-by-step explanation:
You can tell from the direction of the arrows. They represent parallel.
can someone plz plz plz help me choose an answer for this idk what it is
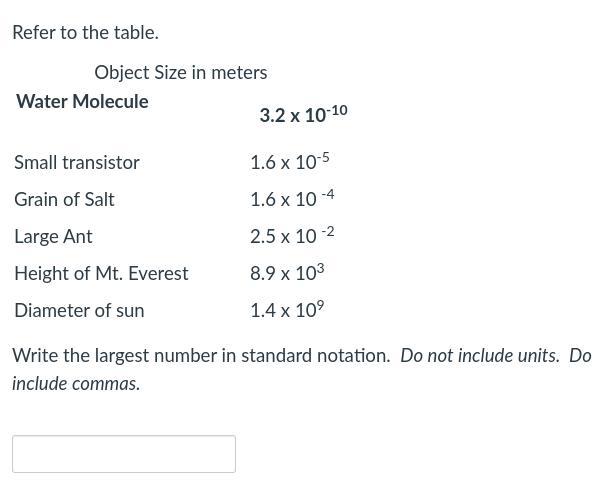
Answers
Answer:
Largest number ins scientific notation: 1.4 x 10⁹ (Diameter of sun)
Step-by-step explanation:
We need to find the largest number ins scientific notation.
The number which has highest degree would be the largest.
In the given options, Diameter of Sun has highest power i.e 10⁹
All other options have lower degrees.
So, largest number ins scientific notation: 1.4 x 10⁹ (Diameter of sun)
What is the area, in square centimeters, of the shaded part of the rectangle below?

Answers
Answer:
Step-by-step explanation:
17*8= the entire rectangle which is 136
Area of triangle=(base*height)divided by 2
The base of the white triangle is 4 because 8-4=4 and the height is 17
17*4=68
68/2=34
34= the area of the triangle
Area of the rectangle-area of the triangle=102
102 is your answer.
hopefully i did this right:)
for simultaneous equations in variables x and y d x² = 49 Dy= -63, D= 7 then what is the value of x?
Answers
Step-by-step explanation:
hope it will be helpful to you

Simplify the following algebraic fraction. Write the answer with positive exponents. v-3-w -W V+W Select one: V+w O a. v3w "(v3-14 V+W Ob. VW O c. w4_13 vw (v+w) O d. 1 3** 4 O e. v4+w
Answers
The simplified form of the algebraic fraction (v^-3 - w)/(w(v + w)) is (v^4 + w).
To simplify the fraction, we start by multiplying both the numerator and the denominator by v^3 to eliminate the negative exponent in the numerator: (v^-3 - w)(v^3)/(w(v + w))(v^3) This simplifies to: 1 - wv^3/(w(v + w))(v^3)
Next, we cancel out the common factors in the numerator and denominator: 1/(v + w) Finally, we simplify further by multiplying the numerator and denominator by v^4: v^4/(v + w) Therefore, the simplified form of the algebraic fraction is v^4 + w.
Learn more about algebraic fraction here: brainly.com/question/11525185
#SPJ11
A rectangle is 8m longer than it is wide. If the length is increased by 2m and the width is increased 2m, the area is
increase by 68m 2. Find the original dimensions.
Answers
Answer:
width=x
length=x+8
area= x(x+8)
new width =x+2
new length=x+10
New Area = (x+2)(x+10)
(x+2)(x+10)-x(x+8)= 68
after solving:
4x=68-20
4x=48
x=12
width=12m
length=12+8=20m
Answer: what
Step-by-step explanation:
. You want to buy some underwear. A
package of 8 costs $3.12. A package of 15
costs $6.15. A package of 20 costs $7.60.
Which package is the best buy?

Answers
Answer:
the second
Step-by-step explanation:
Using either logarithms or a graphing calculator, find the time required for the initial amount to be at least equal to the final amount. $3000, deposited at 6% compounded quarterly, to reach at least $4000 The time required is year(s). (Type an integer or decimal rounded to the nearest hundredth as needed.)
Answers
The time required for $3000, deposited at 6% compounded quarterly, to reach at least $4000 is approximately 6.59 years.
To find the time required for the initial amount of $3000 to reach at least $4000 when compounded quarterly at an interest rate of 6%, we can use the compound interest formula and solve for time.
The compound interest formula is given by:
A = P(1 + r/n)^(nt),
where A is the final amount, P is the principal amount (initial deposit), r is the interest rate (in decimal form), n is the number of compounding periods per year, and t is the time in years.
In this case, we have:
A = $4000,
P = $3000,
r = 6% = 0.06 (converted to decimal form),
n = 4 (quarterly compounding),
and we need to solve for t.
Rearranging the formula, we get:
t = (1/n) * log(A/P) / log(1 + r/n).
Substituting the given values into the formula and solving for t:
t = (1/4) * log(4000/3000) / log(1 + 0.06/4) ≈ 6.59 years.
Therefore, the time required for the initial amount of $3000 to reach at least $4000, compounded quarterly at 6%, is approximately 6.59 years
Learn more about compound interest here:
brainly.com/question/14295570
#SPJ11
In circle V, m/WVX = 97°. Solve for z if mW X = (3x +49)°. If necessary,
round your answer to the nearest tenth.
30

Answers
Answer:
x = 16
Step-by-step explanation:
the measure of an arc has the same measure as the central angle that intercepts it , that is
3x + 49 = 97 ( subtract 49 from both sides )
3x = 48 ( divide both sides by 3 )
x = 16
rationalise the denominator
\( 1\div 7 + 3 \sqrt{2} \)
Answers
The final answer is (1 + 21√2)/(21√2). The process of rationalization involves changing the form of an expression to eliminate radicals from its Denominator, or to eliminate denominators from a radical expression.
To rationalize the denominator 1/7 + 3√2,
A rational number is a number that can be expressed as a ratio of two integers, with the denominator not equal to zero. The fraction 4/5, for example, is a rational number since it can be expressed as 4 divided by 5.
Step-by-Step SolutionTo rationalizes the denominator 1/7 + 3√2, we'll need to follow these steps.
Step 1: First, we need to create a common denominator for the two terms. The common denominator is 7. Thus, we can convert the expression to the following form:(1/7) + (3√2 × 7)/(7 × 3√2).
Step 2: Simplify the denominator to 7. (1/7) + (21√2)/(21 × 3√2).
Step 3: The numerator and denominator can now be simplified. (1 + 21√2)/(7 × 3√2).Step 4: Simplify further. (1 + 21√2)/(21√2).We have successfully rationalized the denominator!
The final answer is (1 + 21√2)/(21√2).
The final answer is (1 + 21√2)/(21√2). The process of rationalization involves changing the form of an expression to eliminate radicals from its denominator, or to eliminate denominators from a radical expression.
For more questions on Denominator.
https://brainly.com/question/20712359
#SPJ8
You plan to purchase a house for 12.5 million baht, using a 30-year mortgage. You will make a down payment of 15 percent of the purchase price and you will not pay off the mortgage early. Ignore taxes in your analysis. Your bank offers you the following two options for payment.
Option 1: Mortgage rate of 7.25 percent and zero points.
Option 2: Mortgage rate of 6.75 percent and 4 points.
You would choose option ------- (1 or 2) because the Net Present Value of choosing option 2 is------ . (The answer can be negative. Do not round intermediate calculations and round your answer to two decimal places, e.g., 32.16.)
Answers
Option 2 has a lower NPV and thus should be chosen as it results in less total payment and better savings. Hence, you would choose option 2 as the Net Present Value of choosing option 2 is -9,903,615.48.
Given:
Purchase price = 12.5 million baht
Down payment = 15%30-year mortgage
We have to calculate the option which is best for us.
Option 1: Mortgage rate = 7.25%
Option 2:Mortgage rate = 6.75%
(a) Monthly Payment of Option 1:
Loan Amount = 12.5 * (100-15)%
= 10,625,000 baht
n = 30 * 12
= 360
i = 7.25%/12
= 0.006042857
Yearly Interest Paid = i * 10,625,000
= 64,515.48 baht
Monthly Interest Paid = 64,515.48 / 12
= 5,376.29 baht
EMI =\([P x R x (1+R)^n] / [(1+R)^n-1]\)
where P = Loan Amount, R= Interest Rate per month, n= total number of payments.
EMI = \([10,625,000 x 0.006042857 x (1+0.006042857)^360] / [(1+0.006042857)^360-1]\)
EMI = 69,677.31 baht
(b) Monthly Payment of Option 2:
Loan Amount = 12.5 * (100-15)%
= 10,625,000 baht
n = 30 * 12 = 360
i = 6.75%/12 = 0.005625
Yearly Interest Paid = i * 10,625,000
= 59,765.63 baht
Monthly Interest Paid = 59,765.63 / 12
= 4,980.47 baht
Point cost = 4% of Loan Amount
= 10,625,000 x 4/100
= 425,000 baht
Loan Amount after Deducting Points = 10,625,000 - 425,000
= 10,200,000 baht
EMI = \([P x R x (1+R)^n] / [(1+R)^n-1]\)
where P = Loan Amount, R= Interest Rate per month, n= total number of payments.
EMI =\([10,200,000 x 0.005625 x (1+0.005625)^360] / [(1+0.005625)^360-1]\)
EMI = 66,128.92 baht
(c) We have to calculate the NPV of the payments to decide which option is better.
We use the formula to calculate NPV:-
NPV = - \([B + (PMT / i) * (1 - (1 + i)^-n)] / ((1 + i)^t)\)
Where B = amount borrowed, PMT = monthly payment, i = interest rate, n = total number of payments, t = year.
NPV =\(- [10625000 + (69677.31 / 0.006042857) * (1 - (1 + 0.006042857)^-360)] / ((1 + 0.006042857)^30)\)
= -9,892,571.85 baht
NPV = \(- [10200000 + (66128.92 / 0.005625) * (1 - (1 + 0.005625)^-360)] / ((1 + 0.005625)^30)\)
= -9,903,615.48 baht
Know more about the Net Present Value
https://brainly.com/question/13228231
#SPJ11
-3xsquared -12x -23= y-8 use complete ig the square to find the vertex of the parabola
Answers
The vertex of the parabola represented by the equation -3x² - 12x - 23 = y - 8 is (-2, -63).
To find the vertex of the parabola using completing the square, we can rewrite the equation in the form:
\(y = a(x - h)^2 + k\)
Where (h, k) represents the coordinates of the vertex.
Let's complete the square for the given equation:
\(-3x^2 - 12x - 23 = y - 8\)
First, we'll move the constant term to the right side of the equation:
\(-3x^2 - 12x = y - 8 + 23\\-3x^2 - 12x = y + 15\)
Next, we'll factor out the coefficient of \(x^2 (-3)\) from the first two terms:
\(-3(x^2 + 4x) = y + 15\)
To complete the square, we need to take half of the coefficient of x (4), square it (16), and add it inside the parentheses. However, since we added it inside the parentheses, we need to subtract 16 * (-3) from the right side to maintain the equality:
\(-3(x^2 + 4x + 4) = y + 15 - 16 * (-3)\\-3(x + 2)^2 = y + 15 + 48\\-3(x + 2)^2 = y + 63\\\)
Now, we can rewrite the equation in the vertex form:
\(y = -3(x + 2)^2 - 63\)
Comparing this with the vertex form equation: \(y = a(x - h)^2 + k\), we can see that the vertex is at the point (-2, -63).
To know more about parabola,
https://brainly.com/question/33549692
#SPJ11
The vertex of the parabola is (-2, -19).
To find the vertex of the parabola given by the equation \(-3x^2 - 12x - 23 = y - 8\), we can complete the square. The general form of a quadratic equation is \(y = ax^2 + bx + c,\) where (h, k) represents the vertex of the parabola.
First, let's rewrite the equation in the standard form:
\(-3x^2 - 12x + 15 = y.\)
Now, we can complete the square. To do this, we need to take half of the coefficient of x (-12) and square it: \((-12/2)^2 = 36.\)
Add 36 to both sides of the equation: \(-3x^2 - 12x + 15 + 36 = y + 36.\)
Simplify: \(-3x^2 - 12x + 51 = y + 36.\)
Now, we can rewrite the equation in vertex form by factoring the left side: \(-3(x^2 + 4x - 17) = y + 36.\)
Next, we complete the square within the parentheses:
\((x + 2)^2 = -3(y + 19).\)
Now, the equation is in the form \((x - h)^2 = 4p(y - k)\), where (h, k) represents the vertex. In this case, the vertex is (-2, -19).
Overall, the process involves rewriting the equation in standard form, completing the square, and then rearranging the equation to match the vertex form. This allows us to identify the vertex of the parabola.
Learn more about vertex of the parabola
https://brainly.com/question/29267743
#SPJ11
The lines below are parallel. If the slope of the green line is -2, what is the slope of the red line?
Answers
HELP NEEDED NOT HARD 5 PTS
You are renting a moving truck and need to find one with
enough space to carry your furniture. All the trucks are 7 feet
tall and 6 feet wide, but the lengths vary.
You need a truck with a volume of 840 cubic feet. Use the
volume of a rectangular prism formula V lwh to find the
length of the truck you need?
Answers
Answer:
20 Feet
Step-by-step explanation:
If the trucks are all 7 feet tall, and 6 feet wide, then we can find out how long we need the truck to be by using this equation:
(l = length)
7 × 6 x l = 840
Or we can simply multiply 6 and 7 then divide 840 by that number.
6 × 7 = 42
840 ÷ 42 = 20
The truck would need to be 20 feet long to carry all of your furniture.
Construct both a 98% and a 80% confidence interval for B₁. B₁=46, s=5.7, SSzz = 57, n = 12 98%:
Answers
To construct a 98% confidence interval for B₁, we can use the t-distribution since the sample size is small (n = 12).
Given the sample mean (B₁ = 46), sample standard deviation (s = 5.7), and sum of squares (SSzz = 57), we can calculate the confidence interval.
The formula for a confidence interval is:
Confidence Interval = Sample Mean ± (Critical Value * Standard Error)
For a 98% confidence level and n = 12, the critical value is approximately 2.681 (obtained from a t-distribution table).
The standard error is calculated as the sample standard deviation divided by the square root of the sample size (s / √n).
Plugging in the values:
Standard Error = 5.7 / √12 ≈ 1.647
Confidence Interval = 46 ± (2.681 * 1.647)
Therefore, the 98% confidence interval for B₁ is approximately (42.21, 49.79).
In conclusion, we can be 98% confident that the true value of B₁ falls within the range of 42.21 to 49.79 based on the given sample data.
To know more about confidence interval visit :
https://brainly.com/question/15712887
#SPJ11
the voltage across a 1 uf capacitor is given. what is the sinusoidal expression for the current? a) 30sin200t b) 60×10^(-3) sin377t
Answers
To determine the sinusoidal expression for the current in a capacitor, we will use the following equation:
I(t) = C * (dV(t)/dt)
Where I(t) is the current at time t, C is the capacitance (1 μF in this case), and dV(t)/dt is the derivative of the voltage function V(t) with respect to time.
Let's examine both voltage expressions:
a) V(t) = 30sin(200t)
b) V(t) = 60×10^(-3)sin(377t)
Now, let's find the derivatives:
a) dV(t)/dt = 30 * 200 * cos(200t)
b) dV(t)/dt = 60 * 10^(-3) * 377 * cos(377t)
Next, we will multiply each derivative by the capacitance C (1 μF):
a) I(t) = 1×10^(-6) * 30 * 200 * cos(200t)
b) I(t) = 1×10^(-6) * 60 * 10^(-3) * 377 * cos(377t)
Finally, we can simplify the expressions:
a) I(t) = 6 * 10^(-3) cos(200t) A
b) I(t) = 22.62 * 10^(-6) cos(377t) A
Thus, the sinusoidal expressions for the current in each case are:
a) I(t) = 6 * 10^(-3) cos(200t) A
b) I(t) = 22.62 * 10^(-6) cos(377t) A
Know more about sinusoidal expression,
https://brainly.com/question/31428905
#SPJ11
hi amazing people please help i’ll give brainliest thanks!

Answers
Answer:
Step-by-step explanation:
Well, if it is C or D, let’s think about it.
Would you want to include a quotation to present another person’s viewpoint? Probably.
Would you want to include a quotation to analyze specific data? Yes, you would.
This all comes down to what essay you are writing, whether it is an analytical or argumentative essay. If it is an argumentative writing, then it’d probably be C) to present another’s viewpoint. If it is an analytical writing, then D) to analyze specific data would make sense.
In ΔSTU, u = 220 cm, ∠T=145° and ∠U=18°. Find the length of s, to the nearest 10th of a centimeter.
Answers
Answer:
208.1
Step-by-step explanation:
Dawn earned $97.50 for 10 hours of work. Amy earned 120 in 12 hours of work how much did each person earn per hour
Answers
Answer:
Amount earned in 1 hour \(=\$9.750\)
Amount earned in 1 hour \(=\$10\)
Step-by-step explanation:
Total amount earned by Dawn = $97.50
Dawn earned $97.50 for 10 hours of work
Amount earned in 1 hour \(=\frac{97.50}{10}=\$9.750\)
Total amount earned by Amy in 12 hours = $120
Amy earned $120 in 12 hours of work
Amount earned in 1 hour \(=\frac{120}{12}=\$10\)
ASAP!! I NEED HELPPPP!!!!111!!!!1!!!111111!!!!

Answers
The x and y intercepts of each of the following is (a) x intercepts are 3 and -5, y intercept is 16. (b) x intercepts are 1 and 2.5, y intercept is -2.5. (c) x intercept is 8 and the y intercept is -16.
What is x Intercept and y Intercept?x intercept is the x coordinate of the point on the line where it touches the X axis. The y coordinate will be 0 there.
y intercept is the y coordinate of the point on the line where it touches the Y axis. The x coordinate will be 0 there.
(a) We have the points from the table.
(3, 0) and (-5, 0)
y coordinate is 0. So x intercepts are 3 and -5.
We also have (0, 16).
y intercept is 16.
(b) We have the points (1, 0) and (2.5, 0).
x intercepts are 1 and 2.5.
We also have (0, -2.5).
y intercept is -2.5.
(c) We have (8, 0) and (0, -16).
x intercept is 8 and the y intercept is -16.
Hence the x and y intercepts are found.
Learn more about x and y Intercepts here :
https://brainly.com/question/14180189
#SPJ1
Use this graph to answer the questions.Max20X + 10Ys.t.12X + 15Y ≤ 18015X + 10Y ≤ 1503X − 8Y ≤ 0X, Y ≥ 0Which area (I, II, III, IV, or V) forms the feasible region?
Answers
The feasible region for the graph is Region III.
Here we have been given the Objective Function
Max 20X + 10Y
The constraints to the function are
12X + 15Y ≤ 180
15X + 10Y ≤ 150
3X − 8Y ≤ 0
Now here we will first draw the graph of the function. We will do this by joining the intercepts of the constraints and shading the feasible region
Now,
12X + 15Y ≤ 180
Here putting X = 0 we get Y = 12 and putting Y = 0, we get X = 15
Hence the intercepts are
(0, 12) and (15, 0)
15X + 10Y ≤ 150
Here we will get the intercepts to be (0, 15) and (10, 0)
3X − 8Y ≤ 0
For this, we will get the intercept as
(0 , 0) and we will get another point as (8 , 3)
Hence now we see that all have a less-than-equal sign, hence all the lines will have their shadings toward the origin.
Hence we get the feasible region as ABCD as shown in the image.
This matches with region III from the question. Hence we get region III as the feasible region
To learn more about Linear Programming visit
https://brainly.com/question/17622497
#SPJ4
Complete Question
(Image Attached)


Every matrix transformation is a linear transformation.a. Trueb. false
Answers
True , Every matrix transformation is a linear transformation.
Is there a linear transformation for every matrix operation?
Despite the fact that every linear transformation is a matrix transformation, not all linear transformations are matrix transformations.
Because of this, it's possible that we'll encounter a linear transformation in which we're unable to locate a matrix to carry out the mapping.
What alteration does matrix entail?
In a two-dimensional or three-dimensional space, the transformation matrix converts one vector into another vector, which may be comprehended geometrically.
Stretching, squeezing, rotation, reflection, and orthogonal projection are some of the often utilized transformations.
Learn more about transformation matrix
brainly.com/question/16956653
#SPJ4
6x + 3 > -33 on a number line
Answers
Answer:
Step-by-step explanation:

I need to know 502 divided by 70 for thi aignment. It i important and critical for me to get good grade
Answers
Answer:
7.171428571
Step-by-step explanation:
<3
Which of the following statements is true for a function with equation f(x) = 5(3)x?
Answers
The graph has y-intercept (0,5) and increases with a constant ratio of 3.
What is the function?A function in mathematics is a connection between a set of inputs (sometimes referred to as the domain) and a set of outputs (also referred to as the range). Each input value is given a different output value.
The y-intercept lies at (0, 5) because the value of the function at x=0 is 530 = 5. The 'constant ratio' is 3, meaning that any increment of 1 in x causes the function value to grow by a factor of 3. (That serves as the exponential term's foundation.)
Learn more about function:https://brainly.com/question/30721594
#SPJ1
Missing parts;
Which of the following statements is true for a function with equation f(x) = 5(3)*?
The graph has y-intercept (0,5) and increases with a constant ratio of 3.
The graph has y-intercept (0, 3) and decreases with a constant ratio of 3.
The graph has y-intercept (0, 3) and increases with a constant ratio of 5.
The graph has y-intercept (0,5) and decreases with a constant ratio of 3.

Will mark brainliest
A. What is the solution to the
system based on the graph?
B. How do you know it is the
solution?

Answers
Answer:
(0, 2)
Step-by-step explanation:
The solution to the system based on the graph is (0, 2)
Reason: Lines are intersecting at point (0, 2) which satisfy the given equations.
Pls help me with this :)

Answers
Answer:
looks like school work
Step-by-step explanation:
DO IT ON YOUR OWN
i need help with 5 pls!!! I’ll give brainliest:)

Answers
A machine fills bags with sweets.
There are 4275 sweets.
There are 28 sweets in each full bag.
The machine fills as many bags as possible.
How many sweets are left?
Answers
Answer:
19
Step-by-step explanation:
maximum no. of bag filled by 4275 sweets,is
4275/28 ~=152
therefore , there is 4256 sweets in 152 bags
therefore,4275-4256=19 sweets are left
Lanya's house is located at (2 2/3, 7 1/3) on a map. The store where she works is located at (-1 1/3, 7 1/3). what is the distance from Lanya's home to the store?

Answers
Answer:
Option A: 4 units is the correct answer.
Step-by-step explanation:
Given that:
Layana's house is located at (\(2\frac{2}{3}, 7\frac{1}{3}\))
Store is located at (\(-1\frac{1}{3},7\frac{1}3}\))
We can find distance between two points by using distance formula.
S = \(\sqrt{(x_2-x_1)^2+(y_2-y_1)^2\)
The points can also be written as;
House (8/3, 22/3)
Store (-4/3, 22/3)
Putting values in the formula.
S = \(\sqrt{\frac{8}{3}-(-\frac{4}{3}))^2+(\frac{22}{3}-\frac{22}{3})^2\)
S = \(\sqrt(\frac{8}{3}+\frac{4}{3}+0\)
S = \(\sqrt{(\frac{8+4}{3})^2\)
S = \(\sqrt{(\frac{12}{4})^2\)
S = \(\sqrt{(4)^2\)
S = 4 units
Hence,
Option A: 4 units is the correct answer.