Find the total surface area of the following
cone. Leave your answer in terms of a.
4 cm
3 cm
SA = [ ? ]7 cm
Hint: Surface Area of a Cone = tre + B
Where e = slant height, and B = area of the base
Answers
The total surface area of the cone is 44π cm², where π represents the mathematical constant pi.
We have,
To find the total surface area of a cone, we need to calculate the lateral surface area (denoted by L) and the base area (denoted by B), and then sum them.
The lateral surface area of a cone is given by L = πrℓ, where r is the radius of the base and ℓ is the slant height.
The base area is given by B = πr², where r is the radius of the base.
Given the dimensions:
Radius of the base (r) = 4 cm
Slant height (ℓ) = 7 cm
We can calculate the lateral surface area as L = π(4)(7) = 28π cm².
The base area can be calculated as B = π(4^2) = 16π cm².
Now, to find the total surface area (SA), we sum the lateral surface area and the base area:
SA = L + B = 28π + 16π = 44π cm².
Therefore,
The total surface area of the cone is 44π cm², where π represents the mathematical constant pi.
Learn more about cones here:
https://brainly.com/question/13798146
#SPJ12
Related Questions
how can I solve this linear equation x+y=7 3x+y=15
Answers
Answer:
(x, y) = (4, 3)
Step-by-step explanation:
The first step in solving any math problem is to read and understand what is given and what is asked.
GivenTwo linear equations are given, each in standard form. The coefficients of y are the same in the two equations, and both coefficients are 1 in the first equation.
x +y = 73x +y = 15FindYou are asked to "solve" this system of equations. That means you want an (x, y) ordered pair that will satisfy both equations. On a graph, these are the coordinates of the point where the lines cross.
SolutionThere are many ways to solve a system of linear equations. In this case, the y-coefficients being the same suggests that "elimination" or "linear combination" would be a good choice of solution method.
We note that the coefficients in the second equation are generally larger than those in the first equation. Subtracting the first equation from the second equation will generally leave positive coefficients, making the arithmetic less error-prone.
(3x +y) -(x +y) = (15) -(7) . . . . . . subtract the first equation from the second
2x = 8 . . . . . . simplify
x = 4 . . . . . . divide by 2
Using this value in the first equation, we can find y:
4 +y = 7
y = 3 . . . . . . subtract 4
The solution to this pair of equations is (x, y) = (4, 3).
__
Additional comment
A graphical solution is attached.
Either equation can be solved for y, and that expression substituted into the other. Solving the first equation for y gives ...
y = 7 -x
Substituting that into the second equation, we have ...
3x +(7 -x) = 15
2x = 8 . . . . . . . subtract 7
x = 4 . . . . . . divide by 2
y = 7-4 = 3
(x, y) = (4, 3) is the solution.
We have shown 3 methods of solving the given system of equations. There are other methods that can be used, too, including matrix methods and methods that make use of arrays of the coefficients.

Given the situation: Nick takes 7 steps up the stairs of the Tanjay City Science High School and then 5 steps to a landing area. Write an expression the represents the number of steps nick has taken.
Answers
Answer:
7 + 5 = 12
Step-by-step explanation:
7 steps plus 5 steps equals 12 steps
The sum of the angle measures of any triangle is 180 degrees. Angle #2 is twice the length of Angle #1 and Angle #3 is 15 degrees less than the Angle #2. What is the measure of each angle?
Answers
Answer: The measure of angle 1 is 39 degrees,the measure of angle 2 is 78 degrees and the measure of angle 3 is 63 degrees.
Step-by-step explanation:
We will represent the measure of angle 1 by x because it is unknown.
It says that the measure of angle 2 is twice angle 1 so we could represent that as 2x and the measure of angle 2 is 15 less than angle 3 and we could also represent that by 2x - 15.
Now we know they all add up to 180 so add them up and set them equal to 180 and solve for x.
x + 2x + 2x -15 = 180
5x - 15 = 180
+15 +15
5x = 195
x = 39
If x is equal to 39 then the measure of angle 1 is 39 degrees and the measure of angle 2 is twice that so 2(39) = 78 which also gives us the measure of angle 2 as 78.
For angle 3 it says that it has to be 15 less than the measure of angle so subtract 15 from 78 to find the measure of angle 3.
78 - 15 = 63 The measure angle 3 is 63 degrees. Now add the measures all together to see if they equal 180 degrees .
Check:
39 + 78 + 63 = 180
180 = 180
Determine the nature of the roots: 2x^(2) +8x +3=0 a. two distinct real solutions c. cannot be determined b. no real solutions d. a unique real solution
Answers
Answer:
a
Step-by-step explanation:
Given a quadratic equation in standard form ax² + bx + c = 0 (a ≠ 0 )
Then the discriminant b² - 4ac determines the nature of the roots.
• If b² - 4ac > 0 then 2 real and distinct roots
• If b² - 4ac = 0 then 2 real and equal roots
• If b² - 4ac < 0 then no real roots
Given
2x² + 8x + 3 = 0 ← in standard form
with a = 2, b = 8, c = 3 , then
b² - 4ac = 8² - (4 × 2 × 3) = 64 - 24 = 40
Since b² - 4ac > 0 then 2 real and distinct roots → a
Answer:
a
Step-by-step explanation:
Which expression has a simplified expression of 5x - 12?
O 3(4x - 4) - 7x
0-314x - 4) - 7x
O 31-4x + 4) - 7x
O-31-4x - 4) - 7x
Answers
Answer:
3(4x-4)-7x
Step-by-step explanation:
PLEASE HELP ME WITH THIS QUESTION AND PROVIDE AN EXPLANATION PLEASE
FIND A UNIT RATE!!!!

Answers
Answer:
No
Step-by-step explanation:
No, it is not because the 3 dollars is a steady price. A expression will look like 7n+3. Since the 3 dollars is a steady price you can never have a unit rate.
Answer:
It's proportional
Step-by-step explanation:
10-3=7 7/1 = 7
17 - 3= 14 1 4/2 = 7
31 - 3 = 28 28/4 = 7
38 -3 = 35 35/5 = 7
73 - 3 = 70 70/10 = 7
Can someone help me out?

Answers
Answer:
d
Step-by-step explanation:
Find the direction angles of the vector. Write the vector in terms of its magnitude and direction cosines, v=v(cosa)i + (cos )j + (cos yk]. v=3i-2j+2k α= (Round to the nearest tenth as needed.) B=(Ro
Answers
The direction angles of vector v are approximately α ≈ 38.7°, β ≈ 142.1°, and γ ≈ 57.3°.
To find the direction angles of the vector v = 3i - 2j + 2k, we can use the direction cosines. The direction cosines are given by the ratios of the vector's components to its magnitude.
The magnitude of vector v is:
|v| = √(3² + (-2)² + 2²) = √17
The direction cosines are:
cosα = vₓ / |v| = 3 / √17
cosβ = vᵧ / |v| = -2 / √17
cosγ = vᵢ / |v| = 2 / √17
To find the direction angles α, β, and γ, we can take the inverse cosine of the direction cosines:
α = cos⁻¹(3 / √17)
β = cos⁻¹(-2 / √17)
γ = cos⁻¹(2 / √17)
Calculating the direction angles using a calculator, we get:
α ≈ 38.7° (rounded to the nearest tenth)
β ≈ 142.1° (rounded to the nearest tenth)
γ ≈ 57.3° (rounded to the nearest tenth)
Therefore, the direction angles of vector v are approximately α ≈ 38.7°, β ≈ 142.1°, and γ ≈ 57.3°.
To know more about vector, visit:
https://brainly.com/question/31707305
#SPJ11
let v1= 0 0 −4 , v2= 0 −4 12 , and v3= 6 −5 −8 . does v1,v2,v3 span ℝ3? why or why not?
Answers
Yes, the vectors v1 = (0,0,-4), v2 = (0,-4,12), and v3 = (6,-5,-8) do span ℝ3. This is because any 3 vectors in ℝ3 will span the entire space, meaning that any vector in ℝ3 can be written as a linear combination of these 3 vectors.
To determine if a set of vectors spans a space, we can create a matrix with the vectors as columns and row reduce it to see if there are any free variables. If there are no free variables, the vectors span the space.
The matrix with v1, v2, and v3 as columns is:
| 0 0 6 |
| 0 -4 -5 |
| -4 12 -8 |
Row reducing this matrix gives us:
| 1 0 0 |
| 0 1 0 |
| 0 0 1 |
Since there are no free variables, the vectors v1, v2, and v3 do span ℝ3.
know more about vectors here
https://brainly.com/question/29740341#
#SPJ11
HELP! Will give brainliest
What is the center and radius of the following circle:
(x - 3)2 + (y + 2)2 = 25
Answers
Where the center is (h,k) and the radius is r
Center would be (3,-2) and the radius is r^2=25
after squaring each side r=5
Answer: Center is (3,-2) and the radius is r=5
Please heelp.. I suck at math

Answers
Answer:
The size of the garden is 75 by 1! The perimeter is 75 + 1 + 75 + 1, or 152.
Step-by-step explanation:
Alright, so what I'm seeing is that the measure of the sides will never change. It's always 2. That makes your life easier because you just take the remaining 2 sides!
So, to figure that out, first, you've gotta subtract 2 from 152. That's 150. Then, for the remaining 2 sides, you have to divide 150 by 2, which is 75.
Therefore, the size of the garden is 75 by 1! The perimeter is 75 + 1 + 75 + 1, or 152.
*mike dropeth*
Hakeem has a coupon for 13 off the regular price, t, of a tent. Which expressions represent the price of the tent using the coupon? Select all the correct expressions.
1/3t
2/3t
t−2/3t
t−1/3
t−2/3
t−1/3t
Answers
Answer:
123456789101112131415
set up an integral that represents the length of the part of the parametric curve shown in the graph. x = t − 4 sin(t), y = 1 − 4 cos(t), 0 ≤ t ≤ 4
Answers
The integral representing the length of the parametric curve is ∫[0, 4] √(17 - 8 cos(t)) dt.
How to find the curve length?To find the length of the parametric curve represented by the equations x = t − 4 sin(t) and y = 1 − 4 cos(t) over the interval 0 ≤ t ≤ 4, we can use the arc length formula for parametric curves. The arc length formula is given by:
L = ∫[a, b] √(dx/dt)^2 + (dy/dt)^2 dt
where [a, b] represents the interval of the parameter, dx/dt and dy/dt are the derivatives of x and y with respect to t, and √ denotes the square root.
Let's calculate the integral for the given parametric curve:
dx/dt = 1 - 4 cos(t)
dy/dt = 4 sin(t)
Now we can set up the integral for the arc length:
L = ∫[0, 4] √((1 - 4 cos(t))^2 + (4 sin(t))^2) dt
Simplifying the integrand:
L = ∫[0, 4] √(1 - 8 cos(t) + 16 cos^2(t) + 16 sin^2(t)) dt
= ∫[0, 4] √(1 - 8 cos(t) + 16) dt
= ∫[0, 4] √(17 - 8 cos(t)) dt
Therefore, the integral that represents the length of the given parametric curve is:
L = ∫[0, 4] √(17 - 8 cos(t)) dt
Learn more about parametric curve
brainly.com/question/15585522
#SPJ11
If the probability of observing at least one car on a highway during any 20-minute time interval is 609/625, then what is the probability of observing at least one car during any 5-minute time interval
Answers
If the probability of observing at least one car on a highway during any 20-minute time interval is 609/625, then the probability of observing at least one car during any 5-minute time interval is 609/2500
Given The probability of observing at least one car on a highway during any 20 minute time interval is 609/625.
We have to find the probability of observing at least one car during any 5 minute time interval.
Probability is the likeliness of happening an event among all the events possible. It is calculated as number/ total number. Its value lies between 0 and 1.
Probability during 20 minutes interval=609/625
Probability during 1 minute interval=609/625*20
=609/12500
Probability during 5 minute interval=(609/12500)*5
=609/2500
Hence the probability of observing at least one car during any 5 minute time interval is 609/2500.
Learn more about probability at https://brainly.com/question/24756209
#SPJ4
Which number has a 2 with a value ten times less than the 2 in the number 725,983?
Answers
Answer:
"Which number has a 2 with a value ten times less than the 2 in the number 725,983?"
First, the value of the 2 in the number 725,983 can be find by separatig each digit as:
725,983 = 700,000 + 20,000 + 5,000 + 900 + 80 + 3.
Then the value of the 2 is: 20,000.
Now we want a number that has a 2 with a value then times less than 20,000.
this is:
20,000/10 = 2,000.
Then we want a number that has a 2 in the thousands place.
For example, 132,000 is a number that can be an answer, the only thing you need is that the 2 is in the thousands place (the fourth digit counting from the right)
Please help!!
Will give brainliest!

Answers
Answer:
-10.08
Step-by-step explanation:
Using order of operations:
\( - 17 + (\frac{ - 2.3 + 8.12}{6} ) \times {4}^{2} - 8.6\)
\( - 17 + \frac{5.82}{6} \times 16 - 8.6\)
\( - 17 + .97 \times 16 - 8.6\)
\( - 17 + 15.52 - 8.6\)
\( - 10.08\)
write an expression to represent the measure of an angle supplementary to the given angle
Answers
Answer:
80+100=supplementary, right? A sup. angle is 2 angles making 180 digres.
** PLS HELP **
BRAINLIEST!

Answers
Answer:
a and d
or
c and b
Step-by-step explanation:
supplementary angles are angles that add up to 180 degrees
when looking at the rectangle you see two possible answers
Given the graph below, determine the values for a and b in the equation y=blog3(x+a). If a value is a non-integer then type it as a reduced fraction.

Answers
The values of b and a for the logarithmic function in this problem are given as follows:
a = -4.b = -2.1.How to define the logarithmic function?The logarithmic function in the context of this problem has the format given as follows:
\(y = b\log_3{x + a}\)
The vertical asymptote is at x = -4, hence:
\(y = b\log_3{x - 4}\)
When x = 5, y = -1, hence the parameter b is obtained as follows:
\(-1 = b\log_3{5 - 4}\)
0.477b = -1
b = -1/0.477
b = -2.1.
More can be learned about logarithmic functions at https://brainly.com/question/24160402
#SPJ1
The binomial expansion of (x^2+y)^2 is
A. x^4+2x^2y+y^2
B. x^2+2x^2y+y^2
C. x^4+2x^3y+x^2y^2
D. x^2+2xy+y^2
Answers
Answer: It’s A
Step-by-step explanation:
x4 + 2x2y + y2
230% of some number is 48.2. what is the number?
Answers
The number line shows the distance between numbered points on the line
Which number is the same distance from 0 on the number line as 4
Answers
Which expression is equivalent to
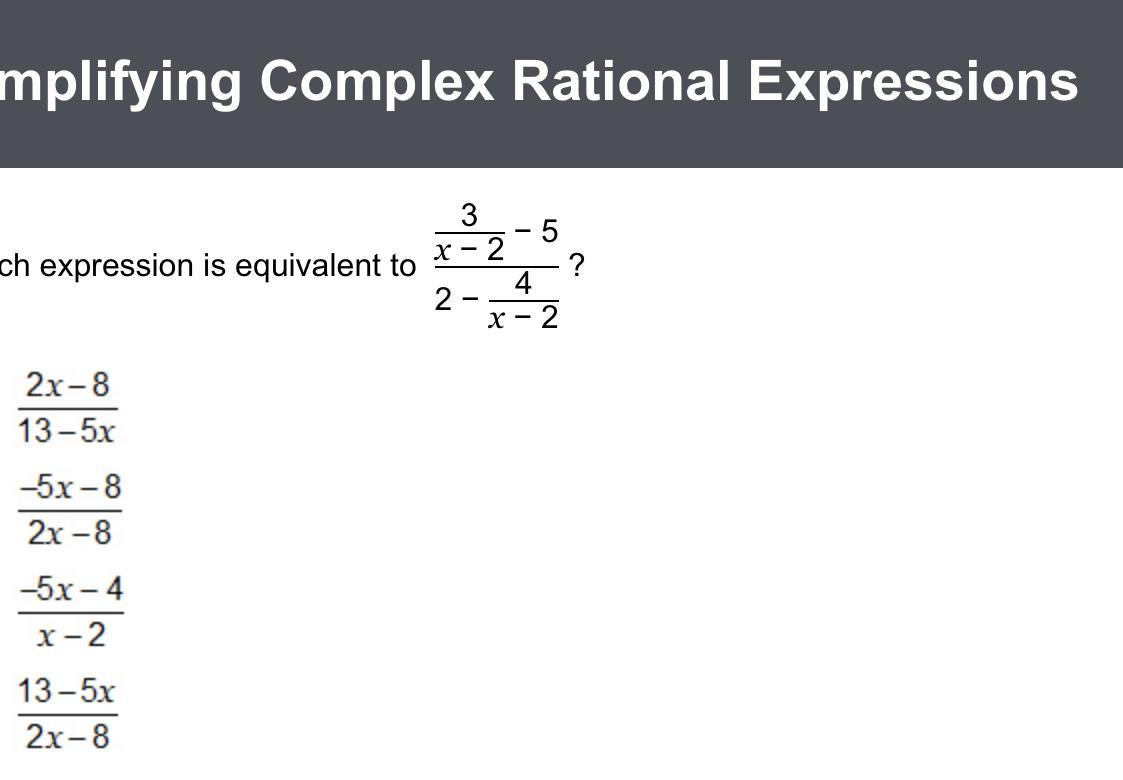
Answers
Answer:
d) \(\frac{13 - 5x}{2x -8}\)
Step-by-step explanation:
Explanation:-
Given expression
\(\frac{\frac{3}{x-2}-5 }{2-\frac{4}{x-2} }\)
we will do L.C.M both numerator term and denominator term
⇒ \(\frac{\frac{3-5(x-2)}{x-2} }{\frac{2(x-2)-4}{x-2} }\)
on simplification , we get
⇒ \(\frac{\frac{13-5x}{x-2} }{\frac{2x-8}{x-2} }\)
cancellation 'x-2'
we will get
\(\frac{13 - 5x}{2x -8}\)
Davon has only red tulips and white tulips in his garden. Three-fourths of his tulips are white. What is the
ratio of red tulips to white tulips in Devon's garden?
1 to
Answers
Answer:
3:1
Step-by-step explanation:
Out of 4/4 tulips...
Davon has 3/4 white tulips.
Davon therefore has 1/4 red tulips.
3/4:1/4 is basically 3:1 (After dividing 1/4 by 3/4)
PLS HELPPPP!!!!! WILL GIVE BRAINLIST!!!!!!!!!!

Answers
Answer: C) y=-2+2
Step-by-step explanation: Rise over run says that the slope is -2 and the line hits the y axis at +2 so the equation would be y = -2 + 2.
Sallaries of 41 college graduates who took a statistics course in college have a mean of $88,513 and a standard deviation of $1,508. Construct a 84.7% confidence interval for estimating the population variance. Enter the lower bound of the confidence interval. (Round your answer to nearest whole number.)
Answers
The lower bound of the confidence interval is $672,743
Given data are: 41 college graduates who took a statistics course in college have a mean of $88,513 and a standard deviation of $1,508.
We are required to construct an 84.7% confidence interval for estimating the population variance.
To find the lower bound of the confidence interval, we use the following formula: Lower bound of confidence interval: χ2 = ((n - 1)s²) / χ2(α/2, n-1)
Where n = sample size, s = sample standard deviation, χ2 = chi-square critical value, and α = level of significance.
Here, n = 41, s = $1,508, α = 1 - 0.847 = 0.153 (using the complement of the given confidence level), and degree of freedom (df) = n - 1 = 41 - 1 = 40.
To find the chi-square critical value, we use the chi-square distribution table:
χ2(α/2, n-1) = χ2(0.0765, 40) = 26.509.
So, Lower bound of confidence interval: χ2 = ((n - 1)s²) / χ2(α/2, n-1) = ((41 - 1) x $1,508²) / 26.509≈ $672,743.
Hence, the lower bound of the confidence interval is $672,743 (rounded to the nearest whole number).
Know more about the chi-square distribution
https://brainly.com/question/4543358
#SPJ11
Find ∠L using law of sines

Answers
Answer:
29°
Step-by-step explanation:
:)
solve the inequality; x2-x-2>0
Answers
Answer:
x > 2/3
Step-by-step explanation:
2x -x - 2 > 0
add 2x and x (It becomes 3x)
3x -2 >0
subtract 0 from both sides
3x> 2
divide 3 on both sides
And the answer is
X> 2/3
Ecuación de la hipérbola con centro en (0;0), focos en abrir paréntesis 0 coma espacio menos raíz cuadrada de 28 cerrar paréntesis espacio y espacio abrir paréntesis 0 coma espacio raíz cuadrada de 28 cerrar paréntesis espacio ,eje conjugado = 2 raíz cuadrada de 3
Answers
Answer:
\(\frac{y^{2}}{25}-\frac{x^{2}}{3}=1\)
Step-by-step explanation:
Para resolver este problema debemos tomar en cuenta los datos que nos dan y la ecuación de una hipérbola. Comencemos con los datos:
centro: (0,0)
focos: \((0,-\sqrt{28}),(0,\sqrt{28})\)
eje conjugado = \(2\sqrt{3}\)
por los focos podemos ver que la hipérbola se dirige hacia el eje y, por lo que debemos tomar la siguiente forma de la ecuación de la parábola:
\(\frac{y^{2}}{a^{2}}+\frac{x^{2}}{b^{2}}=1\)
de los focos podemos obtener que:
\(c=\sqrt{28}\)
y del eje conjugado podemos saber que al dividir la longitud del eje conjugado dentro de 2 obtenemos b, así que:
\(b=\sqrt{3}\)
podemos utilizar la siguiente fórmula para obtener a:
\(c^{2}-a^{2}=b^{2}\)
si despejamos a en la ecuación obtenemos lo siguiente:
\(a=\sqrt{c^{2}-b^{2}}\)
ahora podemos sustituir los valores:
\(a=\sqrt{(\sqrt{28})^{2}-(\sqrt{3})^{2}}\)
\(a=\sqrt{28-3}\)
\(a=\sqrt{25}\)
a=5
así que media vez conozcamos a, podemos sustituir los datos en la ecuación de la hipérbola así que obtenemos lo siguiente:
\(\frac{y^{2}}{a^{2}}+\frac{x^{2}}{b^{2}}=1\)
\(\frac{y^{2}}{(5)^{2}}+\frac{x^{2}}{(\sqrt{3})^{2}}=1\)
\(\frac{y^{2}}{25}+\frac{x^{2}}{3}=1\)
si graficamos la hipérbola, queda como en el documento adjunto.

An angle measures 120° less than the measure of its supplementary angle. What is the measure of each angle?
This is IXL
Answers
By definition, the supplementary angle of x measures 180 - x degrees.
We're told that the angle measures 120 degrees less than its supplementary angle, so we can set up an equation:
x = (180 - x) - 120
Simplifying this equation, we get:
x = 60
Therefore, the angle measures 60 degrees, and its supplementary angle measures 180 - 60 = 120 degrees.