How much space will the tissue box take up on
my desk if the base dimensions are 3.5 inches
and 8 inches?
square inches
Hint: Find the area of the base of the tissue box.
Answers
Answer:
3.5 x 8 = 28 in²
Related Questions
If two negative integers are multiplied together, the product will be
F
negative
G
positive
H
both positive and negative
J
neither positive or negative
Answers
Answer: A
Step-by-step explanation:
Answer:
negative
Step-by-step explanation:
-x*-y=z
What are the 3 types of proofs in math
Answers
Answer:
direct proof, proof by contradiction, proof by induction
Step-by-step explanation:
there u go mate
Karen makes 5 dollars per hour babysitting and 12 dollars per hour giving music lessons. One weekend, she worked a total of 18 hours and made 139 dollars. How many hours did she babysit that weekend
Answers
Answer:
15.06
Step-by-step explanation:
Substituting this value into the first equation gives us b + 2.94 = 18, so b = 15.06 hours. Since Karen can only work whole hours, she must have worked 15 hours babysitting and 3 hours giving music lessons.
Babysit: 11 hours x £5 = £55
£84 + £55 = £139
So she babysit for 11 hours on weekend.
Prove that the set of functions from N to N is uncountable, by using a diagonalization argument. N is the set of natural numbers.
Answers
Hence Proved N to N is uncountable set by using a diagonalization argument
What is Diagonalization Argument?
Georg Cantor published the Cantor's diagonal argument in 1891 as a mathematical demonstration that there are infinite sets that cannot be put into one-to-one correspondence with the infinite set of natural numbers. It is also known as the diagonalization argument, the diagonal slash argument, the anti-diagonal argument, the diagonal method, and Cantor's diagonalization proof.
Proof:
We write f as the sequence of value it generates
that is, say f:N-N is defined as f(x) =x then
we write f as : 1,2,3,4........
similarly if f:N-N were f(x) = 2x then we write it as :2,4,6,8.......
now assume on the contrary that the set of functions from N to N is countable
then,
\(f_{1}\) : \(f_{11}\) \(f_{12}\) \(f_{13}\) , ...........
\(f_{2}\) : \(f_{21}\) \(f_{22}\) \(f_{23}\) , ...........
\(f_{3}\) : \(f_{31}\) \(f_{32}\) \(f_{33}\) , ........... and so on
Here \(f_{ij}\) =\(f_{i}(j)\)
consider the function f as :
f : (\({f11}\) +1 ) , (\({f22}\) +1) , (\({f33}\) +1),.........
Then f wouldn't appear in the list of the function we had above since any \(f_{i}\) would disagree with f at the i th place
Therefore the set of the function from N to N is an uncountable set
To learn more about diagonalization argument visit:
brainly.com/question/16991671
#SPJ4
if you multiply the number by 2 and add 4, then result you get will be the same as three times the number decreased by 7.
Answers
Answer:
Step-by-step explanation:
Let the number be x,
2x+4 = 3x-7
Collect like terms
2x-3x= -4-7
-x =. -11
Multiply both sides by -1
x. =. 11
PLEASE MARK BRAINLIEST.
A 95% confidence interval was computed around a sample mean of 10. The resulting confidence interval is (5, 11). Does this appear to be a valid confidence interval
Answers
Answer: No
Step-by-step explanation:
A confidence interval gives the range of values in which the true population parameter lies.It is computed by considering sample mean in the center.
But here 10 does not lie in the center of (5, 11), as distance between 10 and 5 = 5 units but distance between 10 and 11 = 1 unit
2 different margin of errors cannot be possible.
Hence, the given confidence interval is not valid.
evaluate 3x^4+2x^2-5x+1 when x=3202
Answers
Answer:21
Step-by-step explanation:
Answer:
315359990076847
Step-by-step explanation:
3x^4+2x^2-5x+1 when x=3202
replace the x's with 3202
3(3202)^4+2(3202)^2-5(3202)+1
solve the exponent first
3(105119989862416)+2(10252804)-5(3202)+1
then multiply
315359969587248+20505608-16010+1
add/subtract
315359990076847
How do I find the missing side

Answers
Answer:
x = 11.8639
Step-by-step explanation:
cos∅ = adjacent / hypotenuse
Since we are dealing with right triangles, we can use trig to help solve for missing values:
cos22° = 11/x
xcos22° = 11
x = 11/cos22°
x = 11.8639
3 sides of the triangle are distinct perfect squares. What is the smallest possible perimeter of the triangle?
Answers
Answer:
77
Step-by-step explanation:
At first, you would probably think that the side lengths are 1², 2², 3² = 1, 4 and 9 but these side lengths don't form a triangle. The Triangle Inequality states that the sum of the two shortest side lengths must be greater than the largest side length, and since 1 + 4 > 9 is a false statement, it's not a triangle. Let's try 2², 3², 4² = 4, 9, 16. 4 + 9 > 16 is also false so that doesn't work. 3², 4², 5² = 9, 16, 25 but since 9 + 16 > 25 is false (25 isn't greater than 25), that doesn't work either. 4², 5², 6² = 16, 25, 36 and since 16 + 25 > 36 is true, this is our triangle which means that the perimeter is 16 + 25 + 36 = 77.
Answer:
e
Step-by-step explanation:
e
Two numbers have prime factors of 2, 3, 5, and 11 that they share. What is the greatest common factor of the two numbers?
Answers
Answer:
5
Step-by-step explanation:
HELP ME
Final exam guide due
Show work

Answers
The approximate height of the tree is given as follows:
45.6 ft.
What is the geometric mean theorem?The geometric mean theorem states that the length of the altitude drawn from the right angle of a triangle to its hypotenuse is equal to the geometric mean of the lengths of the segments formed on the hypotenuse.
The altitude segment for this problem is given as follows:
14.5 ft.
The bases are given as follows:
5.2 ft and x ft.
Hence the value of x is given as follows:
5.2x = 14.5²
x = 14.5²/5.2
x = 40.4 ft.
Hence the height of the three is given as follows:
5.2 + 40.4 = 45.6 ft.
More can be learned about the geometric mean theorem at brainly.com/question/10216660
#SPJ1
The graph of F(x), shown below, resembles the graph of G(x) = x², but it has been stretched and shifted. Which of the following could be the equation of F(x)?
A. F(x) = 2/5 x² +6
B. F(x) = (1/3x)^2 +2
C. F(x) = 3x² -2
D. F(x) = (9x)² +2

Answers
Answer:
D. f(x) = (9x)² +2
Step-by-step explanation:
The function transformations we usually study include horizontal and vertical expansion (or compression), and horizontal and vertical translation. These can be summarized in the equation ...
g(x) = v·f((x -a)/h) +b
where v and h are vertical and horizontal expansion factors, respectively, and (a, b) is the (right, up) translation.
__
analysisThe graph of F(x) has had its vertex moved 2 units upward (b=2), It is distinctly narrower than G(x), which could be the result of vertical expansion (v>1), or horizontal compression (h<1). Since the graph of F(x) has been compressed horizontally, the value of h must be less than 1.
__
answer selectionsThere are two answer choices with b=2.
Choice B has h=3, a widening of the graph. Choice D has h=1/9, a narrowing of the graph.
The equation of the graph could be F(x) = (9x)² +2. The attachment shows this is an appropriate choice.
_____
Additional comment
You will notice that in this function, the vertical expansion factor is the reciprocal of the square of the horizontal expansion factor. In this case, h=1/9 is equivalent to v=81, a vertical expansion by a factor of 81.
F(x) = (x/(1/9))² +2 = 81x² +2
The given graphs are not detailed enough to be able to judge either of these factors directly. We can estimate that h=1/9 is approximately correct by looking at the values of x where the graph has its upper boundary. At those points, x≈3 on the G(x) graph, and x<1/2 on the F(x) graph, consistent with a value of about x=1/3. The "expansion" factor is then (1/3)/3 = 1/9.

What is the z-score of a data value that is 3 standard deviations to the left of the mean?
Answers
Answer:
Step-by-step explanation:
look up z-table
3 std div. = 0.00135
The sum of two numbers is 65 . The larger number is 7 more than the smaller number. What are the numbers?
Answers
Answer:
Let a be the larger number
Let b be the smaller number
A= b+7
Step-by-step explanation:
Hope this helps :) Have a great day!!!
6
Solve the inequality. Graph the solution.
11.3 > b /4.3
The solution is
Answers
Step-by-step explanation:
Multiply to remove the fraction, then set equal to
0 and solve.
Inequality Form:
b<48.59
Interval Notation:
(−∞, 48.59)

The solution of the inequality 11.3 > b /4.3 is b< 48.59 along with the number line.
Given Inequality:
11.3 > b /4.3
To solve the inequality 11.3 > b / 4.3,
Multiply both sides of the inequality by 4.3 to eliminate the fraction:
11.3 * 4.3 > (b / 4.3) * 4.3
48.59 > b
The solution to the inequality is b < 48.59.
This means that any value of b that is less than 48.59 will satisfy the inequality.
To graph the solution, indicate that b is less than 48.59 by using an open circle at 48.59 and shading the line to the left of the open circle to represent all the values that satisfy the inequality.
Learn more about Inequality here:
https://brainly.com/question/20383699
#SPJ6

7. N.CN.7 Determine the zeroes for the equation below. Select all that apply.
x² - 6x +13=0
A. 1
B. 5
C. 13
D. -3 + 2i
E. 3+2i
F. 3+4i
G. 6 + 4i
H. 3-21
I .6-41
Answers
Answer:
D. -3 + 2i and E. 3+2i are the zeroes for the equation.
Step-by-step explanation:
plsssssssssssssssssssss help me i need to get these right so i can play roblo.x pls tell me how you got the answer

Answers
Answer:
675 mm²
Step-by-step explanation:
base = 15 x 15 = 225 mm²
4 sides = 4(15)(15)(0.5) = 450 mm²
450 + 225 = 675 mm²
A florist currently makes a profit of $20 on each of her celebration bouquets and sells an average of 30 bouquets every week. She noticed that when she reduces the price such that she earns $1 less in profit from each bouquet, she then sells three more bouquets per week. The relationship between her weekly profit, P(x), after x one-dollar decreases is shown in the graph below.
A graph for p of x is a downward open parabola with its vertex at (5, 725) and passes through the points (negative 10, 0), and (20, 0).
Use the graph to complete each statement about this situation.
The maximum profit the florist will earn from selling celebration bouquets is $.
The florist will break-even after one-dollar decreases.
The interval of the number of one-dollar decreases for which the florist makes a profit from celebration bouquets is ( , ).
Answers
Answer:
The maximum profit the florist will earn from selling celebration bouquets is $725.
The florist will break-even after one-dollar decreases when her profit is zero. From the graph, this occurs at x = 10. So the florist will break-even after 10 one-dollar decreases.
The interval of the number of one-dollar decreases for which the florist makes a profit from celebration bouquets is (0, 10). This is because the profit is positive for values of x between 0 and 10, and becomes negative after 10.
Step-by-step explanation:
The maker of a cell phone screen protector would like to estimate the proportion of customers who file a warranty claim. To do so, they select a random sample of 200 customers and determine that the 96% confidence interval for the true proportion of customers who file a warranty claim to be 0.15 to 0.28. Which of the following would decrease the margin of error?
selecting another sample
decreasing the sample size
increasing the confidence level
decreasing the confidence level
Answers
In order to decrease the margin of error, the best option in this instance is by decreasing the confidence level would make the interval to be narrower.
The correct option is D.
What is the margin of error?The margin of error, also known as the confidence interval, provides information on how closely your survey results will likely reflect the opinions of the general community.
The margin of error is a measure of the potential uncertainty in your survey results. It is more likely that the results will deviate from the "true figures" for the entire population the wider the margin of error.
Learn more about margin of error at: https://brainly.com/question/10218601
#SPJ1
The area of the smaller regular pentagon is about 27.5 cm².
What is the best approximation for the area of the larger regular pentagon?
11cm²
172 cm²
70 cm²
275 cm²

Answers
Answer:172 cm²
Step-by-step explanation:
when we use the formula, and we can solve out is like 172.05 and round to tenth and will have the answer
Formula is 1/2 × p × a
Describe the transformation fromthe graph of f to the graph of h. Write an equation that represents h in terms of x. So confused! #10

Answers
ANSWER :
The graph of h(x) is the transformation of f(x) when shifted 4 units upward.
The equation of h(x) in terms of x is :
\(h(x)=-(x+1)^{2}+2\)EXPLANATION :
From the problem, we have :
\(\begin{gathered} f(x)=-(x+1)^2-2 \\ h(x)=f(x)+4 \end{gathered}\)We can say that h(x) is the result when f(x) is translated 4 units upward.
That will be :
\(\begin{gathered} h(x)=[-(x^+1)^2-2]+4 \\ h(x)=-(x+1)^2+2 \end{gathered}\)What is the answer to these questions?

Answers
1) There are 3.4 * 10^1 in Mexico than in Canada.
2) The number of ounces that were consumed is 20.8 * 10^7 ounces
3) The number of ounces of the students at the community college is 1.25 * 10^1 that of the students in the University.
What is scientific notation?Scientific notation is a way of writing very large or very small numbers using powers of 10. In scientific notation, a number is written as a coefficient multiplied by 10 raised to a power.
1) Ratio of people in Mexico to those in Canada;
1.3 * 10^8/3.8 * 10^7
= 3.4 * 10^1 : 1
2) Number of Ounces consumed by students = 5.2 * 10^4 * 4 * 10^3
= 20.8 * 10^7 ounces
3) Ratio of the number drank by community college to University
= 4 * 10^3/3.2 * 10^2
1.25 * 10^1
Learn more about scientific notation:https://brainly.com/question/18073768
#SPJ1
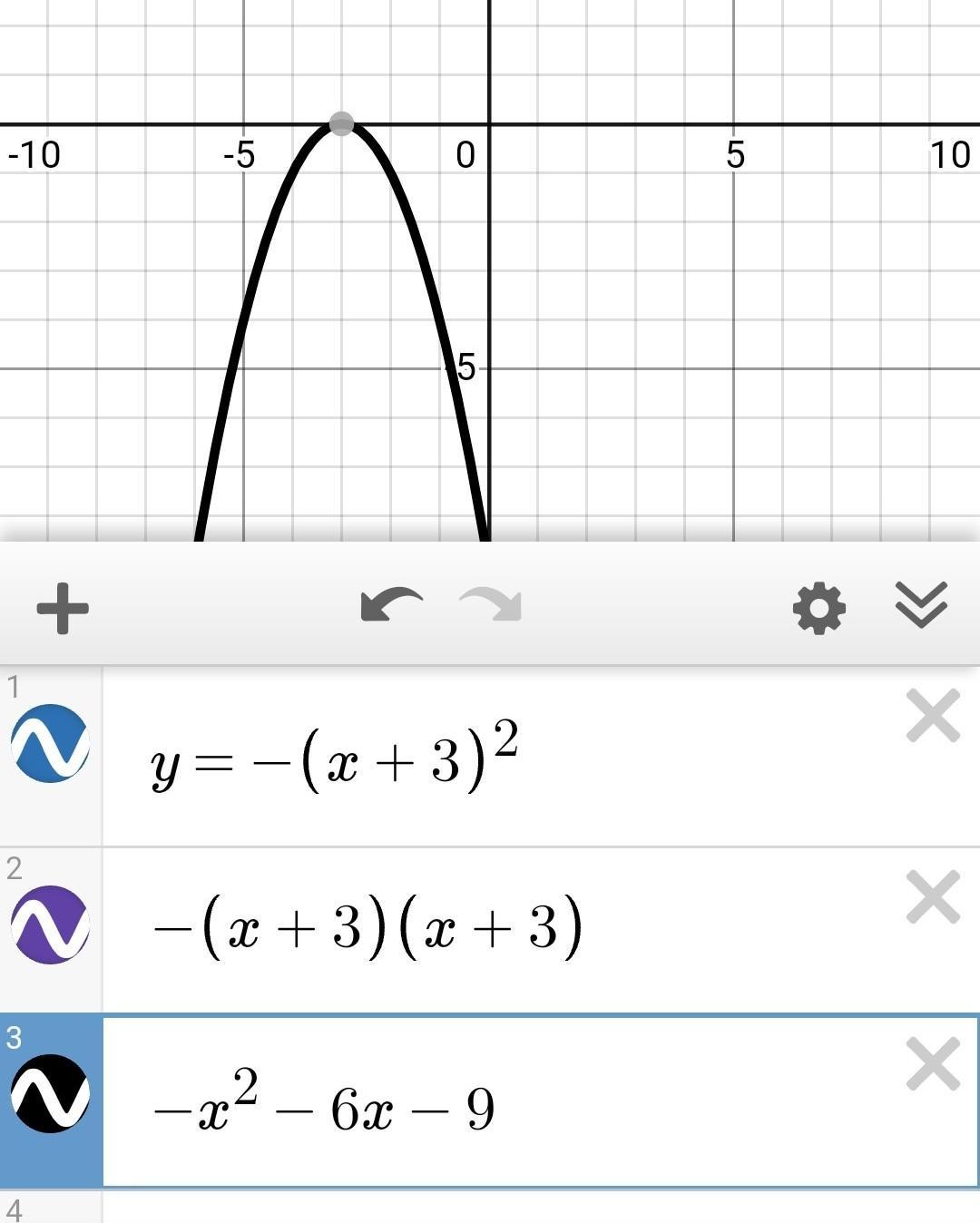
a. Find the vertex form equations (y = a(x - h)2 + k) for the parabolas below.
Assume parameter a = 1.
I
b. Then rewrite the equations in standard form (y = ax + bx + c).
Hint: multiply out your answer from part a.

Answers
Answer:
y=-(x+3)^2
y=-x^2-6x-9
Step-by-step explanation:
i uh hope this is correct
hope thia helps <3
1,3,6,10,15,21,28 as a function
Answers
It seems that the sequence you provided is the sequence of triangular numbers. The function that generates this sequence could be defined as:
f(n) = n*(n+1)/2
Where n is the position of the number in the sequence.
So, for example:
f(1) = 1*(1+1)/2 = 1
f(2) = 2*(2+1)/2 = 3
f(3) = 3*(3+1)/2 = 6
and so on.
Write a quadratic function to model the vertical motion for each situation, given h(t) equals negative 16t squared plus v0t+h0. Find the maximum height. Initial velocity 152
Answers
The maximum height reached by the ball is 908.5 feet.
Using the given values, we can substitute v0 = 152 and h0 = 6 into the equation:
\(h(t) = -16t^2 + 152t + 6\)
This quadratic function models the height of the ball at any time t after it is thrown.
To find the maximum height of the ball, we need to find the vertex of the parabolic curve described by the quadratic function. The vertex can be found using the formula:
t = -b / 2a
where a = -16 and b = 152 are the coefficients of the quadratic function.
t = -152 / 2(-16) = 4.75
So the maximum height is reached after 4.75 seconds. To find the maximum height, we can substitute this value back into the equation:
\(h(4.75) = -16(4.75)^2 + 152(4.75) + 6 = 908.5\)feet
Therefore, the maximum height reached by the ball is 908.5 feet.
for such more question on height
https://brainly.com/question/27987869
#SPJ11
Question
A ball is thrown vertically upward from a height of 6 feet with an initial velocity of 152 feet per second. Write a quadratic function to model the vertical motion of the ball, given that the height of the ball at time t is given by: h(t) = -16t^2 + v0t + h0
one more question whats 6/8 times 40
Answers
Answer:
30
Step-by-step explanation:
hope it helps <3
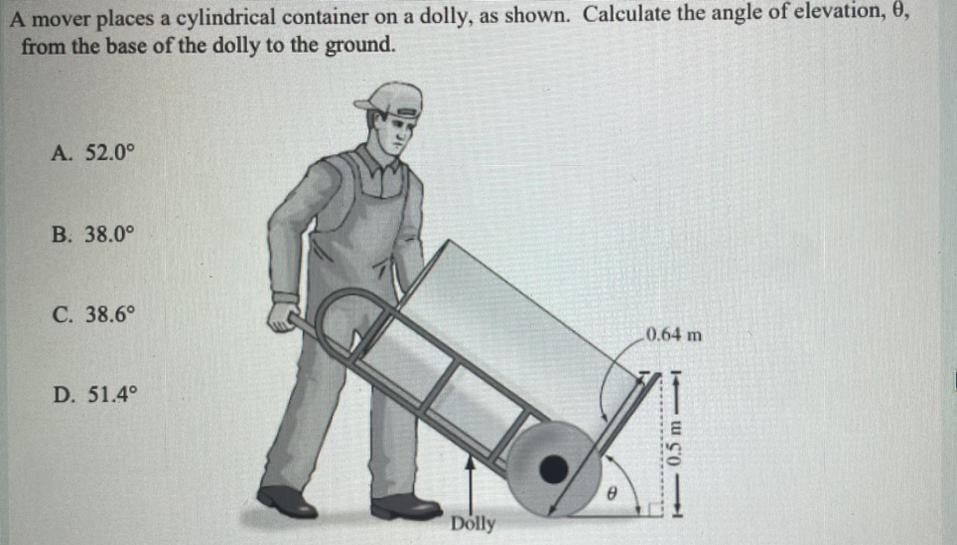
A mover places a cylindrical container on a dolly, as shown. Calculate the angle of elevation, U,
from the base of the dolly to the ground.
A. 52.0°
B. 38.0°
C. 38.6°
D. 51.4°
Answers
Check the picture below.
\(\sin( \theta )=\cfrac{\stackrel{opposite}{0.5}}{\underset{hypotenuse}{0.64}} \implies \sin^{-1}(~~\sin( \theta )~~) =\sin^{-1}\left( \cfrac{0.5}{0.64} \right) \\\\\\ \theta =\sin^{-1}\left( \cfrac{0.5}{0.64} \right)\implies \theta \approx 51.4^o\)
Make sure your calculator is in Degree mode.

Makayla wants to classify the triangle below. Select all of the terms that apply.
Right triangle with base of 5 cm, height of 5 cm, and hypotenuse of 7.1 cm.
A.
acute
B.
isosceles
C.
obtuse
D.
right
E.
scalene
Answers
Answer:
B and D
Step-by-step explanation:
B) Isosceles , this is one answer because two sides of the triangle are exactly the same measurement .
D) Right , this is the second answer because that little box in the corner there represents a 90 degree angle which is in turn a right angle .
Caleb is designing a conveyor belt that has to move manufactured products up an incline, as shown above. To avoid products sliding or toppling, he has determined an ideal angle above the horizontal of 0.19 radians.What distance, d, in meters, will the product be conveyed using Caleb's design? (Round your answer to the nearest tenth of a meter.) HELP MEEE !!!

Answers
Answer:
16.3 meters
Step-by-step explanation:
1 pi radian =180°
0.19 =?
(0.19×180)÷3.142=10.9°
cos 10.9=16/d
d cos 10.9 =16
d=16÷cos 10.9
d=16.3 meters
One of the tables represents a linear function. Which table is it?

Answers
Answer:
C.
Step-by-step explanation:
The rate of change of a linear function is usually constant and the same between any two coordinate pairs all through.
Rate of change is given as ∆y/∆x.
For the table in option C, the rate of change is constant all through.
We have: (12 - 8)/(3 - 2) = (16 - 12)/(4 - 3) = (20 - 16)/(5 - 4) = 4
Rate of change of constant all through. If we graph the table of values, we'd get a straight line.
Therefore, the table in option C represents a linear function.
Answer:
c
Step-by-step explanation: