If Tiva babysits for hours, she will earn $32. how many hours will she get to earn 32 $
Answers
Answer:
The questions does not make any sense
Step-by-step explanation:
You need to say how much they make an hour
Answer:4 hours
Step-by-step explanation:
Related Questions
Elizabeth is going to invest $480 and leave it in an account for 8 years. Assuming the interest is compounded continuously, what interest rate, to the nearest tenth of a percent, would be required in order for Elizabeth to end up with $670?
Answers
Answer:
4.2
Step-by-step explanation:
took a bullet for the team
Elizabeth would need an interest rate of 4.25% to make a profit of $670, to the closest hundredth of a percent.
What is compound interest?The interest earned on savings that are computed using both the original principal and the interest accrued over time is known as compound interest. A = P(1 + r/n)^nt, where P is the principal balance, r is the interest rate, n is the number of times interest is compounded annually, and t is the number of years, which is the formula for compound interest.
Given, Elizabeth is going to invest $480 and leave it in an account for 8 years.
from the formula of compound interest
A = P(1 + r/n) ^nt
in our case,
A =670
P = 480
t = 8
Thus,
670 = 480(1 + r)^8
r = 4.25%
Therefore, an interest rate, to the nearest tenth of a percent, would be 4.25% required in order for Elizabeth to end up with $670
Learn more about compound interest here:
https://brainly.com/question/29335425
#SPJ2
3. Find m2ABC.
(2x+14)
(x+7)°

Answers
Answer:
Step-by-step explanation:

For the function below find a) the critical numbers; b) the open intervals where the function is increasing, and c) the open intervals where it is decreasing f(x)=8x³-42x-48x + 4 a) Find the critical number(s). Select the correct choice below and, if necessary fill in the answer box to complete your choice. A. The critical number(s) is/are (Type an integer or a simplified fraction. Use a comma to separate answers as needed
Answers
A) Function is increasing on (-∞, -1) and (7/2, ∞), and decreasing on (-1, 7/2).
b) The local minimum value of f is; 5608/2197 at x = -42/13, and the local maximum value of f is 139/8 at x = 7/2.
(a) To determine the intervals on which f is increasing or decreasing, we need to determine the critical points and then check the sign of the derivative on the intervals between them.
f(x)=8x³-42x-48x + 4
f'(x) = 24x² - 90
Setting f'(x) = 0, we get
24x² - 90 = 0
24x² = 90
x =± √3.75
So, the critical points are;
x = -1 and x = 7/2.
We can test the sign of f'(x) on the intervals as; (-∞, -1), (-1, 7/2), and (7/2, ∞).
f'(-2) = 72 > 0, so f is increasing on (-∞, -1).
f'(-1/2) = -25 < 0, so f is decreasing on (-1, 7/2).
f'(4) = 72 > 0, so f is increasing on (7/2, ∞).
Therefore, f is increasing on (-∞, -1) and (7/2, ∞), and decreasing on (-1, 7/2).
(b) To determine the local maximum and minimum values of f, we need to look at the critical points and the endpoints of the interval (-1, 7/2).
f(-1) = -49
f(7/2) = 139/8
f(-42/13) = 5608/2197
Learn more about maximum value here:
brainly.com/question/23504923
#SPJ4
I need this answer, ASAP please

Answers
Answer:
43
Step-by-step explanation:
2 significant figures means we keep the first 2 and round the 1 place
42.598 we round to 43 since next to the 2 is a 5 so we round up
And 43 are 2 significant figures since none of them are zero
Hopes this helps please mark brainliest
solve this questionn

Answers
I don't think anyone can, that's the wrong pic
PLSSSS HELPPPP PLSSSS PLS HELPPPPP!!!!!!
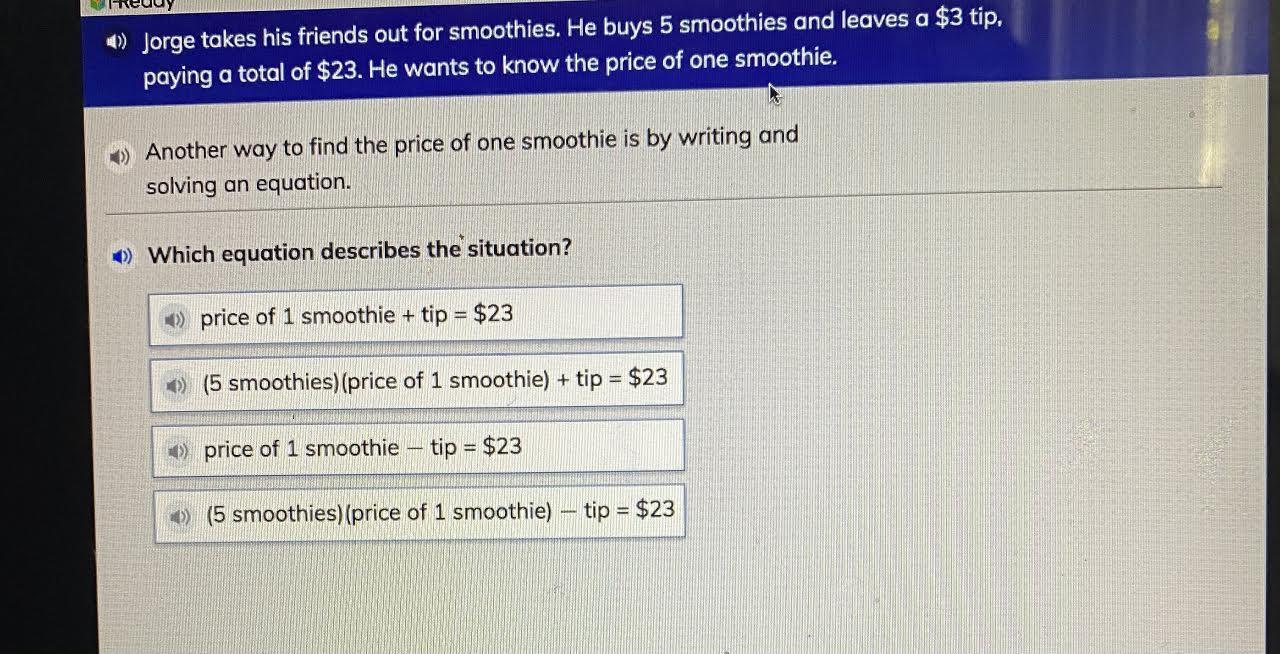
Answers
Answer:
B -- (5 smoothings)(price of 1 smoothie) + tip = $23
Step-by-step explanation:
5 smoothies(price of 1 smoothie)+tip=23
Q5. A farm raises cows and chickens. The farmer has a total of 42 animals. One day he counts the legs of all of his animals and realizes he has a total of 114.
a) How many cows does the farmer have?
b) How many chickens?
c) What is the importance of farmer?
Answers
Answer:
let cow=x and chickens =y
cows have 4 legs, 4x. chickens have 2 legs, 2y

Answer:
a= 15 cows b= 27 chickens c= none, he is not an animal.
Step-by-step explanation:
cows have four legs
chickens have two
15 cows = 60 legs
27 chickens = 54 legs
60+54 = 114
please make me the brainliest if you find this helpful :)
Find the distance between (-5,3) and (2,-1).
Answers
Answer:
distance = sqrt( 65 ) ~= 8.06
Step-by-step explanation:
To find the distance between two points, we can use the distance formula.
distance = sqrt( [x2 - x1]^2 + [y2 - y1]^2)
distance = sqrt( [-5 - 2]^2 + [3 - -1]^2)
distance = sqrt( [-7]^2 + [4]^2)
distance = sqrt( 49 + 16 )
distance = sqrt( 65 ) ~= 8.06
Cheers.
Answer: d=8.06
Step-by-step explanation:
To this the distance between two points, you would need the distance formula.
Distance Formula: \(d=\sqrt{(x_{2}-x_{1} )^2+(y_{2} -y_{1} )^2}\)
Since we have two given points, all we have to do is plug them in.
\(d=\sqrt{(2-(-5) )^2+(-1 -3 )^2}\) [parentheses]
\(d=\sqrt{(7)^2+(-4 )^2}\) [exponent]
\(d=\sqrt{49+16}\) [add]
\(d=\sqrt{65}\) [square root]
\(d=8.06\)
Now we know that the distance is d=8.06.
Please Help Me. I really don't understand this question. I attached the question. Please zoom in to see better.

Answers
Step-by-step explanation:
I don't see the main question but this is the binomial expansion of the expression (4x-2y)^5
Answer:
below
Step-by-step explanation:
The answer of the first expert is correct, this is from Pascal triangle 5th level.
shown in the attached picture, when we extend the brackets the whole function will become...
f(x) = 1*(4x)⁵(-2y)⁰ + 5*(4x)⁴(-2y)¹ + 10*(4x)³(-2y)² + 10* (4x)²(-2y)³ + 5*(4x)¹(-2y)⁴ + 1*(4x)⁰(-2y)⁵
= (4x - 2y)⁵
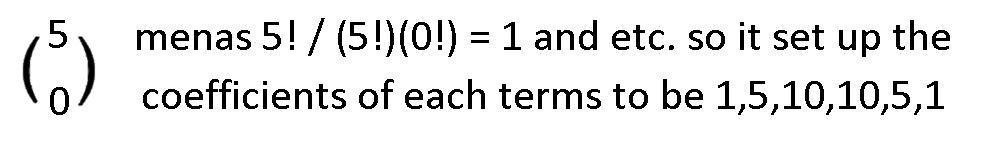
PLEASE HELP 30 POINTS EASY

Answers
Answer
Step-by-step explanation:
Let's solve for z.
a(t+z)=45z+67
Step 1: Add -45z to both sides.
at+az+−45z=45z+67+−45z
at+az−45z=67
Step 2: Add -at to both sides.
at+az−45z+−at=67+−at
az−45z=−at+67
Step 3: Factor out variable z.
z(a−45)=−at+67

Willow homework sheet is 3×19 how can you use base 10 blocks to show 3×19
Answers
Answer: 27
Step-by-step explanation:
two base ten blocks then 7
Suppose the future value of a \( 7.75 \% \) simple interest loan is \( \$ 1,321.17 \) at the end of 230 days. Find the present value of the loan. State your result to the nearest penny.
Answers
The present value of the loan is found to be approximately $70.28.
To find the present value of a loan, we can use the formula:
Present Value = Future Value / (1 + (interest rate * time))
Given that the future value is $1,321.17, the interest rate is 7.75%, and the time is 230 days, we can plug in these values into the formula:
Present Value = 1321.17 / (1 + (0.0775 * 230))
Calculating the expression in the parentheses first:
Present Value = 1321.17 / (1 + 17.8075)
Simplifying further:
Present Value = 1321.17 / 18.8075
Evaluating the division:
Present Value ≈ $70.28
Therefore, the present value of the loan is approximately $70.28.
Know more about the present value
https://brainly.com/question/30390056
#SPJ11
Suppose V is finite-dimensional. Prove that every linear map on a subspace of V can be extended to a linear map on V. In
other words, show that if U is a subspace of V and S â L(U, W), then there exists T â L(V, W) such that Tu = Su for all
u â U.
Answers
To prove that every linear map on a subspace of V can be extended to a linear map on V. We have to show that there exists a linear map T from V to W that extends S and satisfies Tu = Su for all u in U.
Let U be a subspace of a finite-dimensional vector space V, and let S be a linear map from U to another vector space W. du in U.
Since V is finite-dimensional, ready to select a premise {u1, u2, ..., um} of U and expand it to the premise {u1, u2, ..., um, v1, v2, ... increment. Let {w1, w2, ..., wk} be the premise of W.
You can define T using the base elements of V as follows:
T(uj) = S(uj) for j = 1, 2, ..., m (since uj is in U and S is a linear map from U to W)
T(vi) = 0 for i = 1, 2, ..., n (to ensure that T is a linear map)
We can extend T linearly to all of V by defining T as
For any vector v in V, we can write v as a linear combination of basis elements.
v = a1u1 + a2u2 + ... + amount + b1v1 + b2v2 + ... + bnvn
Then we can define T(v) as
T(v) = a1T(u1) + a2T(u2) + ... + amT(um) + b1T(v1) + b2T(v2) + ... + bnT(vn)
Structurally, this definition of T agrees with the definition of S on the subspace U, since T(uj) = S(uj) for j = 1, 2, ..., m. Since T is a linear map on V, it is also well-defined and satisfies the linear property.
T(cv + w) = cT(v) + T(w)
For every vector v, w in V, and every scalar c. Thus, we showed that there exists a linear map T from V to W that extends S and satisfies Tu = Su for all u in U.
learn more about vector space
brainly.com/question/13058822
#SPJ4
8 9 and 10 please will mark brainliest!

Answers
Answer:
i dont know sorry
Step-by-step explanation:
i dont know sorry
Answer:
please. mark brainlist
Step-by-step explanation:
8) is a right triangle
9)i think this one is a actute triangle am not sure
10)is a right triangle
Joyce paid $70 for an item at the store that was 70% off the original price. What was the original price?
Answers
Answer:119
Step-by-step explanation:
Solve the following differential equation using series solutions. y"(x) + 3y(x) = 0. Problem 3. Solve the following differential equation using series solutions. ry'(a) + 2y(x) = 42², with the initial condition y(1) = 2.
Answers
To solve the differential equation y"(x) + 3y(x) = 0 using series solutions, we can assume a power series solution of the form:
y(x) = ∑[n=0 to ∞] (a_n * \(x^n),\)
where \(a_n\)are the coefficients to be determined.
Differentiating y(x) with respect to x, we get:
y'(x) = ∑[n=0 to ∞] (n * \(a_n\)* \(x^(n-1)).\)
Differentiating y'(x) with respect to x again, we get:
y"(x) = ∑[n=0 to ∞] (n * (n-1) * \(a_n\)\(* x^(n-2)).\)
Substituting these expressions into the original differential equation:∑[n=0 to ∞] (n * (n-1) * \(a_n\) * x^(n-2)) + 3 * ∑[n=0 to ∞] \(a_n\) * \(x^n)\)= 0.
Now, we can rewrite the series starting from n = 0:
\(2 * a_2 + 6 * a_3 * x + 12 * a_4 * x^2 + ... + n * (n-1) * a_n * x^(n-2) + 3 * a_0 + 3 * a_1 * x + 3 * a_2 * x^2 + ... = 0.\)
To satisfy this equation for all values of x, each coefficient of the powers of x must be zero:
For n = 0: 3 * \(a_0\) = 0, which gives \(a_0\) = 0.
For n = 1: 3 * \(a_1\) = 0, which gives\(a_1\) = 0.
For n ≥ 2, we have the recurrence relation:
\(n * (n-1) * a_n + 3 * a_(n-2) = 0.\)
Using this recurrence relation, we can solve for the remaining coefficients. For example, a_2 = -a_4/6, a_3 = -a_5/12, a_4 = -a_6/20, and so on.
The general solution to the differential equation is then:
\(y(x) = a_0 + a_1 * x + a_2 * x^2 + a_3 * x^3 + ...,\)
where a_0 = 0, a_1 = 0, and the remaining coefficients are determined by the recurrence relation.
To solve the differential equation\(ry'(x) + 2y(x) = 42^2\) with the initial condition y(1) = 2 using series solutions, we can proceed as follows:
Assume a power series solution of the form:
y(x) = ∑[n=0 to ∞] (\(a_n\) *\((x - a)^n),\)
where\(a_n\)are the coefficients to be determined and "a" is the point of expansion (in this case, "a" is not specified).
Differentiating y(x) with respect to x, we get:y'(x) = ∑[n=0 to ∞] (n *\(a_n * (x - a)^(n-1)).\)
Substituting y'(x) into the differential equation:
r * ∑[n=0 to ∞] (n * \(a_n\)* \((x - a)^(n-1))\) + 2 * ∑[n=0 to ∞] (\(a_n\)*\((x - a)^n\)) = \(42^2.\)
Now, we need to determine the values of \(a_n\) We can start by evaluating the expression at the initial condition x = 1:
y(1) = ∑[n=0 to ∞] \((a_n * (1 - a)^n) = 2.\)
This equation gives us information about the coefficients \(a_n\)and the value of a. Without further information, we cannot proceed with the series solution.
Please provide the value of "a" or any additional information necessary to solve the problem.
Learn more about differential equation here:
https://brainly.com/question/1164377
#SPJ11
A cell phone company uses the equation C=$0.15t+$35.00 to determine the total cost, C, for a month of service based on the number of text messages, t. Identify the slope.
Answers
Slope is $0.15 of the equation of cost C=$0.15t+$35.00.
What is Slope of Line?The slope of the line is the ratio of the rise to the run, or rise divided by the run. It describes the steepness of line in the coordinate plane.
The slope intercept form of a line is y=mx+b, where m is slope and b is the y intercept.
The slope of line passing through two points (x₁, y₁) and (x₂, y₂) is
m=y₂-y₁/x₂-x₁
Given that A cell phone company uses the equation C=$0.15t+$35.00
C is the total cost for a month of service.
t is the number of text messages.
We have to find the slope of the equation.
slope is 0.15 and 35.00 is the y intercept of the equation given.
Hence, slope is $0.15 of the equation of cost C=$0.15t+$35.00.
To learn more on slope of line click:
https://brainly.com/question/14511992
#SPJ1
What is the measure of each interior angle of a regular pentagon Step by step pls
Answers
Answer:
To find the measure of each interior angle of any regular polygon, we use the formula {(n - 2) × 180} / n degrees, where n is the number of sides of the polygon.
Step-by-step explanation:
if an object is dropped from a height of 38 feet, the function h(t) = -16t square + 38 gives the height of the object after t seconds. Graph the function.
Answers
The graph of the quadratic equation can be seen below.
How to graph the quadratic equation?
Here we have a quadratic equation that represents the height:
\(h(t) = -16t^2 + 38\)
Where this represents the height in ft.
This is just a quadratic function, so to graph it we can just get some points and then connect them, by evaluating the function we will get:
\(h(0) = -16*0^2 + 38 = 38\\\\h(1) = -16*1^1 + 38 = 22\\\\h(2) = -16*1.5 + 38 = 2\)
So we have the 3 points (0, 38), (1, 22) and (2, 2), we graph them and connect them with a parabola. The graph can be seen below.
If you want to learn more about quadratic equations:
https://brainly.com/question/1214333
#SPJ1
You deposit $6,500 in an account that pays 4.5% annual interest. Find the balance after 5 years when the interest is compounded monthly.
Answers
The balance after 5 years is equal to $8,136.67.
How to determine the future value after 5 years?In Mathematics and Financial accounting, compound interest can be calculated by using the following mathematical equation (formula):
\(A(t) = P(1 + \frac{r}{n})^{nt}\)
Where:
A represents the future value.n represents the number of times compounded.P represents the principal.r represents the interest rate.t represents the time measured in years.By substituting the given parameters into the formula for compound interest, we have the following;
\(A(5) = 6,500(1 + \frac{0.045}{12})^{12 \times 5}\\\\A(5) = 6,500(1.00375)^{60}\)
Future value, A(5) = $8,136.67
Read more on compound interest here: brainly.com/question/16608367
#SPJ1
WILL GIVE BRANLIEST AND BE SOOO HAPPY PLEASE HELP!!! 30 POINTS SHARE YOUR SMARTNESS!!


Answers
Answer:
s4 = 270
18+36+72+144 = 270
-- convergent sums to a single value
Step-by-step explanation:
S4 = 270
Step-by-step explanation:
The 4th partial sum of the sequence is the sum of the first 4 terms:
S4 = a(1) + a(2) + a(3) + a(4)
Because the sequence is geometric any term can be written in terms of the previous one:
a(n) = r a(n - 1)
And more importantly in terms of the first term a(1) so that
a(2) = r a(1)
a(3) = r a(2) = r^2 a(1)
a(4) = r a (3) = r^3 a(1)
Then the 4th partial sum is
S4 = a(1) + r a(1) + r^2 a(1) + r^3 a(1)
S4 = a(1) (1 + r + r^2 + r^3)
With a a(1) = 18 and r = 2 we have
S4 = 18 (1 + 2 + 4 + 8) = 270
Therefore, the 4th partial sum is 270
Which equation can be used to prove 1 + tan2(x) = sec2(x)?
StartFraction cosine squared (x) Over secant squared (x) EndFraction + StartFraction sine squared (x) Over secant squared (x) EndFraction = StartFraction 1 Over secant squared (x) EndFraction
StartFraction cosine squared (x) Over sine squared (x) EndFraction + StartFraction sine squared (x) Over sine squared (x) EndFraction = StartFraction 1 Over tangent squared (x) EndFraction
StartFraction cosine squared (x) Over tangent squared (x) EndFraction + StartFraction sine squared (x) Over tangent squared (x) EndFraction = StartFraction 1 Over tangent squared (x) EndFraction
StartFraction cosine squared (x) Over cosine squared (x) EndFraction + StartFraction sine squared (x) Over cosine squared (x) EndFraction = StartFraction 1 Over cosine squared (x) EndFraction
Answers
The equation that can be used to prove 1 + tan2(x) = sec2(x) is StartFraction cosine squared (x) Over tangent squared (x) EndFraction + StartFraction sine squared (x) Over tangent squared (x) EndFraction = StartFraction 1 Over tangent squared (x) EndFraction. the correct option is d.
How to explain the equationIn order to prove this, we can use the following identities:
tan(x) = sin(x) / cos(x)
sec(x) = 1 / cos(x)
tan2(x) = sin2(x) / cos2(x)
sec2(x) = 1 / cos2(x)
Substituting these identities into the given equation, we get:
StartFraction cosine squared (x) Over tangent squared (x) EndFraction + StartFraction sine squared (x) Over tangent squared (x) EndFraction = StartFraction 1 Over tangent squared (x) EndFraction
Therefore, 1 + tan2(x) = sec2(x).
Learn more about equations on
https://brainly.com/question/2972832
#SPJ1
ep 3 of 3: which statistic is most appropriate for the pizzeria owner to determine the usefulness of the regression model and why?
Answers
To determine the usefulness of the regression model for the pizzeria owner, the most appropriate statistic would be the coefficient of determination (R-squared).
The coefficient of determination, also known as R-squared, measures the proportion of the variation in the dependent variable that is explained by the independent variables in a regression model. It provides a measure of how well the regression model fits the data.
By examining the R-squared value, the pizzeria owner can determine the usefulness of the regression model in predicting or explaining the variability in their business-related variable, such as pizza sales. A high R-squared value (close to 1) indicates that the model is successful in explaining a large portion of the variation in the dependent variable. On the other hand, a low R-squared value (close to 0) suggests that the model is not effective in capturing the relationships between the independent and dependent variables.
Therefore, the pizzeria owner should use the coefficient of determination (R-squared) as the most appropriate statistic to assess the usefulness of the regression model, as it quantifies the model's ability to explain the observed variation in the dependent variable.
Learn more about dependent variables: brainly.com/question/25223322
#SPJ11
Find the value of x and y.

Answers
Answer:
X=63 y=27
Step-by-step explanation:
X and 117 are on a straight line. All straight lines = 180 degrees.
180 - 117 = 63
90 - 63 = 27
help..564 patrons can borrow up to 6 books. If all the patrons are currently holding on to 6 books each, how many books are left in the library?
Answers
Answer:
3,384
Step-by-step explanation:
So this is a classic multiplication problem. If each patron has 6 books, and there's 564 patrons, you multiply:
564 x 6 = 3,384
hope this helps:D
Do you ever subtract using the z-score?
A) yes
B) no
C) sometimes
Answers
the busy bee store bottles fresh jars of honey at a constant rate. in 2 hours, it bottles 20 jars, and in 5 hours, it bottles 50 jars of honey. determine the constant of proportionality. 0.1 5 10 20
Answers
The constant of proportionality is 10. The correct option is C.
Given that the busy bee store bottles fresh jars of honey at a constant rate in 2 hours, it bottles 20 jars, and in 5 hours, it bottles 50 jars of honey.
We have to find the constant of proportionality.
The constant of proportionality is the ratio of the so-called proportional relationship that relates two given values to each other.
As it is given that in 2 hours 20 jars of honey filled.
That means the constant of proportionality is ratio of jars with time and we get
r₁=20/2
r₁=10 jars in hour
Similarly, it is given that in 5 hours 50 jars of honey filled.
That means the constant of proportionality is ratio of jars with time and we get
r₂=50/5
r₂=10 jars in hour
Here, r₁=r₂=10 which is the constant of proportionality.
Hence, the constant of proportionality when the busy bee store bottles fresh jars of honey at a constant rate. in 2 hours, it bottles 20 jars, and in 5 hours, it bottles 50 jars of honey is 10.
Learn more about the constant of proportionality from here brainly.com/question/26171814
#SPJ4
Find f(2) if f(x)=2x+1
Answers
\(f(x) =2 x+1 \\\\ \text{If x = 2,}\\\\f(2) = 2(2) +1 = 4+1 = 5\)
Answer:
5
Step-by-step explanation:
plug 2 in for x
f(2) = 2(2) + 1
f(2) = 5 [Asnwer]
PLEASE RATE!! I hope this helps!!
If you have any questions comment below
62.4% of what number is 17.16?
What percent of 51 is 127.5?
(Those are two questions but like on the same thing )
Answers
Answer:
first: 27.5
second: 250%
Step-by-step explanation:
first: 17.16 ÷ 62.4% = 0.275, 27.5
second: 51 ÷ 127.5 = 0.4, 40%,
100% ÷ 40%, = 2.5, 250%
PLS HELP WITH THIS
In the diagram below, m
pls state reasons with answers

Answers
Answer:
DCB=50 degree (sum of interior angle of quadrilateral)