in right triangle ABC, m
Answers
Answer:
In right triangle ABC, right angled at C, M is the mid-point of hypotenuse AB. C is joined to M and produced to a point D such that DM = CM.
Related Questions
What do the Nineteenth Amendment and the Indian Citizenship Act of 1924
have in common?
O A. They both affected American Indians directly.
O B. They both allowed certain Americans to own property.
O C. They both permitted free expression with some restrictions.
O D. They both provided suffrage to a group of Americans.
Answers
Answer:
D
Step-by-step explanation:
Suffrage is the right to vote, and the nineteenth amendment gave women the right to vote, and the indian citizenship act of 1924 gave native americans the right to vote
can someone help with another question i posted 5 minutes ago
Answers
Answer:
Awnser
what is the question
which of the following are identities

Answers
The identities among the given statements are:
tan x = sin x / cos x
sin x / tan x = cos x
We have,
Let's analyze each of the given statements to determine whether they are identities:
tan x = sin x / cos x:
This is an identity.
It is known as the fundamental identity of trigonometry, as it holds true for all values of x (except when cos x is equal to 0).
tan x cos x = sin x:
This is not an identity.
It is a specific equation that may be true for certain values of x, but it does not hold true for all values of x.
cos x = tan x sin x:
This is not an identity.
Similar to the previous statement, it is a specific equation that may be true for certain values of x, but it does not hold true for all values of x.
sin x / tan x = cos x:
This is an identity.
It can be derived from the fundamental identity by dividing both sides by cos x, resulting in sin x / cos x = cos x / cos x, which simplifies to sin x / tan x = cos x.
Thus,
The identities among the given statements are:
tan x = sin x / cos x
sin x / tan x = cos x
Learn more about trigonometric identities here:
https://brainly.com/question/14746686
#SPJ1
What does cutting Della's hair symbolize?
Answers
Cutting Della's hair symbolizes her mean sacrifice and desperation to provide a Christmas gift for her husband. It is a symbol of her love and devotion to him, even in the face of poverty.
Cutting Della's hair symbolizes her selfless devotion to her husband and her willingness to make a sacrifice in order to provide a Christmas gift for him. Despite lacking the money to buy a gift, she still wanted to give him something to show her love and appreciation for him. This is why she cut off her long, beautiful hair and sold it to the hairdresser for twenty dollars in order to buy her husband a platinum watch chain. Her act of cutting her hair is a powerful symbol of her love and devotion to him, even in the face of extreme poverty. Additionally, it symbolizes her strength and resilience in the face of difficult circumstances. Her sacrifice is a reminder of how far love can go in times of hardship.
Learn more about mean here
https://brainly.com/question/15323584
#SPJ4
Please answer the following equation? 4x^2-27x+18Factor and solve for the x-intercept
Answers
SOLUTION
Write out the expression
\(4x^2-27x+18\)step1: Multiply the coefficient of the first and last term
\(4x^2\times18=72x^2\)Step2: Obtain the factors of the term above that can conveniently replace the second term n the expression
\(\begin{gathered} 72x^2=-24x\times-3x \\ -27x=-24x-3x \end{gathered}\)Step3: Replace the second term with the two terms obtained
\(\begin{gathered} 4x^2-27x+18\text{ becomes } \\ 4x^2-24x-3x+18 \end{gathered}\)Step4: Group the term and obtain the common factors
\(\begin{gathered} (4x^2-24x)-(3x+18) \\ 4x(x-6)-3(x-6) \\ (x-6)(4x-3) \end{gathered}\)Hence the factors of the expression are (4x-3)(x-6)
The X- intercept is the point where the expression to zero
\(\begin{gathered} (4x-3)(x-6)=0 \\ \text{equate each to zero} \\ 4x-3=0\text{ or x-6=0} \\ 4x=3\text{ or x=6} \\ \text{Then } \\ x=\frac{3}{4},6 \end{gathered}\)Hence the X-intercept is (3/4,0) and (6,0)
Which whole number property was used?
23 + 2 = 2 + 23
Commutative Property of Addition
Associative Property of Addition
Identity Property of Addition
Answers
Answer:
Commutative property of addition is the correct option.
Answer:
the answer commutative my friend
Please answer it now in two minutes

Answers
Answer:
59.0
Step-by-step explanation:
Given a right angled triangle, ∆XYZ, to know which trigonometric ratio formula to apply in finding the measure of angle X, note the following:
Opposite side to angle X = 6
Hypotenuse = 7
Therefore, we would apply the following trigonometric ratio formula to solve for m<X:
\( sin X = \frac{6}{7} \)
\( sin X = 0.8571 \)
\( X = sin^{-1}(0.8571) \)
\( X = 58.99 \)
\( m < X = 59.0 \) (rounded to nearest tenth)
tell whether each equation has one zero or infinitely many solutions.
2. 1/2n + 7 = (n+14)/2
Answers
Answer:
n/2 + 7 is always equal to (n + 14)/2
Step-by-step explanation:
Both equations are the same, re-written.
Simplify the following:
n/2 + 7
Put each term in n/2 + 7 over the common denominator 2: n/2 + 7 = n/2 + 14/2:
n/2 + 14/2
n/2 + 14/2 = (n + 14)/2:
Answer: (n + 14)/2
A dairy produces 420,000 quarts of milk each day. There are 4 quarts in a gallon. How many gallons of milk are produced each day? A 105,000 gal B) 1,680,000 gal С 1,050,000 gal D 10,500 gal Question 10 2 Points
Answers
Answer:
150000
Step-by-step explanation:
i use cal
The figure below shows the dimensions of a section of Mr. Green's gardent that he will use for planting flowers. What is the area of Mr.
Green's garden that he will use?

Answers
Answer:
39
Step-by-step explanation:
a smallercircle passes through the center of, and is tanget to a larger cirlc the area of the smaller circle is 9 square units. What is teh area of the larger circle in square units
Answers
36 square units make up the larger circle's surface area.
We can use the fact that the smaller circle is tangent to the larger circle and passes through its center to find the area of the larger circle. Since the radius of the smaller circle is tangent to the larger circle, the radius of the larger circle is double the radius of the smaller circle.
Let's call the radius of the smaller circle "r" and the radius of the larger circle "R". We know that R = 2r.
We know that the area of a circle is given by the formula A = πr^2.
We know that the area of the smaller circle is 9 square units, we can use this formula to find the radius:
A = πr^2 => r^2 = 9/π => r = sqrt(9/π)
Now we can use the formula of the area of a circle with R-value to get the area of the larger circle:
A = πR^2 => A = π(2r)^2 => A = 4πr^2 => A = 4π(9/π) => A = 36
So the area of the larger circle is 36 square units
To learn more about the area of the circle, refer to the link:brainly.com/question/28642423
#SPJ4
Use the graph shown below to identify the multiplicity of the roots of f(x)=0.

Answers
If the multiplicity of the roots was 3, the curve would still be tangent to the x-axis at -3 and 3, but the curve would cross the x-axis.
The graph below is the graph of the equation f(x) = 0. The equation is graphed on the x-y plane. To determine the multiplicity of the roots, one needs to look at the graph closely.
The multiplicity of the roots of a polynomial function is a way of determining the behavior of the function as it approaches a particular point on the x-axis. In particular, it tells us how quickly the function approaches zero at that point.
The graph shows a curve that intersects the x-axis at -3 and 3. At these two points, the curve is tangent to the x-axis. Since the curve is tangent to the x-axis at these points, this means that the roots are repeated.
The multiplicity of the roots of f(x) = 0 is 2. This means that the curve touches the x-axis at -3 and 3, but doesn't cross it. This is because the roots are repeated.
If the multiplicity of the roots was 3, the curve would still be tangent to the x-axis at -3 and 3, but the curve would cross the x-axis.
For more such questions on tangent, click on:
https://brainly.com/question/30162650
#SPJ8
Here are the first four terms of
an arithmetic sequence.
5 8 11 14
Find an expression, in terms of
for the..th term of the
sequence.
Answers
————————————————-
An interval estimate for the average number of first year students at UQ in Semester 1 of 2019 was reported to be 33112 to 36775 students. This interval estimate was based on a sample of 47 students. The variance of the student population was determined from previous studies to be 44885212 students squared. What level of confidence can be attributed to this interval estimate? State your answer as a percentage, correct to the nearest whole number.
Answers
The level of confidence interval estimate for the average number of first-year students at UQ in Semester 1 of 2019, ranging from 33,112 to 36,775 students, based on a sample of 47 students, can be calculated.
To determine the confidence level, we need to consider the concept of margin of error. The margin of error is the maximum likely difference between the sample estimate and the true population value.
In this case, the margin of error can be calculated by taking half the width of the interval estimate, which is (36,775 - 33,112)/2 = 1,831.5 students.
The confidence level is related to the margin of error through the formula:
Confidence level = 1 - α
Here, α represents the significance level, which is the probability of making a Type I error (rejecting a true null hypothesis). The complement of α gives us the confidence level. In other words, a confidence level of 95% corresponds to a significance level of 0.05.
To calculate the confidence level, we need to find the critical value associated with the sample size and the chosen significance level. Since the sample size is 47 and the variance of the student population is known to be 44,885,212, we can use the t-distribution for small sample sizes.
Using a calculator, we find that the critical value for a significance level of 0.05 and 46 degrees of freedom (47 - 1) is approximately 2.014. The critical value is the number of standard errors away from the mean needed to capture the desired confidence level.
Finally, we can calculate the confidence level as follows:
Confidence level = 1 - α = 1 - 0.05 = 0.95 = 95%
Therefore, the level of confidence that can be attributed to this interval estimate is 95%.
To know more about confidence intervals, refer here:
https://brainly.com/question/32546207#
#SPJ11
what is 90 divided into the ratio 1:3:5
Answers
Answer:
10
Step-by-step explanation:
So 1:3:5 will be a total of 9 (1 + 3 + 5), so 90 divided into the ratio of 1:3:5 will be 10. I hope this helps, and hopefully you could give me Brainliest! Have a nice day :D
Please help me with this

Answers
Answer:
i dont know
Step-by-step explanation:
Work out x^2- 2x
when x =4
Answers
Answer:
8
Step-by-step explanation:
\(x^2-2x \\x=4\\(4)^2-2(4)\\16-8\\8\)
George has a spinner with three equal sections colored orange, blue, and white. He spins the spinner 100 times. What is the experimental probability of landing on the orange section?
Answers
Answer:
1/3
Step-by-step explanation:
Probability calculates the likelihood of an event occurring. The likelihood of the event occurring lies between 0 and 1. It is zero if the event does not occur and 1 if the event occurs.
For example, the probability that it would rain on Friday is between o and 1. If it rains, a value of one is attached to the event. If it doesn't a value of zero is attached to the event.
Experimental probability is based on the result of an experiment that has been carried out multiples times
probability of landing on the orange section = proportion of the orange section / total proportions of all the colours
= 1/3
Using simplex method to solve the following problems: (Manual calculations and then confirm your calculation by any software) Max. Z=5A+4B Subject to constraints: 6 A+4 B≤24, A+2 B≤6,−A+B≤1, B≤2, A, B≥0
Answers
Using the simplex method, the maximum value of Z=5A+4B is found to be 19.2 when A=3.6 and B=1.2. The calculations can be confirmed by using any software that solves linear programming problems.
To solve the given linear programming problem using the simplex method, we start by converting the problem into standard form. We introduce slack variables to convert the inequalities into equations.The initial tableau is as follows:
| A | B | S1 | S2 | S3 | S4 | RHS
------------------------------------------
Z | -5 | -4 | 0 | 0 | 0 | 0 | 0
------------------------------------------
S1 | 6 | 4 | 1 | 0 | 0 | 0 | 24
S2 | 1 | 2 | 0 | 1 | 0 | 0 | 6
S3 | -1 | 1 | 0 | 0 | 1 | 0 | 1
S4 | 0 | 1 | 0 | 0 | 0 | 1 | 2
We perform the simplex iterations until the optimal solution is reached. After applying the simplex method, the final tableau is obtained as follows:
| A | B | S1 | S2 | S3 | S4 | RHS
------------------------------------------------------
Z | 0 | 1.8 | 0.2 | -1 | -0.4 | 0.4 | 19.2
------------------------------------------------------
S1 | 0 | 0 | 0 | 1.5 | -1 | 1 | 3
S2 | 1 | 0 | -0.5 | 0.5 | 0.5 | -0.5 | 1.5
A | 1 | 0 | 0.5 | -0.5 | -0.5 | 0.5 | 0.5
S4 | 0 | 0 | 1 | -1 | -1 | 1 | 1
From the final tableau, we can see that the maximum value of Z is 19.2 when A=3.6 and B=1.2. This solution satisfies all the constraints of the problem. The calculations can be verified using any software that solves linear programming problems, which should yield the same optimal solution.
Learn more about linear programming here:- brainly.com/question/30763902
#SPJ11
pls if you can help

Answers
Answer:
x ≈ 8.4
Step-by-step explanation:
10
using the cosine ratio in the right triangle
cos64° = \(\frac{adjacent}{hypotenuse}\) = \(\frac{MN}{LN}\) = \(\frac{3.7}{X}\) ( multiply both sides by x )
x × cos64° = 3.7 ( divide both sides by cos64° )
x = \(\frac{3.7}{cos64}\) ≈ 8.4 ( to the nearest tenth )
Who is the richest person in the world?
Elon Musk
Jeff Bezos
Bill Gates
Bernard Arnault
Answers
Answer:
Elon Musk
Step-by-step explanation:
He just became the richest person in the world with a networth of $185 billion. Passing Jeff Bezos who has a networth of $184 billion.
Answer:
hey there!Step-by-step explanation:
it is elon muskhope it helps and have a great day!
A baker produces 500 loaves of bread. On Monday
Answers
Answer:
What is the question?
Step-by-step explanation:
Two circles of different sizes are drawn below. The diameter of the smaller circle is equal to the radius of the larger circle. The common tangent to both circles is 16cm in length and the distance between the centers is 20cm. Determine the diameter of the larger circle. Complete the diagram with appropriate information to support your solution.
I drew a diagram, and it's a rectangle and then a right triangle between the two circles like in my textbook. I tried solving using a right triangle in the diagram however I'm a bit confused and could use some help! By the way, I gave an example of what the diagram looks like below. Minus the numbers.

Answers
Answer:
Diameter of the larger circle = 48 cm
Step-by-step explanation:
From the figure attached,
Let the radius of the smaller circle is 'BC' = x.
Then the radius of the large circle 'AD' = 2x
Distance between the centers of the circles 'CD' = 20 cm
Length of the common tangent 'AB' = 16 cm
A construction has been done by drawing a perpendicular CE from C to AD by forming a rectangle ABCE.
Therefore, length of CE = length of AB = 16 units
By applying Pythagoras theorem in right triangle CED,
CD² = CE² + DE²
(20)² = (16)² + x²
400 = 256 + x²
x² = 400 - 256
x = √144
x = 12
Radius of the larger circle = 2x
= 2×12
= 24 cm
Therefore, diameter of the larger circle = 2 × radius
= 2 × 24
= 48 cm

You Use the distributive property to find the product 3/4 x 2 1/3
Answers
Answer:
1 1/4
Step-by-step explanation:
3/4 times 2 1/3 you make that an omproper fractions
The area of a sign in the shape of a square is 219 square inches. Which measurement is the closest to the side length of this sign in inches ?
A- 55 in.
B- 15 in
C- 7 in
D- 110in
Answers
Answer:
A.
Step-by-step explanation:
The closest value from the option given which matches the side length of the square is 15 inches
The Area of a square can be calculated using the relation :
Area of square = a² ; where, a = side lengthArea of the square shape = 219 in² ; we can express this as :
219 = a²
Take the square root of both sides
a = √219
a = 14.798
a = 15 inches (nearest whole number)
Therefore, the closest value of the side length is 15 inches.
Learn more :https://brainly.com/question/18112348
A train covers 165 km in 1.5 hours. How far will the train travel in 2.2 hours if it maintains the same speed?
Answers
Answer:
242
Step-by-step explanation:
165 ÷ 15 = 110
110 × 2.2 = 242
The distance that trains travel in 2.2 hours with the same ratio or same speed will be 242 km.
What are the ratio and proportion?The ratio is the division of the two numbers.
For example, a/b, where a will be the numerator and b will be the denominator.
Proportion is the relation of a variable with another. It could be direct or inverse.
As per the given,
A train covers 165 km in 1.5 hours.
The ratio of distance covered to the number of hours is 165/1.5.
Let's say in 2.2-hour train travel x number of km.
The ratio of distance covered to the number of hours x/2.2
Both ratios must be the same,
x/2.2 = 165/1.5
x = 110 x 2.2 = 242 km
Hence "The distance that trains travel in 2.2 hours with the same ratio or same speed will be 242 km".
For more information about ratios and proportions,
brainly.com/question/26974513
#SPJ2
The water level of certain body of water is changing at a rate of W(t) = 1/2cos(3 - t/2) inches per hour; where represents hours since 12ampart c: use your calculator to evaluate your integral from part b. explain the meaning of the answer in terms of the context. (3 points)
Answers
Since we don't have the function or limits of integration mentioned in part b of the question, we cannot evaluate the integral.
However, we can explain the meaning of the answer once we have the integral.
Assuming the integral represents the change in water level over a certain time interval, the answer to the integral would give us the total change in water level over that time interval. In other words, it would give us the net increase or decrease in the water level during that time period.
Since the rate of change of water level is given in inches per hour, the answer to the integral would also be in inches.
We can use this information to determine how much the water level has changed and whether it has increased or decreased during the given time interval.
To know more about function or limits of integration, refer here:
https://brainly.com/question/31956165
#SPJ11
I need to know the answer

Answers
Answer:
\(18+0.08B\geq 75\)
Step-by-step explanation:
there is a flat fee of $18
there is an incremental fee of $0.08 per ball which can be shown as \(0.08B\)
so the amount that Carolina will spend would be \(18+0.08B\)
the promotion only applies if Carolina spends $75 or more. the greater than or equal to symbol is \(\geq\)
so the inequality would be \(18+0.08B\geq 75\)
however, we could simplify this to \(0.08B\geq 57\) but it is not necessary
Consider the sequence defined by the recursive formulaA(N)=2A(N-z)+A(N-2) and starting with A(1) = 1, A(2) = 1a) Find the next 4 termsb) Find A(8)
Answers
a) The next four terms in the sequence defined by the recursive formula A(N) = 2A(N-1) + A(N-2), starting with A(1) = 1 and A(2) = 1, are: 1, 1, 3, 5.
b) To find A(8) in the sequence, we need to apply the recursive formula and work our way up from the initial values. Starting with A(1) = 1 and A(2) = 1, we can calculate A(3) using the formula: A(3) = 2A(3-1) + A(3-2) = 2A(2) + A(1) = 2(1) + 1 = 3. Continuing in a similar manner, we find A(4) = 2A(3) + A(2) = 2(3) + 1 = 7, A(5) = 2A(4) + A(3) = 2(7) + 3 = 17, A(6) = 2A(5) + A(4) = 2(17) + 7 = 41, A(7) = 2A(6) + A(5) = 2(41) + 17 = 99, and finally, A(8) = 2A(7) + A(6) = 2(99) + 41 = 239.
Using the recursive formula, we can generate the next four terms in the sequence as 1, 1, 3, 5. Additionally, calculating A(8) based on the recursive formula, we find that A(8) is equal to 239.
Learn more about recursive formula here: brainly.com/question/32794966
#SPJ11
Determine the equation of the circle graphed below
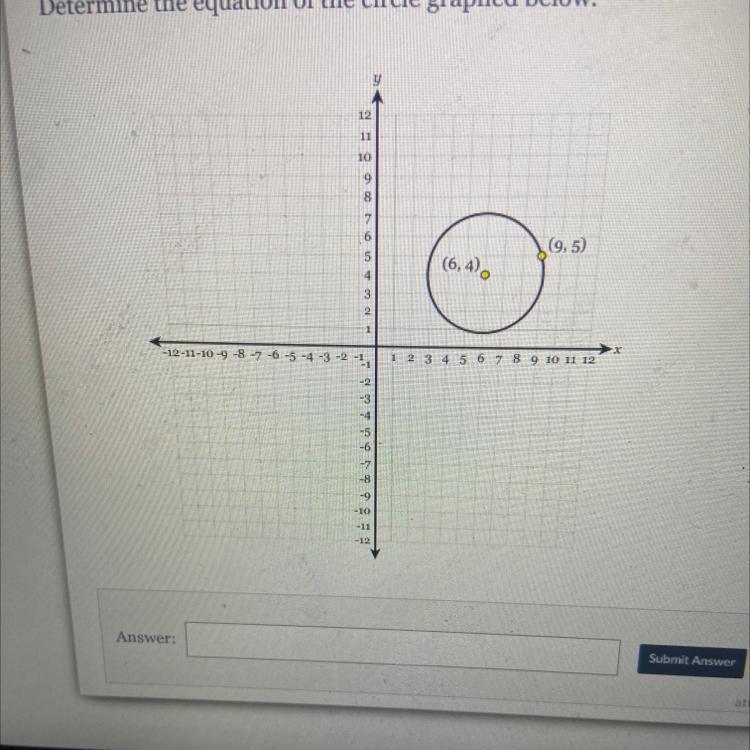
Answers
Answer:
(x-6)^2+(y-4)^2=10
Step-by-step explanation:
use the equation of a circle ( x- x coordinate of center)^2+(y-y coordinate of the center)^2=radius squared.
to solve for the radius, make a right triangle from the center to the point on the circle. 1^2+3^2=r^2. So r^2 is 10