one instructor believes that students take more than 2 classes per quarter on average. he randomly interviewed a class of 16 students and found out the mean number of classes per quarter is 2.3 classes and standard deviation of 0.8. assume alpha is 0.01. (b) what is the test statistic?
Answers
The test statistic for randomly interviewed a class of 16 students is given by 1.5.
The population mean is given by = µ = 2
The mean of the sample of class of 16 students is given by = 2.3
Length of size of sample is given by = (n) = 16
Standard deviation is given by = (s) = 0.8
So the test statistic for the test with randomly interviewed a class of 16 students is given by
= (mean of sample - µ)/(s/√n)
= (2.3 - 2)/(0.8/√16)
= 0.3/(0.8/4)
= 0.3/0.2
= 3/2
= 1.5
Hence the test statistic is given by 1.5.
To know more about test statistic here
https://brainly.com/question/15110538
#SPJ4
Related Questions
cindy baught 1/4 amount of ribbon and jacob baught 7/8 amount of ribbon how much ribbon does jacob have
Answers
Answer:
0.875
Step-by-step explanation:
Find the sum of the first 20 terms of the arithmetic series 53,46,39,32...
Answers
The answer can be found by assuming that we have an arithmetic progression that stars in 53 with a common difference of 7. The equation for the sum of an arithmetic progression up to n terms is given by:
\(s_n=\frac{n}{2}(2a+(n-1)d)\)Where, for this case
\(a=53\text{ , }n=20\text{ and }d=7\)So, applying the equation with these data, we obtain:
\(\begin{gathered} s_{20}=\frac{20}{2}(2(53)+(20-1)7) \\ s_{20}=10(106+19(7)) \\ s_{20}=10(106+19(7))=10(239)=2390 \end{gathered}\)Thus, the sum up to 20 terms of the given series is 2390.
there are 8 classes in the cafeteria. the ratio of the kindergarten classes to other classes was 1:3. how many classes were NOT from kindergarten
(6TH GRADE)
Answers
Answer:
6
Step-by-step explanation:
1+3=4
8÷4=2
Kindergarten = 2×1 =2
Other class = 2×3=6
Ratio of kindergarten classes to other classes is 2:6
Find the change in profit P for the given marginal. Assume that the number of units x increases by 5 from the specified value of x. (Round your answer to two decimal places.) Marginal Number of Units, x dP dx = 12.1 60 − 3 x x = 121
Answers
The change in profit (ΔP) when the number of units (Δx) increases by 5, based on the given marginal profit function, is -18331.50
To find the change in profit (ΔP) when the number of units (Δx) increases by 5.
we need to evaluate the marginal profit function and multiply it by Δx.
The marginal profit function is given by dP/dx = 12.1(60 - 3x).
We are given the value of x as 121, so we can substitute it into the marginal profit function to find the marginal profit at that point.
dP/dx = 12.1(60 - 3(121))
= 12.1(60 - 363)
= 12.1(-303)
= -3666.3
Now, we can calculate the change in profit (ΔP) by multiplying the marginal profit by Δx, which is 5 in this case.
ΔP = dP/dx×Δx
= -3666.3 × 5
= -18331.5
To learn more on Change in Profit click:
https://brainly.com/question/31420071
#SPJ4
Josiah plants vegetable seeds in rows. Each row has the same number of seeds in it. He plants more than one row of seeds. What could be the total number of seeds he plants?
Answers
The total number of seeds that Josiah would plant would be = nR×S
How to determine the total number of seeds that Josiah will plant?To determine the total number of seeds that Josiah will plant will be to add the seeds in the total number of rooms he planted.
Let each row be represented as = nR
Where n represents the number of rows planted by him.
Let the seed be represented as = S
The total number of seeds he planted = nR×S
Therefore, the total number of seeds that was planted Josiah would be = nR×S.
Learn more about multiplication here:
https://brainly.com/question/30340107
#SPJ1
Find the volume of the following figure using unit cubes.
A.23 units3
B.25 units3
C.27 units3
D.none above
Answers
Is (x + 6) a possible length of a rectangle if the area is x^2 + x − 30? Use an area model to prove your answer.
Can you use an area model to find the length and width of:
Answers
The answer is yes, (x + 6) is a possible length of a rectangle with area x² + x − 30.
To determine whether (x + 6) is a possible length of a rectangle with area x^² + x − 30, we can use an area model to visualize the situation.
First, we need to find the width of the rectangle.
The area of a rectangle is given by the formula A = lw,
where A is the area, l is the length, and w is the width.
We are given that the area is x² + x − 30, so we can set this equal to lw and solve for w:
x² + x − 30 = (x + 6)w
w = (x² + x − 30)/(x + 6)
The length of a rectangle must be greater than or equal to its width, so we need to check whether (x + 6) is greater than or equal to (x² + x − 30)/(x + 6). This simplifies to:
(x + 6) ≥ x² + x − 30
Expanding the left side and simplifying, we get:
x² + 12x + 36 ≥ x² + x − 30
11x ≥ -66
x ≥ -6
Since x must be a positive number (since we are dealing with lengths), we can conclude that (x + 6) is the possible length of the rectangle. Therefore, the answer is yes, (x + 6) is a possible length of a rectangle with an area x² + x − 30.
Learn more about Area here:
https://brainly.com/question/27683633
#SPJ1
if you could help me answer this I'll give you 30 points what is the answer for 2x + 3y + 4x + 553 equal to 360 + 22x + 10 y
Answers
ㅤㅤㅤ➠ 193 = 16x + 7 y
Ⲋⲟⳑⳙⲧⳕⲟⲛ :On solving the given equation :
ㅤㅤ➙ 2x + 3y + 4x + 553 = 360 + 22x +10y
ㅤㅤ➙ 6x + 3y + 553 = 360 + 22x + 10y
ㅤㅤ➙ 553 - 360 = 22x - 6x + 10y - 3y
ㅤㅤ➙ 193 = 16x + 7y
ㅤ ㅤㅤㅤ~Hence, Solved !
\( \rule{300pt}{1pt}\)
Suppose that the time it takes for juniors in high school to complete the SAT exam is uniformly distributed with a minimum of 126 minutes and a maximum of 183 minutes. What is the probability that a high school junior will take between 173 and 190 minutes to complete the SAT exam? 00.1754 0.2982 0.1149
Answers
The probability that a high school junior will take between 173 and 190 minutes to complete the SAT exam is 0.2982.
The given problem involves a uniform distribution where the time it takes for high school juniors to complete the SAT exam is uniformly distributed between 126 and 183 minutes. We need to find the probability that a junior will take between 173 and 190 minutes to complete the exam.
To solve this problem, we need to find the area under the probability density function (PDF) curve for the given range of values. Since the distribution is uniform, the PDF is a horizontal line with a height of 1/(183-126) = 1/57, and the area under the curve for the given range can be found by multiplying the height of the PDF with the width of the range:
Probability = height * width = (1/57) * (190-173) = 0.2982
Therefore, the probability that a high school junior will take between 173 and 190 minutes to complete the SAT exam is 0.2982.
For more questions like Probability click the link below:
https://brainly.com/question/30034780
#SPJ11
simple random sampling is least likely to . group of answer choices ensure that each individual has an equal chance of selection remove all bias and discrimination from the selection process guarantee that every individual in the population has a chance of being selected guarantee that the sample will be representative and unbiased
Answers
Simple random sampling is least likely to guarantee that the sample will be representative and unbiased.
Simple random sampling is a technique that is used in research in which each member of the population is given an equal chance of being selected. It's the most straightforward and most basic technique for selecting a random sample. Sampling is a statistical method used to gather and analyze data from a subset of a population to provide estimates, hypotheses, or projections of the whole population. A sample is a subset of a population chosen to represent it since it would be difficult to collect data on the entire population. A sample is thus chosen using sampling techniques, which are statistical approaches for choosing representative samples that guarantee unbiased and precise results.
Biased sampling is a type of sampling method that can lead to misleading or erroneous conclusions. It happens when the sample that has been chosen is not representative of the population it is meant to represent. Biased sampling occurs when the selection method used to obtain the sample is flawed.
Learn more about Simple random sampling visit:
brainly.com/question/30403914
#SPJ11
in a certain industrial facility, accidents occur infrequently. it is known that the probability of an accident on any given day is 0.005 and accidents are independent of each other. what is the probability that in any given period of 400 days there will be an accident on one day? use binomial approximation to poisson distribution.
Answers
The probability that in any given period of 400 days there will be an accident on one day is 0.2707
Given that;
In a certain industrial facility, accidents occur infrequently. it is known that the probability of an accident on any given day is 0.005 and accidents are independent of each other.
n = 400, p = 0.005
Poisson distribution is given by;
e P(x) = (λˣe^λ ) / x!
Here, Mean(λ) = np
= 400*0.005
=2
The probability that there will be an accident on one day;
(P(x = 1)) = (λxe-λ)/x!
= 2*e-2
= 0.2707
The probability that in any given period of 400 days there will be an accident on one day is 0.2707
To learn more about probability click here:
brainly.com/question/11234923
#SPJ4
I need to step-by- step ways on how this is or isn’t a solvable equation. Please HELP
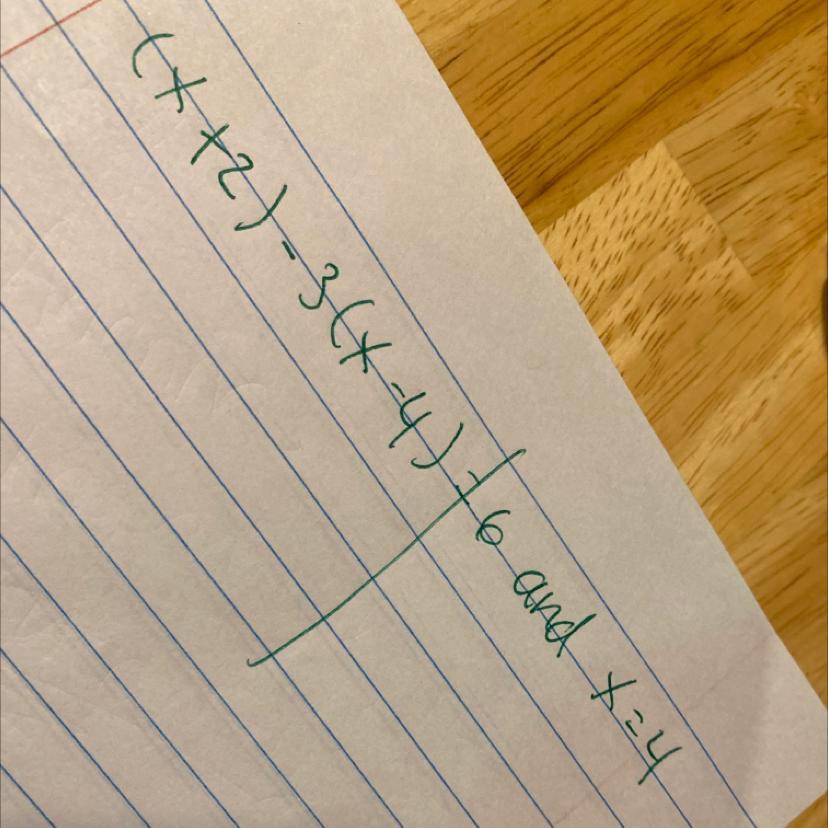
Answers
Answer:
it is a solvable equation
Step-by-step explanation:
(x + 2) -3(x - 4) = 6 and x = 4
if x = 4 then fill in all the x's with 4 so the equation is now:
(4 + 2) -3(4 - 4) = 6
Use PEMDAS
Now the equation is 6 - 3(0) = 6
-3 times 0 is 0 so it's 6-0=6
6 = 6
when you put the 4 in for the x variables and the numbers on both sides of the equal sign are the same it makes the equation true
hope this makes sense and hope it helps :)
solve: 5/3x+1/3x=21 2/3+7/3
Answers
Answer: x = 12
Step-by-step explanation:
1) Convert mixed numbers into improper fractions: \(21\frac{2}{3} =\frac{21*3+2}{3} =\frac{65}{3}\)
New equation written as: \(\frac{5}{3}x+\frac{1}{3}x=\frac{65}{3}+\frac{7}{3}\)
2) \(\frac{5}{3}x+\frac{1}{3}x=\frac{65+7}{3}\)
3) Add the numbers 65 + 7 = 72: \(\frac{5}{3}x+\frac{1}{3}x=\frac{72}{3}\)
4) Divide 72/3 = 24: \(\frac{5}{3}x+\frac{1}{3}x=24\)
5) Multiply both sides by 3. Note: * = multiply: \(\frac{5}{3}x*3+\frac{1}{3}x*3=24*3\)
6) Simplify: 6x = 72
7) Divide both sides by 6: \(\frac{6x}{6}=\frac{72}{6}\)
x = 12
Collin wanted to purchase a truck with four-wheel drive, a CD player, and a GPS. Since he had saved just enough for the base model without these features, he decided to buy the base model and forego getting a car loan. Which biblical principle did he follow?
Answers
Colin has lived by the biblical ideal of avoiding debt, purchasing the lowest item, repaying a loan, and being financially honest.
A car loan is what?With an auto loan, you may borrow money from a bank and use it to purchase a vehicle. The loan must be repaid with interest over a defined period of time in fixed instalments from you.
Lenders will aim for a credit score of at least 750 when you apply for a vehicle loan.
The additional costs won't dramatically raise the price of the automobile because of the low interest rate. The periodic payments won't put undue strain on your current or next finances.
Read more on car loan here:
https://brainly.com/question/25696681
#SPJ4
Round to the hundredths place.
$4.56 for 18 cookies
Answers
HELP PLEASE! What are the coordinates of the image of ΔABC after a dilation with center (0, 0) and a scale factor of 1/2?
? Drag numbers to complete the coordinates. Numbers may be used once, more than once, or not at all.
Answers
The coordinates of the image of triangle ABC after a dilation with center (0, 0) and a scale factor of 1/2 are:
A'(x1', y1') = (1/2)x1, (1/2)y1)
B'(x2', y2') = (1/2)x2, (1/2)y2)
C'(x3', y3') = (1/2)x3, (1/2)y3)
How do we calculate?
We multiply the coordinates of each vertex of the original triangle by the scale factor, in order to find the coordinates of the image.
We assume that the coordinates of the vertices of triangle ABC are A(x1, y1), B(x2, y2), and C(x3, y3).
After the dilation with a scale factor of 1/2, the new coordinates of these vertices will be:
A'(x1', y1') = (1/2)x1, (1/2)y1)
B'(x2', y2') = (1/2)x2, (1/2)y2)
C'(x3', y3') = (1/2)x3, (1/2)y3)
Learn more about coordinates at:
https://brainly.com/question/30227780
#SPJ1
If there are two 6-sided dice, one green and one yellow, and each are fair dice, what is the probability that, when rolling both dice, the sum is 7 or 11
Answers
The probability of rolling a sum of 7 or 11 when rolling both dice is approximately 0.111 or 11.1%.
The probability of rolling a sum of 7 or 11 with two 6-sided dice, we can first calculate the total number of possible outcomes when rolling both dice.
Each die has six possible outcomes, so the total number of possible outcomes when rolling both dice is 6 x 6 = 36.
To count the number of outcomes where the sum is 7 or 11.
There are two ways to roll a sum of 7:
(1, 6) and (6, 1).
There are also two ways to roll a sum of 11:
(5, 6) and (6, 5).
There are four possible outcomes that result in a sum of 7 or 11.
Thus, the probability of rolling a sum of 7 or 11 is:
4/36
= 1/9
≈ 0.111
The total number of outcomes while rolling both dice in order to determine the likelihood of rolling a sum of 7 or 11 using two 6-sided dice.
Rolling both dice, there are a total of six possible results for each die, for a total of 36 options in all.
to determine how many possibilities there are where the total is 7 or 11. Rolling a 7, there are two possible outcomes: (1, 6) and (6, 1).
Additionally, there are two methods to roll an 11: (5, 6) and (6, 5).
There are four events that can lead to a total of either 7 or 11.
As a result, the likelihood of rolling a 7 or 11 is 4/36 = 1/9 0.111.
For similar questions on probability
https://brainly.com/question/30700350
#SPJ11
Solve for d:
d over 12 = 5.76
1. d = 17.76
2. d = 69.12
3. d = 0.48
Answers
d over 12 = 5.76
d = 69.12
PLEASE HELP MEEE
1. Megan works at Amazon’s warehouse. She is responsible for packing boxes into trucks for shipment. Each truck can hold 22 boxes, and she needs to ship 539 boxes total.
a. Megan thinks she can fit all 539 boxes into 24 trucks. Is her estimate correct? Why or why not?
Answers
Answer:
Her estimate is wrong. If she can only fit 22 boxes in a truck and she is only using 24 trucks, she have 11 boxes left.
Step-by-step explanation:
24x22=528
539-528=11
Her estimate is wrong. If she can only fit 22 boxes in a truck and she is only using 24 trucks, she has 11 boxes left.
What is an expression?Expression in maths is defined as the collection of numbers variables and functions by using signs like addition, subtraction, multiplication, and division.
Numbers (constants), variables, operations, functions, brackets, punctuation, and grouping can all be represented by mathematical symbols, which can also be used to indicate the logical syntax's order of operations and other features.
Given that each truck can hold 22 boxes, she needs to ship 539 boxes in total. Megan thinks she can fit all 539 boxes into 24 trucks.
For 24 trucks the number of the boxes will be calculated as below:-
24x22=528
The remaining boxes are:-
539-528=11
Therefore, the estimate is wrong. If she can only fit 22 boxes in a truck and she is only using 24 trucks, she has 11 boxes left.
To know more about an expression follow
https://brainly.com/question/24844671
#SPJ2
differences on the dependent measure between the levels of one variable within one level of another variable are known as
Answers
The differences in dependent measures between levels are the main effects
The differences in the dependent measure between the levels of one variable within one level of another variable are known as simple main effects. These effects can be tested statistically to determine if they are statistically significant, which would indicate that the difference between the levels of the first variable is meaningful within the context of the second variable.
One independent variable having a certain degree of another independent variable is the simple main effect of a factorial experiment. The levels of the other independent variable are averaged over the primary effect of one independent variable. Results for a straightforward main effect are examined as though every level of the other independent variables had its own experiment.
Read more about the main effects on:
https://brainly.com/question/29613380
#SPJ4
find the nth term of this quadratic sequence 4, 7, 12, 19, 28, . ..
Answers
Answer:
an = a1 + (n-1)d
Untuk barisan ini, kita dapat menentukan a1 = 4 dan d = 3, karena selisih antar suku bertambah 3. Jadi, rumusnya menjadi:
an = 4 + (n-1)3
a5 = 4 + (5-1)3
a5 = 4 + 12
a5 = 16
Jadi, suku ke-5 dari barisan ini adalah 16.
Untuk Konsultasi Tugas Lainnya: WA 0813-7200-6413
The figure is formed from rectangles. Find the total area. The diagram is not to scale.

Answers
Answer:
68
Step-by-step explanation:
look at the picture, the answer is in there along with the explanation

find the equation of the plane tangent to the surface z = 3x2 3y3 at (2, 1, 15).
Answers
The equation of the plane tangent to the surface z = 3x^2 - 3y^3 at the point (2, 1, 15) is 12x - 9y + z - 30 = 0.
To find the equation of the plane tangent to the surface z = 3x^2 - 3y^3 at the point (2, 1, 15), we can use the concept of partial derivatives and the equation of a plane.
1. Compute the partial derivatives of the surface equation with respect to x and y. Taking the partial derivative with respect to x treats y as a constant, and vice versa. For the given equation, we have:
∂z/∂x = 6x
∂z/∂y = -9y^2
2. Substitute the coordinates of the point (2, 1, 15) into the partial derivatives:
∂z/∂x = 6(2) = 12
∂z/∂y = -9(1)^2 = -9
3. The normal vector of the plane is obtained by taking the coefficients of the partial derivatives:
Normal vector = (12, -9, 1)
4. Now, we have the normal vector and a point on the plane (2, 1, 15). Using the equation of a plane, which is of the form Ax + By + Cz = D, we can substitute the values:
12(x - 2) - 9(y - 1) + (z - 15) = 0
12x - 24 - 9y + 9 + z - 15 = 0
12x - 9y + z - 30 = 0
Therefore, the equation of the plane tangent to the surface z = 3x^2 - 3y^3 at the point (2, 1, 15) is 12x - 9y + z - 30 = 0.
The equation represents a plane that is tangent to the given surface at the specified point. The coefficients in the equation correspond to the components of the normal vector, and the constant term is determined by evaluating the equation at the given point.
To know more about the equation of a plane refer here:
https://brainly.com/question/28456872
#SPJ11
Find a Taylor series polynomial of degree at least four which is a solution of the boundary value problem that follows. f'(x) = (-5+5xJy and f(0) = -3 Write out the first five terms from the series.
Answers
The Taylor series polynomial of degree four or higher that satisfies the given boundary value problem is f(x) = -3 - 5x + (5/2)x² - (5/6)x³ + (5/24)x⁴. The first five terms of the series are -3, -5x, (5/2)x², -(5/6)x³, and (5/24)x⁴.
To find the Taylor series polynomial, we'll start by calculating the derivatives of f(x). The first derivative of f(x) is f'(x) = -5 + 5x. Now, we need to find the higher derivatives of f(x). Differentiating again, we get f''(x) = 5, f'''(x) = 0, and f''''(x) = 0. Since all higher derivatives are zero, we can conclude that the Taylor series polynomial of degree four or higher is given by:
f(x) = f(0) + f'(0)x + (f''(0)/2!)x² + (f'''(0)/3!)x³ + (f''''(0)/4!)x⁴
Substituting the initial condition f(0) = -3 and the derivatives f'(0) = -5, f''(0) = 5, f'''(0) = 0, and f''''(0) = 0 into the equation, we obtain:
f(x) = -3 - 5x + (5/2)x² - (5/6)x³ + (5/24)x⁴
The first five terms from the series are -3, -5x, (5/2)x², -(5/6)x³, and (5/24)x⁴. These terms represent an approximation of the solution to the given boundary value problem.
Learn more about derivative here: https://brainly.com/question/29144258
#SPJ11
How many solutions does this equation have?
4v = 6v − 2v
no solution
one solution
Infinitely many solutions
Answers
Answer:
Infinitely many solutions.
Step-by-step explanation:
4v=6v-2v Combine like terms.
4v=4v These are already equal without diving, so there are infinitely many solutions.
Hope this helps and have a nice day ❤
Answer:
Infinitely many solutions
Step-by-step explanation:
4v=6v-2v
4v=4v
when two expressions on opposite sides of an equals sign are equivalent, that means there are infinitely many solutions
P.S. Please mark me Brainliest if it is correct.
I need help with this problem Try not to answer this try to give me steps

Answers
Answer:
Step-by-step explanation:
80 inches = 6 feet and 8 inches
A new type of pump can drain a certain pool in 9 hours. An older pump can drain the pool in 11 hours. How long will ittake both pumps working together to drain the pool?
Answers
Given:
A new type of pump can drain a certain pool in 9 hours.
An older pump can drain the pool in 11 hours.
To find:
The required time when both pumps work together to drain the pool.
Explanation:
One hour's work of the new pipe is,
\(\frac{1}{9}\)One hour's work of the old pipe is,
\(\frac{1}{11}\)So, one hour's work of both the pipe together is,
\(\begin{gathered} \frac{1}{9}+\frac{1}{11}=\frac{11+9}{9(11)} \\ =\frac{20}{99} \end{gathered}\)Therefore, the time taken to drain the pool for both the pipes together is,
\(\begin{gathered} \frac{99}{20}hours \\ (or) \\ 4\text{ }hours\text{ 57minutes} \end{gathered}\)Final answer:
The required time to drain the pool for both the pipes together is 4 hours 57 minutes.
remove the largest common factor. check your answer by multiplication.
21x^5+14x^3-35
Factor out the greatest common factor.
Answers
Therefore :
7(3x^5+2x^3-5) is the correct answer
We can’t factor x because 35 does not have any x
Most common is 7
So
7(3x⁵+2x³-5)Use distributive law to verify
21x⁵+14x³-35Verified
GCF is 7
An average football field has the dimensions of 160 ft by 360 ft. If the expressions to find these dimensions were (3x + 7) and (7x + 3) what value of would give the dimensions of the football field ?
Answers
Answer:
47
Step-by-step explanation:
Because that's what it is i might be wrong tho lol
HOW I GOT THE ANSWER:I think it the best because it make most sense and it seems correct hope this helps u it took long to get
PLEASE HELP QUICK, WILL MARK BRAINLIEST!
Solve for x: −6 < x − 1 < 9
5 < x < 10
−5 < x < 10
−5 > x > 10
5 > x > −10
Answers
Answer:
−5 < x < 10
Step-by-step explanation:
−6 < x − 1 < 9
Add 1 to all sides
−6+1 < x − 1+1 < 9+1
−5 < x < 10
Answer:
B
Step-by-step explanation:
Add one to everything
-5 < x < 10
Best of Luck!