Answers
Related Questions
Which is the first step in simplifying the expression 2(x 3) 5? 2x 2(3) 5 2x 3 5 2 x 3 5 2(5) x 3
Answers
The first step in simplifying the expression 2(x+3)+5 = 2x+2(3)+5.
Given expression in 'x' is 2(x+3)+5.
In order to simplify this expression, we need to expand it by opening the parenthesis. That is, we need to distribute 2 over (x+3) first. For this we multiply 2 into (x+3) and then only we can add 5 to it.
So, 2(x+3) +5 = 2x + 2(3) +5
= 2x +6 +5
= 2x+11
Learn more about simplifying expressions at https://brainly.com/question/723406
#SPJ4
which sum or difference is modeled by the algebraic tiles?

Answers
Answer:
C
Step-by-step explanation:
What is the DOMAIN of the following relation? {(-5,4), (-4,2), (0,2), (1,3), (2,4)}
Answers
Answer:
{-5, -4, 0, 1, 2}
Step-by-step explanation:
The domain is the inputs or the x values
{-5, -4, 0, 1, 2}
Answer:
See below.
Step-by-step explanation:
The domain of a relation is all of the x-coordinates.
So we have the relation:
{(-5,4), (-4,2), (0,2), (1,3), (2,4)}
This means that the domain will be the following values:
{(-5,4), (-4,2), (0,2), (1,3), (2,4)}
So, our domain is:
\(\{-5, -4, 0, 1, 2\}\)
Note that the domain is in ascending order.
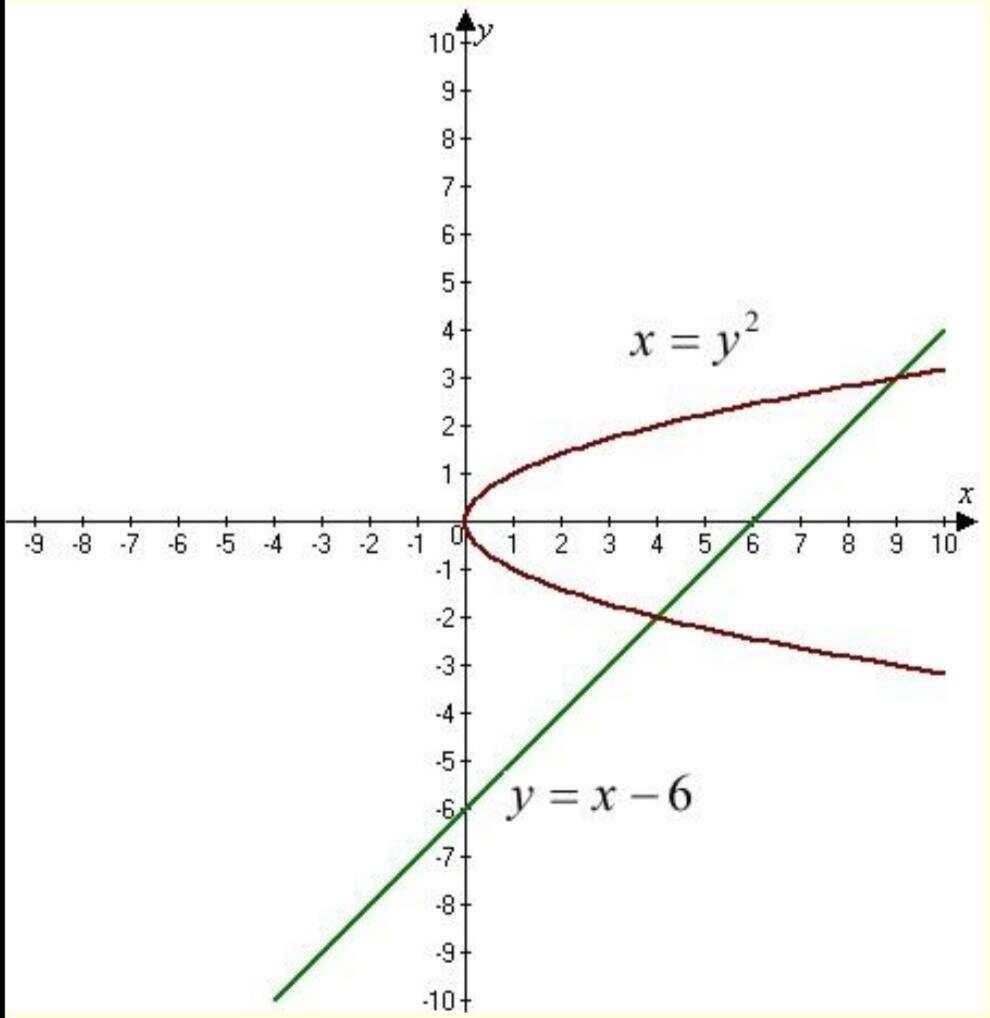
Set up iterated integrals for both orders of integration. Then evaluate the double integral using the easier order.
y dA, D is bounded by y = x − 6; x = y2
D
Answers
The value of the double integral using the easier order, ydA bounded by y = x − 6; x = y² is 125/12.
The double integral, indicated by ', is mostly used to calculate the surface area of a two-dimensional figure. By using double integration, we may quickly determine the area of a rectangular region. If we understand simple integration, we can easily tackle double integration difficulties. Hence, first and foremost, we will go over some fundamental integration guidelines.
Given, the double integral ∫∫yA and the region y = x-6 and x = y²
y = x-6
x = y²
y² = y +6
y² - y - 6 = 0
y² - 3y +2y - 6 = 0
(y-3) (y+2) = 0
y = 3 and y = -2
\(\int\int\limits_\triangle {y} \, dA\\ \\\)
= \(\int\limits^3_2 {y(y+6-y^2)} \, dx \\\\\int\limits^3_2 {(y^2+6y-y^3)} \, dx \\\\(\frac{y^3}{3} + 3y^2-\frac{y^4}{4} )_-_2^3\\\\\frac{63}{4} -\frac{16}{3} \\\\\frac{125}{12}\)
The value for the double integral is 125/12.
Integration is an important aspect of calculus, and there are many different forms of integrations, such as basic integration, double integration, and triple integration. We often utilise integral calculus to determine the area and volume on a very big scale that simple formulae or calculations cannot.
Learn more about Double Integral:
https://brainly.com/question/19053586
#SPJ4
The length of a
rectangular garden is
three feet less than twice
its width. If the perimeter
of the garden is 42 feet,
what is its length?
Systems word problems
Answers
Answer:
The length will be 13 feet.
Step-by-step explanation:
Let the width be represented by w.
Based on the information given, the length will be:
= (2 × w) - 3
= 2w - 3
Therefore, the perimeter will be:
2(w) + 2(2w - 3) = 42
2w + 4w - 6 = 42
6w = 42 + 6
6w = 48
w = 48/6
w = 8
Therefore, the length will be:
L = 2w - 3
L = 2(8) - 3
L = 16 - 3
L = 13 feet
The length is 13 feet.
Answer:
The length will be 13 feet.
Step-by-step explanation:
Let the width be represented by w.
Based on the information given, the length will be:
= (2 × w) - 3
= 2w - 3
Therefore, the perimeter will be:
2(w) + 2(2w - 3) = 42
2w + 4w - 6 = 42
6w = 42 + 6
6w = 48
w = 48/6
w = 8
Therefore, the length will be:
L = 2w - 3
L = 2(8) - 3
L = 16 - 3
L = 13 feet
The length is 13 feet.
you toss a coin and spin a spinner. the spinner has four equal sections that are numbered from 1 to 4. find the probability of getting tails and spinning a 4.
also, make a table to show the sample space and find the number of outcomes.
Answers
Answer:
12.5% or .125
Step-by-step explanation:
4 options for the spinner all with equal probability, chance of spinning any number is 1/4; 2 options for the coin of equal probability so any side has a probability of 1/2. 1/4*1/2=1/8 chance; this is equal to 12.5% or .125.
there are 8 possible outcomes; 4 with spinner, 2 with coin, 4*2=8.

is i get one point each 5 minutes how long until i get 50 points?
Answers
Answer:
250 minutes.
Step-by-step explanation:
1/5=50/x
cross product
5*50=1*x
250=x
The coordinates of Point P are
.
The coordinates of Point T are
.
Point
is located at (1
2
, −21
2
).
Answers
The minimum distance between Point P and Point T is √(97)
The coordinates of point P are (-2, 6).
The coordinates of point T are (6, -2).
Point S is located at (1/2, -21/2).
To find the coordinates of Point P and Point T, use the distance formula.
The distance formula for two points, A(x1, y1) and B(x2, y2) is given as:
Distance, AB = √[ (x2 - x1)² + (y2 - y1)² ]
Now, substituting the coordinates of Point P and Point T into the distance formula gives
:Distance, PT = √[ (xT - xP)² + (yT - yP)² ]... Equation (1)
Let d be the distance PT. Using the coordinates of Point S, we can express the distance PT as the sum of two smaller distances, PS and ST.
Distance, PT = PS + ST... Equation (2)
Substituting the coordinates of Point P and Point S into Equation (2),
we get: Distance, PS = √[ (1/2 - (-2))² + (-21/2 - 6)² ] = √(97)Distance,
ST = √[ (6 - 1/2)² + (-2 - (-21/2))² ] = √(97)
Therefore, d = PS + ST = 2 √(97).
By Pythagoras theorem, if x is the distance from Point P to Point S along the x-axis, then:
Distance, PS = |x - 1/2|And, if y is the distance from Point P to Point S along the y-axis, then:
Distance, PS = |y - (-21/2)|
Thus, the distance PT can be expressed in terms of x and y as follows: d = |x - 1/2| + |y + 21/2|... Equation (3)
Now, we need to find the minimum value of d. We can do this by first minimizing the first term |x - 1/2| and then minimizing the second term |y + 21/2|.
To minimize the first term |x - 1/2|, x should be as close as possible to 1/2.
Therefore, let x = 1/2.
Then, substituting x = 1/2 into Equation (1)
gives:|y - (-21/2)| = 2 √(97)Solving for y,
we get: y = -21/2 ± 2 √(97)
Substituting y = -21/2 + 2 √(97) into Equation (3),
we get: d = √(97)
To know more about distance visit:
https://brainly.com/question/13034462
#SPJ11
which percent is equivalent to 12/25
Answers
Answer:
48 percent
Step-by-step explanation:
12 divided by 25 gets you .48 which can be expressed as 48%
Answer:
48%
Step-by-step explanation:
1. Change 12/25 to a fraction with a denominator of 100
25 x 4=100
12 x 4=48
2. Find the percent
48/100= 48%
Martin is using unit cubes to build a tower in the shape of a right rectangular prism. A description of the tower is listed below. Bottom layer is made of 16 unit cubes bottom layer is in the shape of a square prism 9 more equal layers of unit cubes are added on top of the bottom layer What is the total volume, in cubic units, of the completed tower?
Answers
Answer:
160 sq units
Step-by-step explanation: multiple 16 times 10 and you get that
Answer the questions below about the quadratic function.
f(x) = -3x² – 30x – 71
Does the function have a minimum or maximum value?
Where does the minimum or maximum value occur?
What is the function's minimum or maximum value?
Answers
Answer:
there is a maximum value
the maximum occurs at the point (-5, 4)
The maximum value is 4
Step-by-step explanation:
When graphed the function is a parabola that opens down because of the -3
Therefore, there is a maximum value and it is the k value of the vertex (h, k)
h = - b/2a a = -3 b = -30
= -(-30)/2(- 3) = 30/-6 = -5
k = -3\((-5)^{2}\) -30(-5) - 71
= -3(25) + 150 - 71
= -75 + 150 - 71
= 4
The vertex is (-5, 4) So the maximum occurs at the point (-5, 4)
The maximum value is 4
Please please help I would give anything just please help and check if I’m right it’s algebra 2

Answers
Answer:
In no 4 you have written option B but it should be option C. All of the other except it is correct.
what will a 20kg bag weigh if it's mass is increased by 40%
Answers
Answer:
28
Step-by-step explanation:
20 x 1.4 = 28
An increase of 40% is the same as multiplying by the multiplier 1.4
FOR 50 POINTS AND BRAINLIEST!!
Directions: Determine the domain, range, and tell if the graph is a function or not

Answers
Answer:
Domain: (-∞, 1) ∪ (1, ∞)
Range: (-∞, -2) ∪ (2, ∞)
Yes, it's a function.
Step-by-step explanation:
Information
An open circle indicates the value is not included in the interval.A closed circle indicates the value is included in the interval.An arrow shows that the function continues indefinitely in that direction.DomainThe domain of a function is the set of all possible input values (x-values).
From inspection of the given graph:
There is an open circle at (1, 2) and (1, -2).The arrows show that the x-values continue indefinitely towards -∞ and ∞.Therefore, the domain is all values of x except x = 1:
Interval notation: (-∞, 1) ∪ (1, ∞)RangeThe range of a function is the set of all possible output values (y-values).
From inspection of the given graph:
There is an open circle at (1, 2) and (1, -2) so the range does not include any values of y between (and including) -2 and 2.The arrows show that the y-values continue indefinitely towards -∞ and ∞.Therefore, the range is all values of y except between -2 and 2:
Interval notation: (-∞, -2) ∪ (2, ∞)FunctionsA function is a special type of relationship where each input (x-value) is related to exactly one output (y-value).
From inspection of the graph, as the two points where x = 1 are not included, each value in the range (y-values) corresponds to exactly one value in the domain, and so it is a one-to-one function.
List three ordered pairs that represent solutions to the equation x - y = 5.
Answers
Answer:
2x-y=3
Step-by-step explanation:
five people plan to meet after school, and if they all show up, there will be one group of five people. however, if only two of them show up, in how many ways is this possible?
Answers
If only two out of the five people show up for the meeting, it is possible in 10 different ways.
If five people plan to meet after school and there will be one group of five people if they all show up, but only two people show up, we need to determine the number of ways this can happen.
To find the number of ways two people can show up out of the five, we can use combinations. In a combination, the order of selection does not matter.
The number of ways to choose two people out of five can be calculated using the formula for combinations, denoted as "nCr", where n is the total number of people and r is the number of people we want to choose.
In this case, we want to choose 2 people out of 5, so the calculation would be:
5C2 = (5!)/(2!(5-2)!) = (5!)/(2!3!) = (5 \(\times\) 4)/(2 \(\times\) 1) = 10
Therefore, there are 10 possible ways for two people to show up out of the five if all of them plan to meet after school.
These 10 possibilities could be different combinations of any two individuals out of the five.
To determine the specific combinations, you can list all the pairs or use a combination formula calculator.
It's important to note that the order in which the two people show up does not matter, as long as they are two out of the five originally planning to meet.
For similar question on combinations.
https://brainly.com/question/4658834
#SPJ8
A company with 17 employees gives each employee a bonus of $459. How much does the company spend on bonuses?
O $27
O $476
O $4,590
O $7,803
Answers
Answer: Option D: $7803
Step-by-step explanation:
just multiply 459 by 17 and you get your answer.
help me please, im struggling

Answers
Answer:
8.57
Step-by-step explanation:
Hope it helps.......
Answer:
x = 8.57
Step-by-step explanation:
Hi! So first of all, I attached an image that has my best visual explanation of how the 2 triangles overlap but are indeed two separate triangles!
Since the question states that the triangles are similar, that basically means in simple terms that their sides are proportional or, are all based on the same fraction (proportion)! (An example of this is the triangles with the sides that correlate [in this problem, 11 to 7] would be 1 to 2, 3 to 6, and 4 to 8)
Now, we have to figure out which of our sides correspond, the first one being 11 to 7, and the second one being x + 15 (which I got by adding the small portion I have from the big triangle, and the portion I have from the smaller triangle together because the small triangle is inside of the big one!) to 15.
Next, I have to find out what x is based on the fraction (proportion) I already have which is 11 to 7 or \(\frac{11}{7}\). Then, I compare this by setting this proportion equal to are other proportion with x in it!
\(\frac{11}{7} = \frac{x+15}{15}\)
Finally, I have to get x by itself to find what it is equal to, which you can see in the image! (If you have a number in the denominator and a variable in the numerator, and a regular number or fraction without a variable on the other side, you can just multiply both sides by the number in the denominator to cancel out the bottom of said variable fraction!)
So! X is equal to 8.57!
Hope this Helps! :)
Have any questions? Ask below in the comments and I will try my best to answer.
-SGO

10 Points Given
The treasure chest below is a prism with a
cross-section formed of a rectangle and a
semicircle.
Work out the volume of the treasure chest.
If your answer is a decimal, give it to 2 d.p.
0.7 m
0.8 m
1.7 m
Not drawn accurately

Answers
The volume of the treasure chest is approximately 1.3785 cubic meters.
To calculate the volume of the treasure chest, we need to determine the area of the cross-section and multiply it by the depth of the prism.
The cross-section of the treasure chest is formed by a rectangle and a semicircle. Let's break down the calculations step by step:
Rectangle: The length of the rectangle is given as 0.7 m and the width as 0.8 m. Therefore, the area of the rectangle is calculated as length multiplied by width: 0.7 m \(\times\) 0.8 m = 0.56 square meters.
Semicircle: The semicircle has a diameter equal to the width of the rectangle, which is 0.8 m. The radius of the semicircle is half the diameter, so the radius is 0.8 m / 2 = 0.4 m. The area of a semicircle is calculated as half the area of a full circle, so the area of the semicircle is \(1/2 \times \pi \times (0.4 m)^2\) = 0.2513 square meters (rounded to 4 decimal places).
Total cross-sectional area: To find the total area of the cross-section, we add the areas of the rectangle and the semicircle: 0.56 square meters + 0.2513 square meters = 0.8113 square meters (rounded to 4 decimal places).
Depth: The depth of the prism is given as 1.7 m.
Volume: To calculate the volume of the prism, we multiply the cross-sectional area by the depth: 0.8113 square meters \(\times\) 1.7 m = 1.3785 cubic meters (rounded to 4 decimal places).
For more such questions on volume
https://brainly.com/question/463363
#SPJ8
Confused please help Brainliest will be rewarded!!!!!!!
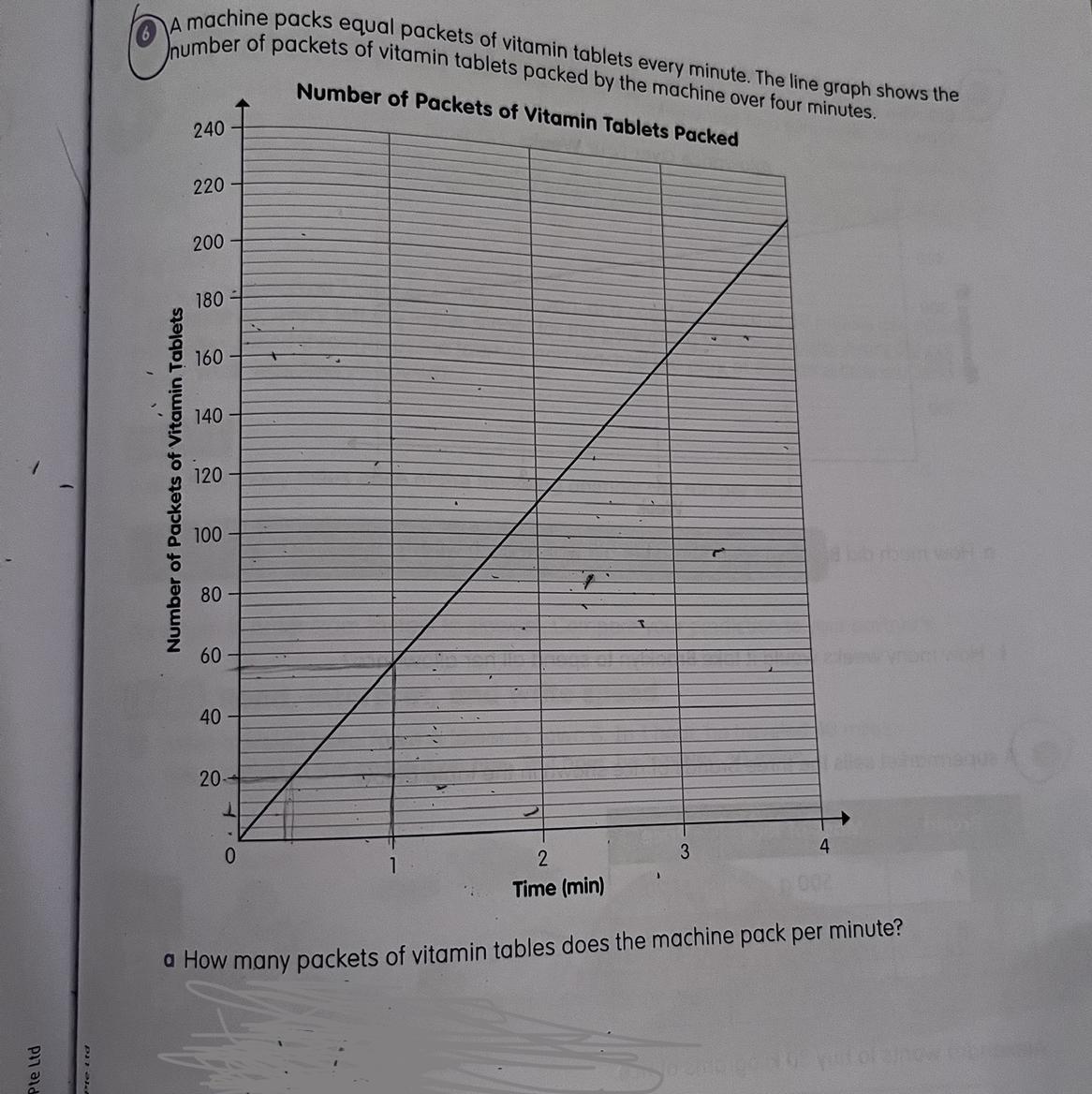
Answers
Answer:
56
Step-by-step explanation:
Take two points as far apart on the line (for maximum accuracy), preferably on grid points, and then calculate the vertical difference divided by the horizontal difference.
So (4, 224) and (0,0) are on the line, and (224-0)/(4-0) = 56
Which of the following is equivalent to :
19 9
∑ (n² - 6n + 9) - ∑ (n² - 6n + 9) ?
n=1 n=1
19
A.) ∑ (n-3)²
n=10
19
B.) ∑ (n-3)²
n=8
19
C.) ∑ (n+3)²
n=10
19
D.) ∑ (n+3)²
n=8
Answers
Step-by-step explanation:
n=24
n=39 to limt thier balance
n=45
n=7
The equivalent expression for ∑₁₀¹⁹(n² - 6n + 9) is A. ∑₁₀¹⁹(n - 3)²
How to find the equivalent expression?Since we have the expression ∑₁₀¹⁹(n² - 6n + 9) we desire to find the equivalent expression, we proceed as follow
Since we have the expression ∑₁₀¹⁹(n² - 6n + 9), we simplify the expression in the bracket by factorizing it. so, we have that
∑₁₀¹⁹(n² - 6n + 9) = ∑₁₀¹⁹(n² - 3n - 3n + 9)
Now, grouping like terms together, we have that
∑₁₀¹⁹(n² - 3n - 3n + 9) = ∑₁₀¹⁹[n(n - 3) - 3(n - 3)]
Factorizing the brackets, we have that
∑₁₀¹⁹[n(n - 3) - 3(n - 3)] = ∑₁₀¹⁹[(n - 3)(n - 3)]
Since the term is repeated, so we square it. Thus, we have that
= ∑₁₀¹⁹(n - 3)²
So, ∑₁₀¹⁹(n² - 6n + 9) = ∑₁₀¹⁹(n - 3)²
So, ∑₁₀¹⁹(n² - 6n + 9) is A. ∑₁₀¹⁹(n - 3)²
Learn more about equivalent expression here:
https://brainly.com/question/29582897
#SPJ1
Simplify.
8w^10 x 6w^5
Answers
when multiplying values with exponents, you add the exponents instead of multiplying them
Given rectangles ABCD and A'B'C'D describe the transformation that takes place from ABCD to A'B'C'D:
10
a reflection over the y-axis, then a reflection over
the rads, and a 90° rotation clockwise about the
origin
8
A
B6
a 90 counterclockwise rotation about the origin
and a translation of 6 units to the right
ck
DI
-10 -8 -6 -4 2.0
-2
sa
46
А"
8 10%
-4
a reflection over the x-axis, then a reflection over
the y-ands, and a 90° rotation clockwise about the
origin
B'
-6
C
-8
-10
a 90 clockwise rotation about the origin and a
translation of 7 units down
Answers
Given rectangles ABCD and A'B'C'D, the transformation that takes place from ABCD to A'B'C'D is described as follows:
The given rectangle ABCD is shown below: Let's perform the transformations in the given order: i) A reflection over the y-axis: Reflect the rectangle ABCD over the y-axis to obtain the rectangle A''B''C''D''. ii) A reflection over the x-axis:
Reflect the rectangle A''B''C''D'' over the x-axis to obtain the rectangle A'''B'''C'''D'''. iii) A 90° rotation clockwise about the origin. Therefore, the transformation that takes place from ABCD to A'B'C'D is a reflection over the y-axis, then a reflection over the x-axis, and a 90° rotation clockwise about the origin.
To know more about rectangles visit :
https://brainly.com/question/15019502
#SPJ11
NEED HELP ASAP!!!!!!

Answers
Answer:
_31. I think
Step-by-step explanation:
PLEASE check
Pls help me with my homework

Answers
Answer:
Cos(F) = 0.47059
Step-by-step explanation:
Cos(F) = adjacent / hypotenuse
adjacent = 8
hypotenuse = 17
Cos(F) = 8 / 17
Cos(F) = 0.47059
What is the value of x? Round to the nearest tenth if necessary. 11 cm 4 cm cm x cm 8 cm
Answers
The volume of the given triangular prism is 192 cubic centimeters.
To find the volume of a triangular prism, we need to multiply the area of the base triangle by the height of the prism. In this case, the base of the triangle has a length of 12 cm and an altitude of 8 cm. Thus, the area of the base can be found using the formula for the area of a triangle, which is (1/2) x base x height.
Area of base triangle = (1/2) x 12 cm x 8 cm = 48 cm²
The height of the prism is given as 4 cm. Therefore, we can now find the volume of the prism by multiplying the area of the base by the height of the prism.
Volume of triangular prism = area of base x height of prism = 48 cm² x 4 cm = 192 cm³
To know more about volume here
https://brainly.com/question/11168779
#SPJ1
Complete Question:
Find the volume of the solid. Round to the nearest tenth, if necessary. triangular prism: base of triangle 12 cm, altitude of triangle 8 cm, height of prism 4 cm
Lucy has $7 less than Kristine and $5 more than Nina together,the three have $35 how much does Lucy have?
Answers
Lucy has $7 less than Kristine and $5 more than Nina together, the three have $35. Lucy has $11.
Let's denote the amount of money that Kristine has as K, the amount of money that Lucy has as L, and the amount of money that Nina has as N.
According to the given information, we can form two equations:
Lucy has $7 less than Kristine: L = K - 7
Lucy has $5 more than Nina: L = N + 5
We also know that the three of them have a total of $35: K + L + N = 35
We can solve this system of equations to find the values of K, L, and N.
Substituting equation 1 into equation 3, we get:
K + (K - 7) + N = 35
2K - 7 + N = 35
Substituting equation 2 into the above equation, we get:
2K - 7 + (L - 5) = 35
2K + L - 12 = 35
Since Lucy has $7 less than Kristine (equation 1), we can substitute K - 7 for L in the above equation:
2K + (K - 7) - 12 = 35
3K - 19 = 35
Adding 19 to both sides:
3K = 54
Dividing both sides by 3:
K = 18
Now we can substitute the value of K into equation 1 to find L:
L = K - 7
L = 18 - 7
L = 11
Finally, we can find the value of N by substituting the values of K and L into equation 3:
K + L + N = 35
18 + 11 + N = 35
N = 35 - 18 - 11
N = 6
Therefore, Lucy has $11.
Learn more about Kristine from
https://brainly.com/question/27244350
#SPJ11
On which number line do the letters represent −5 and +3?

Answers
Explanation: because on the line between -4 and -6, -5 must go in between them two. Also for the between 2 and 4, 3 must go in between. If you’re just counting up that’s how you’ll get those. Hope this helped! God bless
In integers what is -11 -6
Answers
Answer:
-17
Step-by-step explanation:
Let P(A) = 0.3 and P(B) = 0.4. Suppose A and B are independent.
What is the value of P(B|A)?
A. 0.12
B. 0.3
C. 0.4
D. 0.7
For two independent events A and B: P(B|A) = P(B).
Answers
The value of P(B|A) is 0.4, which is option C.
If two events A and B are independent, then the probability of one event occurring does not affect the probability of the other event occurring. In other words, P(B|A) = P(B) and P(A|B) = P(A).
Independent events are those events whose occurrence is not dependent on any other event.
For example, if we flip a coin in the air and get the outcome as Head, then again if we flip the coin but this time we get the outcome as Tail. In both cases, the occurrence of both events is independent of each other.
Given that P(A) = 0.3 and P(B) = 0.4, we can use the formula for independent events to find the value of P(B|A):
P(B|A) = P(B) = 0.4
For more similar questions on probability:
brainly.com/question/13604758
#SPJ11
