What is the component form of the vector that translates the top house figure to the bottom house figure?
(7, -2)
(6, -4)
(5, -1)
(-4, 5)

Answers
Answer:
Mark me the brainliest
Step-by-step explanation:
Okay, let's break this down step-by-step:
The top figure represents the original vector: (7, -2)
To translate it to the bottom figure: (6, -4)
We need to determine the component form of the translation.
The x-component is 7 - 6 = 1 (it decreased by 1)
The y-component is -2 - (-4) = -2 (it decreased by 2)
Therefore, the component form of the translation is: (1, -2)
So the translation vector that transforms the top figure (7, -2) into the bottom figure (6, -4) is (1, -2).
Does this make sense? Let me know if you have any other questions!
Related Questions
How do you solve for x in an angle?
Answers
To solve for x at an angle, you must first understand what type of angle you are working with. If the angle is a right angle, you can use the Pythagorean theorem to solve for x. If the angle is an obtuse angle, you can use the law of cosines to solve for x. If the angle is an acute angle, you can use the law of sines to solve for x.
How to solve for x at a right angle?To solve for x at a right angle using the Pythagorean theorem, you must have the lengths of the two neighboring sides of the triangle. The sum of the squares of the two sides is equal to the square of the hypotenuse, according to the Pythagorean theorem. By rearranging the components and solving for x, this equation may be used to find x.
How to solve for x at an obtuse angle?To use the law of cosines to solve for x at an obtuse angle, you must know the lengths of all three sides of the triangle. According to the law of cosines, the square of one side's length equals the sum of the squares of the other 2 sides minus twice the product of the other 2 sides multiplied by the cosine of the obtuse angle. You can use this equation to solve for x by rearranging the terms and solving for x.
How to solve for x in an acute angle?To apply the law of sines to solve for x in an acute angle, you should first know the lengths of the triangle's two sides as well as the acute angle's measurement. According to the law of sines, the ratio of one side's length to the sine of the opposite angle equals the ratio of the other side's length to the sine of the acute angle. By rearranging the terms and solving for x, you may use this equation to solve for x.
Learn more about acute angle here: brainly.com/question/6979153
#SPJ4
Given the following arithmetic sequence find the formula.

Answers
Answer:
D. an = 4n
Step-by-step explanation:
The formula for finding a term of an arithmetic sequence is:
an = a1 + (n-1)d
where an is the nth term, a1 is the first term and d is the common difference between the terms.
To find d we can take any two adjacent terms and subtract one from the other. e.g. 8 - 4 = 4, 16-12 = 4 etc.
The common difference here is 4, and we can see that the first term of the sequence is also 4, therefore our formula now looks like this:
an = 4 + (n-1)4
Now we can just expand out the brackets to find the final answer:
an = 4 + 4n - 4
an = 4n
Hope this helped!
1. t — 19 = 2
2. 1 + s = 3
3. 24 = 4c
4. v/5 = 9
(show work)
Answers
2. S=2. 3-1=2
3. C= 6. 24/4=6
4. V=45. 9x5=45
You do the opposite function to find your answer
Answer:
1. t = 21
2. s = 2
3. c = 6
4. v = 45
Step-by-step explanation:
1. t - 19 = 2
*Add 19 to both sides.
t = 21
2. 1 + s = 3
*Subtract 1 from both sides.
s = 2
3. 24 = 4c
*Divide each side by 4.
6 = c
4. v/5 = 9
*Multiply each side by 5.
v = 45
Fill The Blank ?a function is a rule that assigns to each value of the_____
Answers
In essence, a function is a rule that assigns to each value of the input set (also known as the domain), a unique value of the output set (also known as the range).
A function is a fundamental mathematical concept that is used to describe the relationship between two sets of values.
To understand the idea of a function, imagine a machine that takes in an input and produces an output. The input values are the domain of the function, and the output values are the range. A function can be represented as an equation, a graph, or a table. For example, the equation f(x) = x + 3 represents a function that takes in an input value x and produces an output value that is 3 greater than the input value.
One of the key features of a function is that each input value must have a unique output value. This means that if you input the same value into the function twice, you should get the same output value both times. In mathematical terms, we say that a function is well-defined if it has a unique output value for each input value.
Functions are used in a wide range of mathematical applications, from algebra and calculus to statistics and data analysis. They provide a powerful tool for describing and analyzing relationships between different sets of values.
To know more about function here
https://brainly.com/question/28193995
#SPJ4
HELP ASAP, LINKS AND ABSURD ANSWERS WILL BE REPORTED! I WILL MARK BRAINLIEST!!

Answers
Answer:
5/3 or 3/5
Step-by-step explanation:
MRK ME BRAINLIEST PLZ
Can you guys help me with this question?

Answers
Answer:
it represents 10 stars in the galaxies
Step-by-step explanation:
10 to the power of 12 would be 1,000,000,000,000, 10 to the power of 11 would be 100,000,000,000, and those two number divided equals simply 10.
Can someone please help mee?
Using the chart, find the equation from
the relation.
input x 15 16 17 18
output y 8 9 10 11
y = x + [?]
Answers
On solving the provided question, we can say that the linear equation from the relation is: y = x + (-7) => y = x - 7
What is a linear equation?A linear equation is one that has the form y=mx+b in algebra. B is the slope, and m is the y-intercept. It's usual to refer to the previous clause as a "linear equation with two variables" because y and x are variables. The two-variable linear equations known as bivariate linear equations. There are several instances of linear equations: 2x - 3 = 0, 2y = 8, m + 1 = 0, x/2 = 3, x + y = 2, and 3x - y + z = 3. It is referred to as being linear when an equation has the form y=mx+b, where m stands for the slope and b for the y-intercept.When an equation has the formula y=mx+b, with m denoting the slope and b the y-intercept, it is referred to as being linear.
The equation can be found using the slope-intercept form of a line, y = mx + b, where m is the slope and b is the y-intercept.
To find the slope (m), you can use the formula: m = (change in y) / (change in x)
For the points (15, 8) and (16, 9), the change in y is 9 - 8 = 1 and the change in x is 16 - 15 = 1, so m = 1/1 = 1.
To find the y-intercept (b), you can use one of the points and the slope to solve for b. For example, using the point (15, 8), substitute x = 15 and y = 8 into the equation y = mx + b, and solve for b:
8 = 1 * 15 + b
8 = 15 + b
b = 8 - 15
b = -7
So, the equation from the relation is:
y = x + (-7)
y = x - 7
To know more about linear equation visit:
https://brainly.com/question/11897796
#SPJ1
I need help please I will give brainliest to the best answer...
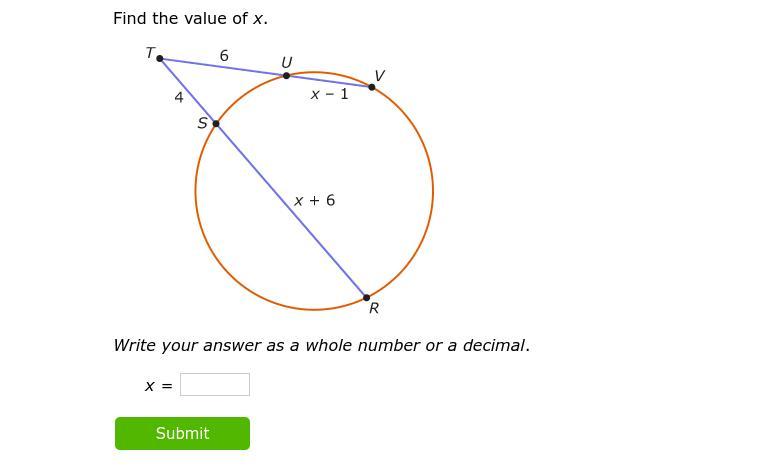
Answers
The value of x in the intersecting chords that extend outside circle is 5
Calculating the value of xFrom the question, we have the following parameters that can be used in our computation:
intersecting chords that extend outside circle
Using the theorem of intersecting chords, we have
4 * (x + 6 + 4) = 6 * (x - 1 + 6)
Evaluate the like terms
So, we have
4 * (x + 10) = 6 * (x + 5)
Using a graphing tool, we have
x = 5
Hence. the value of x is 5
Read more about intersecting chords at
https://brainly.com/question/13950364
#SPJ1
Evaluate the expression.

Answers
Answer:
1/9, decimal form = 0.1 repeating
Answer:
1/9
Step-by-step explanation:
3^-2
We know that a^-b = 1/a^b
1/3^2
1/9
the probability that a student pilot passes the written test for a private pilot’s license is 0.7. find the probability that a student will pass the test before the third try
Answers
The probability that a student will pass the test before the third try is 0.91 or 91%.
The probability that a student pilot passes the written test for a private pilot’s license is 0.7.
To find the probability that a student will pass the test before the third try, we can use the geometric distribution.
The Geometric Distribution is a discrete probability distribution that describes the number of failures before a success in a sequence of independent and identical Bernoulli trials. It is one of the essential examples of distributions in probability theory and statistics
Let P be the probability that a student pilot passes the written test for a private pilot’s license.
Then the probability that a student will fail the test on the first two tries is given by:
P(failure) = (1 - P)² [i.e., the probability of not passing the test]
Then the probability that the student will pass the test before the third try is the complement of the above probability, which is given by:
P(pass before 3rd try) = 1 - (1 - P)²
Let P = 0.7.
Then the probability that a student will pass the test before the third try is:
P(pass before 3rd try) = 1 - (1 - 0.7)²
P(pass before 3rd try) = 1 - 0.09 = 0.91
Therefore, the probability that a student will pass the test before the third try is 0.91 or 91%.
Know more about the Geometric Distribution
https://brainly.com/question/30478452
#SPJ11
7. A bag of marbles contains 3 green, 7 red, 2 white
and 8 black marbles. If a marble is randomly
chosen, what is the probability that the marble will
NOT be green or white?
a. 25%
b. 50%
C. 60%
d. 75%
e. 80%
Answers
Answer: 50%
Step-by-step explanation:
Answer:
D. 75%
Step-by-step explanation:
There are 20 marbles, so each 1 has a chance of 5% If you were to choose randomly 75% of the time you will get either red or black and 25% you will get green or white
which expression is equivalent to 12a + 8
Answers
Answer:
i cant see the choices but i think this is khan academy
Step-by-step explanation:
A.
By using distributive property you can figure out all the answers.
A: 4*3a, 4*2= 12a + 8
B: 4*3a, 4*8= 12a + 32
C: 6*6a, 6*2= 36a + 12
D: 6*6a, 6*8= 36a + 48
Answer:
a =-2/3
Step-by-step explanation:
I can't see the answer choices but I'm going to try helping you.
12a + 8 is like a two step equation make it equal to zero
12a + 8 = 0
Subtract 8 on each side
12a = -8
Now divide by 12 on each side
a = -2/3
Hope this helps!
Please help. The picture is below

Answers
Answer:
yea you got it right its c
Step-by-step explanation:
Let Xt be a Poisson process with parameter λ. Independently, let T∼Exp(μ). Find the probability mass function for X(T).
Answers
To find the PMF for X(T), we first find the conditional distribution of X(t) given T = t, which is a Poisson distribution with parameter λt. Then, we multiply this conditional distribution by the density function of T, which is μe^(-μt), and integrate over all possible values of t.
The probability mass function (PMF) for X(T), where Xt is a Poisson process with parameter λ and T is exponentially distributed with parameter μ, can be expressed in two steps. First, we need to find the conditional probability distribution of X(t) given T = t for any fixed t. This distribution will be a Poisson distribution with parameter λt. Second, we need to find the distribution of T. Since T is exponentially distributed with parameter μ, its probability density function is fT(t) = μe^(-μt) for t ≥ 0. To find the PMF for X(T), we can multiply the conditional distribution of X(t) given T = t by the density function of T, and integrate over all possible values of t. This will give us the PMF for X(T).
Now, let's explain the answer in more detail. Given that T = t, the number of events in the time interval [0, t] follows a Poisson distribution with parameter λt. This is because the Poisson process has a constant rate of λ events per unit time, and in the interval [0, t], we expect on average λt events to occur.
To obtain the PMF for X(T), we need to consider the distribution of T as well. Since T is exponentially distributed with parameter μ, its probability density function is fT(t) = μe^(-μt) for t ≥ 0.
To find the PMF for X(T), we multiply the conditional distribution of X(t) given T = t, which is a Poisson distribution with parameter λt, by the density function of T, and integrate over all possible values of t. This integration accounts for the uncertainty in the value of T.
The resulting PMF for X(T) will depend on the specific form of the density function fT(t), and the Poisson parameter λ. By performing the integration, we can derive the expression for the PMF of X(T) in terms of λ and μ.
Learn more about Poisson distribution here:
https://brainly.com/question/30388228
#SPJ11
Two customers took out automobile loans.
-Katy took out a 5-year loan for $18,000 and paid 7.00% annual simple interest.
-Frank took out a 6-year loan for $18,000 and paid 6.00% annual simple interest.
What is the difference between the amounts of interest Katy and Frank paid for their loans?
A. $180
B. $240
C. $300
D. $360
Answers
Answer:
A. $180
Step-by-step explanation:
18000*5*7/100 =6300
18000*6*6/100=6480
6480-6300=180
Becky orders pens from an office supply company. The table shows how many pens have black ink based on the total number of pens ordered Total Pens Pens with Black Ink 144 60 288 120 432 180 If 90 pens with black ink came in order, how many total pens were ordered?
you will get 100 points just please hurry!
Answers
If 90 pens with black ink were ordered, the total number of pens ordered would be 216.
To solve this problem, we need to find the ratio between the total number of pens and the number of pens with black ink. We can then use this ratio to determine the total number of pens when given the number of pens with black ink.
Let's calculate the ratio for the first set of data:
Ratio = (Pens with Black Ink) / (Total Pens) = 60 / 144
We can simplify this ratio by dividing both the numerator and denominator by their greatest common divisor, which is 12:
Ratio = 5 / 12
Now, we can use this ratio to find the total number of pens when 90 pens with black ink are ordered:
Total Pens = (Pens with Black Ink) / Ratio = 90 / (5 / 12)
Dividing 90 by 5/12 is the same as multiplying 90 by the reciprocal of 5/12:
Total Pens = 90 * (12 / 5) = 216
Therefore, if 90 pens with black ink were ordered, the total number of pens ordered would be 216.
For more such questions on total number , Visit:
https://brainly.com/question/9879870
#SPJ11
f(x) = x3 -27
Find the zeros
Answers
Answer:
x=9
y=-27
Step-by-step explanation:
What angular resolution would you need to see the Sun and Jupiter as distinct points of light? Express your answer in arcseconds to two significant figures. Jupiter 195| ΑΣΦ % ? 11 Suppose you were looking at our own solar system from a distance of 6.0 light-years.
Answers
An angular resolution of 0.56 arcseconds is required to see the Sun and Jupiter as separate objects. This is an extremely small angle and would necessitate the use of a large telescope.
Angular resolution is defined as the minimum angle between two objects that enables a viewer to see them as distinct objects rather than as a single one. A better angular resolution corresponds to a smaller minimum angle. The angular resolution formula is θ = 1.22 λ / D, where λ is the wavelength of light and D is the diameter of the telescope. Thus, the angular resolution formula can be expressed as the smallest angle between two objects that allows a viewer to distinguish between them. In arcseconds, the answer should be given to two significant figures.
To see the Sun and Jupiter as distinct points of light, we need to have a good angular resolution. The angular resolution is calculated as follows:
θ = 1.22 λ / D, where θ is the angular resolution, λ is the wavelength of the light, and D is the diameter of the telescope.
Using this formula, we can find the minimum angular resolution required to see the Sun and Jupiter as separate objects. The Sun and Jupiter are at an average distance of 5.2 astronomical units (AU) from each other. An AU is the distance from the Earth to the Sun, which is about 150 million kilometers. This means that the distance between Jupiter and the Sun is 780 million kilometers.
To determine the angular resolution, we need to know the wavelength of the light and the diameter of the telescope. Let's use visible light (λ = 550 nm) and assume that we are using a telescope with a diameter of 2.5 meters.
θ = 1.22 λ / D = 1.22 × 550 × 10^-9 / 2.5 = 2.7 × 10^-6 rad
To convert radians to arcseconds, multiply by 206,265.θ = 2.7 × 10^-6 × 206,265 = 0.56 arcseconds
The angular resolution required to see the Sun and Jupiter as distinct points of light is 0.56 arcseconds.
This is very small and would require a large telescope to achieve.
In conclusion, we require an angular resolution of 0.56 arcseconds to see the Sun and Jupiter as separate objects. This is an extremely small angle and would necessitate the use of a large telescope.
To know more about angular resolution visit:
brainly.com/question/31858807
#SPJ11
HELP PLEASE Can you please give an explication when you answer
The temperature at 4:00 am was -3°C, and the temperature rose 9°C during the morning. What was the temperature by noon?
A. -6°C
B. -12°C
C. 6°C
D. 12°C
Answers
Answer:
C. 6°C
Step-by-step explanation:
-3°C + 9°C = 6°C
A button has a diameter of 4 centimeters. What is the area of the button
Answers
diameter = 4 cm
so radius r = 4 : 2 = 2 cm
area of a circle = pi x r² ≈ 3,14 x 2² = 12,56 cm²
Directions: Solve for x, then find each angle measure.

Answers
what is 1\2 y =1 in standard form
Answers
Answer:
y=2
Step-by-step explanation:
6.RP.3d-2016 (#39) Fei Yen's dog eats 8 ounces of dog food each day. Fei Yen bought a 28-pound bag of dog
food. How many 8-ounce servings are in a 28-pound bag of dog food?
A 14
B 56
C 224
D 448
Answers
There are 56 servings in a 28 pound bag of dog food.
What is Unitary Method ?The unitary technique involves first determining the value of a single unit, followed by the value of the necessary number of units.
For example, Let's say Ram spends 36 Rs. for a dozen (12) bananas.
12 bananas will set you back 36 Rs. 1 banana costs 36 x 12 = 3 Rupees.
As a result, one banana costs three rupees. Let's say we need to calculate the price of 15 bananas.
This may be done as follows: 15 bananas cost 3 rupees each; 15 units cost 45 rupees.
Given:
Each day Fei yen's dog eat dog food = 8 ounces
Fei yen bought a 28 pound bag of dog food.
And we know that 1 pound = 16 ounces
Then ,the food in ounces
= 28 x 16
= 448 ounces
Now, the number of 8 ounces servings are in a 28 pound bag of dog food
= 448 / 8
= 56
Learn more about Unitary Method here:
https://brainly.com/question/2565841
#SPJ9
What angle of rotation maps X to X”?

Answers
Solve-3(z-6) ≥ 2z-2 for z
Answers
Answer: Z<4
Step-by-step explanation:
Rearrange the equation
-3(z-6) - (2z-2)>0
-3z+18-2z+2>0
-5z +20>0
-5(z-4)>0
divide both side by -5
z-4<0
z<4
I NEED HELPPPPPPPPPPPOOOPPPPPPPPPPPPPPP

Answers
Answer:
A
Step-by-step explanation:
A is between -3 and -4
Write the equation of the line perpendicular to y = -3x - 1 that passes through the point (3, 4)
Answers
Answer:
y = 1/3x + 3
Step-by-step explanation:
Draw (daily) budget constraints with leisure on the x-axis and income on the y-axis (assuming the price of all-other-goods is $1) to illustrate the two proposals for a representative individual as well as the original budget constraint with no policy. Assume that an individual works, on average, 200 days per year so the $3000 income guarantee is equivalent to $15 per day. Also assume the wage rate is $5 per hour.
Answers
To illustrate the budget constraints, let's consider the two proposals for a representative individual and the original budget constraint with no policy.
Proposal 1: Income Guarantee of $3000 per year ($15 per day)
In this proposal, the individual receives an income guarantee of $3000 per year, which is equivalent to $15 per day. We can plot the budget constraint with leisure on the x-axis and income on the y-axis.
The slope of the budget constraint represents the wage rate, which is $5 per hour. Assuming an individual works an average of 200 days per year, the maximum income can be calculated as follows:
Max Income = Wage Rate * Hours per Day * Days per Year
= $5/hour * 8 hours/day * 200 days/year
= $8000
Therefore, the budget constraint with the income guarantee of $3000 per year would be a straight line passing through the point (0, $3000) and (160, $5000) since $5000 is the income level when all days are worked (200 days * $25 per day).
Proposal 2: Original Budget Constraint with No Policy
In this proposal, we consider the original budget constraint with no policy. Assuming the wage rate is $5 per hour and the individual works an average of 200 days per year, the maximum income is still $8000. The budget constraint without any policy would also be a straight line passing through the point (0, $0) and (160, $8000).
To illustrate both proposals and the original budget constraint on the same graph, we can plot the budget constraints as straight lines with leisure on the x-axis and income on the y-axis. The budget constraints would intersect at different points representing the different policies.
Note: Without specific information about the leisure-income trade-off or any additional policies, it is difficult to provide precise coordinates for the intersection points or the slopes of the budget constraints. The graph provided is a general representation to illustrate the concepts.
To know more about budget refer here
https://brainly.com/question/31952035#
#SPJ11
letter b: Original quantity 20 new quantity 8
letter c: original quantity : 50 new quantity : 38
can someone please help and explain how to get the percentage of these please?
Answers
The percentage of the new quantity in letter b is 40% and the Percentage of the new quantity in letter c is 76%.
What is percentage?A percentage, often known as percent, is a division by 100. Percentage, which means "per 100," designates a portion of a total sum.
Given data:
letter b: Original Quantity: 20 new quantity: 8
letter c: original quantity: 50 new quantity: 38
Calculate the percentage as shown below,
Percentage of new quantity in letter b = new quantity / Original Quantity × 100
Percentage of new quantity in letter b = 8 / 20 × 100 = 40%
Percentage of new quantity in letter c = new quantity / Original Quantity × 100
Percentage of new quantity in letter c = 38 / 50 × 100 = 76%
Therefore, the percentage of the new quantity in letter b is 40% and the Percentage of the new quantity in letter c is 76%.
To know more about percentages:
brainly.com/question/20818421
#SPJ1
Help me please help me please help me please please

Answers
1. The length of x and y are 5 and 8.66 respectively
2. The area of the equilateral triangle is 9√3 cm²
What is trigonometric ratio?Trigonometric Ratios are defined as the values of all the trigonometric functions based on the value of the ratio of sides in a right-angled triangle.
sin(tetha) = opp/hyp
cos(tetha) = adj/hyp
tan(tetha) = opp/adj
sin30 = x/10
0.5 = x/10
x = 0.5×10
x = 5
sin60 = y/10
0.866 = y/10
y = 0.866 × 10
y = 8.66
2. The height of the triangle = √6²-3²
= √36-9
= √27
= 3√3
area = 1/2bh
= 1/2 × 6 × 3√3
= 9√3 cm²
learn more about trigonometric ratio from
https://brainly.com/question/24349828
#SPJ1