Plz answer this question I'll give brainliest to first person who answers.

The equation of the tangent plane to the surface 3z = xe^xy + ye^x at the point (6, 0, 2) is x + 37y + 3z - 12 = 0.

To find the equation of the tangent plane to the surface 3z = xe^xy + ye^x at the point (6, 0, 2), we will follow these steps:

Find the partial derivatives of the surface equation with respect to x, y, and z.

Partial derivative with respect to x:

∂(3z)/∂x = e^xy + xye^xy

Partial derivative with respect to y:

∂(3z)/∂y = x^2e^xy + e^xy

Partial derivative with respect to z:

∂(3z)/∂z = 3

Evaluate the partial derivatives at the point (6, 0, 2).

∂(3z)/∂x = e^(60) + 60e^(60) = 1

∂(3z)/∂y = (6^2)e^(60) + e^(60) = 37

∂(3z)/∂z = 3

The equation of the tangent plane can be written as:

∂(3z)/∂x(x - 6) + ∂(3z)/∂y(y - 0) + ∂(3z)/∂z(z - 2) = 0

Substituting the evaluated partial derivatives:

1(x - 6) + 37(y - 0) + 3(z - 2) = 0

x - 6 + 37y + 3z - 6 = 0

x + 37y + 3z - 12 = 0

Therefore, the equation of the tangent plane to the surface 3z = xe^xy + ye^x at the point (6, 0, 2) is x + 37y + 3z - 12 = 0.

Now, let's use Lagrange multipliers to find the minimum value of the function f(x, y, z) = x^2 - 4x + y^2 - 6y + z^2 - 2z + 5, subject to the constraint x + y + z = 3.

Define the Lagrangian function L(x, y, z, λ) as:

L(x, y, z, λ) = f(x, y, z) - λ(g(x, y, z) - c)

Where g(x, y, z) is the constraint function (x + y + z) and c is the constant value (3).

L(x, y, z, λ) = x^2 - 4x + y^2 - 6y + z^2 - 2z + 5 - λ(x + y + z - 3)

Compute the partial derivatives of L with respect to x, y, z, and λ.

∂L/∂x = 2x - 4 - λ

∂L/∂y = 2y - 6 - λ

∂L/∂z = 2z - 2 - λ

∂L/∂λ = -(x + y + z - 3)

Set the partial derivatives equal to zero and solve the system of equations.

2x - 4 - λ = 0 ...(1)

2y - 6 - λ = 0 ...(2)

2z - 2 - λ = 0 ...(3)

x + y + z - 3 = 0

To learn more about equation, refer below:

https://brainly.com/question/29657983

#SPJ11

The value of x as shown in the diagram is 2.

Tangent to a circle is the line that touches the circle at only one point.

Note: Tangent drawn from the same external points are equal.

To calculate the value of x, we use the above fact.

From the diagram,

Given:

Substitute into equation 1 and solve for x

Hence,  x = 2.

Learn more about tangent here: https://brainly.com/question/30085302

#SPJ1

The direction of greatest increase in heat from the point (2, 5) on the metal plate described by the temperature function t(x, y) = x^3 y^2 is along the positive x-axis. This means that the temperature increases the most as we move in the direction of increasing x-coordinate.

To find the direction of greatest increase in heat from the point (2, 5) on the metal plate, we need to calculate the gradient vector of the temperature function t(x, y) = x^3 y^2. The gradient vector represents the direction of the steepest increase in a scalar function.

Let's calculate the partial derivatives of t(x, y) with respect to x and y:

∂t/∂x = 3x^2 y^2

∂t/∂y = 2x^3 y

Now, evaluate the partial derivatives at the given point (2, 5):

∂t/∂x = 3(2)^2 (5)^2 = 300

∂t/∂y = 2(2)^3 (5) = 80

The gradient vector (∇t) at (2, 5) is given by (∂t/∂x, ∂t/∂y) = (300, 80). The direction of the gradient vector indicates the direction of the steepest increase in heat. Since the x-component (300) is larger than the y-component (80), the direction of greatest increase in heat is along the positive x-axis.

To learn more about function click here: brainly.com/question/30721594

#SPJ11

The correct option c.) one-sample z-test, for the inference test used by the Cate.

As stated in the assertion

As a result, cate uses one sample Z Test.

To know more about the one-sample z-test, here

https://brainly.com/question/17144617

#SPJ4

The probability of the sample mean contents of the 10 bottles will depend on the distribution of the contents in the population, the sample size, and the sample mean.

The probability of the sample mean contents of the 10 bottles will depend on the distribution of the contents in the population from which the sample is drawn. If the distribution is normal, then the probability of the sample mean can be calculated using the formula:

P(x) = (1 / √(2πσ^2)) * e^(-(x-μ)^2 / (2σ^2))

Where μ is the population mean, σ is the population standard deviation, and x is the sample mean. The probability of the sample mean will be the area under the normal curve between the sample mean and the population mean.

If the distribution is not normal, then the probability of the sample mean can be calculated using the central limit theorem, which states that the distribution of sample means approaches a normal distribution as the sample size increases. In this case, the probability of the sample mean can be calculated using the formula for the normal distribution, with the sample mean as the mean and the standard error of the mean as the standard deviation.
Learn more about probability:https://brainly.com/question/25870256

#SPJ11

The absolute value inequality to determine the range of acceptable bead lengths is |B - 4.3| ≤ 0.2, and the range of acceptable lengths is 4.1 ≤ B ≤ 4.5 centimeters.

Given that the target length (L) of the ceramic bead is 4.3 centimeters with an acceptable allowance (T) of 0.2 centimeters, we can write the absolute value inequality as follows:

|B - 4.3| ≤ 0.2

This inequality states that the absolute difference between the bead length (B) and the target length (4.3) must be less than or equal to 0.2.

To determine the range of acceptable bead lengths, we can solve this inequality. Let's consider two cases:

Case 1: B - 4.3 ≤ 0.2

Solving for B:

B ≤ 4.5

Case 2: -(B - 4.3) ≤ 0.2

Solving for B:

-B + 4.3 ≤ 0.2

4.3 - 0.2 ≤ B

B ≥ 4.1

Combining both cases, the range of acceptable bead lengths is:

4.1 ≤ B ≤ 4.5

Therefore, the range of acceptable bead lengths is from 4.1 centimeters to 4.5 centimeters.

To know more about inequality, refer here:

https://brainly.com/question/32844662

#SPJ4

Complete Question:

The length of a manufactured ceramic bead is 4.3 centimeters with an acceptable allowance of 0.2 centimeters. Beads that are too big or too small are discarded.

What absolute value inequality can be written to determine the range of acceptable bead lengths, and what is this range of lengths?

Step-by-step explanation:

According to angles of intersecting chords theorem    ( angle S is also 117):

117 = 1/2 (208 + 2x-4)  

so  x = 15

then 2x-4 = 26 degrees

The expression for the cost of buying n book will be 20 + 13n.

Expression simply refers to the mathematical statements which have at least two terms which are related by an operator and contain either numbers, variables, or both. Addition, subtraction, multiplication, and division are all possible mathematical operations.

It is a finite collection of symbols that are well-formed in accordance with context-dependent principles. Numbers, variables, operations, functions, brackets, punctuation, and grouping can all be represented by mathematical symbols, which can also be used to indicate the logical syntax's order of operations and other features.

In this case, the person some books online for myself and friends and each book costs $13 and the store charges a one-time shipping fee of $20.

The expression for the cost of buying n books is:

= 20 + (13 × n)

= 20 + 13n

Learn more about expressions on:

brainly.com/question/723406

#SPJ1

Answer:

x=4

Step-by-step explanation:

5(x−3)=5

Step 1: Simplify both sides of the equation.

5(x−3)=5

(5)(x)+(5)(−3)=5(Distribute)

5x+−15=5

5x−15=5

Step 2: Add 15 to both sides.

5x−15+15=5+15

5x=20

Step 3: Divide both sides by 5.

5x / 5 = 20 / 5

x=4

Answer:

= (ln(9))^2 = 4(ln(3))^2 = 4.8278

Step-by-step explanation:

Using L'hopital Theorem

Defrentiante the numerator and denominator twice each by its self.

like in here :

http://ar.symbolab.com/solver/limit-calculator/%5Clim_%7Bx%5Cto0%7D%5Cleft(%5Cfrac%7B9%5E%7Bx%7D%2B9%5E%7B-x%7D-2%7D%7Bx%5E%7B2%7D%7D%5Cright)

for any other limit calculation use this : http://ar.symbolab.com/solver/limit-calculator/

The total amount of the monthly payments and the finance charge is 144.

Instalment is one of the regular payments that anyone can  make for anything until you have fully paid that full amount.

Using the appropriate interest relation, the solution to the problems posed are :

Down payment = 0.05k

144 monthly payments

Total monthly payment made = 144g

Down payment :

5% of total cost

Total cost = k

5% × k = 0.05k

B.)

Number of monthly payments :

Number of months per year = 12

Number of years = 12

Number of monthly payments = 12 × 12 = 144

C.)

Total charge :

Monthly payment = $g

Number of monthly payments × amount paid

g × 144 = 144g

To know more about instalment click-

https://brainly.com/question/16032019

#SPJ1

Answer:

<7= 101

<8= 101

Hence, <1= 101, <2=79, <3= 101, <4= 101, <5= 79, <6= 79, <7= 101, and <8= 101

Step-by-step explanation:

3x + 19 = 5x - 29 ( opposite angles)

2x = 48

x = 24

Hence, the value N = 2y

< 2 = 3x + 7 ( Verticaly opposite angles).

<2 = 3 (24) + 7

<2 = 79

<1 + <2 = 180

<1 = 180 - <2 = 180 - 79 = 101

<1 = 101

<1 = 3 ( Opposite angle )

<3 = 101

<5 = 3x + 7 ( Adjacent angles )

<5 = 79

<4 + <5 = 180

<4 = 101

<6 = <5 ( Verticaly opposite angles )

<6 = 79

<7 = <4 ( Adjacent Angles )

< 8 = 4x + 5

<7 = 101

<8 = 101

Hence, <1 = 101, <2 = 79, <3 = 101, <4 = 101, <5 = 79, <6 = 79, <7 = 101, <8 = 101

Answer:

2×1=2

2×2=4

2×3=6

2×4=8

2×5=10

2×6=12 ==> Answer

Answer:

3/2 o 1 entero 1/2

Step-by-step explanation:

150% es igual a 150 sobre 100= 150/100

(% este simbolo significa que el numero será divido por 100)

simplificas y te va a salir 3/2

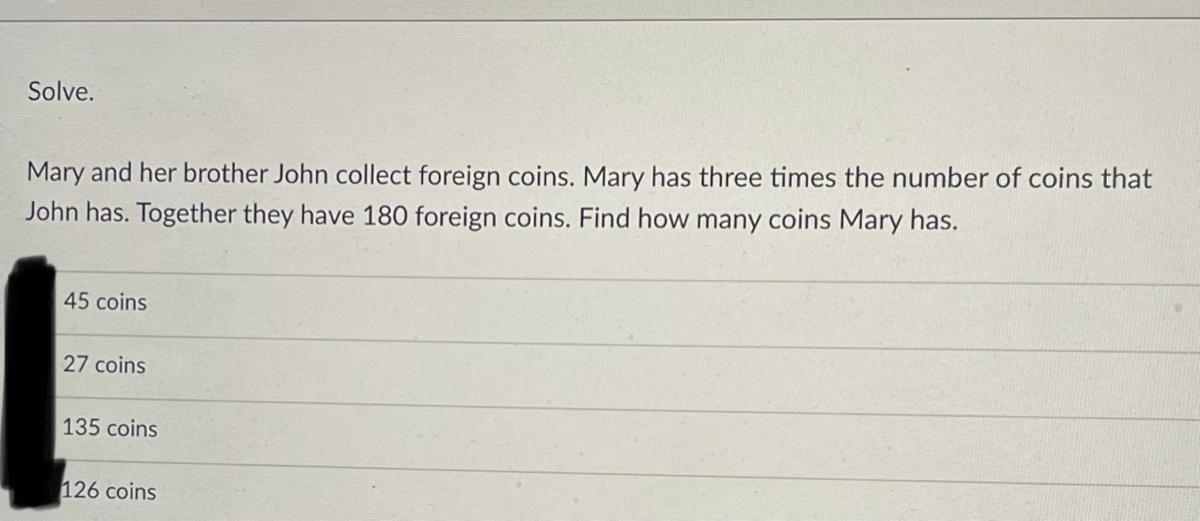
Answer:

mary has 135 coins.

Step-by-step explanation:

let the coins that john has be x

and coin mary than = 3x

according to the question equation is

x + 3x = 180

4x = 180

x = 180 / 4

x = 45

coins mary has  = 3x

=3*45

=135

The number of toffee cookies Sarah baked is:

4x = 4(17) = 68

If 2/7 of the cookies are chocolate cookies then the number of chocolate cookies Sarah baked is:

2/7 x 420 = 120

If 35% of the cookies are raisin cookies then the number of raisin cookies Sarah baked is:

35/100 x 420 = 147

Let the number of toffee cookies be 4x and the number of plain cookies be 5x x is a constant.

The total number of cookies is the sum of the number of chocolate raisin toffee and plain cookies:

Total number of cookies = Number of chocolate cookies + Number of raisin cookies + Number of toffee cookies + Number of plain cookies

Substituting the values we know:

420 = 120 + 147 + 4x + 5x

Simplifying and solving for x:

420 = 267 + 9x

9x = 153

x = 17

The number of toffee cookies Sarah baked is:

4x = 4(17) = 68

For similar questions on cookies

https://brainly.com/question/29104131

#SPJ11

Value of x = √2/3, and co-ordinate of point will be (√2/3 , \(\sqrt{7} /3\) )

Unit circle:

                 Circle having radius 1 unit is called unit circle.

Let us consider,

                          co-ordinate of point is (x , \(\sqrt{7} /3\) )

as the above point is lying on the first quadrant and on unit circle.

         for the unit circle,

                                        \(x^{2} + y^{2} =1\)   center of circle (0,0)

parametric form of equation

                                   x = cosθ   and     y=sinθ

so any point on the unit circle will be,

                   (x, y) = ( cosθ ,sinθ )

for,

          (x , \(\sqrt{7} /3\) )

                               y = \(\sqrt{7} /3\)

                            sinθ =   \(\sqrt{7} /3\)

             x = cosθ  

                  = ± √(1 - sin^2 θ)

                  = ± √(1 - 7/9)

                   = ± √((9- 7)/9)

                    = ± √(2/9)

                   = ± √2/3

but we can not take the negative value, because co-ordinate point is lying in the first quadrant.

                            so,

                                    x = √2/3

thus ,

                               co-ordinate of point will be

                                            (x, y) = (√2/3 , \(\sqrt{7} /3\) )

To learn more about unit circle visit : https://brainly.com/question/23519482

#SPJ9

Answer: \(\sqrt{68}\) units.

Step-by-step explanation:

The distance between points (a, b) and (c, d) is given by :_

\(D=\sqrt{(d-b)^2+(c-a)^2}\)

Given points : (-10,-7) (-8,1)

Distance between points (-10,-7) and (-8,1) = \(\sqrt{(1-(-7))^2+(-8-(-10))^2}\)

\(=\sqrt{(1+7)^2+(-8+10)^2}\\\\=\sqrt{8^2+2^2}\\\\=\sqrt{64+4}\\\\=\sqrt{68}\)

Hence, distance between given points = \(\sqrt{68}\) units.

Send a better cropped

photo

As a quality controller in a manufacturing company, I would conduct a hypothesis test to determine if there is a significant difference in the mean measurements of shaft diameters between two groups.

To conduct the hypothesis test, I would collect a sample of shaft measurements from each group and calculate the sample means. Then, I would perform a statistical test, such as a two-sample t-test, to compare the means of the two groups. The null hypothesis (H0) would state that there is no difference in the mean shaft measurements between the groups, while the alternative hypothesis (Ha) would suggest that there is a significant difference.

The test would provide a p-value, which indicates the probability of observing a difference in the means as extreme as the one obtained in the sample, assuming that the null hypothesis is true. If the p-value is below a predetermined significance level (e.g., 0.05), we would reject the null hypothesis and conclude that there is a significant difference in the mean measurements of the shaft diameters between the two groups. This would indicate the presence of a systematic variation or discrepancy in the manufacturing process that needs to be addressed to ensure consistent quality in the shaft production.

Learn more about probability here: brainly.com/question/32117953

#SPJ11

The deseasonalized sales for this period, when the actual sales are 2,763.00 units and the seasonal index for this period is 0.84, are 3,289.29 units.

To find the deseasonalized sales for a given period, we need to divide the actual sales by the seasonal index for that period. This will give us the sales that would have occurred if there were no seasonal fluctuations.

In this case, the actual sales are 2,763.00 units and the seasonal index is 0.84. So, we can use the following formula to find the deseasonalized sales:

Deseasonalized sales = Actual sales / Seasonal index

Deseasonalized sales = 2,763.00 / 0.84

Deseasonalized sales = 3,289.29 units

Therefore, the deseasonalized sales for this period are 3,289.29 units.

You can learn more about deseasonalized sales at

https://brainly.com/question/29846523

#SPJ11

Answer:

1. 0.45

2. 0.06

3. 0.39

4. 7.77

Step-by-step explanation:

causeeeeeeee

Remember that any natural number can be written as a product of prime numbers. Prime numbers are those numbers that are only divisible by one and themselves.

In the case of 72:

Therefore, the answer is the third option 72=2^3*3^2

By applying logarithmic differentiation to the equation

\(x^2 + (y - ∛(x^2))^2 = 1\), we can find the derivative of y with respect to x. The derivative is given by \(dy/dx = -4x(y - ∛(x^2)) / (2x^2 + 3(y - ∛(x^2))^2)\).

To use logarithmic differentiation, we start by taking the natural logarithm of both sides of the equation: \(ln(x^2 + (y - ∛(x^2))^2) = ln(1).\) Applying the logarithmic property, we can rewrite the equation as

\(ln(x^2) + ln((y - ∛(x^2))^2) = 0.\)

Next, we differentiate both sides of the equation with respect to x. Using the chain rule and the fact that the derivative of ln(u) is du/u, we obtain:

\((2x/x^2) + (2(y - ∛(x^2))/ (y - ∛(x^2))) * (1/2(y - ∛(x^2))) * (d(y - ∛(x^2))/dx) = 0\).

Simplifying the equation, we have

\(2/x + (2(y - ∛(x^2))) / (2(y - ∛(x^2))) * (d(y - ∛(x^2))/dx) = 0\).

Canceling out common factors, we get:

\(2/x + d(y - ∛(x^2))/dx = 0\).

Rearranging the equation to solve for

\(d(y - ∛(x^2))/dx\), we have\(d(y - ∛(x^2))/dx = -2/x.\)

Finally, using the power rule for differentiation, we can express the derivative of y with respect to x as

\(dy/dx = -4x(y - ∛(x^2)) / (2x^2 + 3(y - ∛(x^2))^2).\)

Learn more about derivative here:

https://brainly.com/question/29144258

#SPJ11

The volume generated by rotating the region bounded by the curve y = x about the y-axis using the method of cylindrical shells is 486π cubic units.

To find the volume generated by rotating the region bounded by the curve y = x about the y-axis using the method of cylindrical shells, we can follow these steps:

First, let's sketch the region bounded by the curve y = x. This is a straight line passing through the origin with a slope of 1. It forms a right triangle in the first quadrant, with the x-axis and y-axis as its legs.

Next, we need to determine the limits of integration. Since we are rotating about the y-axis, the integration limits will correspond to the y-values of the region. In this case, the region is bounded by y = 0 (the x-axis) and y = x.

The height of each cylindrical shell will be the difference between the upper and lower curves. Therefore, the height of each shell is given by h = x.

The radius of each cylindrical shell is the distance from the y-axis to the x-value on the curve. Since we are rotating about the y-axis, the radius is given by r = y.

The differential volume element of each cylindrical shell is given by dV = 2πrh dy, where r is the radius and h is the height.

Now we can express the volume of the solid of revolution as the integral of the differential volume element over the range of y-values:

V = ∫[a, b] 2πrh dy

Here, [a, b] represents the range of y-values that define the region. In this case, a = 0 and b = 9 (as y = x, so the curve intersects y-axis at y = 9).

Substituting t

he values of r and h into the integral, we have:

V = ∫[0, 9] 2πy(y) dy

Simplifying, we get:

V = 2π ∫[0, 9] y^2 dy

Evaluating the integral, we have:

V = 2π [y^3/3] from 0 to 9

V = 2π [(9^3/3) - (0^3/3)]

V = 2π [(729/3) - 0]

V = 2π (243)

V = 486π

to learn more about integration.

https://brainly.com/question/31744185

#SPJ11

Answer:

Step-by-step explanation:

10x - 4y=24

7x -4y=12 multiply this equation by -1

-7x+4y=-12 we add it to the first and we get

3x=12

x=12/3=4

replace x with 3 in first equation

40-4y=24

40-24=4y

16=4y

y=4, x=4

Step-by-step explanation:

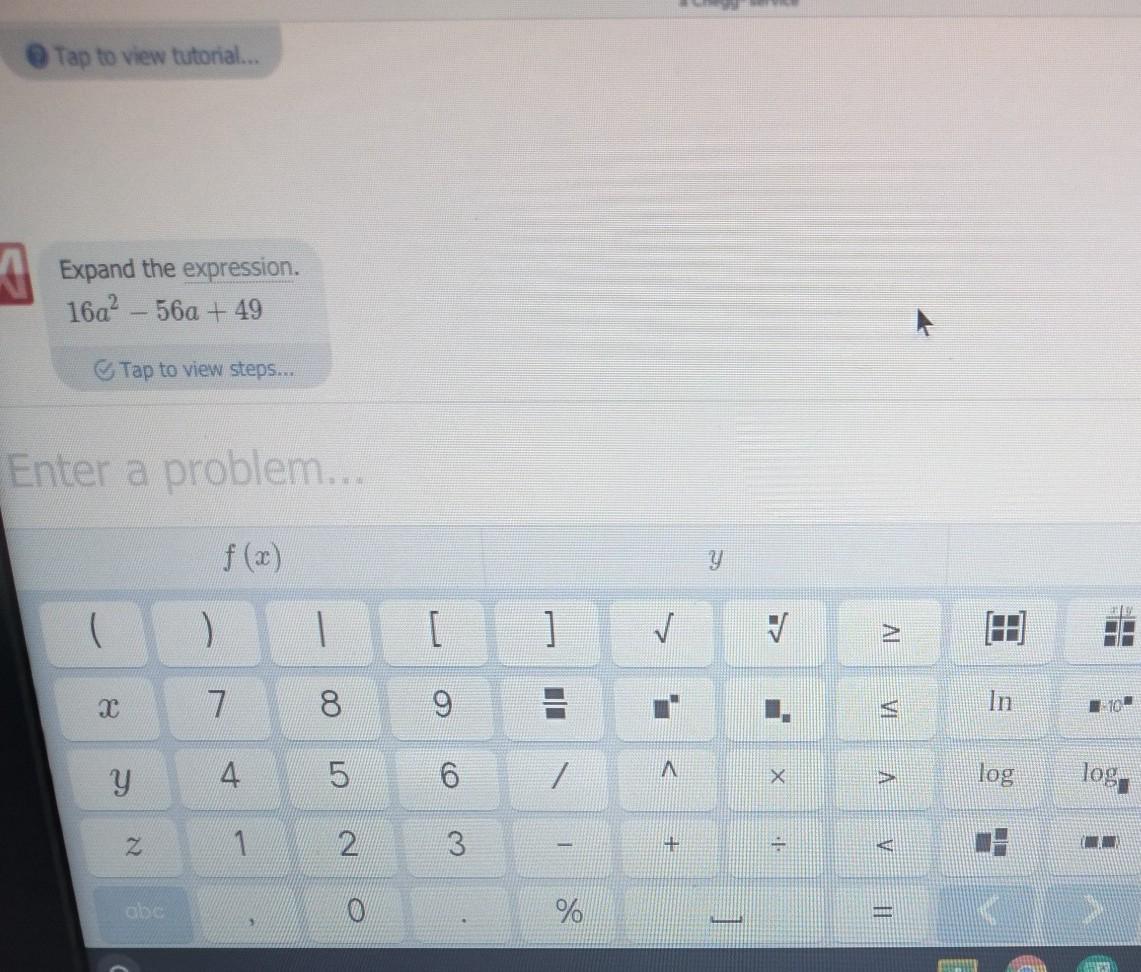
16a^2-56a+49

used the an app to find it