Solve the following system of equations by graphing each line.
x+y=4
3x+4y=10
*mark the solution with a point
Answers
Answer:
y = -2
x = 6
Step-by-step explanation:
hope it help
plz follow me
Related Questions
Rationalise the denominator and simplify 5 root 7/root 35
Answers
Answer:
Exact Form: Root 5
Decimal Form: 2.23606797...
Step-by-step explanation:
Multiply the numerator and denominator by the conjugate.
The simplified evaluation of the given expression would be √5 in exact form or 2.23606797... in decimal form.
What is a rational number?A rational number is defined as a numerical representation of a part of a whole that represents a fraction number.
It can be a/b of two integers, a numerator a, and a non-zero denominator b.
We have been the given expression as :
⇒ 5 √7/√35
∵ √35 = √7 ×√5
⇒ 5 √7/√(7×5)
Reduce the identical terms, and we get
⇒ 5 √7/(√7)×(√5)
⇒ 5 /√5
⇒ (√5 ×√5)/√5
∵ 5 = √5 ×√5
⇒ √5
Exact Form: √5
Decimal Form: 2.23606797...
Therefore, the simplified evaluation of the given expression would be √5 in exact form or 2.23606797... in decimal form.
Learn more about rational numbers here:
brainly.com/question/10354322
#SPJ2
find the value of tn–1,α/2 needed to construct a 95onfidence interval with sample size 7. round the answer to three decimal plac
Answers
The value of t(n-1, α/2) needed to construct a 95% confidence interval with a sample size of 7 is 2.447, rounded to three decimal places.
To find the value of t(n-1, α/2) needed to construct a 95% confidence interval with a sample size of 7, you need to follow these steps:
1. Identify the sample size (n): n = 7
2. Calculate degrees of freedom (df): df = n - 1 = 7 - 1 = 6
3. Determine the α value: Since it's a 95% confidence interval, α = 1 - 0.95 = 0.05
4. Divide α by 2 to get α/2: α/2 = 0.05 / 2 = 0.025
5. Consult a t-distribution table or use a calculator to find the t-score for the given df and α/2: t(6, 0.025)
By looking up the value in a t-distribution table or using a calculator, the t-score for 6 degrees of freedom and 0.025 is approximately 2.447.
So, the value of t(n-1, α/2) needed to construct a 95% confidence interval with a sample size of 7 is 2.447, rounded to three decimal places.
To know more about confidence interval, refer here:
https://brainly.com/question/24131141
#SPJ11
As men and women age, their risk of death increases. There are also health issues that can greatly impair older citizens' abilities to participate in activities and travel. Assume you are planning a 60th high school reunion and are wondering how many of the older adults would be able to attend. If you know that 24 women and 20 men are still alive, and are told that exactly 12 of them will attend, what is the probability that you would have exactly six men and exactly six women attend?
Answers
The probability of having exactly six men and six women attend the reunion can be calculated using the concept of probability and combinatorics.
To calculate the probability, we need to determine the number of favorable outcomes and the total number of possible outcomes. The number of favorable outcomes corresponds to the number of ways to select six men from a group of 20 and six women from a group of 24, which can be calculated using combinatorics.
The number of ways to choose six men from a group of 20 can be calculated as C(20, 6), which represents the number of combinations. Similarly, the number of ways to choose six women from a group of 24 can be calculated as C(24, 6).
The total number of possible outcomes, which is the denominator, can be calculated as C(44, 12), representing the number of ways to choose 12 attendees from the combined group of men and women.
By dividing the favorable outcomes by the total outcomes, we obtain the probability of having exactly six men and six women attend the reunion. Therefore, the probability can be calculated as [C(20, 6) * C(24, 6)] / C(44, 12).
Learn more about probability here:
https://brainly.com/question/32117953
#SPJ11
non-normality of the residuals from a regression can best be detected by looking at the residual plots against the fitted y values.truefalse
Answers
The statement that non-normality of the residuals from a regression can best be detected by looking at the residual plots against the fitted y values is a false statement.
We have a statement and we need to find out if it is true. A negative residual indicates that the model did not fit. This means that the error produced by the model is not the same between variables and observations (that is error is not random). The content is not regular, that is, the content is distributed regularly and we can conclude that the linear model is the appropriate model. If the subject sees a curvilinear pattern such as a U-shaped pattern, we can conclude that a linear pattern is not suitable and a non-linear pattern would be better. These charts are useful for identifying inconsistencies, inconsistencies in error variance, and inconsistencies. Two well-known tests for normality of residues are the Kolmogorov Smirnov test and the Shapiro-Wilk test. So, residual plot can't be used to check the non-normality of the residuals from a regression. Therefore, it is false statement.
For more information about residuals, visit :
https://brainly.com/question/9329287
#SPJ4
2. Tim started feeding his dog a new brand of dog food. After the first six
months, his dog has lost 2 pounds. After the next six months, his dog had
gained 2 pounds. What is his dog's net weight change after one year?
(Carefully match & submit final answer below).
Answers
Answer:
0
Step-by-step explanation:
he had lost and gained 2 pounds so he netheir gained nor lost pounds
Stella wnats to buy an atlas that costs 510, a novel that costs $8, and a comc that costs $6. She saved 516 from her pocket money. How much
more money does she need to save? What equation is correct?
Answers
Answer:
where are the equations
Step-by-step explanation:
m is how much more she needs to save
510+8+6=516+m
m=8
hi i need help for this question, what's the slope for this

Answers
Answer:
sorry for being late :P
Step-by-step explanation:
slope = rise / run
by inspection of the graph.. it looks like the line goes over 2 units and then up one unit so 1/2.... and b/c it's going up... like up hill.. it's positive...
+ 1/2 is the slope
Discuss how well you data on resistivity (obtained based on the Eqn. 3) agrees with the given values. Discuss the sources of experimental error.
Eqn.3) rho = (slope)A = (slope)Ï(d/2)2
Answers
Based on the equation 3, the data on resistivity agrees well with the given values. However, there may be some sources of experimental error that can affect the accuracy of the data. These sources of error which include Measurement, Human, Random errors.
1. Measurement errors: These can occur due to the inaccuracy of the measuring instruments used in the experiment. For example, if the instrument used to measure the slope is not calibrated correctly, it can lead to inaccurate results.
2. Human errors: These can occur due to the mistakes made by the experimenter. For example, if the experimenter does not read the measurements correctly, it can lead to inaccurate results.
3. Random errors: These can occur due to the uncontrollable factors that can affect the experiment. For example, if there is a change in temperature during the experiment, it can affect the resistivity of the material.
Overall, it is important to consider these sources of experimental error in order to obtain accurate data on resistivity.
Know more about resistivity here:
https://brainly.com/question/17010736
#SPJ11
Translate this sentence into an equation. Fifteen more than z times six is y times two minus eleven.
Answers
Answer:
15 > Z * 6 = Y * 2 - 11
Kira is a lovable dog who is full of energy. Her owner thought it would be fun to train her by throwing a frisbee for her to catch. When the frisbee is thrown, it follows a parabolic path that is modeled by the function h(t) = – 0.07t2 + 0.007t + 5. How many seconds will it take for the frisbee to hit the ground?
–8.5 seconds
–5.0 seconds
5.0 seconds
8.5 seconds
Answers
Solving the quadratic equation, we will see that the frisbee will hit the ground after 8.5 seconds.
How many seconds will it take for the frisbee to hit the ground?
We know that the height of the frisbee is modeled by the quadratic equation:
h(t) = -0.07*t^2 + 0.007*t + 5
The frisbee will hit the ground when h(t) = 0, so we just need to solve the quadratic equation:
0 = -0.07*t^2 + 0.007*t + 5
We can just use the quadratic formula to get the solutions:
\(t = \frac{-0.007 \pm \sqrt{0.007^2 - 4*(-0.07)*5} }{2*-0.07} \\\\t = \frac{-0.007 \pm 1.18 }{-0.14}\)
We only care for the positive solution, which is:
t = (-0.007 - 1.18)/-0.14 = 8.5
So the frisbee will hit the ground after 8.5 seconds.
Learn more about quadratic equations:
https://brainly.com/question/1214333
#SPJ1
I need someone to help
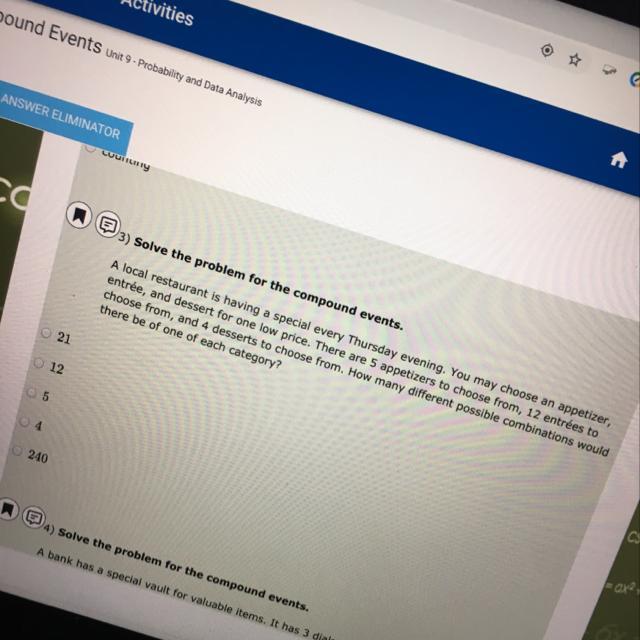
Answers
Answer:
240
Step-by-step explanation:
You must multiply 4 by 5, which will give you 20. Then multiply 20 by 12, which will give you the final result of 240.
If th ere are 10,000 stu dents enrolled in the college an d the institution is funded at the rate of $300 per student unit. At the 95 % confidence level, estimate the interval th at covers the total funding of the college . for full credit, round the answer to TWO decimal places!
Answers
Complete question :
A college is funded depending on the mean number of credit units a student takes. A survey involving 16 students yielded the mean number 12 units with a standard deviation 3 units. Assume normal population
If th ere are 10,000 stu dents enrolled in the college an d the institution is funded at the rate of $300 per student unit. At the 95 % confidence level, estimate the interval th at covers the total funding of the college . for full credit, round the answer to TWO decimal places!
Answer: (35823600 , 36176400)
Step-by-step explanation:
Mean number of units = 12
Standard deviation = 3
Number of samples (n) = 10000
Fund rate = 300
Mean = 10000 * 300 * 12 = 36000000
Standard deviation = 10000^0.5 * 300 * 3 = 90000
Fir 95% C. I
Z0.05 = 1.96
Interval = mean ± 1.96 * SE
(36000000 - 1.96*90000) ; (36000000 + 1.96*90000)
(35823600 , 36176400)
Jim had three more than twice the number of doughnuts as Jane. Jim had 15 doughnuts. What is the equation to find out how many doughnuts Jane had?
Answers
Answer:
x = 15 * 2 + 3
Step-by-step explanation:
hope this helps!
at what two distances from the base of the stump after it jumped was the frog 4.3 ft above the ground
Answers
Let's assume that the initial velocity of the frog is zero. Using the kinematic equations of motion, the height of the frog at any time can be determined as given below.
s = ut + 1/2at², where s is the distance, u is the initial velocity, a is the acceleration, and t is the time taken.
Here, s = 1.24 m - h (since the initial height of the frog is 1.24 m), u = 0 (since the initial velocity of the frog is zero),
a = -9.8 m/s² (the acceleration due to gravity), and t is the time taken. Let's assume that the frog reaches the ground after t seconds. Hence, 4.3 ft = 1.3 m
The height of the frog when it is at 4.3 ft above the ground can be written as: 1.3 m = -4.9t² + 1.24 m
By solving the above equation for time, we get, t = 0.575 s. The time taken by the frog to reach the ground is 0.575 seconds. Therefore, the horizontal distance traveled by the frog can be determined as: d = vt, where d is the horizontal distance, v is the velocity of the frog, and t is the time taken. v can be determined using the formula:
v = u + at. Here, u = 0 (since the initial velocity of the frog is zero), a = -9.8 m/s² (the acceleration due to gravity), and t is the time taken. Hence, v = -9.8 x 0.575 = -5.63 m/s. Thus, the horizontal distance traveled by the frog is given by:
d = vt = -5.63 x 0.575 = -3.24 m. Since the distance cannot be negative, the horizontal distance traveled by the frog is 3.24 m. Using the same method, we can determine the horizontal distance traveled by the frog in the other direction. Therefore, the two distances from the base of the stump where the frog was 4.3 feet above the ground after it jumped are 3.24 m and -3.24 m.
To know more about velocity refer here:
https://brainly.com/question/17127206
#SPJ11
Put the times in chronological order

Answers
Answer:
b,c,e,d,a,f
Step-by-step explanation:
Factor the following polynomial completely x2 - 3x - 10

Answers
Answer:
the answer is (x-5) (x+2)
A computer company claims that the lifespan of its batteries is 2. 7 years. The population standard deviation is 0. 85 years. A sample of 25 batteries was tested, and their mean lifespan was 3. 1 years. Using a 95% confidence level, determine if the company's claim is correct. Reject the null hypothesis. There is enough evidence to oppose the company's claim. Fail to reject the null hypothesis. There is enough evidence to oppose the company's claim. Reject the null hypothesis. There is not enough evidence to oppose the company's claim. Fail to reject the null hypothesis. There is not enough evidence to oppose the company's claim
Answers
Using the z-distribution, as we have the standard deviation for the population, it is found that the correct decision is given by:
Reject the null hypothesis. There is enough evidence to oppose the company's claim.
What are the hypothesis tested?At the null hypothesis, it is tested if the mean is of 2.7 years, that is:
\(H_0: \mu = 2.7\)
At the alternative hypothesis, it is tested if the mean is different of 2.7 years, hence:
\(H_1: \mu \neq 2.7\)
What is the test statistic?The test statistic is given by:
\(z = \frac{\overline{x} - \mu}{\frac{\sigma}{\sqrt{n}}}\)
The parameters are:
\(\overline{x}\) is the sample mean.\(\mu\) is the value tested at the null hypothesis.\(\sigma\) is the standard deviation of the sample.n is the sample size.In this problem, the values of the parameters are given by:
\(\overline{x} = 3.1, \mu = 2.7, \sigma = 0.85, n = 25\)
Hence, the value of the test statistic is given by:
\(z = \frac{\overline{x} - \mu}{\frac{\sigma}{\sqrt{n}}}\)
\(z = \frac{3.1 - 2.7}{\frac{0.85}{\sqrt{25}}}\)
\(z = 2.35\)
What is the decision?Considering a two-tailed test, as we are testing if the mean is different of a value, with a significance level of 0.05, the critical value is of \(|z^{\ast}| = 1.96\).
Since the absolute value of the test statistic is greater than the critical value, the correct decision is:
Reject the null hypothesis. There is enough evidence to oppose the company's claim.
More can be learned about the z-distribution at https://brainly.com/question/16313918
Answer:
Reject the null hypothesis. There is enough evidence to oppose the company's claim.
Step-by-step explanation:
The test statistic needs to fall within the rejection regions that are above and below the critical z-score associated with a 5% level of significance in order to reject the null hypothesis. Otherwise, we will fail to reject the null hypothesis.
what is the range of the function f(x)= -(x+3)^2+7
Answers
Answer:
thats the answer to rhjs problem

pls help will give brainliest

Answers
Hope this helps
The greatest common factor (GCF) of two or more numbers is the _[blank 1]_ they have in common. To find the GCF, find the _[blank 2]_ of both numbers and identify their common _[blank 3]_s. Then, multiply them together to get the GCF
Answers
The correct answer is GCF.
Description: GCF stands for Greatest Common Factor. By definition, it is the greatest of all divisors of two or more numbers.
GCF stands for Greatest Common Divisor. By definition, it is the greatest of all divisors of two or more numbers.
To find the GCF, first find the prime factorization of any number. This is a list of prime factors that are multiplied to get each number. Then take the common factors and multiply them together. This will give you the GCF.
The GCF (greatest common divisor) of two or more numbers is the largest number among all the common divisors of the given numbers. The GCD of two natural numbers x and y is the largest number that divides both x and y. There are three common ways to compute the GCF: division, multiplication, and prime factorization.
The GCF (Greatest Common Divisor) of two or more numbers is the largest number among all the common divisors of the given numbers. The GCD of two natural numbers x and y is the largest number that divides both x and y. There are three common ways to compute the GCF: division, multiplication, and prime factorization.
Example: Find the greatest common divisor of 18 and 27.
Solution:
First line up the divisors of 18 and 27, then find the common divisor.
Divisors of 18: 1, 2, 3, 6, 9, 18
Divisors of 27: 1, 3, 9, 27
Common divisors of 18 and 27 are 1, 3, 9. , where 9 is the largest (greatest) number. So the GCF of 18 and 27 is 9. This is written as: GCF(18, 27) = 9. Therefore, the greatest common divisor is also called the greatest common divisor (or GCD). In the example above, the greatest common divisor (GCD) of 18 and 27 is 9, which can be written as
Therefore, GCD(18, 27) = 9
Learn more about Greatest Common Factor:
https://brainly.com/question/29584814
#SPJ4
I just need an explanation for this.

Answers
Answer:
This image is a graph of a normal distribution, also known as a Gaussian distribution. The normal distribution is a common probability distribution that has a bell-shaped or symmetrical curve. The graph shows the probability density function of a normal distribution with a mean (μ) of 0 and a standard deviation (σ) of 1.
In a normal distribution, the mean is the central value and the standard deviation measures the spread of the distribution. The standard deviation of 1 in this graph indicates that most of the data values are within 1 standard deviation from the mean. The curve of the distribution shows that the highest point (peak) is at the mean (0) and that the probability density decreases as we move away from the mean in either direction.
The axis on the left side of the graph represents the probability density, which is the probability per unit of measurement. The area under the curve represents the total probability, which is equal to 1. The x-axis represents the range of values that the random variable can take.
Overall, this graph is a useful tool for understanding the properties of a normal distribution and for making statistical inferences or predictions about a population based on a sample of data.
Find the inverse of the following
F(x)=-3/4x+6
Answers
Answer:
\( {f}^{ - 1} (x)= - \frac{4}{3} x + 8\)
Step-by-step explanation:
\(f(x) = - \frac{3}{4} x + 6\)
\(y = - \frac{3}{4} x + 6\)
\(x = - \frac{3}{4} y + 6\)
\( - \frac{3}{4} y + 6 = x\)
\( - 3y + 24 = 4x\)
\( - 3y = 4x - 24\)
\(\boxed{\green{ {f}^{ - 1} (x)= - \frac{4}{3} x + 8}}\)
Read the problem below and find the solution.
Draw a diagram on your own paper to help solve it.
Sharon drops a rubber ball from a height of 160 feet. Every bounce sends the ball half as high as it was before. What is the total vertical (up and down) distance the ball has traveled, from the moment it is dropped to the moment it hits the floor for the fourth time?
Answers
Answer:
440 ftStep-by-step explanation:
Ball dropped and we start count from that moment:
160 ft down80 ft up and down40 ft up and down20 ft up and down, endTotal distance:
160 + 80*2 + 40*2 + 20*2 = 440 ftA dental student is conducting a study on the number of people who visit their dentist regularly. Of the 520 people surveyed, 312 indicated that they had visited their dentist within the past year.Find the population proportion, as well as the mean and standard deviation of the sampling distribution for samples of size n=60.Round all answers to 3 decimal places.p=Up=Op=
Answers
Answer:
Step-by-step explanation:
312/520 equals 60%
other people 40%
orm the indicated operations and simplify the expressi (3(3x-2)^((1)/(3))-(x-1)(3x-2)^(-(2)/(3)))/((3x-2)^((2)/(3)))
Answers
The simplified expression is (8x-7) / ((3x-2)\(^{((2)/(3)))}\).
To simplify the expression (3(3x-2)\(^{((1)/(3))}\) - (x-1)(3x-2)\(^{(-(2)/(3)))/((3x-2)}\)\(^{((2)/(3)))}\) first we need to perform the indicated operations.
1. Using the power rule, we can rewrite (3x-2)\(^{((1)/(3))}\) as 3x-2 and (3x-2)^(-(2)/(3)) as 1/(3x-2).
Therefore, the expression can be rewritten as (3(3x-2) - (x-1)/(3x-2)) / ((3x-2)\(^{((2)/(3)))}\).
2.Now, using the distributive property, we can simplify the expression to (9x-6-(x-1)) / ((3x-2)\(^{((2)/(3)))}\).
Finally, simplifying further, we get the expression (8x-7) / ((3x-2)\(^{((2)/(3)))}\).
Learn more about simplified expression here:
https://brainly.com/question/403971#
#SPJ11
I need it soon thanks

Answers
= 3 × 4
= 3 × 2²
x = 1
2.Cross multiplication= 3 × 15 = 5x
= 45 /5 = x
= 9 = x
3. 14, 35, 424. 7 , 35. 572, 527, 725, 7526. a ÷ 2 = a/27. a.2 = 2a8. a² = a . a9. a + a + a = 3a10. 0a = 0Answer:
Step-by-step explanation:
1. 4
2. 9
3. 7 ,14 , 21 , 28 ,35 , 42 , 49
4. 7 , 3
5. 527 , 752 , 572 , 725
6. 6 = d ( a / 2 )
7 = e ( 2a )
8 = a ( a * a )
9 = b ( 3a )
10 = c ( 0 )
Carmen uses 4 centimeters of tape for every present she wraps. How many presents did
Carmen wrap if she used 48 centimeters of tape?
Answers
Answer:
12
Step-by-step explanation:
4 centimeters for each present and she used 48 centimeters of tape, so u would take 48 divided by 4 and get 12
Find the approximate area under the graph of (x)=1/x^2f over the interval [2, 4] using four equal subintervals (n = 4) and the right endpoint method.Select one:a.) 0.3014b.) 0.2076c.) 0.4540d.) 0.3521
Answers
To approximate the area under the graph of f(x) = 1/x^2 over the interval [2, 4] using four equal subintervals and the right endpoint method, we can use the following formula:
Approximate Area = Δx * [f(x1) + f(x2) + f(x3) + f(x4)]
where Δx is the width of each subinterval and xi represents the right endpoint of each subinterval.
In this case, the interval [2, 4] is divided into four equal subintervals, so Δx = (4 - 2) / 4 = 0.5.
Now, let's evaluate the function at the right endpoints of the subintervals:
f(2.5) = 1/(2.5)^2 = 0.16
f(3) = 1/(3)^2 = 0.1111
f(3.5) = 1/(3.5)^2 = 0.0816
f(4) = 1/(4)^2 = 0.0625
Substituting these values into the formula:
Approximate Area = 0.5 * [0.16 + 0.1111 + 0.0816 + 0.0625]
Approximate Area = 0.5 * 0.4152
Approximate Area = 0.2076
Therefore, the approximate area under the graph of f(x) = 1/x^2 over the interval [2, 4] using four equal subintervals and the right endpoint method is approximately 0.2076.
learn more about graph here
https://brainly.com/question/10712002
#SPJ11
A company buys machinery for $500000 and pays it off by 20 equal six-monthly instalments, the first payment being made six months after the loan is taken out. If the interest rate is 12%pa, compounded monthly, how much will each instalment be?
Answers
Each installment will be approximately $15,280.55.
To calculate the equal six-monthly installment, we can use the formula for the present value of an annuity.
Principal amount (P) = $500,000
Interest rate (r) = 12% per annum = 12/100 = 0.12 (compounded monthly)
Number of periods (n) = 20 (since there are 20 equal six-monthly installments)
The formula for the present value of an annuity is:
\(P = A * (1 - (1 + r)^(-n)) / r\)
Where:
P = Principal amount
A = Equal installment amount
r = Interest rate per period
n = Number of periods
Substituting the given values into the formula, we have:
$500,000 = \(A * (1 - (1 + 0.12/12)^(-20)) / (0.12/12)\)
Simplifying the equation:
$500,000 = A * (1 - (1.01)^(-20)) / (0.01)
$500,000 = A * (1 - 0.6726) / 0.01
$500,000 = A * 0.3274 / 0.01
$500,000 = A * 32.74
Dividing both sides by 32.74:
A = $500,000 / 32.74
A ≈ $15,280.55
Therefore, each installment will be approximately $15,280.55.
Learn more about Principal amount here:
https://brainly.com/question/30163719
#SPJ11
find the value of given expression
\( \sqrt{32 + 4} \)
Answers
Answer:
± 6
Step-by-step explanation:
Given
\(\sqrt{32+4}\)
= \(\sqrt{36}\)
= + 6 or - 6 , that is ± 6
Answer:
√{32+4}=√36=√6²=±6 is your answer