The Science Club went on a field trip. The members paid $60 for transportation plus $15 per ticket to the planetarium.
Choose an expression to represent the total cost for n members of the club.
Answers
Answer:
60 +(n x 15) = total cost
Step-by-step explanation:
Related Questions
Write the composite function in the form f(g(x)). [Identify the inner function u = g(x) and the outer function y = f(u).] (Use non-identity functions for f(u) and g(x).) y = et + 9 (f(u), g(x)) = dy Find the derivative dx dy dx
Answers
To write the composite function in the form f(g(x)), we need to identify the inner function u = g(x) and the outer function y = f(u).
In this case, the given function is y = et + 9. We can see that the inner function is u = g(x) = t, and the outer function is y = f(u) = et + 9.
To find the derivative \(\frac{dy}{dx}\), we can use the chain rule. The chain rule states that if we have a composite function y = f(g(x)), then the derivative \(\frac{dy}{dx}\) is given by \(\frac{dy}{dx} = \frac{dy}{du} \cdot \frac{du}{dx}\).
In this case, we have y = f(u) = et + 9, where u = g(x) = t.
First, let's find the derivative of y with respect to u, \(\frac{dy}{du}\). Since f(u) = et + 9, the derivative \(\frac{dy}{du}\) is simply f'(u), which is \(e^u\).
Next, let's find the derivative of u with respect to x, \(\frac{du}{dx}\). Since u = t, which is a variable separate from x, the derivative \(\frac{du}{dx}\) is zero.
Finally, we can apply the chain rule by multiplying \(\frac{dy}{du}\) and \(\frac{du}{dx}\):
\(\frac{dy}{dx} = \left(\frac{dy}{du}\right) \cdot \left(\frac{du}{dx}\right) = (e^u) \cdot 0 = 0\)
Therefore, the derivative \(\frac{dy}{dx}\) of the composite function y = et + 9 is zero.
To know more about Function visit-
brainly.com/question/31062578
#SPJ11
An equation was created for the line of best fit from actual enrollment data. It was used to predict the dance studio enrollment values shown in the table:
Enrollment Month
January February March April May June
Actual 500 400 550 550 750 400
Predicted 410 450 650 650 600 450
Residual 90 −50 −100 −100 150 −50
Analyze the data. Determine whether the equation that produced the predicted values represents a good line of best fit. (1 point)
No, the equation is not a good fit because the sum of the residuals is a large number.
No, the equation is not a good fit because the residuals are all far from zero.
Yes, the equation is a good fit because the residuals are not all far from zero.
Yes, the equation is a good fit because the sum of the residuals is a small number.
Answers
The correct option regarding the linear regression equation is:
No, the equation is not a good fit because the sum of the residuals is a large number.
How to find the equation of linear regression using a calculator?To find the equation, we need to insert the points (x,y) in the calculator.
In possession of the equation, the residuals are given by the difference of the predicted values(with the equation) and the actual values.
The model represents a good fit if the sum of the residuals is close to 0.
For this problem, the sum of the residuals is given by:
90 - 50 - 100 - 100 + 150 - 50 = -60.
The sum is a large number, hence the model is not a good fit.
More can be learned about linear regression at https://brainly.com/question/22992800
#SPJ1
5184 divided by 27
JUST ANSWER
Answers
Answer:
\(5184 \div 27 = 192\)
Step-by-step explanation:
Hope this helps you !!pla shop mathematics

Answers
The number of trees more than 10m tall but not more than 20m tall is 18 trees.
How many of the trees are more than 10m tall but not more than 20m tall?0 < h ≤ 5 = 5
height greater than 0m less than or equal to 5m
5 < h ≤ 10 = 9
height greater than 5m less than or equal to 10m
10 < h ≤ 15 = 13
height greater than 10m less than or equal to 15m
15 < h ≤ 20 = 5
height greater than 15m less than or equal to 20m
20 < h ≤ 25 = 1
height greater than 20m less than or equal to 25m
The number of trees that are more than 10m tall but not more than 20m tall are;
10 < h ≤ 15 = 13
15 < h ≤ 20 = 5
So,
13 + 5 = 18 trees
Therefore, the total number of trees which are 10m tall but not more than 20m tall is 18 trees.
Read more on inequality:
https://brainly.com/question/25275758
#SPJ1
please help me with math

Answers
pls help asap if you can!!!!

Answers
The statement that proves that angle XWY is equal to angle ZYW is
A. If two parallels are cut by a transverse, then alternate interior angles are congruent
What are alternate interior anglesAlternate interior angles are a pair of angles that are formed on opposite sides of a transversal line when two parallel lines are intersected by the transversal.
When a transversal intersects two parallel lines, it creates eight angles. Among these angles, the alternate interior angles are located on the inside of the parallel lines and on opposite sides of the transversal.
In a parallelogram, the two opposite sides are parallel to each other hence the line crossing them will lead to formation of alternate interior angles
Learn more about alternate interior angles at
https://brainly.com/question/20344743
#SPJ1
1) find a div m and a mod m when
a) a= -111, m = 99
b) a= -9999, m= 101
c) a= 10299, m=999.
d) a= 123456, m= 1001.
2) find the value of ( 893 mod 79)4 mod 26
Answers
a) Using the formula a = q * m + r, we have:
-111 = (-2) * 99 + 87
Therefore, a div m = -2 and a mod m = 87.
b) Using the same formula:
-9999 = (-99) * 101 + 12
So, a div m = -99 and a mod m = 12.
c)
10299 = 10 * 999 + 369
Thus, a div m = 10 and a mod m = 369.
d)
123456 = 123 * 1001 + 733
Therefore, a div m = 123 and a mod m = 733.
We can solve this by using the modulo arithmetic property that states that (a^b) mod m = ((a mod m)^b) mod m. Applying this property, we have:
(893 mod 79)4 mod 26 = (12^4) mod 26 = 20736 mod 26 = 8. Therefore, (893 mod 79)4 mod 26 = 8.
Visit here to learn more about formula brainly.com/question/20748250
#SPJ11
The formula to convert °F to °C is C = C equals StartFraction 5 Over 9 EndFraction left-parenthesis F minus 32 right-parenthesis.(F – 32).
Convert 50°C to °F.
10°F
20°F
122°F
132°F
Answers
The temperature in degree Fahrenheit is (c) 122
The function is given as:
\(C = \frac 59(F - 32)\)
When the temperature is 50 degrees Celsius, it means that:
C =50
Substitute 50 for C in \(C = \frac 59(F - 32)\)
\(50 = \frac 59(F - 32)\)
Multiply through by 9
\(450 = 5(F - 32)\)
Divide both sides by 5
\(90 = F - 32\)
Add 32 to both sides
\(F = 90 +32\)
\(F = 122\)
Hence, the temperature in degree Fahrenheit is (c) 122
Read more about unit conversions at:
https://brainly.com/question/9510506
At the library, Newton borrows 8 books and Descartes borrows 4 books. 7 of their books are nonfiction. The rest are fiction. How many fiction books did they borrow together?
Answers
5
First order equations include linear equations. In the coordinate system, the linear equations are defined for lines. A linear equation in one variable is one in which there is a homogeneous variable of degree 1 (i.e., only one variable). Multiple variables may be present in a linear equation. Linear equations in two variables, for example, are used when a linear equation contains two variables. Examples of linear equations include 2x - 3 = 0, 2y = 8, m + 1 = 0, x/2 = 3, and 3x - y + z = 3.
Total books borrowed = 8+4 = 12
No. of non - fiction books = 7
No. of fiction books = 12 -7
= 5
To learn more about linear equation , refer to https://brainly.com/question/26310043
#SPJ9
Find all points where the tangent line is horizontal: x
2
+
x
y
+
y
2
=
1
?
Answers
The points where the tangent line is horizontal on the curve \(x^2 + xy + y^2 = 1\) are (√(1/3), -2√(1/3)) and (-√(1/3), 2√(1/3)).
First, let's differentiate the equation implicitly with respect to x:
\(d/dx (x^2 + xy + y^2) = d/dx\) (1)
Using the product rule and chain rule:
2x + x(dy/dx) + y + 2y(dy/dx) = 0
Rearranging the equation and factoring out dy/dx:
(dy/dx)(x + 2y) = -2x - y
To find the points where the tangent line is horizontal, we set dy/dx equal to zero:
dy/dx = 0
This leads to the equation:
0(x + 2y) = -2x - y
0 = -2x - y
y = -2x
Now we substitute this expression for y back into the original equation to find the corresponding x-values:
\(x^2 + x(-2x) + (-2x)^2 = 1\\x^2 - 2x^2 + 4x^2 = 1\\3x^2 = 1\\x^2 = 1/3\)
Taking the square root of both sides:
x = ± √(1/3)
Therefore, the x-values where the tangent line is horizontal are x = √(1/3) and x = -√(1/3).
Substituting these x-values back into the equation y = -2x, we find the corresponding y-values:
For x = √(1/3), y = -2√(1/3)
For x = -√(1/3), y = 2√(1/3)
So, the points where the tangent line is horizontal are (√(1/3), -2√(1/3)) and (-√(1/3), 2√(1/3)).
Complete Question:
Find all points where the tangent line is horizontal: \(x^2 + xy + y^2 = 1\).
To know more about tangent line, refer here:
https://brainly.com/question/31617205
#SPJ4
the number of minutes needed to solve an exercise set of variation problems varies directly as the number of problems and inversely as the number of people working on the solutions. it takes 4 people 36 minutes to solve 18 problems. how many minutes will it take 6 people to solve 42 problems.
Answers
The number of minutes needed to solve an exercise set of variation problems varies directly with the number of problems and inversely with the number of people working on the solutions. it will take 6 people approximately 24 minutes to solve 42 problems based on the given variation relationship.
Let's denote the number of minutes needed to solve the exercise set as "m," the number of problems as "p," and the number of people as "n." According to the given information, we have the following relationships: m ∝ p (direct variation) and m ∝ 1/n (inverse variation).
We can express these relationships using proportionality constants. Let's denote the constant of direct variation as k₁ and the constant of inverse variation as k₂. Then we have the equations m = k₁p and m = k₂/n.
In the initial scenario, with 4 people solving 18 problems in 36 minutes, we can substitute the values into the equations to find the values of k₁ and k₂. From m = k₁p, we have 36 = k₁ * 18, which gives us k₁ = 2. From m = k₂/n, we have 36 = k₂/4, which gives us k₂ = 144.
Now, we can use these values to determine how many minutes it will take 6 people to solve 42 problems. Substituting n = 6 and p = 42 into the equation m = k₂/n, we get m = 144/6 = 24. Therefore, it will take 6 people approximately 24 minutes to solve 42 problems based on the given variation relationship.
Learn more about variation relationship here:
https://brainly.com/question/15210301
#SPJ11
Find the area of the shaded region.

Answers
Simplify the expressions
(24m^-2n)(1/4mn)
(4a^-2)^4
(9ab^-5)^-2
Answers
The simplified expressions are given below\(:(24m⁻²n)(1/4mn) = 6mn⁵/²(4a⁻²)⁴ = 256/a⁸(9ab⁻⁵)⁻² = b¹⁰/81a²\)
1. \((24m⁻²n)(1/4mn\))
Multiplying the given expression, we get:\((24m⁻²n)(1/4mn) = 6mn⁵/²2. (4a⁻²)⁴.\)
Raising \(4a⁻²\) to the power of 4, we get:
\((4a⁻²)⁴ = 4⁴ × (a⁻²)⁴= 256/a⁸3. (9ab⁻⁵)⁻²\)
Raising \(9ab⁻⁵\) to the power of -2, we get: \((9ab⁻⁵)⁻² = 1/(9ab⁻⁵)²= 1/81a²b¹⁰.\)
The simplified expressions are\((24m⁻²n)(1/4mn) = 6mn⁵/², (4a⁻²)⁴ = 256/a⁸ and (9ab⁻⁵)⁻² = b¹⁰/81a².\)
To know more about simplified expressions :
brainly.com/question/29003427
#SPJ11
Consider the data below: 6278 The mode is: A. 54 B. 80 C. 62 D. 78 Clear my choice
Answers
None of the options A, B, C, or D are correct for the mode.
In the given data set {6, 2, 7, 8}, the mode refers to the value(s) that appear most frequently. To find the mode, we look for the value(s) that occur with the highest frequency.
In this case, each value in the data set occurs only once, and there are no repeated values.
Therefore, there is no value that appears more frequently than others. As a result, the data set does not have a mode.
The options provided (A. 54, B. 80, C. 62, D. 78) are not applicable because none of these values are present in the given data set.
In summary, for the data set {6, 2, 7, 8}, there is no mode since all values occur with the same frequency of one.
Therefore, none of the options A, B, C, or D are correct for the mode.
Learn more about mode from the given link
https://brainly.com/question/14532771
#SPJ11
a postal worker counts the number of complaint letters received by the united states postal service in a given day. identify the type of data collected.
Answers
When a postal worker counts the number of complaint letters received by the united states postal service in a given day, the type of data collected is quantitative.
How to explain the dataQuantitative data is data that can be measured and expressed in numbers. In this case, the number of complaint letters received by the United States Postal Service in a given day can be measured and expressed as a number.
Qualitative data, on the other hand, is data that cannot be measured or expressed in numbers. For example, the contents of the complaint letters would be qualitative data.
Learn more about data on
https://brainly.com/question/26711803
#SPJ1
utomobile trips there are major roads from city to city and major roads from city to city . how many different trips can be made from city to city passing through city ?
Answers
There are 8 different trips that can be made from City X to City Z, passing through City Y.
To find the number of different trips that can be made from City X to City Z, passing through City Y, we can use the multiplication principle of counting.
First, we need to choose one of the 2 major roads from City X to City Y. Then, for each of these roads, there are 4 major roads from City Y to City Z, and we need to choose one of these roads.
By the multiplication principle of counting, the total number of different trips from City X to City Z, passing through City Y, is the product of the number of choices at each stage. Thus, we get:
Number of different trips = Number of roads from City X to City Y x Number of roads from City Y to City Z
= 2 x 4
= 8
This calculation shows how the multiplication principle of counting can be used to find the total number of possible outcomes in a multi-stage process where the number of choices at each stage is known.
To learn more about outcomes click on,
https://brainly.com/question/14796795
#SPJ4
Complete question is:
Automobile Trips. There Are 2 Major Roads From City X To City Y And 4 Major Roads From City Y To City Z. How Many Different trips can be made from City X to City Z, passing through City Y?
Let S be the part of the plane 2x+4y+z=2 which lies inthe first octant, oriented upward. Find the flux of the vectorfield
F=1i+1j+2k across the surface S
Answers
The flux of the vector-field F = 1i + 1j + 2k across the surface S is 2. We find out the flux of the vector-field using Green's Theorem.
Define Green's Theorem.Flux form of Green's Theorem for the given vector-field
φ = ∫ F.n ds
= ∫∫ F. divG.dA
Here G is equivalent to the part of the plane = 2x+4y+z = 2.
and given F = 1i + 1j + 2k
divG = div(2x+4y+z = 2) = 2i + 4j + k
Flux = ∫(1i + 1j + 2k) (2i + 4j + k) dA
φ = ∫ (2 + 4 + 2)dA
= 8∫dA
A = 1/2 XY (on the given x-y plane)
2x+4y =2
at x = 0, y = 1/2
y = 0, x = 1
1/2 (1*1/2) = 1/4
Therefore flux = 8*1/4 = 2
φ = 2.
To know more details about Green's Theorem visit:
https://brainly.com/question/27549150
#SPJ4
Consider the sequence $$1,3,4,9,10,12,13,\ldots,$$ which consists of every positive integer that can be expressed as a sum of distinct powers of $3$. What is the $75^{\text{th}}$ term of this sequence
Answers
To find the 75th term of the sequence consisting of positive integers that can be expressed as a sum of distinct powers of 3. Thus, the 75th term of the sequence is $111$.
To find the 75th term of the sequence consisting of positive integers that can be expressed as a sum of distinct powers of 3, we can use the base-3 (ternary) numeral system. The 75th term can be represented as the 74th number in base-3 without a digit 2 (as it will require subtraction to form distinct powers of 3).
The 74th number in base-10 is represented as $74_{10}$, which when converted to base-3 is $2202_3$. Since we need to avoid the digit 2, we can carry out the operation similar to addition with carry-over: $2202_3 + 1111_3 = 10310_3$.
Now, we can convert $10310_3$ back to base-10: $(1 \times 3^4) + (0 \times 3^3) + (3 \times 3^2) + (1 \times 3^1) + (0 \times 3^0) = 81 + 0 + 27 + 3 + 0 = 111$.
Thus, the 75th term of the sequence is $111$.
Visit here to learn more about positive integers : https://brainly.com/question/26051073
#SPJ11
Find the radius and interval of convergence of Σn=1 (-1)n/n23n.xn
Answers
The radius of convergence for the series Σ(-1)^n / n × 2³n × x^n is 1/8, and the interval of convergence is (-1/8, 1/8).
To find the radius and interval of convergence for the series Σ(-1)^n / n × 2×n × x^n, we can use the Ratio Test. Apply the Ratio Test. We take the limit as n approaches infinity of the absolute value of the ratio of consecutive terms:
lim (n → ∞) |(a_(n+1)) / a_n|
In this case, a_n = (-1)^n / n × 2^(3n) × x^n.
Write down a_(n+1) and a_n
a_(n+1) = (-1)^(n+1) / (n+1) × 2^(3(n+1)) × x^(n+1)
a_n = (-1)^n / n × 2^(3n) × x^n
Calculate the limit
lim (n → ∞) |((-1)^(n+1) / (n+1) × 2^(3(n+1)) × x^(n+1)) / ((-1)^n / n × 2^(3n) × x^n)|
Simplify the expression
|((-1)^(n+1) × n × 2^(3(n+1)) × x^(n+1)) / ((-1)^n × (n+1) × 2^(3n) × x^n)|
Cancel out terms and simplify further
|-1 × 2³ × x| = |2³ × x|
Apply the Ratio Test criterion
For the series to converge, the limit should be less than 1:
|2³ × x| < 1
|8x| < 1
Solve for x to find the interval of convergence
-1/8 < x < 1/8
Determine the radius of convergence
The radius of convergence (R) can be found as:
R = 1/8
In conclusion, the radius of convergence for the series Σ(-1)^n / n × 2^3n × x^n is 1/8, and the interval of convergence is (-1/8, 1/8).
More on radius of convergence: https://brainly.com/question/30167798
#SPJ11
A set of three identical small hollow balls have a total volume equal to that of a single
larger hollow ball of radius 3cm. Does it take more paint to paint the large ball or the 3
5/26/23, 1:20 PM
15 pts
QUIZ. 00.00 VOTURIC
smaller balls and how much more (in sq cm)? Show your work for full credit.
Answers
Answer:
Difference = Surface Area_small - Surface Area_large ≈ 63.72 cm^2 - 85.39 cm^2 ≈ -21.67 cm^2
Step-by-step explanation:
Radius of the larger hollow ball (r_outer) = 3 cm
For the smaller hollow balls, since they are identical, we assume that the outer and inner radii are the same:
Radius of the smaller hollow balls (r_inner) = r_outer/2 = 3 cm / 2 = 1.5 cm
Now, let's calculate the surface area for both cases:
Surface area of the larger hollow ball:
Surface Area_large = 4π(r_outer^2 - r_inner^2)
Surface Area_large = 4π(3^2 - 1.5^2)
Surface Area_large = 4π(9 - 2.25)
Surface Area_large = 4π(6.75)
Surface Area_large ≈ 85.39 cm^2
Surface area of the smaller hollow balls (each):
Surface Area_small = 4π(r_outer^2 - r_inner^2)
Surface Area_small = 4π(1.5^2 - 0.75^2)
Surface Area_small = 4π(2.25 - 0.5625)
Surface Area_small = 4π(1.6875)
Surface Area_small ≈ 21.24 cm^2
Now, let's compare the surface areas:
The total surface area of the three smaller hollow balls = 3 * Surface Area_small ≈ 3 * 21.24 cm^2 ≈ 63.72 cm^2
Therefore, it takes more paint to paint the three smaller hollow balls compared to the larger hollow ball. The difference in surface area is given by:
Difference = Surface Area_small - Surface Area_large ≈ 63.72 cm^2 - 85.39 cm^2 ≈ -21.67 cm^2
The difference is negative because the surface area of the larger hollow ball is greater than the combined surface area of the three smaller hollow balls.
If h(x)=x³−2x²+3, find h(−1)
a. -5 go to station 3
b. 5 go to station 6
c. -12 go to station 8
d. 0 go to station 4
e. 2 go to station 12
Answers
By evaluating the function h(x) = x³ − 2x² + 3 at x = -1, we find that h(-1) = -4. Therefore, the correct answer is Option d. 0 go to station 4.
To find h(-1), we substitute -1 into the function h(x) = x³ − 2x² + 3:
h(-1) = (-1)³ − 2(-1)² + 3
Applying the order of operations, we first evaluate the exponents:
h(-1) = -1 - 2(1) + 3
Next, we simplify the multiplication:
h(-1) = -1 - 2 + 3
Now, we combine like terms:
h(-1) = 0
Therefore, h(-1) evaluates to 0. This means that when we substitute -1 into the function h(x) = x³ − 2x² + 3, the output is 0. Hence, the correct answer is 0 go to station 4.
Learn more about composition function here : https://brainly.com/question/32599830
#SPJ11
Please write well.
Answer the following question when X₁, X₂,..., X is a random sample from an exponential family with the following probability density function. f(x 0) = exp (0T(x) + d(0)+ S(x)) a. H: 0= 0 vs H₁
Answers
Given that X₁, X₂,..., X is a random sample from an exponential family with the following probability density function, f(x 0) = exp (0T(x) + d(0)+ S(x)).
To form the hypothesis for the exponential family, we need to consider the null and alternative hypothesis.
Null hypothesis: 0= 0
Alternative hypothesis: 0 ≠ 0
Explanation: The exponential family is a class of distribution families. The density of an exponential family is given by the following expression:
f(x|θ) = h(x) exp{θT(x) − A(θ)},
where h(x) is a nonnegative function of the data that does not depend on the parameter θ and A(θ) is a normalizing function.
The parameter θ is typically called the natural parameter, and T(x) is the vector of sufficient statistics. The exponential family of distributions includes the normal, exponential, chi-squared, gamma, and beta distributions, among others. In hypothesis testing for the exponential family, we typically specify a null hypothesis and an alternative hypothesis, just as in other types of hypothesis testing. The test statistic is usually a ratio of two likelihood ratios.
To know more about exponential family refer to:
https://brainly.com/question/27622986
#SPJ11
According to the video above, the geometric object called a(n) ___ has the characteristics that it has one endpoint and extends in away from that endpoint without end.
Answers
They are used in navigation, astronomy, and surveying. Rays are also used in computer graphics, physics, and optics. In addition, rays are used in the study of optics to describe the behavior of light as it travels through different mediums.
According to the video above, the geometric object called a ray has the characteristics that it has one endpoint and extends in away from that endpoint without end.A ray is a line that starts at a single point and extends in one direction to infinity. Rays are commonly used in geometry to explain lines and line segments. A ray has one endpoint, called the endpoint of the ray, from which it starts. The other end of the ray continues in the direction in which it is pointed without any limit. A ray is named by using its endpoint and another point on the ray, with the endpoint first. For example, if ray A starts at point P and passes through point Q, we write the name of the ray as ray PAQ or ray QAP. Rays can be part of line segments and other geometric objects. They can also be used to explain angles and the direction of a light source. Rays are commonly used in mathematics, science, and engineering.
for more seach question surveying
https://brainly.com/question/17365081
#SPJ8
Find the inverse for each relation: 4 points each 1. {(1,‐2), (2, 3),(3, ‐3),(4, 2)}
Answers
{(-2, 1), (3, 2), (-3, 3), (2, 4)}
Therefore, the inverse of the relation {(1,-2), (2,3), (3,-3), (4,2)} is {(-2,1), (3,2), (-3,3), (2,4)}.
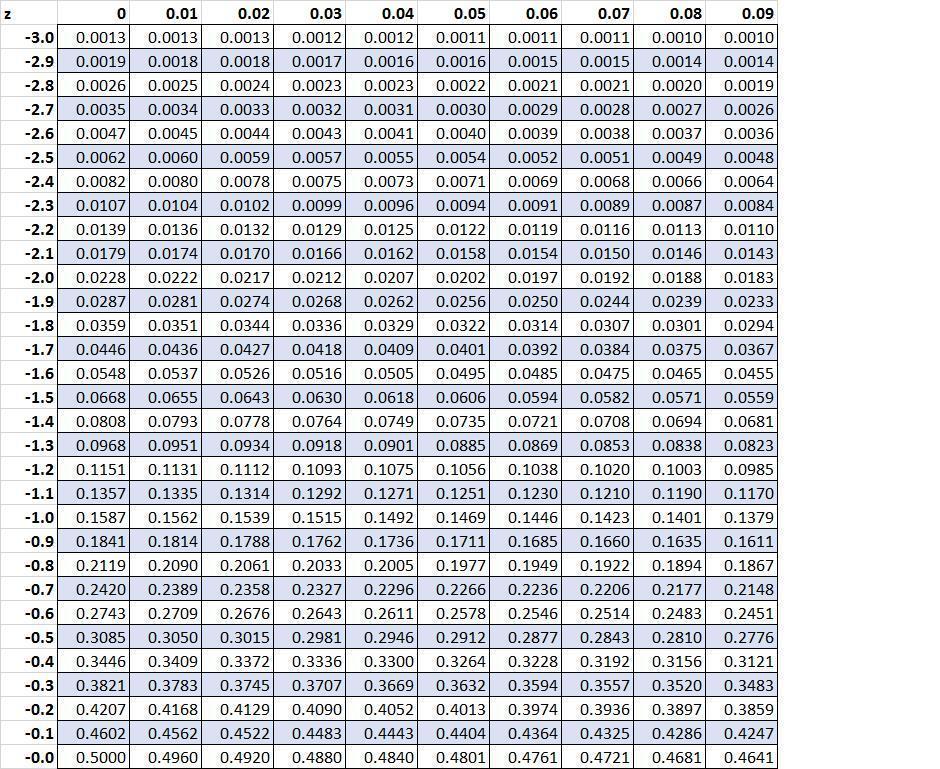
suppose that the ages of medical residents are normally distributed with a mean of 27 years and standard deviation 2 years. what percent of medical residents are less than 28 years old?
Answers
The percent of medical residents less than 28 years old is 69.15%.
For a normally distributed set of data, given the mean and standard deviation, the probability can be determined by solving the z-score and using the z-table.
First, solve for the z-score using the formula below.
z-score = (x – μ) / σ
where x = individual data value = 28
μ = mean = 27
σ = standard deviation = 2
z-score = (28 - 27) / 2
z-score = (1) / 2
z-score = 0.5
Find the probability that corresponds to the z-score in the z-table. (see attached images)
at z = 0.5, p = 0.6915
Multiply the probability by 100 to get the percentage.
% = p x 100
% = 0.6915 x 100
% = 69.15
Hence, the percent of medical residents that are less than 28 years old is 69.15%.
Learn more about probability here: brainly.com/question/26822684
#SPJ4

As a baker you need 1 1/2 cup of butter for each color of frosting. If the cake you are
decorating has 3 different colors, how much butter will you need?
Answers
Answer:
4 1/2 cups of butter
Step-by-step explanation:
1 1/2 cups = 3/2 cups
3/2 x 3 = 9/2
9/2 = 4 1/2
In the given figure, AD is adjacent to BC.
if AB = 13 cm, BD = 5 cm and DC = 16 cm,
find the values of :
(I) sin B
(II) sec B
(III) cot B
(iv) cos C
(v) cosec C
(vi) tan C

Answers
By using the method of trigonometry,
(1)sin B=P/H=AD/AB=12/13
(2)sec B=H/B=AB/BD=13/5
(3)cot B=B/P=BD/AD=5/12
(4)cos C=B/H=CD/AC=16/20=4/5
(5)cosec C=H/P=AC/AD=20/12=5/3
(6)tan C =P/B=AD/DC=12/16=3/4
What is trigonometry?Trigonometry is the study of angles and the angular relationships between planar and three-dimensional shapes. The cosecant, cosine, cotangent, secant, sine, and tangent are the trigonometric functions (sometimes known as the circle functions) that make up trigonometry. The unit circle is the most straight forward way to define the trigonometric functions. Let theta represent an angle that is calculated along a circle's arc counterclockwise from the x-axis.
Given that,
\(AD^{2}= AB^{2}- BD^{2}= 13^{2}- 5^{2}= 169-25= 144\)
=>AD=12cm
(1)sin B=P/H=AD/AB=12/13
(2)sec B=H/B=AB/BD=13/5
(3)cot B=B/P=BD/AD=5/12
\(AC^{2}= AD^{2}+ DC^{2}= 12^{2}+ 16^{2}= 144+ 256= 400\)
=>AC=20cm
(4)cos C=B/H=CD/AC=16/20=4/5
(5)cosec C=H/P=AC/AD=20/12=5/3
(6)tan C =P/B=AD/DC=12/16=3/4
note: P=perpendicular
B=base
H=hypotenuse
To know more about trigonometry visit:
https://brainly.com/question/22986150
#SPJ9
PLEASE HURRY ⏰ select the 2 missing "X Values" and "Y Values" from the table to complete it Select ALL that apply h(x)= -(1/4)^x
Answers
The values for "Y" correspond to the chosen "X" values are \(-0.0625,-0.25,-1,-0.25,-0.0625\)
What are functions?
A function, also known as the domain and the range, is a fundamental idea in mathematics that represents the relationship between two sets of elements. Each element in the domain is paired with a different element in the range.
A function is, more precisely, a rule or a correspondence that links every input value from the domain to precisely one output value from the range. The variable x normally represents the input values, and the variable y or f(x) typically represents the corresponding output values.
A function can be envisioned as a device that accepts an input and outputs a particular result in accordance with the rule or operation specified by the function. Only the input value influences the output, and each
Calculating the corresponding values of "X" and "Y" for each row is necessary to finish the table for the function \(h(x) = -(1/4)x\). I am not able to choose the missing values because the table is not provided. But I can explain to you how to figure out the function's values.
A function that depicts an exponential function is \(h(x) = -(1/4)x\). You can use the provided function to find the values by selecting a range of "X" values and determining the corresponding "Y" values.
Let's pick a range of "X" values from \(-2 to 2\), for illustration:
when \(x = -2:\)
\(h(-2) = -(1/4)^(-2) = -(1/4)^2 = -(1/16) = -0.0625\)
when \(x = -1:\)
\(h(-1) = -(1/4)^{-1} = -(1/4)^1 = -1/4 = -0.25\)
when \(x = 0:\)
\(h(0) = -(1/4)^0 = -1^0 = -1\)
when \(x = 1:\)
\(h(1) = -(1/4)^1 = -1/4 = -0.25\)
when \(x=2:\)
\(h(2) = -(1/4)^2 = -1/16 = -0.0625\)
These are the values for "Y" corresponding to the chosen "X" values. Depending on the table, you can select the appropriate values to complete it.
Learn more about Functions:
https://brainly.in/question/55795682
#SPJ4
Find my number, if the product of my number and 3 is 15 more than thesume of my number and 3
Answers
Which of the following is NOT true about similar figures?
a. Similar figures always have the same shape.
b. Similar figures have always the same size.
c. similar figures have corresponding angles that are always congruent.
d. Similar figures have corresponding sides that are always proportional.
Answers
Answer:
b. Similar figures have always the same size. is a false statement.
since size differs.
The statement that is NOT true about similar figures is: b. Similar figures have always the same size.
What are Similar Figures?Figures that are similar to each other have corresponding angles that are congruent but have corresponding sides that are proportional.
Similar figures have the same shape but different sizes.
Thus, the statement that is NOT true about similar figures is: b. Similar figures have always the same size.
Learn more about similar figures on:
https://brainly.com/question/4381215