The zoom feature on a camera lens allows you dilate what appears on the display. When you change from 100% to 400%, the new image on your screen is an enlargement of the previous image with a scale factor of 4. If the new image is 38 millimeters wide, what was the width of the previous image?
Answers
the width of the previous image is 9.5mm.
What is the width?The widthh can be defined as the horizontal measurement or distance measured from side to side.
In geometry, the shorter side of an object is frequently referred to as its "width". For instance, both of the given rectangles are identical rectangles, but one of them has been rotated to demonstrate that the shorter side of the rectangle is referred to as its width.
In the given problem,
if the width of the previous image is x,
4x=38
⇒x=9.5mm
So, the width of previous image is 9.5mm.
to understand more about width visit:
brainly.com/question/30173060
#SPJ1
Related Questions
You have a mortagege of $275,000
after down payment with an interest
rate of 3% for 30 years.
What does P, r,n, and t are equal to?

Answers
Answer:
Our calculator limits your interest deduction to the interest payment that would be paid on a $1,000,000 mortgage. Interest rate: Annual interest rate for this
Step-by-step explanation:
NUMBER SENSE Events A and B are independent. Suppose P(B)=0.4 and P(A and B)=0.13 . Find P(A) .
Answers
Answer:
Step-by-step explanation:

Warren earns $21.75 per hour and worked 36.5 hours last week and 32 hours the
week before. What is Warren's gross pay for the two weeks? Show your work.
Answers
Answer:
$1,489.88 (nearest cent)
Step-by-step explanation:
To calculate gross pay, multiply the number of hours worked by the pay per hour.
Warren worked 36.5 hours one week and 32 hours the week before.
Therefore, the total number of hours Warren worked was:
36.5 + 32 = 68.5 hoursMultiply the total number of hours worked by Warren's rate of pay of $21.75 per hour:
68.5 × 21.75 = 1489.875Therefore, Warren's gross pay for the two weeks was $1,489.88 (nearest cent).
Answer:
$1489.875
Step-by-step explanation:
Warren's gross pay for 36.5 hours last week can be calculated as follows:
Gross pay for 36.5 hours = $21.75/hour * 36.5 hours = $793.875
Similarly, Warren's gross pay for 32 hours the week before can be calculated as follows:
Gross pay for 32 hours = $21.75/hour * 32 hours = $696
Adding the gross pay for the two weeks, we get:
Gross pay for 2 weeks = $793.875 + $696 = $1489.875
Solve for the total cost. Retail price: $12.95; discount rate: 15%; sales tax rate: 7%
Answers
Answer: $10.24
Step-by-step explanation: 12.95 * 0.15 = 1.94 12.95 - 1.94 = 11.01
11.01 * 0.07 = .77 11.01 - 0.77 = 10.24
Sylvia buys a case of water that contains 24 bottles and sells x number of bottles to her co- workers. Sam buys a case of water that contains 36 bottles and sells three times as man bottles as Sylvia sold to his co-workers. How many bottles of water did Sylvia sell if they ended up with the same number of bottles of water left over?
Answers
Answer:
6 bottles
Step-by-step explanation:
Given the following :
SYLVIA:
Case of water = 24 bottles
Number of bottles sold = x
SAM:
case of water = 36 bottles
Number of bottles sold = 3x
Number of bottles left after sales are the same ;
Number of bottles left :
24 - x = 36 - 3x
Then solve for x
-x + 3x = 36 - 24
2x = 12
x = 12/2
x = 6
Hence, Sylvia sold 6 bottles of water.
find the radius of convergence, r, of the series. [infinity] x^n/ 3n − 1
Answers
The series converges for |x| < 1, and the radius of convergence, r, is 1.
To find the radius of convergence, r, of the series ∑ (infinity, n = 0) x^n / (3n − 1), we can use the ratio test. The ratio test states that for a power series ∑ a_n * x^n, if the limit of the absolute value of the ratio of consecutive terms |a_(n+1) / a_n| exists, then the series converges absolutely if the limit is less than 1, and diverges if the limit is greater than 1.
Let's apply the ratio test to our series:
lim (n → ∞) |(x^(n+1) / (3(n+1) - 1)) / (x^n / (3n - 1))|
Simplifying the expression:
lim (n → ∞) |(x^(n+1)(3n - 1)) / (x^n(3(n+1) - 1))|
The x^n terms cancel out:
lim (n → ∞) |(x(3n - 1)) / (3(n+1) - 1)|
Taking the absolute value and simplifying:
lim (n → ∞) |x(3n - 1) / (3n + 2)|
Since we're interested in the radius of convergence, we want to find the value of |x| that makes the limit less than 1. Thus:
|x(3n - 1) / (3n + 2)| < 1
Taking the limit as n approaches infinity, we can ignore the n terms:
|x| < 1
Therefore, the series converges for |x| < 1, and the radius of convergence, r, is 1.
Learn more about converges here:
https://brainly.com/question/31489835
#SPJ11
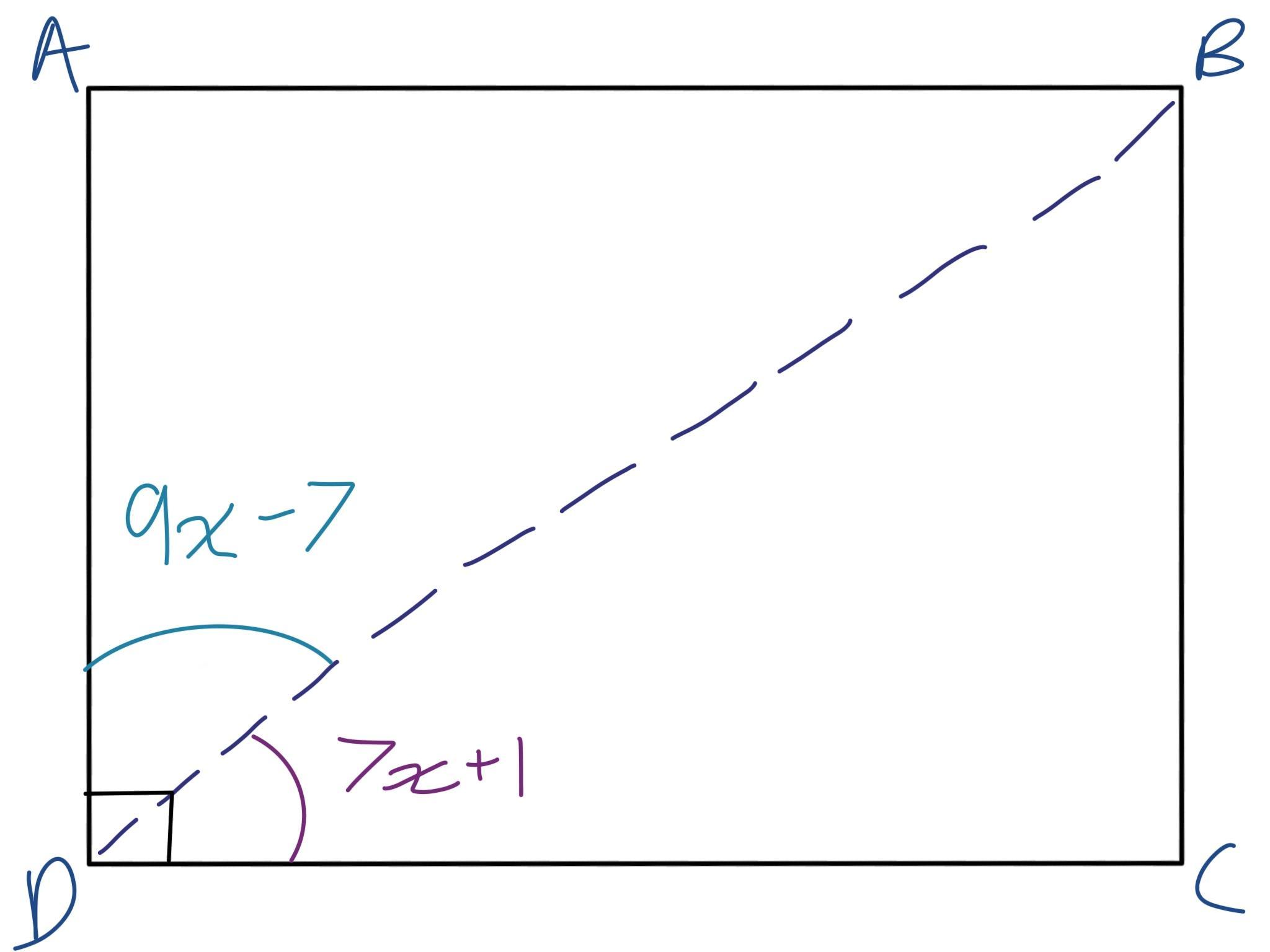
Quadrilateral ABCD is a rectangle.
If BDC = 7x + 1 and ADB=9x - 7, find BDC.
Answers
\(\huge\boxed{45^\circ}\)
See the attached diagram.
Together, angles BDC and ADB form a right angle, which is 90 degrees.
The angles are split straight down the middle, meaning they're equal to each other. This means each one is equal to half of 90 degrees, which is 45 degrees.
BRAINLIEST I NEED HELP BRAINLIEST BRAINLIEST BRAINLIEST BRAINLIEST I looked on google and I am not looking for that

Answers
Answer:
well 20 years ago industrialization and technological advances had not been a major change in humanity as it is now so due natural processes were followed in food production but now with an advancement in research everyday food production now have to go through the laboratories instead of cultivation of farmland in order to satisfy our ever growing population which has reduced significantly the production and consumption of healthy foods coupled with the introduction of junks which many use to substitute normal food.
Answer:
What is the photo
Step-by-step explanation:
A triangle has angle measurements of 44 degrees,87 degrees, and 49 degrees. What kind of triangle is it? acute,right, and obtuse.
Answers
Does an nxn matrix always have eigenvalues?
Answers
Yes, an nxn matrix always has eigenvalues.
What do we mean by eigenvalues of a matrix?An eigenvalue is a scalar that satisfies the equation Ax = λx, where A is the nxn matrix, x is a non-zero vector, and λ is the eigenvalue. An nxn matrix always has n eigenvalues, which may be real or complex numbers. The eigenvalues of a matrix can be found by solving the characteristic equation det(A - λI) = 0, where I is the identity matrix. The eigenvalues of a matrix are important in many areas of mathematics and engineering, as they can provide information about the stability and behavior of a system.
Read more; https://brainly.com/question/14406572
#SPJ11
let e be the region bounded below by the cone z=−√3⋅(x2 y2) and above by the sphere z2=102−x2−y2 . provide an answer accurate to at least 4 significant digits. find the volume of e.
Answers
The volume of the region bounded below by the cone z = -√3⋅(x^2 + y^2) and above by the sphere z^2 = 102 - x^2 - y^2 can be calculated.
To find the volume of the region, we need to determine the limits of integration for x, y, and z. The cone and sphere equations suggest that the region is symmetric about the xy-plane and centered at the origin.
Considering the cone equation, z = -√3⋅(x^2 + y^2), we can rewrite it as z = √3⋅(-x^2 - y^2). This equation represents a cone pointing downwards with a vertex at the origin.
The sphere equation, z^2 = 102 - x^2 - y^2, represents a sphere centered at the origin with a radius of 10.
To find the volume, we integrate the function f(x, y, z) = 1 over the region e. Since the region is bounded below by the cone and above by the sphere, the limits of integration for x, y, and z are determined by the intersection of the two surfaces.
By setting z equal to 0 and solving the equation -√3⋅(x^2 + y^2) = 0, we find that the intersection occurs at the xy-plane.
Therefore, we can set up the triple integral ∫∫∫e 1 dV and evaluate it over the region e. The resulting value will be the volume of the region e
Learn more about volume of the region here:
https://brainly.com/question/15166233
#SPJ11
Robin earned $4,320 in 9 weeks. How much did he earn working for a full year?
Answers
Answer:
4320/9=$480 per week.
Then you do $480 time the 50 weeks he worked for the whole year.
480
*50
------
24000
Robin earns $24,000 from working for the whole year (50 weeks).
feel free to report me if im wrong
What is the slope of the line represented by the equation y = 4/5x - 3?
Answers
Answer:
The slope is 4/5 and the y intercept is -3
Step-by-step explanation:
The equation is in slope intercept form
y= mx+b where m is the slope and b is the y intercept
y = 4/5x -3
The slope is 4/5 and the y intercept is -3
Are two angles that lie in the same plane and have a common vertex and a common side but have no common interior points.
a. True
b. False
Answers
This statement is - true.
Two angles that lie in the same plane and have a common vertex and a common side but have no common interior points are the meaning of adjacent angles.
A figure in which two rays meet at a common point is called an angle. The common endpoint of two rays that form the angle is called a vertex. Interior points are the points that lie within the arms of the angle produced indefinitely.If the two angles share a common vertex and side, those angles are called adjacent angles. Adjacent angles are categorized as complementary angles and supplementary angles.If the sum of two adjacent angles comes to 90°, those angles are called complementary angles because they complement each other. e.g. 40° and 50° are complementary angles.When the sum of two adjacent angles becomes 180° and the angles form a straight line, those angles are known as supplementary angles. e.g. 120° and 60° are supplementary angles.Read more about adjacent angles:
https://brainly.com/question/24533723
#SPJ4
If jay takes 40 days to paint an house and kimmy takes 60 days to paint the same house. if they work togther how many day will it take to paint the house
Answers
The time taken by Jay and Kimmy if they paint together is 24 days.
Speed formula:
Speed = distance : timeDistance = Speed x timeTime = Distance : SpeedJay's takes 40 days to paint an house, so Jay's speed at painting a house in one day is \(\frac{1}{40}\)
Kimmy's takes 60 days to paint an house, so Kimmy's speed at painting a house in one day is \(\frac{1}{60}\)
The time it would take Jay and Kimmy if they painted together.
\(\frac{1}{t}\) = \(\frac{1}{40}\) + \(\frac{1}{60}\)
\(\frac{1}{t}\) = \(\frac{1\times 60}{40\times 60}\) + \(\frac{1\times 40}{60\times 40}\)
\(\frac{1}{t}\) = \(\frac{60}{2400}\) + \(\frac{40}{2400}\)
\(\frac{1}{t}\) = \(\frac{60+40}{2400}\)
\(\frac{1}{t}\) = \(\frac{100}{2400}\)
t = \(\frac{2400}{100}\)
t = 24
So, the time taken by Jay and Kimmy if they paint together is 24 days.
Learn more about speed at https://brainly.com/question/30134814
#SPJ4
Suppose Lisa wants to glue decorative paper onto the block. What is the total surface area that Lisa would need to cover? Show your work.

Answers
The total surface area that Lisa would need to cover is 2 times the sum of the areas of the top and bottom faces plus 2 times the sum of the areas of the side faces.
To calculate the total surface area that Lisa would need to cover, we need to consider all the faces of the block.
Assuming the block is a rectangular prism, it will have six faces: a top face, a bottom face, and four side faces.
Let's denote the length, width, and height of the block as L, W, and H, respectively.
Top and Bottom Faces:
The top and bottom faces have dimensions of L × W each, so their combined area is 2 × (L × W).
Side Faces:
The side faces consist of four rectangles, two with dimensions of L × H and two with dimensions of W × H. So, the combined area of the side faces is 2 × (L × H) + 2 × (W × H).
Now, we can calculate the total surface area by summing up the areas of all the faces:
Total Surface Area = 2 × (L × W) + 2 × (L × H) + 2 × (W × H)
Therefore, the total surface area that Lisa would need to cover is 2 times the sum of the areas of the top and bottom faces plus 2 times the sum of the areas of the side faces.
For such more questions on Surface area of block
https://brainly.com/question/29140137
#SPJ11
What is the volume of a hemisphere with a radius of 34.1 in rounded to the nearest tenth
Answers
Answer:
\(83046.6in {}^{3} \)
Step-by-step explanation:
STEP 1: Write the formula for the volume of hemisphere.
\(volume = \frac{2}{3} \pi \: r {}^{3} \)
STEP 2: Write the given details
radius (r) = 34.1
STEP 3: Substitute the value of r into the formula
\(volume = \frac{2}{3} \times \pi \times 34.1 {}^{3} \\ \\ volume = 83046.5797 \\ \\ volume = 83046.6in {}^{3} \)
I just need help with b
a)
Mark three points L, M and N not in a straight line. By construction find the point which is equidistant from L, M and N.
b)
What would happen if L, M and N were on the same straight line?
Answers
Answer:
I don't know sorry for that I tried my best
Points B(-4,4) and D (10,4) lie on circle G. The line segment connecting the two points is a diameter of the circle.
Which equation represents circle G?
A. (x – 3)2 + (y – 4)2 = 49
O B. (x - 10)2 + (y - 4)2 = 49
O C (x+3)2 + (1 – 4)2 = 49
D. (x+4)2 + (y – 4)2 = 49
Answers
Equation of circle G: \(\bold{(x-3)^{2} + (y-4)^{2} = 49}\)
Equation of circle with center-radius form:"An equation of a circle with center (p, q) and radius r is:\((x-p)^{2}+ (y-q)^{2}=r^{2}\) "
Midpoint Formula:"The coordinates of the midpoint M of two points (a, b) and (x, y) :
\(M(\frac{a+x}{2} ,\frac{b+y}{2} )\) "
Distance formula:"The distance between two coordinate points (a, b), (m, n):
\(d=\sqrt{(n-b)^{2} +(m-a)^{2} }\) "
What is diameter of circle?"A diameter of a circle is the straight line that from one side of a circle to other side and passes through the center."
For given question,
The line segment connecting the two points B(-4,4) and D (10,4) is a diameter of the circle.
This means, the midpoint of the diameter BD would be the center of a circle G.
Let M represents the center of the circle.
First we find the coordinates of the center of the circle.
Using midpoint formula,
⇒ M = \((\frac{-4+10}{2} ,\frac{4+4}{2} )\)
⇒ M = (6/2 , 8/2)
⇒ M = \((3,4)\)
Now, we find the distance between points B(-4,4) and D (10,4) to determine the diameter of a circle G.
Using distance formula,
⇒ \(d=\sqrt{(4-4)^{2} +(10-(-4))^{2} }\)
⇒ \(d=\sqrt{0+(14)^{2} }\)
⇒ \(d=14\) units
So, the radius of the circle r would be,
r = d/2
⇒ r = 14 / 2
⇒ r = 7 units
Now, we find the equation of circle with center M(3, 4) and radius r = 7 units.
Using center - radius form equation of circle would be,
\((x-3)^{2} + (y-4)^{2} = 7^{2}\)
⇒ \(\bold{(x-3)^{2} + (y-4)^{2} = 49}\)
Therefore, option (A) is the correct answer.
Learn more about equation of circle here:
https://brainly.com/question/10618691
#SPJ2
Help me with this Question Please.

Answers
Answer:
\(3 \times \frac{1}{3 } + \frac{1}{2} \times - 12( \frac{1}{3} ) = \frac{1}{3} \)
¿que es la trigonometria?
Answers
Answer:
I don't speak Spanish but I know what your asking. Trigonometry is a thing in physics.
Step-by-step explanation:
Ari said there are three possible outcomes when you
spin this spinner twice: two reds, a yellow and a red,
or two yellows.
So, the probability of getting two yellows is 1/3
Do you agree or disagree? Explain your thinking
Please answer fast :(
Answers
========================================================
Reason:
Ari hasn't considered the case of "red and yellow" which is almost identical to "yellow and red".
It helps to lay out a two by two table. Along the rows and columns, you'll have "red" and "yellow". See below.
Then inside the table are the list of all outcomes in the form (x,y) where x is the horizontal component along the columns, and y is the vertical component along the rows.
Example: if x = red and y = red, then we have the outcome (x,y) = (red, red)
As the table shows, we have 4 outcomes. Therefore the probability of getting two yellows is 1/4
Technically the (red, yellow) = (yellow, red) since ultimately order doesn't matter in this case. For some probability problems, order will matter. But again, order doesn't matter here and it means that we can treat these two situations the same. So I can see why Ari thinks there are only three outcomes.
--------------------------------
Here's another way to look at it:
The probability of landing on yellow is 1/2 assuming red and yellow are equally likely.
This means the probability of two yellow in a row is (1/2)*(1/2) = 1/4
Each is spin is independent of any other.

PLEASE HURRY: Which value is NOT located between these two numbers on the
number line?

Answers
Answer:
C.) π/9
Step-by-step explanation:
√9/3 = 1
2π ≈ 6.28
The only value that is not between these two numbers is π/9 which is approximately 0.35.
Find the sum of 6x+7 and 8x^2 +5x-1
Please hurry I need this asap
Answers
Answer:
11x+8x^(2)+6
Step-by-step explanation:
Answer:
8x² + 11x + 6
Step-by-step explanation:
Expression addition:Combine like terms. Like terms have same variable with same exponent.
5x and 6x are like terms. Add the coefficient of the like terms.
(-1) & 7 are constants and add (-1) & 7.
8x² + 5x - 1 + 6x + 7 = 8x² + 5x + 6x - 1 + 7
= 8x² + 11x + 6
[PLEASE ANSWER ASAP 20 PTS] The entrance fee to a circus for a child is RM(3x-y). The entrance fee for an adult is RM2y more than the entrance fee for a child. Mr Kamal and his wife brings their three children ro the circus, How much should he pay altogether.
Answers
Based on the calculation below, the amount he should pay altogether is RM15x – y.
How to solve a problem by collecting like terms?Let C represent the entrance fee for a child and A represents the entrance fee for an adult, we therefore have:
C = RM(3x-y)
A = RM(3x-y) + RM2y = RM3x – RMy + RM2y = RM(3x – y + 2y) = RM(3x + y)
Since Mr. Kamal and his wife bring their three children to the circus, this implies that we have 2 adults and 3 children.
The amount he should pay altogether (T) can therefore be calculated by collecting the like terms as follows:
T = 3C + 2A = 3RM(3x – y) + 2RM(3x + y)
T = RM9x – 3y + RM6x + 2y
T = RM9x + RM6x – 3y + 2y
T = RM15x – y
Learn more about like terms here: https://brainly.com/question/14557679.
#SPJ1
9. Lines A and B are parallel lines. Find the measures of angles 1, 2 and 3.

Answers
Answer:
<1 = 33
<2 = 90
<3 = 57
Step-by-step explanation:
<3 = 57 because of alternate interior angles
<2 + <3 + 33 = 180
<2 + 57 + 33 = 180
<2 + 90 = 180
<2 = 90
<1 + <2 + 57 = 180
<1 + 90 + 57 = 180
<1 + 147 = 180
<1 = 33
HELP AWARDING BRAINLIEST

Answers
Answer:
Either 2.34 or 2.345
Step-by-step explanation:
15. The line y = - 0.75x + 1.25 is tangent to a circle whose center is located at (2, 6) Find the tangent point and a second tangent point of a line with the same slope as the
Answers
The tangent point and a second tangent point of a line with the same slope as the given line are (2.67, 6) and (2.57, 6.1).
How to calculate the valueThe slope of the given line is -0.75.
The radius of the circle is |6 - 1.25| / -0.75 = 7.
The equation of the tangent line is y = -0.75x + c.
Substituting the coordinates of the center of the circle into this equation, we get 6 = -0.75 * 2 + c
Solving for c, we get c = 8.
The equation of the tangent line is y = -0.75x + 8.
The point of tangency is the point where the tangent line intersects the circle. To find the point of tangency, we need to solve the equation of the tangent line for x.
y = -0.75x + 8
6 = -0.75x + 8
-2 = -0.75x
x = 2.67
The point of tangency is (2.67, 6).
The second tangent point is the point where the tangent line intersects the circle at a different location. The direction of the tangent line is the same as the direction of the vector (-0.75, 1).
We can move the point of tangency a small distance in the direction of the tangent line by adding a small multiple of the vector (-0.75, 1) to the point of tangency.
Let's add a multiple of 0.1 to the point of tangency.
(2.67, 6) + 0.1 * (-0.75, 1)
= (2.57, 6.1)
The second tangent point is (2.57, 6.1).
Learn more about tangent on
https://brainly.com/question/30162650
#SPJ1
The line y = - 0.75x + 1.25 is tangent to a circle whose center is located at (2, 6) Find the tangent point and a second tangent point of a line with the same slope as the given line
Kristen's financial advisor has given her a list of 8 potential investments and has asked her to select and rank her favorite four. In how many different ways can she do this?
Answers
The number of ways in which Kristen can rank her favorite four from 8 using permutations is 1680.
The permutation is a way of finding the number of ways of selecting a set of articles from a larger set of articles, with the order of selection being significant.
If we want to choose r items from n items, where the order of selection is significant, then we can find the number of ways of doing this using the permutation as follows:
nPr = n!/{(n - r)!}.
In the question, we are asked to find the number of ways Kristen can rank her favorite four investments from the 8 potential investments that her financial advisor has given her.
Thus, using permutations, we need to select 4 items from 8 items, with order of selection being significant.
Substituting n = 8, and r = 4 in the formula, we get:
8P4 = 8!/{(8 - 4)!}
= 8!/4!
= 5 * 6 * 7 * 8
= 1680.
Thus, the number of ways in which Kristen can rank her favorite four from 8 using permutations is 1680.
Learn more about permutations at
https://brainly.com/question/12468032
#SPJ4
A conditional statement is not logically equivalent to its converse or inverse. But it is logically equivalent to its contrapositive. Use the laws of propositional logic to prove this. The first step of the proof is given. Prove:p → q ≡ ¬q → ¬p
Answers
As we can see from the truth tables, the column for p → q is the same as the column for ¬q → ¬p. Therefore, we can conclude that p → q is logically equivalent to ¬q → ¬p, proving the desired result.
Note: The converse and inverse of a conditional statement are not logically equivalent to the original statement.
To prove that a conditional statement is logically equivalent to its contrapositive, we'll use the laws of propositional logic. Let's start with the given statement:
p → q
To prove its logical equivalence with the contrapositive, ¬q → ¬p, we'll show that they have the same truth table.
First, let's construct the truth table for p → q:
p q p → q
T T T
T F F
F T T
F F T
Next, let's construct the truth table for ¬q → ¬p:
p q ¬p ¬q ¬q → ¬p
T T F F T
T F F T T
F T T F F
F F T T T
As we can see from the truth tables, the column for p → q is the same as the column for ¬q → ¬p. Therefore, we can conclude that p → q is logically equivalent to ¬q → ¬p, proving the desired result.
Note: The converse and inverse of a conditional statement are not logically equivalent to the original statement.
To know more about the word conditional, visit:
https://brainly.com/question/19258518
#SPJ11