a wireless network predicts that sales will increase 110% this upcoming year. if the sales are projected to be $16000 in the future, what are they in the past?
A 1600
B 7619
C 14400
D 14545
SHOW WORK
Answers
Answer:
Step-by-step explanation:
14545
Make a basic proportion
16,000/x=110/100
Cross multiply
1,600,000=110x
Divide
x=14545
Related Questions
Bill works as a tutor for an hour and as a waiter for an hour. This month, he worked a combined total of hours at his two jobs. Let be the number of hours Bill worked as a tutor this month. Write an expression for the combined total dollar amount he earned this month.
Answers
Answer:
31
Step-by-step explanation:
On a coordinate plane, a line goes through (negative 5, negative 4) and (0, negative 3). A point is at (negative 2, 2).
What is the equation of the line that is parallel to the given line and passes through the point (–2, 2)?
y = One-fifthx + 4
y = One-fifthx + Twelve-fifths
y = –5x + 4
y = –5x + Twelve-fifths
Answers
Rewriting the point-slope equation of the line, the equation of the line that is parallel to the given line is: y = 1/5x + 12/5.
What is the Point-Slope Equation?The point-slope equation is given as y - b = m(x - a), where m is the slope (change in y/change in x), and (a, b) is a point.
Find the slope of the line that passes through, (-5, -4) and (0, -3):
Slope = change in y/change in x = (-3 -(-4)) / (0 -(-5)) = 1/5
Slopeof parallel lines are equal, teherfore, the slope of the line that passes through (-2, 2) would be 1/5.
Substitite (a, b) = (-2, 2) and m = 1/5 into y - b = m(x - a):
y - 2 = 1/5(x -(-2)
y - 2 = 1/5(x + 2)
Rewroite in slope-inetrcept form
y - 2 = 1/5x + 2/5
y = 1/5x + 2/5 + 2
y = 1/5x + 12/5
Therefore, rewriting the point-slope equation of the line, the equation of the line that is parallel to the given line is: y = 1/5x + 12/5.
Learn more about point-slope equation on:
https://brainly.com/question/24907633
z = -3x +5y
Minimum value of z?
Answers
Answer:
minimum value of z is 0
Step-by-step explanation:
if x and y both equal zero, then the value of z is zero, which is your answer.
A certain state has the highest high school graduation rate of all states at 75%.
a. In a random sample of 20 high school students from the state, what is the probability that 16
will graduate?
b. In a random sample of 20 high school students from the state, what is the probability than 15 or fewer
will graduate?
c. What is the probability that at least 16 high school students in our sample of 20 will graduate?
a. What is the probability that 16 out of 20 high school students will graduate?
The probability that 16 students will graduate is 0.190.
(Type an integer or decimal. Round to three decimal places as needed.)
b. What is the probability that 15 or fewer out of 20 high school students will graduate?
The probability that 15 or fewer students will graduate is
(Type an integer or decimal. Round to three decimal places as needed.)
Answers
a. The probability of exactly 17 out of 20 high school students graduating is approximately 0.170 or 17%.
b. The probability of 16 or fewer high school students graduating is approximately 0.026 or 2.6%.
c. The probability of at least 17 high school students graduating in a sample of 20 is approximately 0.974 or 97.4%.
a. To calculate the probability that 17 out of 20 high school students will graduate, we can use the binomial probability formula. In this case, the probability of success (p) is 0.75 (graduation rate) and the number of trials (n) is 20 (sample size).
Using the formula, the probability can be calculated as:
P(X = 17) = C(20, 17) * (0.75)^17 * (1 - 0.75)^(20 - 17)
where C(20, 17) represents the number of combinations of choosing 17 out of 20 students.
Calculating this expression gives us:
P(X = 17) = 0.170
Therefore, the probability that exactly 17 out of 20 high school students will graduate is approximately 0.170 or 17%.
b. To calculate the probability that 16 or fewer students will graduate, we need to calculate the cumulative probability up to 16 students. We can sum the probabilities of each individual case from 0 to 16.
P(X ≤ 16) = P(X = 0) + P(X = 1) + ... + P(X = 16)
Using the binomial probability formula, we can calculate each term and sum them up:
P(X ≤ 16) = ∑ [C(20, k) * (0.75)^k * (1 - 0.75)^(20 - k)] for k = 0 to 16
Calculating this expression gives us:
P(X ≤ 16) = 0.026
Therefore, the probability that 16 or fewer high school students will graduate is approximately 0.026 or 2.6%.
c. To calculate the probability that at least 17 students will graduate, we need to calculate the complementary probability of having 16 or fewer students graduate.
P(X ≥ 17) = 1 - P(X ≤ 16)
Using the previously calculated value for P(X ≤ 16), we can substitute it into the equation:
P(X ≥ 17) = 1 - 0.026
P(X ≥ 17) = 0.974
Therefore, the probability that at least 17 high school students will graduate in a sample of 20 is approximately 0.974 or 97.4%.
For more question on probability visit:
https://brainly.com/question/25839839
#SPJ8
Note the complete question is
A certain state has the highest high school graduation rate of all states at 75%. a. In a random sample of 20 high school students from the state, what is the probability that 17 willgraduate? b. In a random sample of 20 high school students from the state, what is the probability than 16 or fewer willgraduate? c. What is the probability that at least 17 high school students in our sample of 20 will graduate?
Using the translation (x,y) --> (x+1,y-3), what is the image of A (-1,7)
Answers
Answer:
A'(0,4)
Step-by-step explanation:
You would just add 1 to the x and subtract 3 form the y and you would get A prime or A'(0,4) so that would be your answer
A(-1,7)--> (-1+1,7-3)
(0,4)
list seven advantages that aluminum parallel-flow plate-and-fin evaporator has over a standard round copper tube plate-and-fin evaporator.
Answers
The aluminum parallel-flow plate-and-fin evaporator has several key advantages over the standard round copper tube plate-and-fin evaporator are Lighter Weight, Improved Heat Transfer Efficiency.
An evaporator is a device used in air conditioning and refrigeration systems to remove heat from the refrigerant, which helps to cool down the air. There are two main types of evaporators: the aluminum parallel-flow plate-and-fin evaporator and the standard round copper tube plate-and-fin evaporator.
The parallel-flow design of the aluminum evaporator provides a larger surface area for heat transfer, which results in more efficient cooling. This means that less energy is required to achieve the same cooling effect.
Reduced Pressure Drop: The parallel-flow design also reduces the pressure drop in the refrigerant, which can improve the overall efficiency of the air conditioning or refrigeration system.
Aluminum is lighter than copper, which makes the aluminum evaporator easier to handle and install. This can be especially important in large air conditioning or refrigeration systems where weight and handling can be a significant concern.
Increased Durability: Aluminum is a more durable material than copper, which means that the aluminum evaporator is less likely to suffer from corrosion or other types of damage over time.
Better Resistance to Leakage: The parallel-flow design of the aluminum evaporator provides a tighter seal, which reduces the risk of refrigerant leakage. This helps to maintain the efficiency of the air conditioning or refrigeration system.
Lower Maintenance Costs: The increased durability and better resistance to leakage of the aluminum evaporator can help to lower maintenance costs over time.
Improved Environmental Performance: The improved efficiency and reduced pressure drop of the aluminum evaporator can help to lower the energy consumption of the air conditioning or refrigeration system. This can result in a reduction in greenhouse gas emissions and other environmental impacts.
To know more about evaporator here.
https://brainly.com/question/13502489
#SPJ4
Cones A and B both have volume 48bold pi cubic units, but have different dimensions. Cone A has radius 6 units and height 4 units. Find one possible radius and height for Cone B. Explain how you know Cone B has the same volume as Cone A.
Answers
Cone B does not have volume 48bold pi cubic units with these dimensions, but it does have the same volume as Cone A because they both have volume 48bold pi cubic units.
what is a cone?A cone, also known as a vertex, is a three-dimensional polygonal object with a flat base and a soft tapering apex. In a plane with no vertices, a cone is formed by connecting a story arc of lines, half-lines, but rather routes those are common to all focuses on the outpost. A cone is a muti structure with just a peaceful transition from a flat, round or, base towards the vertex and apex, which represents as the base's axis. A three-dimensional polygonal shape with only an upwardly flat curved surface is known as a cone.
Because Cone A has a volume of 48bold pi cubic units, we can use the volume of a cone formula to calculate its height:
Cone volume = 1/3 * base area * height
1/3 * pi * 62 * 4 = 48bold pi
48pi = 48bold pi
As a result, Cone A's height is 4 units. The radius is also 6 units, as we can see.
Cone volume = 1/3 * base area * height
\(1/3 * pi * r2 * h = 48\\144 = r^2 * h\\144 = 8^2 * 3\\144 = 192\)
Cone B does not have volume 48bold pi cubic units with these dimensions, but it does have the same volume as Cone A because they both have volume 48bold pi cubic units.
To know more about cone visit:
https://brainly.com/question/16394302
#SPJ1
graph the line with slope -3/4 passing through the point (2,-1)
Answers
Answer:
Step-by-step explanation:
Slope of the line:
\(m = \frac{y-y1}{x-x1} \\\)
Given:
slope = -3/4
point (x1,y1) = (2, -1)
Substitute and solve
\(m = \frac{y-y1}{x-x1} \\-\frac{3}{4} = \frac{y-(-1))}{x-2} \\-3(x-2) = 4(y+1)\\-3x + 6 = 4y + 4 \\Transpose\\4y = -3x + 6 - 4\\4y = -3x + 2 \\y = -3/4x + 2/4\\y = -3/4x + 1/2\\OR\\4y +3x - 2 = 0\)
1/2 is the y-intercept
(0,1/2)
Since we have 2 points already, we can then graph the line.
(2, -1) and (0, 1/2)

a clock chimes once at 1, twice at 2
Answers
Answer:
3 times at 3
Step-by-step explanation:
Karen runs 3 miles in 28 minutes. At the same rate, how many miles would she run in 42 minutes?
Answers
Answer:
4.5
Step-by-step explanation:
Well, she runs 3 miles in 28 minutes.
42 = 28 ⋅ 1.5
So, her journey would just be running
1.5
times, with each time running
28
minutes.
So, she runs a total of
1.5 ⋅ 3 = 4.5 miles
if sum a n and sum b n are series with positive terms, and lim a n/b n does not exist, and sum b n diverges, then sum a n diverges
Answers
Since lim a n/b n does not exist, it means that the ratio between a n and b n can be larger or smaller depending on the terms.
This implies that the rate of growth of a n and b n are not necessarily the same. If the series b n diverges, it means that the terms of b n increase without bound. Therefore, the terms of a n will also increase without bound, and sum a n will also diverge.
Formally, if lim a n/b n = ∞, then ∑ a n is divergent. We can prove this by contradiction. Suppose ∑ a n converges. Then by the comparison test, lim a n/b n must converge and be finite, which is a contradiction. Therefore, ∑ a n must diverge.
Learn more about comparison test here:
https://brainly.com/question/14620125
#SPJ4
Identify the coordinates for point D Awnser. Fast!
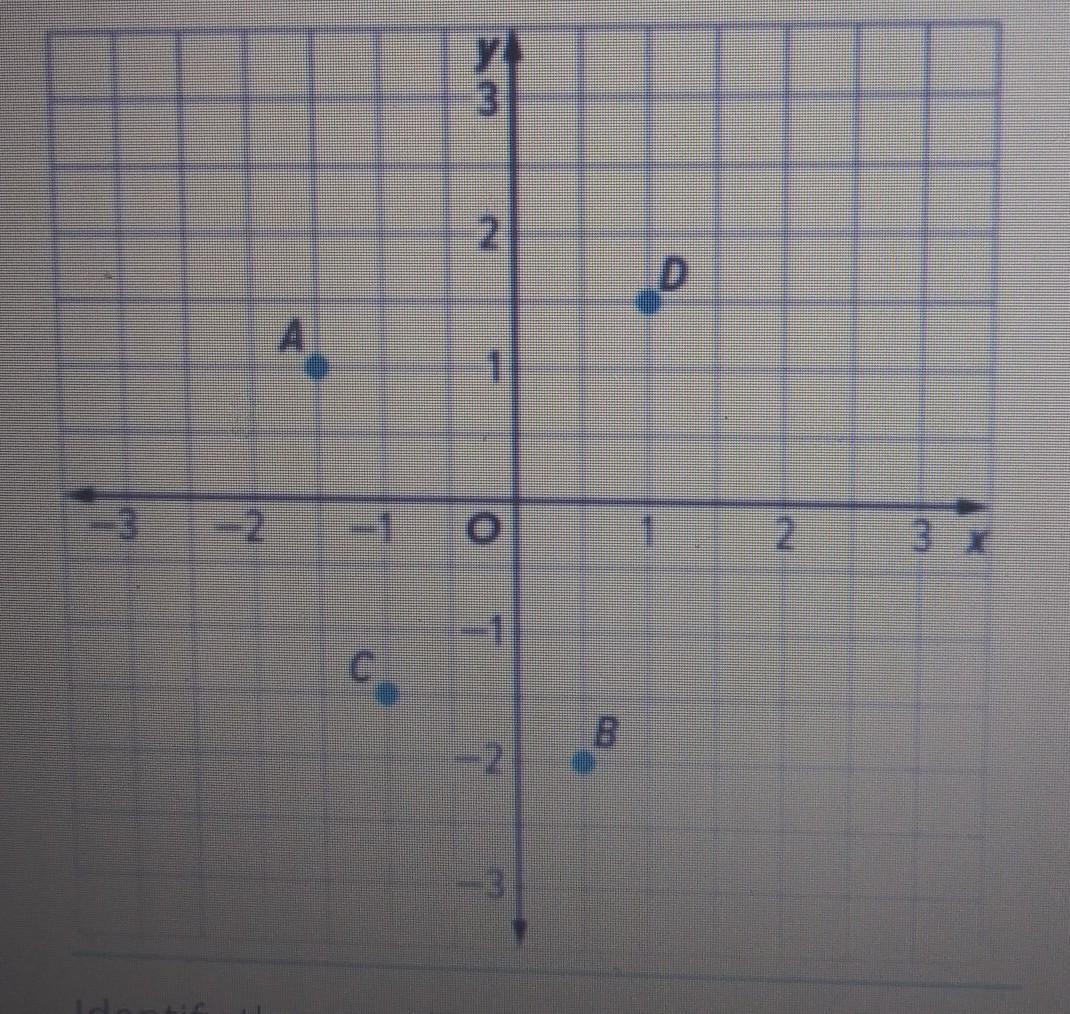
Answers
i hope it’s correct!
Answer:
(1,1.5)
Step-by-step explanation:
x = 1
y = 1.5
The ratio of red marbles to blue marbles was 8 to 3 what was the ratio of blue marbles to red marbles?
Answers
Answer:
3:8
Step-by-step explanation:
Answer: 3 to 8
Step-by-step explanation:
Since there are 8 red marbles and 3 blue marbles, the ratio of blue marbles to red marbles would be 3 to 8 or 3:8.
Hope this helped!! Have a great day :)
Please help me ASAP I’ll mark Brainly

Answers
Answer:
I can't help if I can't see the question
solve pls brainliest

Answers
63 divided by 3 is 21
Lets take this a step further
3 divided by 3 is 1
21 divided by 3 is 7
Answer is 1 over 7 as a fraction
Question 3: 12 pts A box has 26 pens —6 red, 8 green, 5 blue, and 7 black. Sam picks two pens at a time without replacement. What is the probability that Sam picks two blue pens?
A box has 26 pens —6 red, 8 green, 5 blue, and 7 black. Sam picks two pens at a time without replacement. What is the probability that Sam picks two blue pens?
Answers
Answer:
The probability of Sam is 2/5
Step-by-step explanation:
n(e)=2
n(s)=5
Pe=n(e)/n(s)
Pe=2=5
Clara has 3 bottles of paint. She buys 5 more bottles of paint. If she uses 1/4 bottle of paint to paint a picture, how many similar paintings can she paint with all the paint?
ANSWER:
She has 3+5=8 bottles of paint. 1/4 bottle paints a picture.
8 divided by 1/4 = 32 paintings possible with all the paint
Answers
Answer:
32 Paintings
Step-by-step explanation:
8 Bottles 4 per one bottle so 8x4 =32
Hoped this helped.
Given the functions below, find (g•h) (1).
g(x) = х^2 +4+ 2х
h(x) = — 3х + 2
-7
-30
35
7
Answers
Answer:
-7
Step-by-step explanation:
We are given the following functions:
\(g(x) = x^2 + 4 + 2x\)
\(h(x) = -3x + 2\)
(g•h) (1)
The multiplication is:
\((g \times h)(1) = g(1) \times h(1)\)
So
\(g(x) = 1^2 + 4 + 2(1) = 7\)
\(h(1) = -3(1) + 2 = -3 + 2 = -1\)
Then
\(g(1) \times h(1) = 7(-1) = -7\)
So -7 is the answer.
An element with
mass 510 grams decays by 26.3% per minute. How much of the
element is remaining after 7 minutes, to the nearest 10th of a gram?
Answers
Answer:
60.2 grams is remaining after 7 minutes.
Step-by-step explanation:
Exponential equation for amount of substance:
The exponential equation for the amount of a substance is given by:
\(A(t) = A(0)(1-r)^t\)
In which A(0) is the initial amount and r is the decay rate, as a decimal, and t is the time measure.
An element with mass 510 grams decays by 26.3% per minute.
This means that \(A(0) = 510, r = 0.263\)
So
\(A(t) = A(0)(1-r)^t\)
\(A(t) = 510(1-0.263)^t\)
\(A(t) = 510(0.737)^t\)
How much of the element is remaining after 7 minutes?
This is A(7). So
\(A(7) = 510(0.737)^7 = 60.2\)
60.2 grams is remaining after 7 minutes.
What is the equation of the line that passes through the points (2, 4) and (6, 2)?
A. y = 0.25x + 4.5
B. y = 0.25x + 0.5
C. y=-0.25x + 14
D. y =-0.25x + 3.5
Answers
the equation of the line that passes through the points (2, 4) and (6, 2) is y = - 0.5x + 6.
What is standard equation of a straight line?The standard equation of a straight line is -
Ax + By + C = 0
Given is to find the equation of the line that passes through the points (2, 4) and (6, 2).
We can write the slope as -
{m} = (2 - 4)/(6 - 2)
{m} = -2/4
{m} = -1/2
{m} = -0.5
The slope - intercept form would become -
y = - 0.5x + c
For the point (2, 4), we can write the {y} - intercept as -
4 = - 0.5 x 2 + c
c = 6
Therefore, the equation of the line that passes through the points (2, 4) and (6, 2) is y = - 0.5x + 6.
To solve more questions on straight lines, visit the link-
https://brainly.com/question/29223887
#SPJ9
2. The population of a town was 7,250 in 2014
and 7,375 in 2015. What was the percent
increase from 2014 to 2015 to the nearest
tenth of a percent?
A) 1.5%
B) 1.6%
C) 1.7%
D) 1.8%
E) 2.0%
E
Answers
The percentage change of the population is P = 1.7 %
Given data ,
To calculate the percent increase from 2014 to 2015, we need to find the difference between the two population values, divide it by the initial value, and then multiply by 100 to get the percentage.
Population increase = 7,375 - 7,250 = 125
Percent increase = (Population increase / Initial population) * 100
Percent increase = (125 / 7,250) * 100 ≈ 1.7241
Rounding to the nearest tenth of a percent, the percent increase is approximately 1.7%.
Hence , the percentage change is P = 1.7 %
To learn more about percentage click :
https://brainly.com/question/12861068
#SPJ1
c(x)=2x^3+3x^2-1 find rational zeros
Answers
Answer:
1/2
Step-by-step explanation:
The Rational Root Theorem says that any rational root has
1. a numerator that is a factor of the constant term
2. a denominator that is a factor of the leading coefficient (that's attached to the highest-power term)
Numerator: \(\pm 1\)
Denominator: \(\pm 2\)
That means there are only two possible rational roots: \(\pm \frac{1}{2}\)
Try them both by plugging them into the polynomial.
\(2\left(\frac{1}{2}\right)^3 + 3\left(\frac{1}{2}\right)^2 -1= \frac{1}{4}+\frac{3}{4}-1 =0\)
Aha! The negative one-half value does not produce 0
(y3)2 · y7 find the product
Answers
Answer:
2y^(10)
Step-by-step explanation:
Answer:
y^13
Step-by-step explanation:
Viel
Eram
back
A movie theater has 4 groups of seats. The largest group
of seats, in the middle, has 21 rows, with 21 seats in each
row. There are 2 smaller groups of seats on the sides, each
with 21 rows and 7 seats in each row. A group of seats in
the back has 6 rows, with 33 seats in each row.
Step by s
How many seats are in the movie theater
Textbod
side
middle
side
Print
Part 1 out of 6
Complete the explanation for what you need to know.
of the movie theater.
You need to find the number of sections that are in each row
Check
Next
Tuin
Se
and Close
Oo
Answers
Answer:
933 seats
Step-by-step explanation:
Given that :
4 different group of seats are in a movie theater :
The middle group ; 2 side groups and the back group
Number of seats in the movie theater :
THE MIDDLE GROUP:
21 Rows and 21 seats per row
Number of seats = 21 * 21 = 441 seats
THE SIDE GROUPS:
21 rows and 7 seats per row
Number of seats = 2(21 * 7) = 294 seats
BACK GROUP:
6 rows and 33 seats per row
Number of seats = 6 * 33 = 198 seats
Total number of seats :
441 + 294 + 198 = 933 seats
Contrast the mountains that form at different plate boundaries.
6th science (its not math really)
Answers
When two continental plates collide along a convergent plate boundary from a mountain because both plates share the same mass and thickness.
How can tectonic plate theory form mountains?Due to the weight and thickness of the two continental plates being equal during the collision, no plate will sink into the other, which causes mountain development.
Rocks are pushed up to a mountain range as a result of their collision, as opposed to crumpling and folding. A taller mountain will be produced as plate tectonics continue.
The level of collision affects the notion of plate tectonics; continuing collision results in a higher mountain.
Consequently, a mountain is the result of a tectonic plate collision along a convergent border.
To know more about Plate tectonic theory:
https://brainly.com/question/29774532
#SPJ1
Please look at the photo. Thank you!

Answers
The equations of the composite functions are (h o h)(x) = (x² + 3)² + 3 and (f o f)(x) = x
How to calculate the composite functionsFrom the question, we have the following equations that can be used in our computation:
h(x) = x² + 3
f(x) = 3/4x
From the above, we have
(h o h)(x) = h(h(x))
So, we have
(h o h)(x) = (x² + 3)² + 3
Also, we have
(f o f)(x) = 3/[4(3/4x)]
Evaluate
(f o f)(x) = x
Hence, the composite functions are (h o h)(x) = (x² + 3)² + 3 and (f o f)(x) = x
Read more about composite functions at
brainly.com/question/33401202
#SPJ1
The
exact solusion of the IVP

Answers
Answer:
\(\displaystyle y = \sqrt{2}e^{\frac{1}{2} ln|2 + x^2|}\)
General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
Brackets Parenthesis Exponents Multiplication Division Addition Subtraction Left to RightEquality Properties
Multiplication Property of Equality Division Property of Equality Addition Property of Equality Subtraction Property of EqualityAlgebra I
|Absolute Value|FunctionsFunction NotationExponential Rule [Multiplying]: \(\displaystyle b^m \cdot b^n = b^{m + n}\)Algebra II
Logarithms and Natural LogsEuler's number eCalculus
Derivatives
Derivative Notation
Derivative of a constant is 0
Differential Equations
Separation of VariablesAntiderivatives - Integrals
Integration Constant C
Integration Property [Multiplied Constant]: \(\displaystyle \int {cf(x)} \, dx = c \int {f(x)} \, dx\)
U-Substitution
Logarithmic Integration
Step-by-step explanation:
Step 1: Define
Identify
\(\displaystyle y' = \frac{xy}{2 + x^2}\)
\(\displaystyle y(0) = 2\)
Step 2: Rewrite
Separation of Variables
Rewrite Derivative Notation: \(\displaystyle \frac{dy}{dx} = \frac{xy}{2 + x^2}\)[Division Property of Equality] Isolate y's: \(\displaystyle \frac{1}{y} \frac{dy}{dx} = \frac{x}{2 + x^2}\)[Multiplication Property of Equality] Rewrite Derivative Notation: \(\displaystyle \frac{1}{y} dy = \frac{x}{2 + x^2} dx\)Step 3: Find General Solution Pt. 1
Integration
[Equality Property] Integrate both sides: \(\displaystyle \int {\frac{1}{y}} \, dy = \int {\frac{x}{2 + x^2}} \, dx\)[1st Integral] Integrate [Logarithmic Integration]: \(\displaystyle ln|y| = \int {\frac{x}{2 + x^2}} \, dx\)Step 4: Identify Variables
Identify variables for u-substitution for 2nd integral.
u = 2 + x²
du = 2xdx
Step 5: Find General Solution Pt. 2
[2nd Integral] Rewrite [Integration Property - Multiplied Constant]: \(\displaystyle ln|y| = \frac{1}{2}\int {\frac{2x}{2 + x^2}} \, dx\)[2nd Integral] U-Substitution: \(\displaystyle ln|y| = \frac{1}{2}\int {\frac{1}{u}} \, du\)[2nd Integral] Integrate [Logarithmic Integration]: \(\displaystyle ln|y| = \frac{1}{2} ln|u| + C\)[Equality Property] e both sides: \(\displaystyle e^{ln|y|} = e^{\frac{1}{2} ln|u| + C}\)Simplify: \(\displaystyle |y| = e^{\frac{1}{2} ln|u| + C}\)Rewrite [Exponential Rule - Multiplying]: \(\displaystyle |y| = e^{\frac{1}{2} ln|u|} \cdot e^C\)Simplify: \(\displaystyle |y| = Ce^{\frac{1}{2} ln|u|}\)Back-Substitute: \(\displaystyle |y| = Ce^{\frac{1}{2} ln|2 + x^2|}\)Our general solution is \(\displaystyle |y| = Ce^{\frac{1}{2} ln|2 + x^2|}\).
Step 6: Find Particular Solution
Substitute in point: \(\displaystyle |2| = Ce^{\frac{1}{2} ln|2 + 0^2|}\)Evaluate |Absolute Value|: \(\displaystyle 2 = Ce^{\frac{1}{2} ln|2 + 0^2|}\)|Absolute Value| Evaluate exponents: \(\displaystyle 2 = Ce^{\frac{1}{2} ln|2 + 0|}\)|Absolute Value| Add: \(\displaystyle 2 = Ce^{\frac{1}{2} ln|2|}\)|Absolute Value| Evaluate: \(\displaystyle 2 = Ce^{\frac{1}{2} ln(2)}\)[Division Property of Equality] Isolate C: \(\displaystyle \sqrt{2} = C\)Rewrite: \(\displaystyle C = \sqrt{2}\)Substitute in C [General Solution]: \(\displaystyle y = \sqrt{2}e^{\frac{1}{2} ln|2 + x^2|}\)∴ Our particular solution is \(\displaystyle y = \sqrt{2}e^{\frac{1}{2} ln|2 + x^2|}\).
Topic: AP Calculus AB/BC (Calculus I/II)
Unit: Differential Equations
Book: College Calculus 10e
What is the Surface area

Answers
Answer:
surface area =(p+q)/2×h
(8m+3m)/2×6m
11/2×6
5.5m×6m
33m^2
Millie Moo the cow can produce 14
gallons of milk in 3 days. How many gallons
of milk does Millie produce each day?
Round to the nearest hundredth if
necessary.
Answers
Identify all the lines of symmetry of the following rectangle.
Explain, in your own words, how you found the lines

Answers
The triangle presented in the image has 2 axes of symmetry because it is only possible to divide it 2 times.
What are axes of symmetry?The axes of symmetry are a concept of geometry that are classified into two groups:
The plane axis of symmetry: This is an imaginary line that divides a figure into two parts, ensuring that the symmetrical points are at the same distance from it.The axis of axial symmetry: This is the symmetry around an axis, it is a point of translation and rotation in which all the semi-planes taken from a certain perpendicular bisector have identical characteristics.According to the above, it can be inferred that the rectangle presented in the image has only two axes of symmetry.
Learn more about symmetry in: https://brainly.com/question/17759737
#SPJ1
Answer: the verified answer is wrong
Step-by-step explanation:
it's not a triangle is a rectangle but it still has 2 axes tho