Answers
Answer:
\(f^{-1}x=\frac{2x}{x-1}\)
Related Questions
what is the distance of the ramp in feet? in the picture and please help answer the question below !!!

Answers
sin 35 =5/x
0.57=5/x
X= 8.77
Answer:
Option 2) Sin 35 = \(\frac{5}{x}\)
Step-by-step explanation:
Sin 35 = \(\frac{opposite }{hypotenuse}\)
Where opposite = 5' and hypotenuse = x(unknown)
=> Sin 35 = \(\frac{5}{x}\)
why do you get different answers for -5^2 and (-5)^2. And why is one positive (33) and one negative (-18) !!
Answers
Answers to both the given expressions are the same that is 25 and both answers are positive.
What are exponents?The number of times a number is multiplied by itself is referred to as its exponent. For instance, 2 to the third (written as 23) means: 2 x 2 x 2 = 8. 2 x 3 = 6 does not equal 23.Product of powers rule — When multiplying like bases, add powers together.When dividing like bases, apply the power quotient rule.Power of powers rule — When raising power by another exponent, multiply the powers together.So,
-5² = -5 × -5 = 25Similarly,
(-5)² = -5² = -5 × -5 = 25Hence, we can say that the answers to both the expressions are the same and both have positive answers.
As, when we take a square of any negative number, after the multiplication the results always come positive.Therefore, the answers to both the given expressions are the same that is 25 and both answers are positive.
Know more about exponents here:
https://brainly.com/question/11975096
#SPJ1
A survey found that the American family generates an average of 17.2 pounds of glass garbage each year. Assume the standard deviation of the distribution is 2.5 pounds. Find the probability that the mean of a sample of 40 families will be between 17.1 and 18.1 pounds. Assume that the sample is taken from a large population and the correction factor can be ignored. Round your final
answer to four decimal places and intermediate z-value calculations to two decimal places

Answers
The probability that the mean of a sample of 40 families will be between 17.1 and 18.1 pounds is given as follows:
0.5874 = 58.74%.
How to obtain probabilities using the normal distribution?The z-score of a measure X of a variable that has mean symbolized by \(\mu\) and standard deviation symbolized by \(\sigma\) is obtained by the rule presented as follows:
\(Z = \frac{X - \mu}{\sigma}\)
The z-score represents how many standard deviations the measure X is above or below the mean of the distribution, depending if the obtained z-score is positive or negative.Using the z-score table, the p-value associated with the calculated z-score is found, and it represents the percentile of the measure X in the distribution.By the Central Limit Theorem, the sampling distribution of sample means of size n has standard deviation \(s = \frac{\sigma}{\sqrt{n}}\).The parameters for this problem are given as follows:
\(\mu = 17.2, \sigma = 2.5, n = 40, s = \frac{2.5}{\sqrt{40}} = 0.3953\)
The probability that the mean of a sample of 40 families will be between 17.1 and 18.1 pounds is the p-value of Z when X = 18.1 subtracted by the p-value of Z when X = 17.1, hence:
\(Z = \frac{X - \mu}{\sigma}\)
By the Central Limit Theorem:
\(Z = \frac{X - \mu}{s}\)
Z = (18.1 - 17.2)/0.3953
Z = 2.28
Z = 2.28 has a p-value of 0.9887.
Z = (17.1 - 17.2)/0.3953
Z = -0.25
Z = -0.25 has a p-value of 0.4013.
Hence:
0.9887 - 0.4013 = 0.5874 = 58.74%.
More can be learned about the normal distribution at https://brainly.com/question/25800303
#SPJ1
b) A white shirt costs $. 2.50, and a blue shirt costs $. 1.50
Calculate the percentage difference.
Answers
Cost of white shirt = 2.50 $
Cost of blue shirt = 1.50 $
We have asked to find percentage difference\(.\)
_________________________________
\(:\implies\sf\:\dfrac{cost_{blue}-cost_{white}}{cost_{blue}+cost_{white}}\times 100\)
\(:\implies\sf\:\dfrac{2.50-1.50}{2.50+1.50}\times 100\)
\(:\implies\sf\:\dfrac{1}{4}\times 100\)
\(:\implies\:\boxed{\bf{\gray{25\:\%}}}\)
Deion was the lucky journalist assigned to cover the Best Beard Competition. He recorded the contestants' beard colors in his notepad. Deion also noted if the contestants were signed up for the mustache competition later in the day. Dark beard Light beard Only in the beard competition 4 3 Also in the mustache competition 3 2 What is the probability that a randomly selected contestant is only in the beard competition and has a light beard?
Answers
$P(\text{Light beard and only in beard competition}) = \frac{3}{10}$
So, the probability of a randomly selected contestant being only in the beard competition and having a light beard is $\frac{3}{10}$.
Question
From a point P on a level ground and directly west of a pole, the angle of elevation of the top of the pole is 45° and from point Q east of the pole, the angle of elevation of the top of the pole is 58°. If |PQ|= 10m, calculate, correct to 2 significant figures, the:
a) distance from P to the pole;
b) height of the pole.

Answers
a) The distance from point P to the pole is: 6.2 m
b) The height of the Pole is: 6.2 m
How to find the distance and height from angle of elevation?The triangle attached shows us the triangle formed as a result of the given word problem about angle of elevation and distance and height.
Now, we are given that:
The angle of elevation of the top of the pole = 45°
Angle of elevation of the top of the pole = 58°.
|PQ|= 10m
a) PR is distance from point P to the pole and using trigonometric ratios, gives us:
PR/sin 58 = 10/sin(180 - 58 - 45)
PR/sin 58 = 10/sin 77
PR = (10 * sin 58)/sin 77
PR = 8.7 m
b) P O can be calculated with trigonometric ratios as:
P O = PR * cos 45
P O = 8.7 * 0.7071
P O = 6.2 m
Now, the two sides of the isosceles triangle formed are equal and as such:
R O = P O
Thus, height of pole R O = 6.2 m
Read more about distance from angle of elevation at: https://brainly.com/question/25748640
#SPJ1

Question 2 of 10
Which of the following are equal to the expression below? Check all that
apply.
√9/√81
A. 3/9
B. 2/9
C. 3
D. 1/3

Answers
Answer:
A. 3/9 and D. 1/3
Step-by-step explanation:
First, you need to find the square roots of the fraction given.
√9=3 √81=9
Now it's 3/9.
This automatically gives you option A. Now, if you simplify 3/9, you get 1/3.
Hope this helps you out!! :)
What is the result when -6x - 4 is subtracted from -3x + 8?
Answers
Answer:
-3x+4
Step-by-step explanation:
Find the slope of the line through each pair of points.
(4.-4). (14, -13)
Answers
Answer:
X2 -X1 / Y2 - Y1
Step-by-step explanation:
14- 4 / (-13) - (-4)
10/-9
The function f(1) = 60,000(2)
00(2) 410 gives the number
of bacteria in a population & minutes after an initial
observation. How much time, in minutes, does it
take for the number of bacteria in the population to
double?
Answers
It takes 10 minutes for the number of bacteria in the population to double.
To determine the time it takes for the number of bacteria in a population to double, we need to find the value of t when the function f(t) equals twice the initial number of bacteria.
The given function is f(t) = 60,000 * 2^(t/10).
To find the time it takes for the number of bacteria to double, we set f(t) equal to twice the initial number of bacteria, which is 2 * 60,000 = 120,000:
120,000 = 60,000 * 2^(t/10).
Next, we can simplify the equation by dividing both sides by 60,000:
2 = 2^(t/10).
Since both sides of the equation have the same base (2), we can equate the exponents:
t/10 = 1.
To solve for t, we multiply both sides by 10:
t = 10.
Therefore, it takes 10 minutes for the number of bacteria in the population to double.
This result is obtained by setting the growth rate of the bacteria population in the given function. The exponent t/10 determines the rate of growth, and when t is equal to 10, the exponent becomes 1, resulting in a doubling of the initial number of bacteria.
For more scuh questions on population visit:
https://brainly.com/question/31243583
#SPJ8
Raquel Drives 24 3/4 hour per week.Kim drives 15 1/6 hours per week. How many more hours Does Raquel drive then Kim 
Answers
Answer:
24 3/4 - 15 1/6
Step-by-step explanation:
1/6 x 4 = 4/24 3/4 x 6 = 18/24
24-15 = 9
9 14/24 or 9 7/12
Divide 2,756 into 1,375,244
Answers
Step-by-step explanation:
499
im not sure if correct ,
Ira’s Craft and Hobby Shoppe sells the items shown below. (AKA the image that I put) London has $7.00. She buys one of each item. How much money does she have left?
A. $4.90
B. $4.10
C. $3.10
D $2.10
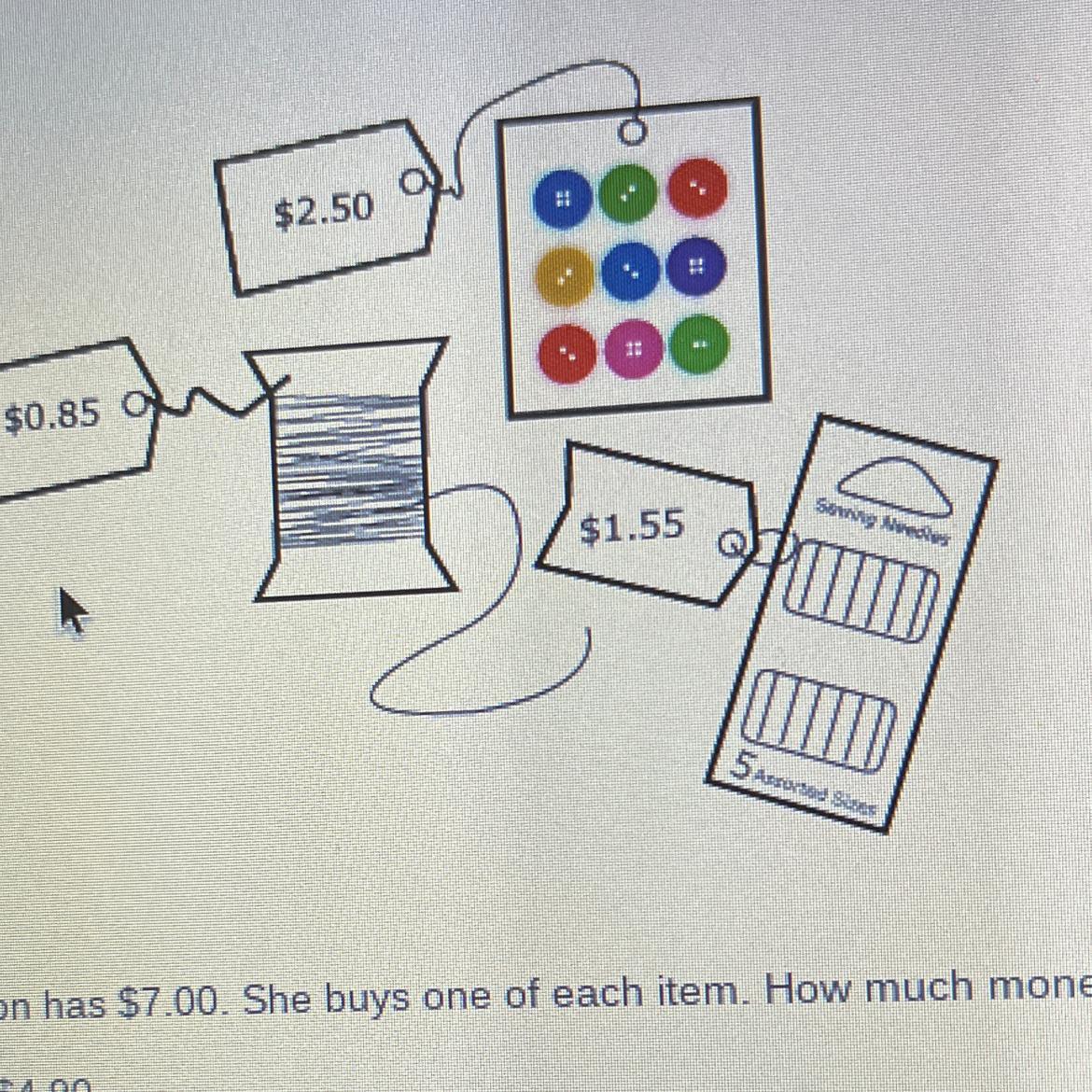
Answers
Answer:
A. $4.90
Step-by-step explanation:
Lightfoot Inc., a software development firm, has stock outstanding as follows: 20,000 shares of cumulative preferred 2% stock, $20 par, and 25,000 shares of $100 par common. During its first four years of operations, the following amounts were distributed as dividends: first year, $3,000; second year, $5,000; third year, $34,500; fourth year, $71,000.
Answers
The amount of Dividends of $3,000, $5,000, $34,500, and $71,000 were distributed to the 20,000 preferred and 25,000 common shareholders of Lightfoot Inc. over the first four years of operations.
To calculate the dividend per share of the preferred and common stock of Lightfoot Inc., the total amount of dividends paid out over the first four years must first be determined. This can be done by adding the given amounts of $3,000, $5,000, $34,500, and $71,000 to get a total of $113,500. To find the dividend per share for the preferred stock, the total dividend is divided by the number of shares (20,000) which gives a dividend per share of $5.68. To find the dividend per share for the common stock, the total dividend is divided by the number of shares (25,000) which gives a dividend per share of $4.54.
Learn more about amount here
https://brainly.com/question/8082054
#SPJ1
Paul is selling $2 raffle tickets for his baseball team. He needs to sell at least
$230 worth of tickets to earn a team jacket. If he has already sold $50 worth of tickets, how many more raffle tickets, x, does Paul need to sell to earn a team jacket?
Answers
Answer: 90 more tickets
Step-by-step explanation:
230 - 50 = 180
180 divided by 2 = 90 tickets
An arrow is shot from 3 ft above the top of a hill with a vertical upward velocity of 108 ft/s. If it strikes the plain below after 9.5 s, how high is the hill?
If the arrow is launched at t0, then write an equation describing velocity as a function of time?
Answers
The height of the hill is approximately 25.73 ft. Where v0 is the initial velocity (108 ft/s), g is the acceleration due to gravity \((-32.2 ft/s^2)\),
To find the height of the hill, we can use the formula for the vertical position of an object under constant acceleration:
h = h0 + v0t + 1/2at^2
where h is the final height, h0 is the initial height, v0 is the initial velocity, t is the time, and a is the acceleration due to gravity (-32.2 ft/s^2).
In this case, we are given that the initial height h0 is 3 ft, the initial velocity v0 is 108 ft/s, and the time t is 9.5 s. We want to find the height of the hill, which we can denote as h_hill. The final height is the height of the plain, which we can denote as h_plain and assume is zero.
At the highest point of its trajectory, the arrow will have zero vertical velocity, since it will have stopped rising and just started to fall. So we can set the velocity to zero and solve for the time it takes for that to occur. Using the formula for velocity under constant acceleration:
v = v0 + at
we can solve for t when v = 0, h0 = 3 ft, v0 = 108 ft/s, and a = -32.2 ft/s^2:
0 = 108 - 32.2t
t = 108/32.2 ≈ 3.35 s
Thus, it takes the arrow approximately 3.35 s to reach the top of its trajectory.
Using the formula for the height of an object at a given time, we can find the height of the hill by subtracting the height of the arrow at the top of its trajectory from the initial height:
h_hill = h0 + v0t + 1/2at^2 - h_top
where h_top is the height of the arrow at the top of its trajectory. We can find h_top using the formula for the height of an object at the maximum height of its trajectory:
h_top = h0 + v0^2/2a
Plugging in the given values, we get:
h_top = 3 + (108^2)/(2*(-32.2)) ≈ 196.78 ft
Plugging this into the first equation, we get:
h_hill = 3 + 108(3.35) + 1/2(-32.2)(3.35)^2 - 196.78
h_hill ≈ 25.73 ft
If the arrow is launched at t0, the equation describing velocity as a function of time would be:
v(t) = v0 - gt
For such more questions on velocity
https://brainly.com/question/25749514
#SPJ8
Jonathan plays on a basketball team. In two games he scored 2/5 of the total number of points his team scored. His team scored 55 points in the first game and 35 points in the second game. What was the number of points Jonathan scored in these two games?
A 18
B 20
C 36
D 90
Answers
Answer:
36 points. Option C
Step-by-step explanation:
Jonathan scored 2/5th of the points of which his team had scored.
His team in the 1st game scored 55 points and 35 in the second.
The team combinedly scored 55+35=90 points.
Jonathan scored 2/5th of the 90 points
\(\frac{2}{5}\) * 90= \(\frac{180}{5}\)
= 36 points.
I need help question
Answers
We want to find the derivative of
\(f(x)=11\sin x+4\cos x\)To solve that we must remember the derivative of sin and cos
\(\begin{gathered} \frac{d}{dx}(\sin x)=\cos x \\ \\ \frac{d}{dx}(\cos x)=-\sin x \end{gathered}\)Therefore, let's use it to find f'(x)
\(\begin{gathered} f^{\prime}(x)=[11\sin(x)+4\cos(x)\rbrack^{\prime} \\ \\ f^{\prime}(x)=[11\sin(x)\rbrack^{\prime}+\lbrack4\cos(x)\rbrack^{\prime} \\ \\ f^{\prime}(x)=11\cdot[\sin(x)\rbrack^{\prime}+4\cdot\lbrack\cos(x)\rbrack^{\prime} \\ \\ f^{\prime}(x)=11\cdot\cos x-4\sin x \end{gathered}\)Therefore
\(f^{\prime}(x)=11\cos x-4\sin x\)Now let's evaluate the derivative at the point 3π/4
\(\begin{gathered} f^{\prime}(3\pi/4)=11\cos\frac{3\pi}{4}-4\sin\frac{3\pi}{4} \\ \\ f^{\prime}(3\pi/4)=11(-\sqrt{2}/2)-4\sqrt{2}/2 \\ \\ f^{\prime}(3\pi/4)=-\frac{11\sqrt{2}}{2}-\frac{4\sqrt{2}}{2} \\ \\ f^{\prime}(3\pi/4)=-\frac{1}{2}(11\sqrt{2}+4\sqrt{2}) \\ \\ f^{\prime}(3\pi/4)=-\frac{1}{2}(15\sqrt{2}) \\ \\ f^{\prime}(3\pi/4)=-\frac{15\sqrt{2}}{2} \end{gathered}\)Hence
\(f^{\prime}(3\pi/4)=-\frac{15\sqrt{2}}{2}\)Trig ratios, pls hurry

Answers
The person is 36m away from the boat.
Applying simultaneous equations to solve angle of depressionThe given figure is triangular in nature. In order to determine the distance of the person from the boat, we will use the pythagoras theorem.
Given the following parameters
GH = 20m
GK = 30m
The measure of the length HK (The distance of the person from the boat,) is calculated as:
HK^2 = GH^2 + GK^2
HK^2 = 20^2+ 30^2
HK^2 = 400+900
HK^2 = 1300
HK = 36m
Hence the distance of the person from the boat is approximately 36m
Learn more on angle of depression here: https://brainly.com/question/17193804
#SPJ1
4x3 = -5x - 21 solve for x
Answers
To solve for x in the equation 4x3 = -5x - 21, we can follow these steps:
1. Move all the terms containing x to one side of the equation, and move the constant term to the other side. We can do this by adding 5x to both sides and then adding 21 to both sides:
4x3 + 5x = -21
2. Factor out x from the left-hand side of the equation:
x(4x2 + 5) = -21
3. Divide both sides by (4x2 + 5):
x = -21 / (4x2 + 5)
So the solution for x is x = -21 / (4x2 + 5). Note that this is a rational function, which means that the value of x depends on the value of the variable x. This equation has no real solutions because the denominator is always positive, and the numerator is negative.
Question 2
No calculations are necessary to answer this question.
3/01
3/02
$1.7420 $1.7360
Date
July GBP Futures
Contract Price
O long; long
Based on the closing prices of July GBP Futures Contract over the 3-day period in March 20XX as shown above, you shou
position on 3/01 and a position on 3/02.
O long; short
O short; short
3/03
short; long
$1.7390
Answers
The given information does not provide any clear indication for determining the position that should be taken on 3/01 and 3/02. Without additional information, it is not possible to make a decision. The table only displays the closing prices of the July GBP Futures Contract on different days, and it is unclear what trading strategy or what scenario is being considered. Additional information about the goals and objectives, the market conditions, and other relevant factors would be necessary to make a decision about trading positions.
Please explain how to do the equation to get 10 units.

Answers
9514 1404 393
Answer:
the equation comes from setting expressions for the perimeters equal to each other
Step-by-step explanation:
Each of the relations is described in terms of the shortest side of the triangle, which is what we're asked to find. It makes sense to let a variable represent that length.
shortest triangle side: s
each of the other two sides: s+1
perimeter of the triangles: s +(s+1) +(s+1) = 3s+2
__
side length of square: s-2
perimeter of square : 4(s -2) = 4s -8
__
The two perimeters are equal, so we have ...
3s +2 = 4s -8 . . . . . your equation
10 = s . . . . . . . . . . . add 8-3s
Solve and check the following equations. Show your solution.
9.) 12 - 3x = 22 + 2x
10.) x + 7x - 12 = -20
11.) 7x + 4 - 13x = -1 + 23
Answers
Answer:
9. -2
10. -1
11. -3
Step-by-step explanation:
9. 12-3X=22+2X
Collect like terms
12-22=2X+3X
-10=5X
Divide both side by 5
X= -10/5 = -2
10. X+7X-12= -20
Collect like terms
X+7X = -20+12
8X= -8
Divide both side by 8
X= -8/8 = -1
11. 7X+4-13X= -1+23
Collect like terms
-7X-13X = -1+23-4
-6X = 18
Divide both side by -6
X= 18/-6 = -3
You invested $18,000 in two accounts paying 7% and 9% annual interest, respectively. If the total interest earned for the year was $1560, how much was invested at each rate?
Answers
hi
Let call money invested at 7% "X " and money at 9 % "Y" .
7% = 7/100 = 0.07
9% = 9 /100 = 0.09
So X+Y = 18 000
0.07X + 0.09Y = 1560
So if : X+Y = 18 000
Y = 18 000 -X
then
0.07X + 0.09 ( 18 000 -X ) = 1560
0.07X +0.09 * 18 000 - 0.09X = 1560
0.07X + 1620 -0.09X = 1560
0.07X-0.09X = 1560-1620
-0.02X = -60
X = -60 /-0.02
X = 3 000
If X = 3 000 then Y = 18 000 -3 000 = 15 000
Conclusion : 3 000 dollars were invested at 7 % and 15 000 at 9%
0.07* 3 000 = 210
15 000* 0.09 =1350
Total = 210+1350 = 1 560
The formulas for total revenue and total cost, in hundreds of dollars, for selling and producing q hundred Items are: total revenue: TR(q) = 30q total cost: Tc(q) = q^3-15q^2+75q+10. (a) Find the smallest quantity at which marginal cost is equal to 15 dollars per Item. (b) Recall: Fixed cost is given by FC = TC(0). Variable cost is given by VC(q) = TC(q) - FC. Average variable cost is given by AVC(q) = VC(q)/q. Find a positive value of q at which average variable cost is equal to marginal cost. (c) Find the longest interval on which marginal revenue exceeds marginal cost. (d) Recall that profit is given by P(q) = TR(q)-TC(q) and On an interval of quantities where MR(q) < MC(q), profit is decreasing. On an interval of quantities where MR(q) > MC(q), profit is increasing Use the sketch you drew in part (c) to find the quantity at which profit is greatest. What is the maximum value of profit?
Answers
(a). The smallest quantity at which marginal cost is equal to 15 dollars per item is 2.76. (b). The positive value of q at which average variable cost is equal to marginal cost is \(\frac{15}{2}\). (c). The longest interval on which marginal revenue exceeds marginal cost is (1.8377, 8.1622). (d). The profit is increasing at 8.1622 at p(q) is maximum. (e). The maximum value of profit is 78.2455 dollars.
The total revenue: TR(q) = 30q
The total cost: Tc(q) = \(q^3-15 q^2+75 q+10$\)
The change in cost is referred to as the change in the cost of production when there is a need for change in the volume of production.
(a). Marginal cost MC\(=\frac{\partial}{\partial q}(T C)$\)
\($$=3q^2-30 q+75 \text {. }$$\)
According to question.
\($$\begin{aligned}& 3 q^2-30 q+75=15 \\& 3 q^2-30 q+60=0 \\& q^2-10 q+20=0 \\& q= \frac{10 \pm \sqrt{100-80}}{2} \\&= \frac{10 \pm 2 \sqrt{5}}{2}=5 \pm \sqrt{5}\end{aligned}\)
\($$$\therefore \quad q=5+\sqrt{5} \quad$ and $q=5-\sqrt{5}$\)
we have to find smallest quantity.
\($$\therefore \quad q=5-\sqrt{5}=2.76 \text {. }$$\)
b)
\($$\begin{aligned}& F C=T C(0)=10 \\& V C(q)=T C(q)-F C \\& =q^3-15 q^2+75 q . \\& \text { AVC(q) }=\frac{V C(q)}{q}=q^2-15 q+75 .\end{aligned}$$\)
When AVC(q)=MC(q)
\($$\begin{array}{ll}\Rightarrow & q^2-15 q+7 ;=3 q^2-30 q+75 \\\Rightarrow & 2 q^2-15 q=0 .\ \Rightarrow q(2 q-15)=0 . \\\Rightarrow & q=0, \frac{15}{2} \quad \Rightarrow \quad q=\frac{15}{2}\end{array}$$\)
Therefore, the positive value of q at which average variable cost is equal to marginal cost is \(\frac{15}{2}\).
(c).
\(& M R(q)=\frac{d}{d q}(T R)=30 . \\\)
\(& M C(q)=3 q^2-30 q+75 . \\\)
Let MR(a)>MC(q)
\(& \Rightarrow \quad 30 > 3 q^2-30 q+75 \text {. } \\\)
\(& \Rightarrow \quad 3 q^2-3 p q+45 < 0 \\\)
\(& \Rightarrow \quad q^2-10 q+15 < 0 \text {. } \\\)
\(& \because \quad q=\frac{10 \pm \sqrt{100-60}}{2} \Rightarrow q=\frac{10 \pm 2 \sqrt{10}}{2} \\\)
\(& q=(5 \pm \sqrt{10}) \\\)
\(& \Rightarrow \quad(q-5+\sqrt{10})(q-5-\sqrt{10}) < 0 . \\\)
\(& \Rightarrow \(q-5+\sqrt{10}) < 0 \quad \& \quad(q-5-\sqrt{10}) > 0 \text {. } \\\)
\(& \text { or } \quad(q-5+\sqrt{10}) > 0 \quad \& \quad(q-5-\sqrt{10}) < 0 \text {. } \\\)
\(& \therefore \quad \text { other } q < 5-\sqrt{10}=1.8377 ., q > 5+\sqrt{10}=8.1622 \text {. } \\\)
\(& \Rightarrow \quad q < 1.8377 \& q > 0.1622 \text {. } \\\)
\(& \text { or } q > 5-\sqrt{10} \quad \& \quad q < 5+\sqrt{10} \text {. } \\\)
\(& \Rightarrow q > 1.8377 \quad < q < 8.1622 \text {. } \\\)
The Interval is (1.8377, 8.1622).
(d). P(q) =TR(q)-TC(q)
\(& =30 q-\left(q^3-15 q^2+75 q+10\right) \\& =-q^3+15 q^2-75 q-10+30 q \\& =-q^3+15 q^2-45 q-10 .\)
on (1.8377,8.1622). we have to find a point such that p"(q)=0 and p"(q)<0.
\(& p^{\prime}(q)=-3 q^2+30 q-45 \\\)
\(& \Rightarrow \quad-3\left(q^2-10 q+15\right)=0 \\\)
\(& \Rightarrow \quad q^2-10 q+15=0 . \\\)
\(& \quad q=1.8377 \text { and } \quad q=8.1622 . \\\)
\(& \Rightarrow \quad p^{\prime \prime}(q)=-6 q+30 . \\\)
\(& p^{\prime \prime}(1.8377)=-6(1.8377)+30 \\\)
\(&=18.9738 \\\)
\(& p^{\prime \prime}(8.1622)=-6(8.1622)+30 . &=-18.9732 < 0 .\)
at 8.1622, p(q) is maximum.
(e).
Max profit-P(8.1622)
=78.2455 dollars
Therefore, the maximum value of profit is 78.2455 dollars.
For more such questions on Marginal cost
https://brainly.com/question/16027422
#SPJ4

x + 121 = 4x - 20 what is x
Answers
Answer:
x = 47
Step-by-step explanation:
x + 121 = 4x - 20
-3x + 121 = - 20
-3x = -141
x = 47
So, the answer is x = 47
At an amusement park, there were 1,500 adults who entered the park during the 9:00 a.m. hour. During the 10:00 a.m. hour, there was a 75% increase. Each ticket costs $49.95.
What is the expression for the total amount collected during the two hours, and why?
Group of answer choice
49.95(1500+1500(0.75)); The expression is 1,500 for the first hour and 1,500 additional people for the second hour.
49.95(1500+1500(1.75)) ; The expression is 1,500 for the first hour and 1,500 additional people for the second hour.
49.95(1500+1500(0.75)) ; The expression is 1,500 for the first hour and 1,500 plus the additional 75 percent for the second hour.
49.95(1500+1500(1.75)) ; The expression is 1,500 for the first hour and 1,500 plus the additional 75 percent for the second hour.
Answers
At an amusement park, having 1,500 adults who entered the park during the 9:00 a.m. hour. and a 75% increase for the 10:00 a.m. hour, with each ticket costs at $49.95 the expression for the total amount collected during the two hours is:
49.95(1500+1500(1.75)) ; The expression is 1,500 for the first hour and 1,500 plus the additional 75 percent for the second hour
How to find the required expression for the costThe expression for the cost is computed from the word problem as follows
At an amusement park, there were 1,500 adults who entered the park during the 9:00 a.m. hour.
= 1500
During the 10:00 a.m. hour, there was a 75% increase
= 1500 * 1.75
= 1500(1.75)
If each ticket costs $49.95, the total cost
= $49.9(1500 + 1500(1.75))
Hence, the expression is 1,500 for the first hour and 1,500 plus the additional 75 percent for the second hour
Learn more about writing expressions at:
https://brainly.com/question/4344214
#SPJ1
Coupons driving visits. A store randomly samples 603 shoppers over the course of a year and nds that 142 of them made their visit because of a coupon they'd received in the mail. Construct a 95% con dence interval for the fraction of all shoppers during the year whose visit was because of a coupon they'd received in the mail.
Answers
Answer:
The 95% confidence interval for the proportion of all shoppers during the year whose visit was because of a coupon they'd received in the mail is (0.2016, 0.2694)
Step-by-step explanation:
In a sample with a number n of people surveyed with a probability of a success of \(\pi\), and a confidence level of \(1-\alpha\), we have the following confidence interval of proportions.
\(\pi \pm z\sqrt{\frac{\pi(1-\pi)}{n}}\)
In which
z is the zscore that has a pvalue of \(1 - \frac{\alpha}{2}\).
For this problem, we have that:
\(n = 603, \pi = \frac{142}{603} = 0.2355\)
95% confidence level
So \(\alpha = 0.05\), z is the value of Z that has a pvalue of \(1 - \frac{0.05}{2} = 0.975\), so \(Z = 1.96\).
The lower limit of this interval is:
\(\pi - z\sqrt{\frac{\pi(1-\pi)}{n}} = 0.2355 - 1.96\sqrt{\frac{0.2355*0.7645}{603}} = 0.2016\)
The upper limit of this interval is:
\(\pi + z\sqrt{\frac{\pi(1-\pi)}{n}} = 0.2355 + 1.96\sqrt{\frac{0.2355*0.7645}{603}} = 0.2694\)
The 95% confidence interval for the proportion of all shoppers during the year whose visit was because of a coupon they'd received in the mail is (0.2016, 0.2694)
Solve the system
4x+5x= -27
x+9y= 1
Answers
Answer:
x=-3, y = 4/9
Step-by-step explanation:
This is in equation form.
Suppose that 11% of all steel shafts produced by a certain process are nonconforming but can be reworked (rather than having to be scrapped). Consider a random sample of 200 shafts, and let X denote the number among these that are nonconforming and can be reworked.Required:a. What is the (approximate) probability that X is at most 30?b. What is the (approximate) probability that X is less than 30?c. What is the (approximate) probability that X is between 15 and 25 (inclusive)?
Answers
Answer:
(a) The probability that X is at most 30 is 0.9726.
(b) The probability that X is less than 30 is 0.9554.
(c) The probability that X is between 15 and 25 (inclusive) is 0.7406.
Step-by-step explanation:
We are given that 11% of all steel shafts produced by a certain process are nonconforming but can be reworked. A random sample of 200 shafts is taken.
Let X = the number among these that are nonconforming and can be reworked
The above situation can be represented through binomial distribution such that X ~ Binom(n = 200, p = 0.11).
Here the probability of success is 11% that this much % of all steel shafts produced by a certain process are nonconforming but can be reworked.
Now, here to calculate the probability we will use normal approximation because the sample size if very large(i.e. greater than 30).
So, the new mean of X, \(\mu\) = \(n \times p\) = \(200 \times 0.11\) = 22
and the new standard deviation of X, \(\sigma\) = \(\sqrt{n \times p \times (1-p)}\)
= \(\sqrt{200 \times 0.11 \times (1-0.11)}\)
= 4.42
So, X ~ Normal(\(\mu =22, \sigma^{2} = 4.42^{2}\))
(a) The probability that X is at most 30 is given by = P(X < 30.5) {using continuity correction}
P(X < 30.5) = P( \(\frac{X-\mu}{\sigma}\) < \(\frac{30.5-22}{4.42}\) ) = P(Z < 1.92) = 0.9726
The above probability is calculated by looking at the value of x = 1.92 in the z table which has an area of 0.9726.
(b) The probability that X is less than 30 is given by = P(X \(\leq\) 29.5) {using continuity correction}
P(X \(\leq\) 29.5) = P( \(\frac{X-\mu}{\sigma}\) \(\leq\) \(\frac{29.5-22}{4.42}\) ) = P(Z \(\leq\) 1.70) = 0.9554
The above probability is calculated by looking at the value of x = 1.70 in the z table which has an area of 0.9554.
(c) The probability that X is between 15 and 25 (inclusive) is given by = P(15 \(\leq\) X \(\leq\) 25) = P(X < 25.5) - P(X \(\leq\) 14.5) {using continuity correction}
P(X < 25.5) = P( \(\frac{X-\mu}{\sigma}\) < \(\frac{25.5-22}{4.42}\) ) = P(Z < 0.79) = 0.7852
P(X \(\leq\) 14.5) = P( \(\frac{X-\mu}{\sigma}\) \(\leq\) \(\frac{14.5-22}{4.42}\) ) = P(Z \(\leq\) -1.70) = 1 - P(Z < 1.70)
= 1 - 0.9554 = 0.0446
The above probability is calculated by looking at the value of x = 0.79 and x = 1.70 in the z table which has an area of 0.7852 and 0.9554.
Therefore, P(15 \(\leq\) X \(\leq\) 25) = 0.7852 - 0.0446 = 0.7406.
